
Are all integers closed under addition?
So, integers are closed under addition. So, if we add any two numbers, we get an integer. So, it is closed. Subtraction. 3 – 5 = –2. –2 is an integer. Also, –1 – 0 = –1.
Are the natural numbers closed under addition?
True. We know that, sum of two natural numbers is always natural number. Therefore, natural numbers are closed under addition. Please log in or register to add a comment.
Are fields closed under multiplication and addition?
Yes -- the definition of "field" mandates that it is closed under multiplication and addition. I suspect you meant to ask something else -- judging by your wording, did you mean to ask about showing whether a subset of a field (with the induced arithmetic operations) is a subfield? Feb 25, 2008. #5.
Are whole numbers closed under the operations of addition?
Two whole numbers add up to give another whole number. This is the closure property of the whole numbers. It means that the whole numbers are closed under addition. If a and b are two whole numbers and a + b = c, then c is also a whole number. 3 + 4 = 7 (whole number).
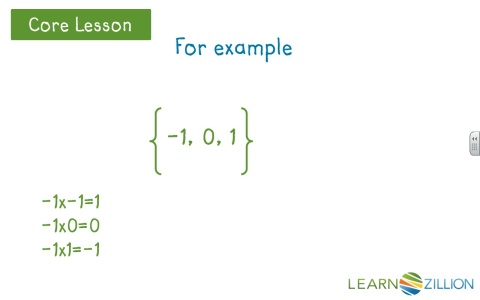
What does it mean for polynomials to be closed under addition?
0:015:51Understand that polynomials are closed under addition - YouTubeYouTubeStart of suggested clipEnd of suggested clipIf i add two polynomials. Together do i always get another polynomial. Is the set of polynomials.MoreIf i add two polynomials. Together do i always get another polynomial. Is the set of polynomials. Closed under addition. In this lesson you will learn that the set of polynomials is closed under
How do you tell if a polynomial is closed?
Closure Property: When something is closed, the output will be the same type of object as the inputs. For instance, adding two integers will output an integer. Adding two polynomials will output a polynomial. Addition, subtraction, and multiplication of integers and polynomials are closed operations.
What is closed under addition?
A set is closed under addition if adding any two numbers from a set produces a number that is still in the set.
How do you prove a polynomial is closed under addition?
TheoremLet (R,+,∘) be a ring.Let (S,+,∘) be a subring of R.be polynomials in the indeterminates {Xj:j∈J} over the ring R.That is, the operation of polynomial addition is closed on the set of all polynomials on a given set of indeterminates {Xj:j∈J}.
Why are polynomials not closed under division?
Since exponents of polynomials are always whole numbers, the exponents will always be in the set (0, 1, 2, 3, etc...). The operation is multiplication, so division by a variable is not possible.
Are Monomials closed under addition?
No. See inside for detailed explanation.
What is not closed under addition?
Answer: ODD INTEGERS. Step-by-step explanation: Odd integers are not closed under addition because you can get an answer that is not odd when you add odd numbers.
How do you know if something is closed under addition?
So a set is closed under addition if the sum of any two elements in the set is also in the set. For example, the real numbers R have a standard binary operation called addition (the familiar one). Then the set of integers Z is closed under addition because the sum of any two integers is an integer.
Which is not closed under the operation addition?
Whole numbers are not closed under operation A Addition class 9 maths CBSE.
Are algebraic numbers closed under addition?
The set of algebraic numbers is closed under addition, subtraction, multiplication and division.
Is the set of polynomial functions closed under division?
Therefore, the set of polynomials is closed under addition, subtraction and multiplication. Division of a polynomial by another polynomial need not be a polynomial (sometimes it is, but not always). Therefore, the set of polynomials is not closed under division.
What is not a polynomial?
So, what cannot be a polynomial? An expression with a variable with negative or fractional exponents, division by a variable, or a variable inside a radical is not a polynomial.
How do you tell whether a set is closed or open?
A set is open if every point in is an interior point. A set is closed if it contains all of its boundary points.
Are polynomials open or closed?
Understand that polynomials form a system analogous to the integers, namely, they are closed under the operations of addition, subtraction, and multiplication; add, subtract, and multiply polynomials.
Is [] open or closed?
The two endpoints are written and separated by a comma using interval notation. A square bracket, [ or ] , is used to indicate a closed interval. Parentheses, ( or ), are used to show an open interval.
How do you determine if a set is closed under multiplication?
The closure property of multiplication states that if a, b are the two numbers that belong to a set M then a × b = c also belongs to the set M. Let a, b ∈ N then a × b = ab ∈ N. Hence, Natural numbers are closed under multiplication.
When will polynomials be closed?
CLOSURE: Polynomials will be closed under an operation if the operation produces another polynomial.
What does polynomial mean?
polynomial- one or more terms: polynomial means "many", but it can also be one term.
What is the degree of a polynomial?
The degree of a polynomial is the highest degree of its terms. Example: 3x2 + 4x + 1 has a degree of 2; x3 - x2 + 5x - 2 has a degree of 3. The standard form of a polynomial is when all like terms are combined and the degrees are arranged in descending order. Polynomial: 2 x + 3 x5 + 4 x3 - 8. Standard form: 3x5 + 4x3 + 2x - 8.
What is a finite sum of terms in which all variables have whole number exponents and no variable appears in?
A polynomialis a finite sum of terms in which all variables have whole number exponents and no variable appears in a denominator.
What is MathBitsNotebook Algebra 1?
MathBitsNotebook Algebra 1 CCSS Lessons and Practice is free site for students (and teachers) studying a first year of high school algebra.
When subtracting polynomials, do the variables and their exponents change?
When subtracting polynomials, the variables and their exponents do not change. Only their coefficients will possibly change. This guarantees that the difference has variables and exponents which are already classified as belonging to polynomials. Polynomials are closed under subtraction.
When are all like terms combined and degrees arranged in descending order?
The standard form of a polynomial is when all like terms are combined and the degrees are arranged in descending order.
