Yes, since each finite sum is continuous, then the uniform convergence of the series implies the continuity of the limit on any compact of R, thus the continuity of the limit sum on R.
When is a sequence of continuous functions uniformly convergent?
A sequence of continuous functions on metric spaces, with the image metric space being complete, is uniformly convergent if and only if it is uniformly Cauchy. which is also continuous, then the convergence is necessarily uniform ( Dini's theorem ).
What is uniform convergence?
We first define uniform convergence for real-valued functions, although the concept is readily generalized to functions mapping to metric spaces and, more generally, uniform spaces (see below ). is a sequence of real-valued functions on it.
What is the difference between uniformly convergent and compactly convergent?
Every locally uniformly convergent sequence is compactly convergent. For locally compact spaces local uniform convergence and compact convergence coincide. A sequence of continuous functions on metric spaces, with the image metric space being complete, is uniformly convergent if and only if it is uniformly Cauchy.
When does pointwise convergence imply uniform convergence?
On an exam question (Question 21H), it is claimed that if K is compact and f n: K → R are continuous functions increasing pointwise to a continuous function f: K → R, then f n converges to f uniformly. I have tried proving this claim for the better part of an hour but I keep coming short.
Does uniform convergence imply continuous?
If a sequence of functions fn(x) defined on D converges uniformly to a function f(x), and if each fn(x) is continuous on D, then the limit function f(x) is also continuous on D.
Does uniform convergence preserve absolute continuity?
No, it does not. Even the set of smooth functions, or the set of polynomials is dense in C0 with respect to uniform convergence, on an interval, say (which is just convergence in the supremums norm). That is, to each continuous function f you will find a sequence fk of smooth functions converging to f uniformly.
Does continuity imply uniform continuity?
Clearly uniform continuity implies continuity but the converse is not always true as seen from Example 1. Therefore f is uniformly continuous on [a, b]. Infact we illustrate that every continuous function on any closed bounded interval is uniformly continuous.
Does uniform convergence imply convergence?
Uniform convergence implies pointwise convergence, but not the other way around. For example, the sequence fn(x)=xn from the previous example converges pointwise on the interval [0,1], but it does not converge uniformly on this interval.
Does uniform convergence imply differentiability?
6 (b): Uniform Convergence does not imply Differentiability. Before we found a sequence of differentiable functions that converged pointwise to the continuous, non-differentiable function f(x) = |x|. Recall: That same sequence also converges uniformly, which we will see by looking at ` || fn - f||D.
Is uniform convergence the same as absolute convergence?
In mathematics, uniform absolute-convergence is a type of convergence for series of functions. Like absolute-convergence, it has the useful property that it is preserved when the order of summation is changed.
What is difference between continuity and uniform continuity?
uniform continuity is a property of a function on a set, whereas continuity is defined for a function in a single point; (b)
How can a function be continuous but not uniformly continuous?
A uniformly continous function is obviously continuous. But the converse is not true. For example, if A = (0,1) and f(x)=1/x, then f is continuous on A, but it is not uniformly continuous on A. The point is that if x is close to zero, then δ needs to be chosen smaller than if x is not close to zero.
How do you prove a continuous function is uniformly continuous?
A function f:(a,b)→R is uniformly continuous if and only if f can be extended to a continuous function ˜f:[a,b]→R (that is, there is a continuous function ˜f:[a,b]→R such that f=˜f∣(a,b))....Answerf(x)=x2 on R.f(x)=sin1x on (0,1).f(x)=ln(x) on (0,∞).
Does pointwise convergence imply continuity?
Although each fn is continuous on [0, 1], their pointwise limit f is not (it is discon- tinuous at 1). Thus, pointwise convergence does not, in general, preserve continuity.
How do you know if a series is continuous?
Theorem 6.2. 1 says that in order for f to be continuous, it is necessary and sufficient that any sequence (xn) converging to a must force the sequence (f(xn)) to converge to f(a).
Does uniform convergence imply L1 convergence?
So pointwise convergence, uniform convergence, and L1 convergence do not imply each other. We do, however, have a few positive results: Theorem 7 If fn → f in L1, then there is a subsequence fnk such that fnk → f pointwise a.e. |g| dµ < ∞ and |fn(x)| ≤ g(x) for all x and n.
Is uniform continuity stronger than continuity?
Uniform continiuty is stronger than continuity, that is, Proposition 1 If f is uniformly continuous on an interval I, then it is continuous on I. Proof: Assume f is uniformly continuous on an interval I. To prove f is continuous at every point on I, let c ∈ I be an arbitrary point. Let ϵ > 0 be arbitrary.
Does uniformly continuous imply Lipschitz?
Any Lipschitz function is uniformly continuous. for all x, y ∈ E. The function f (x) = √x is uniformly continuous on [0,∞) but not Lipschitz.
How do you prove not uniformly continuous?
Theorem: Let E=[a,+∞), function f:E→R is differentiable on E and limx→∞f′(x)=∞. Then f is not a uniformly continuous function.
What is difference between limit and continuity?
The definition of continuity is given with the help of limits as, a function f with variable x is continuous at the point “a” on the real line, if the limit of f(x), when x approaches the point “a”, is equal to the value of f(x) at “a”, that means f(a).
What is uniform convergence?
In the mathematical field of analysis, uniform convergence is a mode of convergence of functions stronger than pointwise convergence. A sequence of functions#N#( f n ) {displaystyle (f_ {n})}#N#converges uniformly to a limiting function#N#f {displaystyle f}#N#on a set#N#E {displaystyle E}#N#if, given any arbitrarily small positive number#N#ϵ {displaystyle epsilon }#N#, a number#N#N {displaystyle N}#N#can be found such that each of the functions#N#f N , f N + 1 , f N + 2 , … {displaystyle f_ {N},f_ {N+1},f_ {N+2},ldots }#N#differ from#N#f {displaystyle f}#N#by no more than#N#ϵ {displaystyle epsilon }#N#at every point#N#x {displaystyle x}#N#in#N#E {displaystyle E}#N#. Described in an informal way, if#N#f n {displaystyle f_ {n}}#N#converges to#N#f {displaystyle f}#N#uniformly, then the rate at which#N#f n ( x ) {displaystyle f_ {n} (x)}#N#approaches#N#f ( x ) {displaystyle f (x)}#N#is "uniform" throughout its domain in the following sense: in order to guarantee that#N#f n ( x ) {displaystyle f_ {n} (x)}#N#falls within a certain distance#N#ϵ {displaystyle epsilon }#N#of#N#f ( x ) {displaystyle f (x)}#N#, we do not need to know the value of#N#x ∈ E {displaystyle xin E}#N#in question — there can be found a single value of#N#N = N ( ϵ ) {displaystyle N=N (epsilon )}#N#independent of#N#x {displaystyle x}#N#, such that choosing#N#n ≥ N {displaystyle ngeq N}#N#will ensure that#N#f n ( x ) {displaystyle f_ {n} (x)}#N#is within#N#ϵ {displaystyle epsilon }#N#of#N#f ( x ) {displaystyle f (x)}#N#for all#N#x ∈ E {displaystyle xin E}#N#. In contrast, pointwise convergence of#N#f n {displaystyle f_ {n}}#N#to#N#f {displaystyle f}#N#merely guarantees that for any#N#x ∈ E {displaystyle xin E}#N#given in advance, we can find#N#N = N ( ϵ , x ) {displaystyle N=N (epsilon ,x)}#N#(#N#N {displaystyle N}#N#can depend on the value of#N#x {displaystyle x}#N#) so that, for that particular#N#x {displaystyle x}#N#,#N#f n ( x ) {displaystyle f_ {n} (x)}#N#falls within#N#ϵ {displaystyle epsilon }#N#of#N#f ( x ) {displaystyle f (x)}#N#whenever#N#n ≥ N {displaystyle ngeq N}#N#.
Who proved that a continuous function is always continuous?
In 1821 Augustin-Louis Cauchy published a proof that a convergent sum of continuous functions is always continuous, to which Niels Henrik Abel in 1826 found purported counterexamples in the context of Fourier series, arguing that Cauchy's proof had to be incorrect. Completely standard notions of convergence did not exist at the time, and Cauchy handled convergence using infinitesimal methods. When put into the modern language, what Cauchy proved is that a uniformly convergent sequence of continuous functions has a continuous limit. The failure of a merely pointwise-convergent limit of continuous functions to converge to a continuous function illustrates the importance of distinguishing between different types of convergence when handling sequences of functions.
How to prove a given inequality?
This theorem is proved by the " ε/3 trick", and is the archetypal example of this trick: to prove a given inequality ( ε ), one uses the definitions of continuity and uniform convergence to produce 3 inequalities ( ε/3 ), and then combines them via the triangle inequality to produce the desired inequality.
Is a sequence of continuous functions on metric space uniformly convergent?
A sequence of continuous functions on metric spaces, with the image metric space being complete, is uniformly convergent if and only if it is uniformly Cauchy.
Can Riemann integrals be exchanged?
Similarly, one often wants to exchange integrals and limit processes. For the Riemann integral, this can be done if uniform convergence is assumed:
Is the uniform limit of a function continuous?
More precisely, this theorem states that the uniform limit of uniformly continuous functions is uniformly continuous; for a locally compact space, continuity is equivalent to local uniform continuity, and thus the uniform limit of continuous functions is continuous.
Who studied the concept of a spherical sphere?
Under the influence of Weierstrass and Bernhard Riemann this concept and related questions were intensely studied at the end of the 19th century by Hermann Hankel, Paul du Bois-Reymond, Ulisse Dini, Cesare Arzelà and others.
Overview
Properties
• Every uniformly convergent sequence is locally uniformly convergent.
• Every locally uniformly convergent sequence is compactly convergent.
• For locally compact spaces local uniform convergence and compact convergence coincide.
History
In 1821 Augustin-Louis Cauchy published a proof that a convergent sum of continuous functions is always continuous, to which Niels Henrik Abel in 1826 found purported counterexamples in the context of Fourier series, arguing that Cauchy's proof had to be incorrect. Completely standard notions of convergence did not exist at the time, and Cauchy handled convergence using infinitesimal methods. When put into the modern language, what Cauchy proved is that a unifor…
Definition
We first define uniform convergence for real-valued functions, although the concept is readily generalized to functions mapping to metric spaces and, more generally, uniform spaces (see below).
Suppose is a set and is a sequence of real-valued functions on it. We say the sequence is uniformly convergent on with limit if for every there exists a natural number such that for all and
The notation for uniform convergence of to is not quite standardized and different authors have u…
Examples
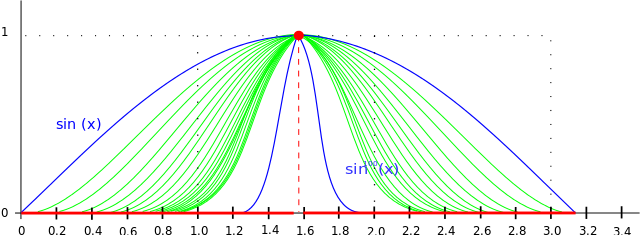
For , a basic example of uniform convergence can be illustrated as follows: the sequence converges uniformly, while does not. Specifically, assume . Each function is less than or equal to when , regardless of the value of . On the other hand, is only less than or equal to at ever increasing values of when values of are selected closer and closer to 1 (explained more in depth further below).
Applications
If and are topological spaces, then it makes sense to talk about the continuity of the functions . If we further assume that is a metric space, then (uniform) convergence of the to is also well defined. The following result states that continuity is preserved by uniform convergence:
This theorem is proved by the "ε/3 trick", and is the archetypal example of this t…
Almost uniform convergence
If the domain of the functions is a measure space E then the related notion of almost uniform convergence can be defined. We say a sequence of functions converges almost uniformly on E if for every there exists a measurable set with measure less than such that the sequence of functions converges uniformly on . In other words, almost uniform convergence means there are sets of arbitrarily small measure for which the sequence of functions converges uniformly on their com…
See also
• Uniform convergence in probability
• Modes of convergence (annotated index)
• Dini's theorem
• Arzelà–Ascoli theorem