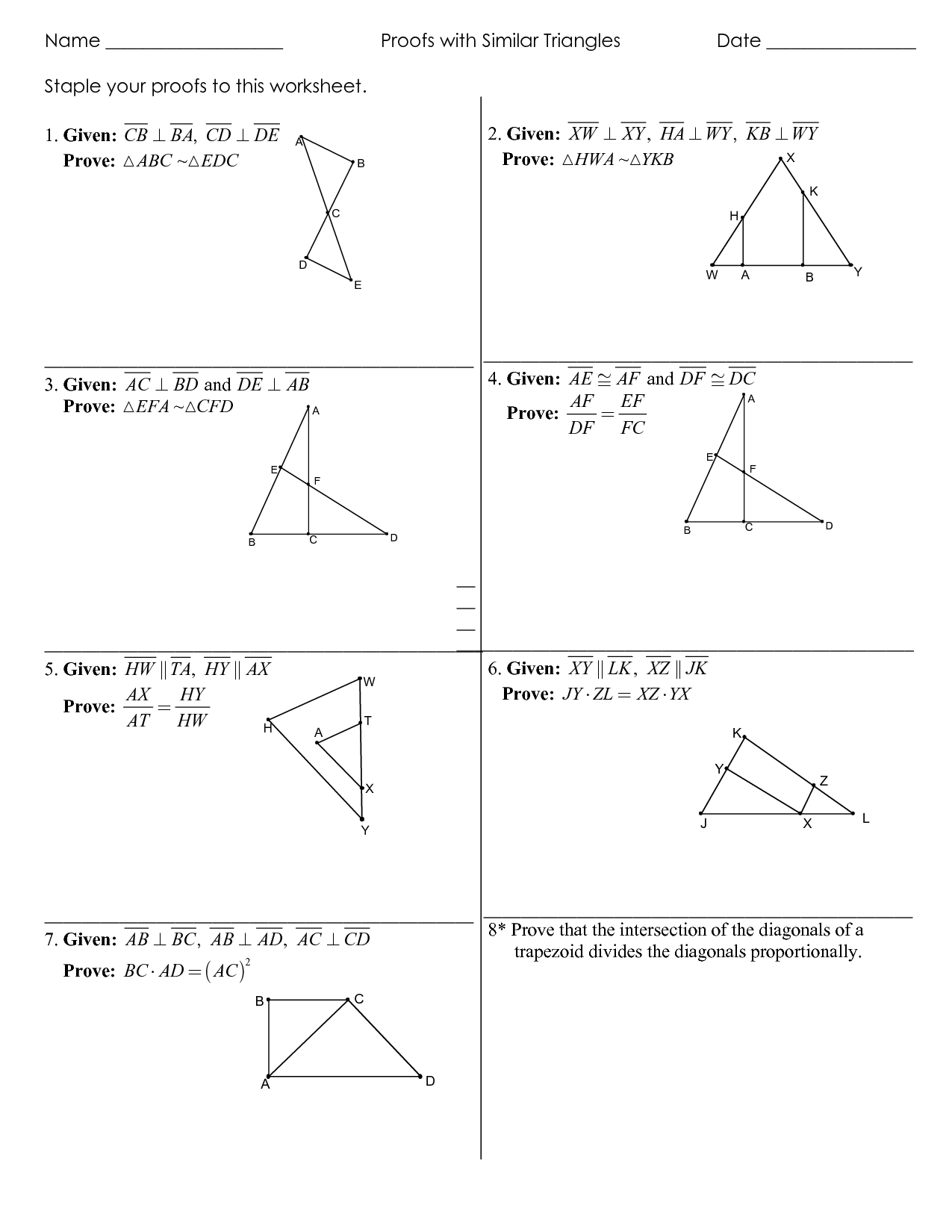
Hypotenuse-Leg Similarity If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar. (You can prove this by using the Pythagorean Theorem
Pythagorean theorem
In mathematics, the Pythagorean theorem, also known as Pythagoras' theorem, is a fundamental relation in Euclidean geometry among the three sides of a right triangle. It states that the square of the hypotenuse (the side opposite the right angle) is equal to the sum of the squares of the other tw…
How to prove the Pythagorean theorem using similar triangles?
So all three triangles are similar, using Angle-Angle-Angle. And we can now use the relationship between sides in similar triangles, to algebraically prove the Pythagorean Theorem.
What are similar triangles in geometry?
Similar Triangles: If the corresponding sides of the given two triangles are proportional and the angles are congruent, then the triangles are considered Similar Triangles. Pythagorean theorem: In a right triangle, the square of the hypotenuse equals the sum of the squares of the other two sides.
What is hypotenuse-leg similarity?
Hypotenuse-Leg Similarity. If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar. (You can prove this by using the Pythagorean Theorem to show that the third pair of sides is also proportional.)
Why do we use similarity in Pythagoras’ theorem?
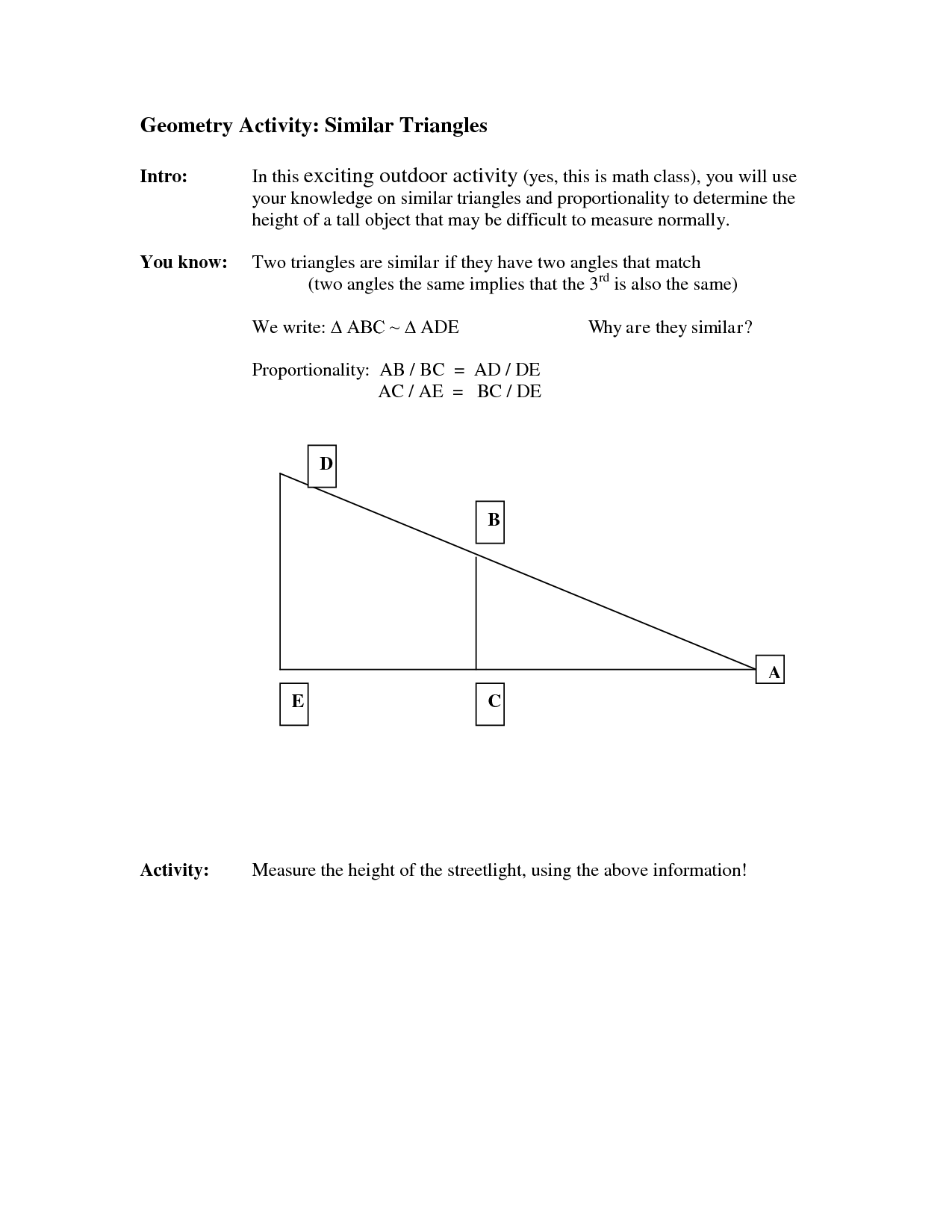
Pythagoras’ Theorem helps us find unknown side length and angles but sometimes the use of similarity is required to find the relevant information. If two shapes are similar they have the same shapes but can be of different sizes or orientation.
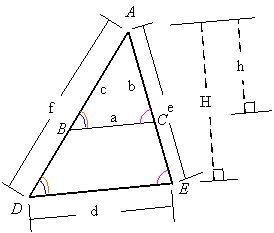
How can you use triangle similarity to prove the Pythagorean Theorem?
Similar Triangles: If the corresponding sides of the given two triangles are proportional and the angles are congruent, then the triangles are considered Similar Triangles. Pythagorean theorem: In a right triangle, the square of the hypotenuse equals the sum of the squares of the other two sides.
What is the connection between similar triangles and right triangles?
If the altitude is drawn to the hypotenuse of a right triangle, then the two triangles formed are similar to the original triangle and to each other.
Why does the Pythagorean Theorem work for right triangles?
0:373:07The Pythagorean Theorem Only Works For Right Triangles - YouTubeYouTubeStart of suggested clipEnd of suggested clipThat will be equal to the two the area of the square made by the hypotenuse. And you can see thatMoreThat will be equal to the two the area of the square made by the hypotenuse. And you can see that that's true so what the Pythagorean theorem would say is a squared plus B squared equals C squared.
Is the Pythagorean Theorem true for all similar triangles?
This is the quintessential proof: with just a single perpendicular line, we have created three similar triangles and discovered that Pythagoras's Theorem must be true. Furthermore, the theorem is true not just for squares, but for any similar shapes on the three sides of the right-angled triangle.
What similarity concept justifies that the two triangles are similar?
SSS or Side-Side-Side Similarity If all the three sides of a triangle are in proportion to the three sides of another triangle, then the two triangles are similar.
How do you prove that two right triangles are similar?
If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar. (You can prove this by using the Pythagorean Theorem to show that the third pair of sides is also proportional.)
Is the Pythagorean Theorem just for right triangles?
Pythagoras' theorem only works for right-angled triangles, so you can use it to test whether a triangle has a right angle or not. In the triangle above, if a 2 < b 2 + c 2 the angle is acute.
Which triangle does the Pythagorean Theorem relate to?
right triangleThe Pythagorean Theorem is a statement relating the lengths of the sides of any right triangle. is equal to the sum of the squares of the other two sides. The theorem has been known in many cultures, by many names, for many years.
What parts of triangle are the most essential in Pythagorean Theorem?
What is the Pythagoras Theorem in Math? The Pythagoras theorem states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of the squares of the other two sides. This theorem can be expressed as, c2 = a2 + b2; where 'c' is the hypotenuse and 'a' and 'b' are the two legs of the triangle.
What do similar triangles have in common?
Similar triangles have the same corresponding angle measures and proportional side lengths. The triangle similarity criteria are: AA (Angle-Angle) SSS (Side-Side-Side)
How would you write the Pythagorean Theorem equation for this right triangle?
0:051:56Pythagorean Theorem | MathHelp.com - YouTubeYouTubeStart of suggested clipEnd of suggested clipOr a squared plus B squared equals C squared where a and B are the lengths of the legs of the rightMoreOr a squared plus B squared equals C squared where a and B are the lengths of the legs of the right triangle.
Is Pythagoras theorem and Pythagorean Theorem same?
The Pythagoras theorem, also known as the Pythagorean theorem, states that the square of the length of the hypotenuse is equal to the sum of squares of the lengths of other two sides of the right-angled triangle.
What is solving problems that involve triangle similarity and right triangle?
Lesson 4: Pythagorean Theorem and Special Right Triangles Solves problems that involve triangles similarity and right triangles.
How do similar right triangles lead to the definitions of the trigonometric ratios?
How do similar right triangles lead to the definitions of the trigonometric ratios? The ratio of two sides of one right triangle is the same of the corresponding sides of any its similar triangles.
Are right triangles similar?
First, right triangles are not necessarily always similar. They must meet the necessary criteria like any other triangles; furthermore, there is no Hypotenuse-Leg Theorem for similarity, only for congruence; therefore, we can eliminate two answer choices.
How are geometric mean and right triangle similar?
5:5716:18Triangles: Similar Right Triangles, Geometric Mean - YouTubeYouTubeStart of suggested clipEnd of suggested clipOkay. So let's go back up here and see if we can find that now according to what we need to now stopMoreOkay. So let's go back up here and see if we can find that now according to what we need to now stop talking about the geometric mean so let's just see what that means no pun intended.
How many copies of a right triangle are there in Pythagorean theorem?
When we introduced the Pythagorean theorem, we proved it in a manner very similar to the way Pythagoras originally proved it, using geometric shifting and rearrangement of 4 identical copies of a right triangle.
Can we use the relationship between sides in similar triangles?
And we can now use the relationship between sides in similar triangles, to algebraically prove the Pythagorean Theorem.
Can we prove the Pythagorean theorem?
Having covered the concept of similar triangles and learning the relationship between their sides, we can now prove the Pythagorean theorem another way, using triangle similarity.
How to tell if two right triangles are similar?
If the lengths of the corresponding legs of two right triangles are proportional, then by Side-Angle-Side Similarity the triangles are similar.
What is the angle similarity of a right triangle?
If one of the acute angles of a right triangle is congruent to an acute angle of another right triangle, then by Angle-Angle Similarity the triangles are similar.
What is the hypotenuse leg similarity?
Hypotenuse-Leg Similarity. If the lengths of the hypotenuse and a leg of a right triangle are proportional to the corresponding parts of another right triangle, then the triangles are similar.
Steps to prove the Pythagorean Theorem Using Similar Triangles
Step 1: Given a right triangle, an altitude drawn from the right-angled vertex divides the hypotenuse into two segments.
Equations & Definitions to prove the Pythagorean Theorem Using Similar Triangles
Similar Triangles: If the corresponding sides of the given two triangles are proportional and the angles are congruent,
Example Problem 1: Prove the Pythagorean Theorem Using Similar Triangles
A right triangle is given with an altitude dividing the hypotenuse into two segments of lengths 10 and 6 units as shown in the diagram below.
Example Problem 2: Prove the Pythagorean Theorem Using Similar Triangles
Given a right triangle ABC, with an altitude dividing the hypotenuse into two segments of lengths 8 and 5 units.