To determine the increasing and decreasing intervals, we use the first-order derivative test to check the sign of the derivative in each interval. The interval is increasing if the value of the function f (x) increases with an increase in the value of x and it is decreasing if f (x) decreases with a decrease in x.
How to tell if a function is increasing or decreasing?
example 1 Determine the intervals on which a function of the form f ( x) = m x + b is increasing or decreasing. The graph of a function of this form is a straight line with slope, m = f ′ ( x). If m > 0, then f ( x) = m x + b is increasing on the interval ( − ∞, ∞) and if m < 0, then it is decreasing on ( − ∞, ∞) .
What is the difference between increasing and decreasing function?
The functions that never decrease, they always increase in value or remain the same (the increasing functions). Similarly, the decreasing functions never increase, they always decrease in value or they remain the same when x becomes bigger.
How to find increasing and decreasing intervals?
Process for finding intervals of increase/decrease
- If possible, factor f ′ . ...
- Find all critical numbers x = c of f.
- Draw a number line with tick marks at each critical number c.
- For each interval (in between the critical number tick marks) in which the function f is defined, pick a number b, and use it to find the sign of the ...
What does increasing interval mean?
The formal definition of an increasing interval is: an open interval on the x axis of (a,d) where every b,c∈(a,d) with b<c has f(b)≤f(c). How do you find the interval of increase? Explanation: To find when a function is increasing, you must first take the derivative, then set it equal to 0, and then find between which zero values the function is positive .
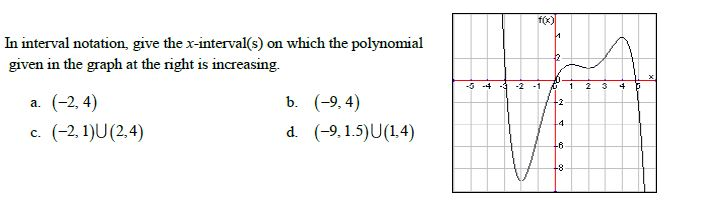
How do you determine the intervals on which a function is decreasing?
Explanation: To find when a function is decreasing, you must first take the derivative, then set it equal to 0, and then find between which zero values the function is negative. Now test values on all sides of these to find when the function is negative, and therefore decreasing.
How do you determine the intervals on which the function is constant?
A function is constant in an interval if it is horizontal in the entire interval.
What is a constant interval?
By definition: A function is constant, if for any x1 and x2 in the interval, f (x1) = f (x2). Example: The graph shown above is constant from the point (-2,1) to the point (1,1), described as constant when -2 < x < 1. The y-values of all points in this interval are "one".
How do you find the interval?
The class interval is the difference between the upper class limit and the lower class limit. For example, the size of the class interval for the first class is 30 – 21 = 9. Similarly, the size of the class interval for the second class is 40 – 31 = 9.
How do you write a constant in interval notation?
1:244:13Interval notation for where functions Increase, Decrease, ConstantYouTubeStart of suggested clipEnd of suggested clipFrom X is negative 2 to X is positive 1 so we're going to say constant I'll just put a C there. AndMoreFrom X is negative 2 to X is positive 1 so we're going to say constant I'll just put a C there. And we're going to go from negative 2 to positive 1 again notice we're using the parenthesis.
What happens when a function decreases?
If the function is decreasing, it has a negative rate of growth. In other words, while the function is decreasing, its slope would be negative. You could name an interval where the function is positive and the slope is negative. The secret is paying attention to the exact words in the question.
Can a function be negative?
Function values can be positive or negative, and they can increase or decrease as the input increases. Here we introduce these basic properties of functions. Google Classroom Facebook Twitter. Email. Intervals where a function is positive, negative, increasing, or decreasing. Increasing, decreasing, positive or negative intervals.
How to find if a function is increasing?
Find the region where the graph goes up from left to right. Use the interval notation.
What is interval notation?
Interval notation: An interval notation is used to represent all the real numbers between two numbers. The notation with round parenthesis {eq} (a, b) {/eq} represents all the real numbers between {eq}a {/eq} and {eq}b {/eq}, not including {eq}a {/eq} or {eq}b {/eq}. When square brackets {eq} [a,b] {/eq} are used, it represent all the real numbers between {eq}a {/eq} and {eq}b {/eq}, including {eq}a {/eq} and {eq}b {/eq}.
What degree does Jiwon have?
Jiwon has a B.S. degree in the mathematics/ science field and over 4 years of tutoring experience. She fell in love with math when she discovered geometry proofs and that calculus can help her describe the world around her like never before.
Where is the flat line on a graph?
There is a flat line in the middle of the graph.
Who is Jenna Feldman?
Jenna Feldman has been a High School Mathematics teacher for ten years. She has worked with students in courses including Algebra, Algebra 2, Precalculus, Geometry, Statistics, and Calculus. She has a bachelor’s degree in mathematics from the University of Delaware and a Master of Education degree from Wesley College.
