How do you tell if data is positively or negatively skewed?
If the mean is greater than the mode, the distribution is positively skewed. If the mean is less than the mode, the distribution is negatively skewed. If the mean is greater than the median, the distribution is positively skewed. If the mean is less than the median, the distribution is negatively skewed.
How do you explain skewness and kurtosis?
Skewness is a measure of symmetry, or more precisely, the lack of symmetry. A distribution, or data set, is symmetric if it looks the same to the left and right of the center point. Kurtosis is a measure of whether the data are heavy-tailed or light-tailed relative to a normal distribution.
What is the purpose of measuring skewness for a dataset?
It measures the deviation of the given distribution of a random variable from a symmetric distribution, such as normal distribution. A normal distribution is without any skewness, as it is symmetrical on both sides.
What does high skewness mean?
Positive Skewness means when the tail on the right side of the distribution is longer or fatter. The mean and median will be greater than the mode. Negative Skewness is when the tail of the left side of the distribution is longer or fatter than the tail on the right side. The mean and median will be less than the mode.
How do you use skewness and kurtosis values to determine normality?
The values for asymmetry and kurtosis between -2 and +2 are considered acceptable in order to prove normal univariate distribution (George & Mallery, 2010). Hair et al. (2010) and Bryne (2010) argued that data is considered to be normal if skewness is between ‐2 to +2 and kurtosis is between ‐7 to +7.
What is acceptable skewness and kurtosis?
Both skew and kurtosis can be analyzed through descriptive statistics. Acceptable values of skewness fall between − 3 and + 3, and kurtosis is appropriate from a range of − 10 to + 10 when utilizing SEM (Brown, 2006).
What is the skewness and kurtosis of a normal distribution?
A normal distribution has skewness and excess kurtosis of 0, so if your distribution is close to those values then it is probably close to normal.
How do you interpret kurtosis in descriptive statistics?
For kurtosis, the general guideline is that if the number is greater than +1, the distribution is too peaked. Likewise, a kurtosis of less than –1 indicates a distribution that is too flat. Distributions exhibiting skewness and/or kurtosis that exceed these guidelines are considered nonnormal." (Hair et al., 2017, p.
What is the difference between skewness and kurtosis?
Skewness and kurtosis are both important measures of a distribution’s shape. Skewness measures the asymmetry of a distribution. Kurtosis measu...
What are the three types of skewness?
The three types of skewness are: Right skew (also called positive skew ) . A right-skewed distribution is longer on the right side of its peak t...
What is a normal distribution?
In a normal distribution , data are symmetrically distributed with no skew. Most values cluster around a central region, with values tapering off...
What is skewed data?
Skewness refers to a distortion or asymmetry that deviates from the symmetrical bell curve, or normal distribution, in a set of data. If the curve is shifted to the left or to the right, it is said to be skewed. Skewness can be quantified as a representation of the extent to which a given distribution varies from a normal distribution.
What Does Skewness Tell You?
Investors note skewness when judging a return distribution because it, like kurtosis, considers the extremes of the data set rather than focusing solely on the average. Short- and medium-term investors in particular need to look at extremes because they are less likely to hold a position long enough to be confident that the average will work itself out.
What is negatively skewed distribution?
Negatively-skewed distributions are also known as left-skewed distributions. Skewness is used along with kurtosis to better judge the likelihood of events falling in the tails of a probability distribution.
What is the difference between a negative skew and a positive skew?
Negative skew refers to a longer or fatter tail on the left side of the distribution, while positive skew refers to a longer or fatter tail on the right. The mean of positively skewed data will be greater than the median. In a distribution that is negatively skewed, the exact opposite is the case: the mean of negatively skewed data will be less ...
How does Pearson's second coefficient of skewness work?
Pearson’s second coefficient of skewness, or Pearson median skewness, subtracts the median from the mean, multiplies the difference by three, and divides the product by the standard deviation.
Why is skewness used in kurtosis?
Skewness is used along with kurtosis to better judge the likelihood of events falling in the tails of a probability distribution.
What is negative skew?
In the curve of a distribution, the data on the right side of the curve may taper differently from the data on the left side. These taperings are known as "tails.". Negative skew refers to a longer or fatter tail on ...
What is skewed data?
Skewed data is data that creates an asymmetrical, skewed curve on a graph. In statistics, the graph of a data set with normal distribution is symmetrical and shaped like a bell. However, skewed data has a "tail" on either side of the graph. The two most common types of skew are:
How to calculate skewness
Here are four key steps that you can follow to calculate the skewness—or amount of skew—in a data set:
Skewed data examples
Here are some real-world examples to help you understand positive and negative skew:
What does skewness mean in statistics?
Skewness is simply how much data set is deviating from its normal distribution. A larger negative value in the data set means that distribution is negatively skewed & larger positive value in the data set means that distribution is positively distributed. It is a good statistical measure that helps the investor to predict returns from ...
What is skewed data?
Skewness describes how much statistical data distribution is asymmetrical from the normal distribution, where distribution is equally divided on each side. If a distribution is not symmetrical or Normal, then it is skewed, i.e., it is either the frequency distribution skewed to the left side or to the right side.
What is the second coefficient of skewness?
As you can see above that Pearson’s first coefficient of skewness has a mode as its one variable to calculate it & it is useful only when data has a more repetitive number in the data set, Like if there are only a few Repetitive data in the data set which belong to mode, then Pearson’s second coefficient of skewness is a more reliable measure of central tendency# N#Central Tendency Central Tendency is a statistical measure that displays the centre point of the entire Data Distribution & you can find it using 3 different measures, i.e., Mean, Median, & Mode. read more#N#as it considers median of the data set instead of mode.
How to find skewness formula?
Based on a statistical formula, the skewness can be positive, negative or undefined. Skewness = ∑Ni (Xi – X)3 / (N-1) * σ3 read more is represented as below –
What is skewness range?
Skewness range from negative infinity to positive infinity & it sometimes becomes difficult for an investor to predict the trend in the data set.
When is a distribution negatively skewed?
Negative: The distribution is negatively skewed when most of the frequency of distribution lies on the left side of distribution & has a longer and fatter left tail. Where the distribution’s Mean < Median < Mode.
What is the mean of a distribution if it is symmetric?
If the distribution is symmetric, then it has a skewness of 0 & its Mean = Median = Mode.
What is skewness in statistics?
Definition of Skewness. Skewness in statistics represents an imbalance and asymmetry from the mean of a data distribution. If you look at a normal data distribution using a bell curve, the curve will be perfectly symmetrical. Now, this doesn't happen all that often!
What does a positive skew mean?
A positive skew means that the extreme data results are larger, bringing the average up and making it larger than the median.
What is standard deviation?
Now, you may be asking: What is standard deviation? Standard deviation tells you how different and varied your data set really is. Standard deviation shows you how far your numbers spread out from the mean and median. Here is the formula to find standard deviation:
Where is the mean in a normal data distribution?
In a normal data distribution, the mean is directly in the middle (and top point) of the bell curve. Imagine that Mrs. Thomas wanted to teach her high school statistics class on the first day about data distributions, standard deviations, and bell curves. She asks her 16 student class to secretly divulge their summer job incomes. Each student provides Mrs. Thomas with a piece of paper with their income. She rounds each income level to the nearest 500 and makes a chart.
What is the difference between mean and mode?
Mean is the average of the numbers in the data distribution . Median is the number that falls directly in the middle of the data distribution. Mode is the number that appears most frequently in the data distribution. In a normal data distribution, the mean is directly in the middle (and top point) of the bell curve.
What is skewness in statistics?
Skewness is a key statistics concept you must know in the data science and analytics fields. Learn what is skewness, and why it’s important for you as a data science professional.
What is skewness in probability?
Skewness is the measure of the asymmetry of an ideally symmetric probability distribution and is given by the third standardized moment. If that sounds way too complex, don’t worry! Let me break it down for you.
Why is Skewness Important?
Now, we know that the skewness is the measure of asymmetry and its types are distinguished by the side on which the tail of probability distribution lies. But why is knowing the skewness of the data important?
Why is the same line in the above image mean, median, and mode?
It is because the mean, median, and mode of a perfectly normal distribution are equal. So far, we’ve understood the skewness of normal distribution using a probability or frequency distribution.
What happens if the length of the left whisker is greater than that of the right whisker?
And if the length of the left whisker is greater than that of the right whisker, then we can say that the data is negatively skewed.
Which side is a positively skewed distribution?
The probability distribution with its tail on the right side is a positively skewed distribution and the one with its tail on the left side is a negatively skewed distribution. If you’re finding the above figures confusing, that’s alright. We’ll understand this in more detail later.
What is the first step in determining the length of whiskers?
So, the first step is always to check the equality of Q2-Q1 and Q3-Q2. If that is found equal, then we look for the length of whiskers.
What is skewness in statistics?
Skewness is a number that indicates to what extent. a variable is asymmetrically distributed. Positive (Right) Skewness Example. Negative (Left) Skewness Example. Population Skewness - Formula and Calculation. Sample Skewness - Formula and Calculation. Skewness in SPSS. Skewness - Implications for Data Analysis.
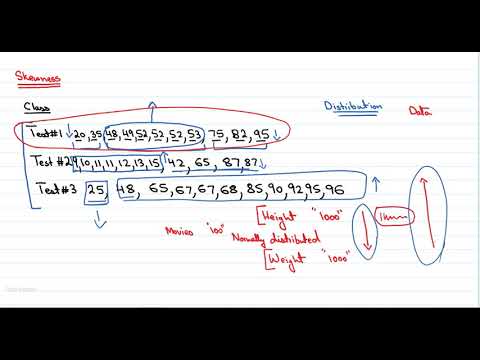
What is the skewness of symmetrical distributions?
Finally, symmetrical distributions have skewness = 0. The scores on test 3 -having skewness = 0.1 - come close.
When normality is really needed, do such tests have low power?
However, when normality is really needed -with small sample sizes- such tests have low power: they may not reach statistical significance even when departures from normality are severe. Like so, they mainly provide you with a false sense of security.
Is skewness a problem in statistical tests?
no real problem for statistical tests. However, skewness is often associated with large standard deviations. These may result in large standard errors and low statistical power. Like so, substantial skewness may decrease the chance of rejecting some null hypothesis in order to demonstrate some effect.
Is a nonparametric test robust against a violation of the normality assumption?
With small sample sizes, many tests are not robust against a violation of the normality assumption. The solution -once again- is using a nonparametric test because these don't require normality. Last but not least, there isn't any statistical test for examining if population skewness = 0.

What Is Skewness?
- Skewness refers to a distortion or asymmetry that deviates from the symmetrical bell curve, or n…
Skewness, in statistics, is the degree of asymmetry observed in a probability distribution. - Distributions can exhibit right (positive) skewness or left (negative) skewness to varying degree…
Investors note right-skewness when judging a return distribution because it, like excess kurtosis, better represents the extremes of the data set rather than focusing solely on the average.
Understanding Skewness
- There are several different types of distributions and skews. The "tail" or string of data points aw…
In addition, a distribution can have a zero skew. Zero skew occurs when a data graph is symmetrical. Regardless of how long or fat the distribution tails are, a zero skew indicates a normal distribution of data. A data set can also have an undefined skewness should the data no…
Measuring Skewness
- There are several ways to measure skewness. Pearson’s first and second coefficients of skewn…
\begin {aligned} &\begin {gathered} Sk _1 = \frac {\bar {X} - Mo} {s} \\ \underline {\qquad\qquad\qquad\qquad\qquad\qquad\qquad\qquad\qquad\qquad\qquad\quad} \\ Sk _2 = \frac {3\bar {X} - Md} {s} \end {gathered}\\ &\textbf {where:}\\ &Sk_1=\text {Pearson's first coeffi…
What Does Skewness Tell You?
- Investors note skewness when judging a return distribution because it, like kurtosis, considers th…
Investors commonly use standard deviation to predict future returns, but the standard deviation assumes a normal distribution. As few return distributions come close to normal, skewness is a better measure on which to base performance predictions. This is due to skewness risk.
Examples of a Skewed Distribution
- The departure from "normal" returns has been observed with more frequency in the last two dec…
The broad stock market is often considered to have a negatively skewed distribution. The notion is that the market more often returns a small positive return more often a large negative loss. However, studies have shown that the equity of an individual firm may tend to be left-skewed. 1
What Does Skewness Tell Us?
- Skewness tells us the direction of outliers. In a positive skew, the tail of a distribution curve is longer on the right side. This means the outliers of the distribution curve are further out towards the right and closer to the mean on the left. Skewness does not inform on the number of outliers; it only communicates the direction of outliers.
What Causes Skewness?
- Skewness is simply a reflection of a data set in which activity is heavily condensed in one range and less condensed in another. Imagine scores being measured at an Olympic long jump contest. Many jumpers will likely land larger distances, while a fewer amount will likely land short distances. This often creates a right-skewed distribution. Therefore, the relationship between th…
Is Skewness Normal?
- Skewness is commonly found when analyzing data sets, as there are situations that occur where skewness is simply a component of the data set being analyzed. For example, consider the average human lifespan. As most people tend to die after reaching an elderly age, fewer individuals relatively tend to pass away when they are younger. In this case, skewness is expect…
What Does High Skewness Mean?
- High skewness means a distribution curve has a shorter tail on one end a distribution curve and a long tail on the other. The data set follows a normal distribution curve; however, higher skewed data means the data is not evenly distributed. The data points favor one side of the distribution due to the nature of the underlying data.