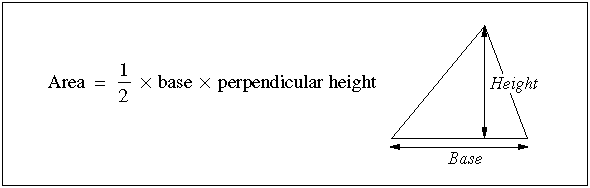
- the second leg is also equal to a.
- the hypotenuse is a√2.
- the area is equal to a²/2.
- the perimeter equals a(2 + √2)
How to solve a 45 45 90 triangle?
How to solve a 45 45 90 triangle: an example 1 Type the given value. In our case, the easiest way is to type the length of the part with the scale. The usual leg... 2 The 45 45 90 triangle calculator shows the remaining parameters. Now you know: More ...
How do you find the perimeter of a triangle?
The perimeter of a triangle is the distance around the outside, and can be found by adding the side lengths together: . Find the perimeter of the triangle below. The first point of business is to realize is that we have a 45-45-90 triangle. That means the two legs of our triangle are congruent and are thus each 8.
How do you find the area of a 45 degree triangle?
For this special angle of 45°, both of them are equal to √2/2. So: a/c = √2/2 so c = a√2 To find the area of such triangle, use the basic triangle area formula is area = base * height / 2. In our case, one leg is a base and the other is the height, as there is a right angle between them.
How to find the perimeter of an isosceles triangle?
We use the Pythagorean Theorem, which states that a 2 + b 2 = c 2, where a and b are the legs and c is the hypotenuse. Let = leg length. Because this is an isosceles triangle, the two legs have the same length. Plug this and the hypotenuse length into the Pythagorean Theorem and solve for x: Thus the perimeter is .
How do you find the perimeter of a 45 45 90?
3:254:28Using 45-45-90 triangle to find the perimeter of a rectangle - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd we can find the perimeter. Just by adding up. 12 plus 12 plus 6 plus 6 which adds up to 36.MoreAnd we can find the perimeter. Just by adding up. 12 plus 12 plus 6 plus 6 which adds up to 36.
How do you solve a 45 45 90 triangle problem?
1:465:4245-45-90 Triangle Examples - YouTubeYouTubeStart of suggested clipEnd of suggested clipIf I know this is a length of two this should also be a length of two. It's that easy to - and soMoreIf I know this is a length of two this should also be a length of two. It's that easy to - and so what's Y gonna be well. If you want to figure out what that is. You can simply tack on.
How do I calculate the perimeter of a triangle?
To calculate the perimeter of a triangle, add the length of its sides. For example, if a triangle has sides a, b, and c, then the perimeter of that triangle will be P = a + b + c.
How do you find the third side of a 45 45 90 triangle?
0:121:16Determine the missing hypotenuse of a 45 45 90 triangle ex 3 - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo a special right triangle which is a 45 45 90 triangle and you can see i have the template of howMoreSo a special right triangle which is a 45 45 90 triangle and you can see i have the template of how the sides are related for a 45-45 degree triangle and one thing. I. Sorry one thing you notice about
What is the rule for a 45 45 90 triangle Quizizz?
It is the same length as the given leg. Multiply that leg's length by √2. Multiply that leg's length by 2. Divide that leg's length by √2.
How do you find the sides of a triangle with hypotenuse?
If you have the hypotenuse, multiply it by sin(θ) to get the length of the side opposite to the angle. Alternatively, multiply the hypotenuse by cos(θ) to get the side adjacent to the angle. If you have the non-hypotenuse side adjacent to the angle, divide it by cos(θ) to get the length of the hypotenuse.
How do you find a missing perimeter?
4:225:17Finding Perimeter when a Side Length is Missing (Composite Figures)YouTubeStart of suggested clipEnd of suggested clipSo eight plus 10 is 18 plus 8 is 20 6 plus 10 is 30 6. Plus 2 is 30 8. Plus 20 is 58 plus 18 isMoreSo eight plus 10 is 18 plus 8 is 20 6 plus 10 is 30 6. Plus 2 is 30 8. Plus 20 is 58 plus 18 is going to be 76. Plus 20 gives us 96.
What is the perimeter formula?
The total length of the boundary of a closed shape is called its perimeter. Hence, the perimeter of that shape is measured as the sum of all the sides. Thus, the perimeter formula is Perimeter(P) = Sum of all the sides.
How do you find the perimeter of a triangle given 3 points?
0:106:04Find the perimeter of a triangle on a coordinate plane | GeometryYouTubeStart of suggested clipEnd of suggested clipNow remember the perimeter is going to be the sum of all the lengths for our triangle. So theMoreNow remember the perimeter is going to be the sum of all the lengths for our triangle. So the perimeter is going to equal the length xy.
How do you find the side of a right triangle with a side and an angle?
1:5011:12How to Solve Right Triangle Given One Angle and one Side - YouTubeYouTubeStart of suggested clipEnd of suggested clipBy hypotenuse and tan theta is equals to opposite side divided by adjacent side so these are theMoreBy hypotenuse and tan theta is equals to opposite side divided by adjacent side so these are the three trigonometric ratios that you have to use in order to solve.
Which of the following could be the lengths of the sides of a 45 45 90 triangle?
Summary: 3√2, 3√2, 6 could be the side lengths of a 45°-45°-90° triangle.
How do you find the missing side of a special right triangle?
0:083:48How to use special right triangles to find missing side lengths - YouTubeYouTubeStart of suggested clipEnd of suggested clipYou just take the leg times the square root of 2 to find the hypotenuse. So the rule is x x x root 2MoreYou just take the leg times the square root of 2 to find the hypotenuse. So the rule is x x x root 2 and this is exactly what you're going to see on a standardized.
How to find the area of a 45 degree triangle?
So: a/c = √2/2 so c = a√2. To find the area of such triangle, use the basic triangle area formula is area = base * height / 2.
What are the rules for a 45 90 triangle?
The most important rule is that this triangle has one right angle, and two other angles are equal to 45°. It implies that two sides - legs - are equal in length and the hypotenuse can be easily calculated.
How to find the hypotenuse of a triangle?
The legs of such a triangle are equal, the hypotenuse is calculated immediately from the equation c = a√2. If the hypotenuse value is given, the side length will be equal to a = c√2/2 . Triangles (set squares). The red one is the 45 45 90 degree angle triangle
How to find the hypotenuse of a 45-45-90 triangle?
To solve for the hypotenuse length of a 45-45-90 triangle, you can use the 45-45-90 theorem, which says the length of the hypotenuse of a 45-45-90 triangle is the √2 2 times the length of a leg.
Which side of a triangle is congruent in length?
Another rule is that the two sides of the triangle or legs of the triangle that form the right angle are congruent in length. Knowing these basic rules makes it easy to construct a 45-45-90 triangle.
How to find the length of a leg?
If you know the measure of the hypotenuse, divide the hypotenuse by √2 2 to find the length of either leg. If you know the length of one leg, you know the length of the other leg (legs are congruent) If you know either leg's length, multiply the leg length times √2 2 to find the hypotenuse.
45 45 90 Pythagorean Theorem Shortcut
Since the two legs of a 45 45 triangle are congruent, we can simplify the Pythagorean theorem. Remember that the Pythagorean theorem tells us a 2 + b 2 = c 2.
45 45 90 Triangle Rules
Four handy rules that apply to the 45 45 90 triangle: 1.) The three internal angles are 45, 45, and 90 degrees. 2.) The legs are congruent. 3.) The hypotenuse length is √2 times the leg length. 4.) It can be created by cutting a square in half at the diagonal as shown below.
Finding the Area and Perimeter
The equation for area of a 45 45 90 triangle is given as: A = 1/2b2 Where A is the area and b is the leg length.
What is the ratio of a 45-45-90-degree triangle?
The side lengths of this triangle are in the ratio of; Side 1: Side 2: Hypotenuse = n: n: n√2 = 1:1: √2. The 45°-45°-90° right triangle is half of a square.
How to find hypotenuse of 45-45-90-90?
We can calculate the hypotenuse of the 45°-45°-90° right triangle as follows: Let side 1 and side 2 of the isosceles right triangle be x.
What is the ratio of isosceles right triangle?
An isosceles right triangle is the same as the 45°-45°-90° right triangle. So, we apply the ratio of n: n: n√2 to calculate the hypotenuse’s length. Given that n = 5√2/2 cm; ⇒ n√2 = (5√2/2) √2. ⇒ (5/2) √ (2 x 2) ⇒ (5/2) √ (4) ⇒ (5/2)2. = 5. Hence, the two legs of the triangle are 5 cm each.
How to find the length of a hypotenuse?
To get the length of the hypotenuse, multiply the side length by √2. ⇒ 12 √2 = 10 √2. Hence, the diagonal is 10 √2 cm. Example 8. Find the lengths of the other two sides of a square whose diagonal 4√2 inches. Solution. A half of a square makes a 45°- 45°-90°right triangle.
How many angles are there in a square?
This is because the square has each angle equal to 90°, and when it is cut diagonally, the one angle remains as 90°, and the other two 90° angles bisected (cut into half) and become 45° each. The diagonal of a square becomes hypotenuse of a right triangle, and the other two sides of a square become the two sides (base and opposite) ...
What is the ratio of the side lengths of a 45-45-90 triangle?
The ratio of the side lengths of a 45-45-90 triangle are: The legs opposite the 45° angles (the shorter sides) are of the length of the hypotenuse (the side opposite the 90° angle) The hypotenuse is times the length of either leg. Since a 45-45-90 triangle is also an isosceles triangle, the two legs are equal in measure.
What is the ratio of the side opposite the 45 degree angle of a right triangle?
For example, sin (45°), read as the sine of 45 degrees, is the ratio of the side opposite the 45° angle of a right triangle, to its hypotenuse. Using the side lengths , for the 45-45-90 triangle above: Similarly: tan (45°) = 1.