
Unit Circle and Trigonometric Identities
- sinθ = y/1
- cosθ = x/1
- tanθ = sinθ/cosθ = y/x
- sec (θ = 1/x
- csc (θ) = 1/y
- cot (θ) = cosθ/sinθ = x/y
How do you find the value of sin in a circle?
The unit circle can be used to find the values of sinθ and Cosθ. In a unit circle of radius 1 unit and having the center at (0, 0), let us take a radius inclined to the positive x-axis at an angle θ, and the endpoint of the radius as (x, y).
How do you find angles with the same sine value?
For any given angle in the first quadrant, there is an angle in the second quadrant with the same sine value. Because the sine value is the y -coordinate on the unit circle, the other angle with the same sine will share the same y -value, but have the opposite x -value.
What is the sine function?
The sine function relates a real number t to the y -coordinate of the point where the corresponding angle intercepts the unit circle. More precisely, the sine of an angle t. t. In Figure 2, the sine is equal to
What are the unit circle identities of sin and cosine?
The unit circle identities such as cosecant, secant, cotangent are the respective reciprocal of the sine, cosine, tangent. Further, we can obtain the value of tanθ by dividing sinθ with cosθ, and we can obtain the value of cotθ by dividing cosθ with sinθ.
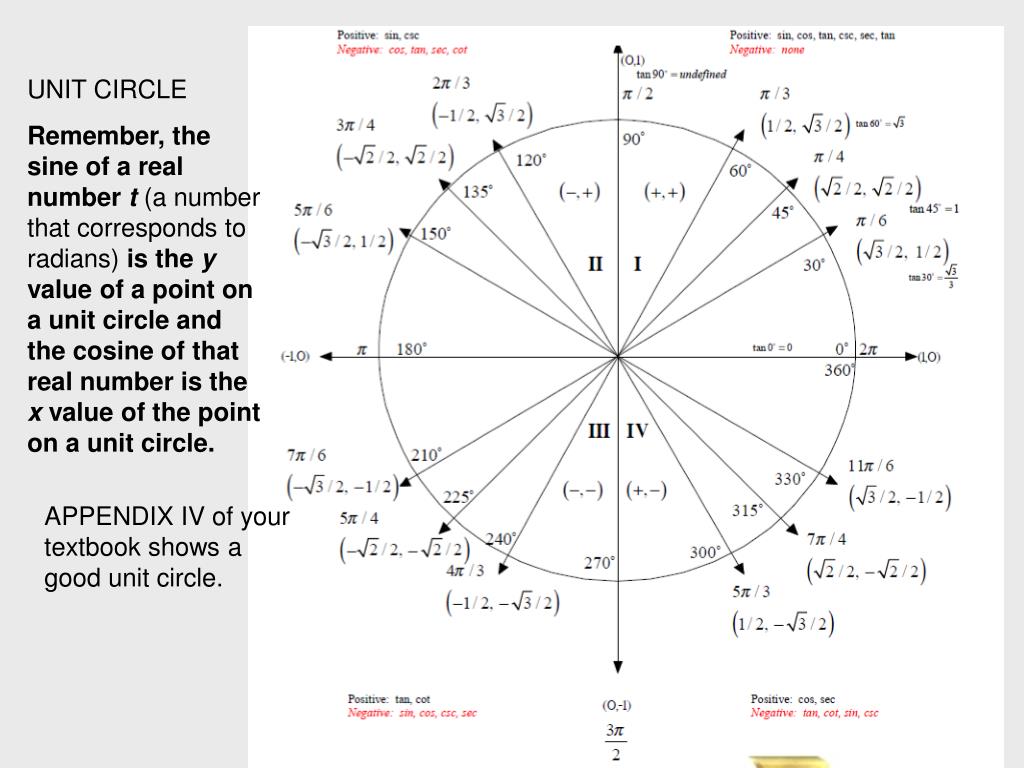
How do you find the sine and cosine values on the unit circle?
Using the unit circle, the sine of an angle t equals the y-value of the endpoint on the unit circle of an arc of length t whereas the cosine of an angle t equals the x-value of the endpoint.
How do you find the sine value?
0:512:48Trigonometry - Find the exact value of sin cos and tan - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo I'm thinking about this angle in the bottom left corner and I'm going to take the opposite sideMoreSo I'm thinking about this angle in the bottom left corner and I'm going to take the opposite side and put it over the hypotenuse. So sine would simply be 5 thirteenth's.
How do you find sin 30 on unit circle?
Sin 30 Degrees Using Unit Circle To find the value of sin 30 degrees using the unit circle: Rotate 'r' anticlockwise to form a 30° angle with the positive x-axis. The sin of 30 degrees equals the y-coordinate(0.5) of the point of intersection (0.866, 0.5) of unit circle and r.
How do you find sin 45 on the unit circle?
The value of sin 45 degrees can be calculated by constructing an angle of 45° with the x-axis, and then finding the coordinates of the corresponding point (0.7071, 0.7071) on the unit circle. The value of sin 45° is equal to the y-coordinate (0.7071). ∴ sin 45° = 0.7071.
How do you calculate sine manually?
0:042:19How to Calculate Sin, Cos & Tan With No Calculator : Math Lessons & TipsYouTubeStart of suggested clipEnd of suggested clipSo sine is going to be the opposite over the hypotenuse cosine will be the adjacent. Over theMoreSo sine is going to be the opposite over the hypotenuse cosine will be the adjacent. Over the hypotenuse. And tangent will be the opposite. Over the adjacent.
How do you calculate sine by hand?
5:0731:07How to evaluate sine, cosine, tangent by hand (radians) | Math HacksYouTubeStart of suggested clipEnd of suggested clipSo all I need to do here is is look at the Y value for sine of zero. And my Y value is zero toMoreSo all I need to do here is is look at the Y value for sine of zero. And my Y value is zero to evaluate cosine of zero I'm going to look at the x coordinate in my point.
What is the value of sin?
The value of sin and cos infinity lies between -1 to 1. There are no exact values defined for them. The value of sin x and cos x always lies in the range of -1 to 1. Also, ∞ is undefined thus, sin(∞) and cos(∞) cannot have exact defined values.
How do you find trig functions using the unit circle?
0:014:22Determine 6 Trig Function Values Using The Unit Circle (Radians)YouTubeStart of suggested clipEnd of suggested clipOr one divided by y. And cotangent theta is equal to the reciprocal of tangent theta which is xMoreOr one divided by y. And cotangent theta is equal to the reciprocal of tangent theta which is x divided by y. For the next step we determine where the terminal.
How do you find the exact value of a trig function using the unit circle?
0:072:31Exact values of trig functions from the unit circle, ex1 - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo this is a unit circle all right so we should indicate that the radius here is 1 remember on theMoreSo this is a unit circle all right so we should indicate that the radius here is 1 remember on the unit circle the x value here represents the cosine value and the Y right here represents the sine.
What's the sine of 90?
1What is the exact value of sin 90 degrees? The exact value of sin 90 degrees is equal to 1.
What is the sine of the 45 angle?
1 / 2= 1 / 2Sine 0°0Sine 45° or Sine π/41 / 2Sine 60°or Sine π/33 / 2Sine 90° or Sine π/21Sine 120° or Sine 2π/33 / 25 more rows
What is the value of sin 60⁰?
Value of Sin 60 DegreeRadian0π/3Sin03 / 2Cos11/2Tan03
What is the sine of θ?
Formulas for right triangles If θ is one of the acute angles in a triangle, then the sine of theta is the ratio of the opposite side to the hypotenuse, the cosine is the ratio of the adjacent side to the hypotenuse, and the tangent is the ratio of the opposite side to the adjacent side.
What is the value of sin 60⁰?
Value of Sin 60 DegreeRadian0π/3Sin03 / 2Cos11/2Tan03
What is sin equal to?
The sine of an angle is equal to the ratio of the opposite side to the hypotenuse whereas the cosine of an angle is equal to the ratio of the adjacent side to the hypotenuse.
What is the value of sin 30 *?
0.5Answer: The value of sin 30 degrees is 1/2 or 0.5.
Answer
In the unit circle sine and cosine are given by the y-coordinate and x-coordinate, respectively. Tangent is the ratio y-coordinate / x-coordinate.
Further explanation
In trigonometry, there are 3 angular functions, namely sin, cos and tan, based on a right triangle
New questions in Mathematics
Pamella late for school 10% of time. Richard late for 15% of the time.a) What percentage are they both late?b) What is the probability only one of the …
Finding Function Values for the Sine and Cosine
To define our trigonometric functions, we begin by drawing a unit circle, a circle centered at the origin with radius 1, as shown in Figure 2. The angle (in radians) that t t intercepts forms an arc of length s. s. Using the formula s = r t, s = r t, and knowing that r = 1, r = 1, we see that for a unit circle, s = t. s = t.
Unit Circle
A unit circle has a center at ( 0, 0) ( 0, 0) and radius 1 1. In a unit circle, the length of the intercepted arc is equal to the radian measure of the central angle t. t.
Sine and Cosine Functions
Given a point P ( x, y) ( x, y) on the unit circle corresponding to an angle of t, t, find the sine and cosine.
Example 1
Point P P is a point on the unit circle corresponding to an angle of t, t, as shown in Figure 4. Find cos ( t) cos ( t) and sin ( t). sin ( t).
Finding Sines and Cosines of Special Angles
We have already learned some properties of the special angles, such as the conversion from radians to degrees. We can also calculate sines and cosines of the special angles using the Pythagorean Identity and our knowledge of triangles.
Identifying the Domain and Range of Sine and Cosine Functions
Now that we can find the sine and cosine of an angle, we need to discuss their domains and ranges.
Finding Reference Angles
We have discussed finding the sine and cosine for angles in the first quadrant, but what if our angle is in another quadrant? For any given angle in the first quadrant, there is an angle in the second quadrant with the same sine value.
How to find the trigonometric ratio of a unit circle?
The unit circle having an equation of x 2 + y 2 = 1 is helpful to find the trigonometric ratios of sinθ = y and cosθ = x. Using these values we can conveniently find the value of tanθ = sinθ/cosθ = y/x. Tanθ will be undefined for cosθ = 0, i.e., when θ is equal to 90° and 270°.
What are the coordinates of a unit circle?
Any point on the unit circle has coordinates (x, y), which are equal to the trigonometric identities of (cosθ, sinθ). For any values of θ made by the radius line with the positive x-axis, the coordinates of the endpoint of the radius represent the cosine and the sine of the θ values. Here we have cosθ = x, and sinθ = y, and this is helpful to compute the other trigonometric ratio values. Applying this further we have tanθ = sinθ/cosθ or tanθ = y/x.
What are unit circle identities?
The unit circle identities such as cosecant, secant, cotangent are the respective reciprocal of the sine, cosine, tangent. Further, we can obtain the value of tanθ by dividing sinθ with cosθ, and we can obtain the value of cotθ by dividing cosθ with sinθ.
What is the unit circle of a trigonometric ratio?
The unit circle represents a complete angle of 2π radians. And the unit circle is divided into four quadrants at angles of π/2, π. 3π/2, and 2π respectively. Further within the first quadrant at the angles of 0, π/6, π/4, π/3, π/2 are the standard values, which are applicable to the trigonometric ratios. The points on the unit circle for these angles represent the standard angle values of the cosine and sine ratios. On close observation of the below figure the values are repeated across the four quadrants, but with a change in sign. This change in sign is because of the reference x-axis and y-axis, which is positive on one side and negative on the other side of the origin. Now with the help of this we can easily find the trigonometric ratio values of standard angles, across the four quadrants of the unit circle.
How are right triangles and unit circles connected?
The equation of a circle x 2 + y 2 = 1 completely satisfies the Pythagoras theorem related to the right triangle. Also, the right triangle within the unit circle is helpful to derive the trigonometric ratio values.
What is the x-coordinate of 0°?
For θ = 0°, the x-coordinate is 1 and the y-coordinate is 0. Therefore, we have cos0º = 1, and sin0º = 0. Let us look at another angle of 90º. Here the value of cos90º = 1, and sin90º = 1. Further, let us use this unit circle and find the important trigonometric function values of θ such as 30º, 45º, 60º. Also, we can also measure these θ values in radians. We know that 360° = 2π radians. We can now convert the angular measures to radian measures and express them in terms of the radians.
How to find hypotenuse of a triangle?
Draw a perpendicular from the end of the radius to the x-axis and it forms a right-angled triangle with the radius as the hypotenuse. The adjacent side of this triangle is the x value, the opposite side of the triangle is the y value and the hypotenuse is of 1 unit.
What is a unit circle?
The "Unit Circle" is a circle with a radius of 1 and a center at (0, 0). Because the radius is 1, we can directly measure sine, cosine and tangent. The angle (in radians) that t intercepts forms an arc of length s.Using the formula s=rt, and knowing that r=1, we see that for a unit circle, s=t.
What is the tangent of a unit circle?
, then (because 45° is in first quadrant). The tangent of an angle is the ratio of the y-value to the x-value of the corresponding point on the unit circle.
What is the second bonus?
The second bonus – the right triangle within the unit circle formed by the cosine leg, sine leg, and angle leg (value of 1) is similar to a second triangle formed by the angle leg (value of 1), the tangent leg, and the secant leg. In this second triangle the tangent leg is similar to the sin leg the angle leg is similar to the cosine leg and ...
Which leg is similar to the sin leg?
In this second triangle the tangent leg is similar to the sin leg the angle leg is similar to the cosine leg and the secant leg (the hypotenuse of this triangle) is similar to the angle leg of the first triangle.
What is the tangent of a parabola at 45 degrees?
You are left with something that looks a little like the right half of an upright parabola. At negative 45 degrees the tangent is -1 and as the angle nears negative 90 degrees the tangent becomes an astronomically large negative value. This portion looks a little like the left half of an upside down parabola.
When the angle is close to zero, the tangent line is near vertical?
When the angle is close to zero the tangent line is near vertical and the distance from the tangent point to the x-axis is very short. As the angle nears 90 degrees the tangent line becomes nearly horizontal and the distance from the tangent point to the x-axis becomes remarkably long.
How many degrees are in a Pi radian?
Pi radians is equal to 180 degrees. They are two different ways of measuring angles. If you want to know why pi radians is half way around the circle, see this video: https://www.khanacademy.org/math/trigonometry/unit-circle-trig-func/intro-to-radians-trig/v/introduction-to-radians.
