The Binomial Theorem
Binomial theorem
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the polynomial (x + y) into a sum involving terms of the form a x y, where the exponents b and c are nonnegative integers with b + c = n, and the coefficient a of each term is a specific positive integer depending on n and b. For exampl…
Full Answer
How to use the rational zeros theorem?
- Determine all factors of the constant term and all factors of the leading coefficient.
- Determine all possible values of p q p q, where p is a factor of the constant term and q is a factor of the leading coefficient. ...
- Determine which possible zeros are actual zeros by evaluating each case of f (p q) f ( p q).
How to use the inverse function theorem?
Use the inverse function theorem to find the derivative of g(x) = x + 2 x. Compare the resulting derivative to that obtained by differentiating the function directly. The inverse of g(x) = x + 2 x is f(x) = 2 x − 1. We will use Equation 3.7.4 and begin by finding f′ (x). Thus, f′ (g(x)) = − 2 (g(x) − 1)2 = − 2 (x + 2 x − 1)2 = − x2 2.
What is the significance of the binomial theorem?
Some Interesting Properties of Binomial Theorem:
- The total number of each and every term in the expansion is n + 1 .
- The sum total of the indices of x and y in each term is n .
- The expansion shown above is also true when both x and y are complex numbers.
- The coefficient of all the terms is equidistant (equal in distance from each other) from the beginning to the end.
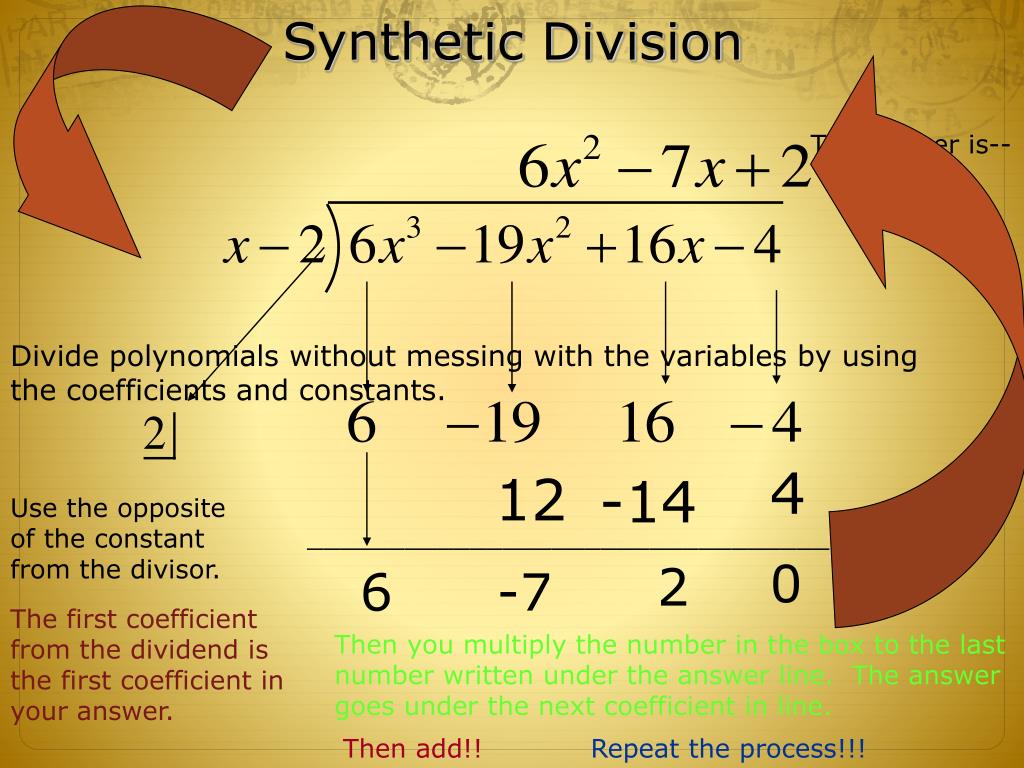
How does the remainder theorem work with polynomials?
The polynomial remainder theorem, the polynomial remainder theorem tells us that if we take some polynomial, p of x and we were to divide it by some x minus a then the remainder is just going to be equal to our polynomial evaluated at our polynomial evaluated at a. So let's just see what's a in this case.
How do you interpret binomial distribution?
Analyzing Binomial Distribution The expected value, or mean, of a binomial distribution is calculated by multiplying the number of trials (n) by the probability of successes (p), or n x p. For example, the expected value of the number of heads in 100 trials of heads or tales is 50, or (100 * 0.5).
What binomial theorem tells us?
The Binomial theorem tells us how to expand expressions of the form (a+b)ⁿ, for example, (x+y)⁷. The larger the power is, the harder it is to expand expressions like this directly. But with the Binomial theorem, the process is relatively fast! Created by Sal Khan.
What is binomial in simple terms?
Definition of binomial 1 : a mathematical expression consisting of two terms connected by a plus sign or minus sign. 2 : a biological species name consisting of two terms.
How do you prove a binomial theorem?
Proof of the binomial theorem by mathematical inductionWe first note that the result is true for n=1 and n=2.Let k be a positive integer with k≥2 for which the statement is true. So.Hence the result is true for k+1. By induction, the result is true for all positive.integers n.
What is the importance of the binomial theorem?
The binomial theorem gives us the general formula for the expansion of (a+b)n for any positive integer n. It also enables us to determine the coefficient of any particular term of an expansion of (a+b)n.
What is the use of binomial theorem in real life?
The Binomial Theorem is used in advanced mathematics and calculating to determine the roots of equations in higher powers. It's also used to prove a lot of important physics and math equations. Weather forecast services, architecture, and cost estimation in engineering projects.
What is a binomial and give an example?
A binomial is a polynomial with two terms. For example, x − 2 x-2 x−2 and x − 6 x-6 x−6 are both binomials.
How do you solve binomials?
1:357:55Solving binomials by factoring (basic algebra) - YouTubeYouTubeStart of suggested clipEnd of suggested clipX is already isolated x minus 2 equals 0. We add 2 both sides to isolate x okay cancel so x equals 0MoreX is already isolated x minus 2 equals 0. We add 2 both sides to isolate x okay cancel so x equals 0 plus 2 2. So x equals 2 thus we have x equals 0. And x equals. 2 are two solutions.
What does a binomial look like?
A polynomial with two terms is called a binomial; it could look like 3x + 9. It is easy to remember binomials as bi means 2 and a binomial will have 2 terms. A classic example is the following: 3x + 4 is a binomial and is also a polynomial, 2a(a+b) 2 is also a binomial (a and b are the binomial factors).
Who was the first to prove the binomial theorem?
Isaac Newton is generally credited with the generalized binomial theorem, valid for any rational exponent.
What is binomial theorem in discrete mathematics?
Binomial Theorem is used to solve binomial expressions in a simple way. It gives an expression to calculate the expansion of (a+b)n for any positive integer n. The Binomial theorem is stated as: (a + b)n = nC0 an + nC1 an-1 b1 + nC2 an-2 b2 + …. + nCr an-r br + ….
What is the general term in binomial expansion?
General Term in binomial expansion: In the binomial expansion of (x + y)n , the rth term from end is (n – r + 2)th .
What is binomial expansion in genetics?
This binomial expansion shows the probability of various combinations of boys and girls in a family of 4 disregarding the sequence of children. For example, 6/16 p2q2 tells that the probability of having 2 boys and 2 girls is 6/16 in a family of 4 children.
Is binomial theorem important for JEE?
Binomial theorem is one of the important concepts in JEE Mathematics.
What is binomial example?
A binomial is a polynomial with two terms. For example, x − 2 x-2 x−2 and x − 6 x-6 x−6 are both binomials.
What is the general term in binomial expansion?
Using the general term for the binomial expansion often simplifies calculations in which we are only interested in specific terms and their coefficients. The formula for the general term for the binomial expansion ( 𝑝 + 𝑞 ) is 𝑇 = 𝐶 𝑝 𝑞 𝑟 = 0 , 1 , … , 𝑛 .
What can we use the binomial theorem for?
We can use the Binomial Theorem to calculate e (Euler's number).
What is a binomial?
A binomial is a polynomial with two terms
What does an exponent of 2 mean?
An exponent of 2 means to multiply by itself (see how to multiply polynomials ):
Which theorem sums up the pattern?
That pattern is summed up by the Binomial Theorem:
Who came up with the formula for exponents of 0?
As a footnote it is worth mentioning that around 1665 Sir Isaac Newton came up with a "general" version of the formula that is not limited to exponents of 0, 1, 2, .... I hope to write about that one day.
Do we need to calculate all other values if we only want one term?
But we don't need to calculate all the other values if we only want one term.)
Can you sum up terms in Sigma?
Yes! The handy Sigma Notation allows us to sum up as many terms as we want:
Why use the binomial theorem?from khanacademy.org
Use the binomial theorem in order to expand integer powers of binomial expressions.
What is the formula for expansion of the binomial theorem?from cuemath.com
In the binomial theorem formula of expansion (x+a) n, we use the combinatorics formula that is denoted as nCr n C r, where n is the exponent in the expansion and r is the term number that ranges from 0 to n.
What are the coefficients of binomial expansion?from cuemath.com
The coefficients in the binomial expansion are nC0 n C 0, nC1 n C 1, nC2 n C 2, ..... nCr n C r ...... nCn n C n. The coefficient value for n = 4 is equal to 1, 4, 6, 4,1, and the coefficient value for n = 5 is 1, 5, 10, 20, 10, 5, 1. The coefficient values can be found either from the pascals triangle or by using the combinations formula of nCr = n! r!.(n−r)! n C r = n! r!. ( n − r)!.
How to find combinations of binomial coefficients?from cuemath.com
The formula to find the combinations of r objects taken from n different objects is nCr = n! r!(n−r)! n C r = n! r! ( n − r )!. Here the coefficients have the following properties.
What is the number of coefficients in the binomial expansion of (x + y) n?from cuemath.com
The number of coefficients in the binomial expansion of (x + y) n is equal to (n + 1).
What is the binomial theorem for a positive value?from en.wikipedia.org
For positive values of a and b, the binomial theorem with n = 2 is the geometrically evident fact that a square of side a + b can be cut into a square of side a, a square of side b, and two rectangles with sides a and b. With n = 3, the theorem states that a cube of side a + b can be cut into a cube of side a, a cube of side b, three a × a × b rectangular boxes, and three a × b × b rectangular boxes.
What is the binomial expansion?from en.wikipedia.org
In elementary algebra, the binomial theorem (or binomial expansion) describes the algebraic expansion of powers of a binomial. According to the theorem, it is possible to expand the polynomial (x + y)n into a sum involving terms of the form axbyc, where the exponents b and c are nonnegative integers with b + c = n, and the coefficient a of each term is a specific positive integer depending on n and b. For example (for n = 4 ),
What is the Binomial Theorem Formula?
The binomial theorem allows us to expand an expression of the form ( x + y) n into a polynomial sum containing x and y terms. A general formula for a binomial expression is given by,
What is the proof for the binomial theorem?
The binomial theorem can be proved in a few different ways, but we will focus on the combinatorial proof.
How to do a binomial expansion?
To understand how to expand a binomial expansion, we will look at an example.
Binomial theorem examples
The binomial theorem can also be used to find a specific term for a binomial expansion. For this, you do not have to carry out the whole expansion but will only be required to find one term. Let's look at an example to see how this can be done.
Binomial Theorem - Key takeaways
The binomial theorem is used for carrying out binomial expansions. This means simplifying expressions of the form ( x + y) n into a polynomial sum in terms of x and y.
Why do I love binomial theorem?
I love, love, love the binomial theorem because it’s so darn clever. It’s essentially a combinatorics approach to solving a horrendously long algebra problem 🙌🏼 and when you toss in Pascal’s Triangle you get pure math magic.
How to find middle number in Pascal's triangle?
For each row thereafter you simply write 1’s on both ends, and find the middle number (s) by adding the two values from directly above it. First six rows of Pascal’s Triangle.
What is the value of k in a n-choose-k equation?
The k values in “n choose k”, will begin with k=0 and increase by 1 in each term. The last term should end with n equal to k, in this case n=3 and k=3.
Do you have to include minus in binomial?
Note that whenever you have a subtraction in your binomial it’s oh so important to remember to include the minus as a negative symbol on the accompanying term. The wonderful thing about the binomial theorem is it allows us to find the expanded polynomial without multiplying a bunch of binomials together.
What is a binomial sum?
On the other hand, a binomial is a sum of exactly two monomials. For example: Finally, a polynomial is a sum of an arbitrary number of monomials. For example: Notice that monomials are a special case of binomials and binomials are a special case of polynomials.
What happens when a binomial is raised to a positive integer?
That is, any binomial raised to a positive integer power becomes a polynomial with a number of terms one more than its power. For example, let’s expand the same polynomial but raised to the power of 3:
What is the difference between binomial distribution and PMF?
The most obvious difference is that in the binomial theorem there’s a sum, whereas the binomial distribution PMF specifies a single monomial. Let’s compare the monomials themselves.
What is a Bernoulli trial?
Remember, a Bernoulli trial is an experiment with only 2 possible outcomes. It has a single parameter p that specifies the probability of a “success” outcome (trial). For example, a single coin flip has a Bernoulli distribution.
Why is Bernoulli distribution important?
It’s important not only because such random variables are very common in the real world but also because the Bernoulli distribution is the basis for many other discrete probability distributions . Well, it is also the basis for the distribution from today’s post! Jacob Bernoulli.
What is a monomial?
A monomial is a product of a constant coefficient and one or more variables, each raised to a power of some natural number (non-negative integer). The simplest possible monomial is the number 0 and any other number, like 1, 3, and 6.4, is also a monomial.
Which theorem asserts equality?
In a nutshell, the binomial theorem asserts the following equality:
How binomial theorem calculator works?
Binominal theorem calculator works steadily and quickly. Follow the simple steps explained below:
What is the purpose of the binomial expansion theorem?
The binomial expansion theorem and its application are assisting in the following fields: To solve problems in algebra, To prove calculations in calculus, It helps in exploring the probability. However, you can handle the binomial expansion by means of binomial series calculator in all the above-mentioned fields.
How to Expand Binomials?
You can use the binomial theorem to expand the binomial. To carry out this process without any hustle there are some important points to remember:
What is a binomial coefficient?
Binomial coefficients refer to all those integers that are coefficients in the binomial theorem. Properties of binomial coefficients are given below and one should remember them while going through binomial theorem expansion:
How to deal with binomial expansions?
The simplest and error-free way to deal with the expansions is the use of binomial expansion calculator. Furthermore, it also reduces the risk of error that arises from manual calculations.
What is a polynomial that has two terms?
In mathematics, a polynomial that has two terms is known as binomial expression . These two terms will always be separated by either a plus or minus and appears in term of series. This series is known as a binomial theorem.
What is the variance of the binomial distribution?
Variance of the binomial distribution: n p ( 1 − p) .