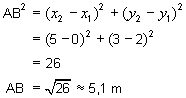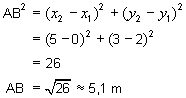
The easiest way to prove that a triangle is isosceles using coordinate geometry is to use the sides. Given the coordinates of the triangle's vertices, to prove that a triangle is isosceles use the distance formula to calculate the side length of each side of the triangle . If any 2 sides have equal side lengths, then the triangle is isosceles.
Is an isosceles triangle always a 45-45-90 triangle?
45/45/90 triangles are always isosceles . This means that two of the legs of the triangle are congruent. In the figure, it's indicates which two sides are congruent.
What is the formula for a right isosceles triangle?
What is the Isosceles Triangle?
- The side of the triangle that is unequal is called the base of the triangle.
- In an isosceles triangle, the height that is drawn from the apex divides the base of the triangle into two equal parts and the apex angle into two equal angles.
- The formula to find the area of isosceles triangle or any other triangle is: ½ × base × height.
What is the 45 45 90 triangle theorem?
What is the 45 45 90 triangle Theorem? The main rule of 45-45-90 triangles is that it has one right angle and while the other two angles each measure 45° . The lengths of the sides adjacent to the right triangle, the shorter sides have an equal length.
Do isosceles triangle always have three congruent angles?
Isosceles triangles have at least two congruent sides and at least two congruent angles. The congruent sides, called legs, form the vertex angle. The other two congruent angles are the base angles.
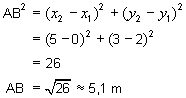
How do you find out if a triangle is isosceles from coordinates?
Explanation: One way of proving that it is an isosceles triangle is by calculating the length of each side since two sides of equal lengths means that it is an isosceles triangle.
How do you prove a triangle is an isosceles right triangle with coordinates?
0:4512:44Proving an Isosceles Right Triangle on the Coordinate Plane. - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo how do we show. Two congruent sides well. We can actually determine the lengths of these sides.MoreSo how do we show. Two congruent sides well. We can actually determine the lengths of these sides. By using the distance formula. So let's go about that let's look for the other side see B right here.
How can you prove a triangle is isosceles What formula?
1:034:38Isosceles Triangle Theorem - Proof | Don't Memorise - YouTubeYouTubeStart of suggested clipEnd of suggested clipWe have to prove that the angles opposite the equal sides in a triangle are congruent that is weMoreWe have to prove that the angles opposite the equal sides in a triangle are congruent that is we need to prove that angle abc is congruent to angle acb.
How do you prove a triangle is a right triangle with coordinates?
Calculate the length of the three sides of the triangle by joining the given coordinates. Let the sides be A, B, and C. The given triangle is right-angled if and only if A2 + B2 = C2.
How do you determine if a triangle is scalene isosceles or equilateral with points?
If none of the sides of a triangle are equal (of equal length), the triangle is scalene. If two or more of the triangles sides are equal, the triangle is isosceles. If all three of the sides of a triangle are equal, it is equilateral. All equilateral triangles are also isosceles, by definition.
What is the rule for an isosceles triangle?
An Isosceles Triangle has the following properties: Two sides are congruent to each other. The third side of an isosceles triangle which is unequal to the other two sides is called the base of the isosceles triangle. The two angles opposite to the equal sides are congruent to each other.
What is the isosceles triangle theorem?
If two sides of a triangle are congruent , then the angles opposite to these sides are congruent.
Which characteristics will prove that Δdef is a right isosceles triangle 1 point?
Which characteristics will prove that ΔDEF is a right, isosceles triangle? The lengths of and are congruent, and their slopes are opposite reciprocals.
What are the coordinates of an equilateral triangle?
If the triangle were equilateral then 2|AO| = |AC|. Since \triangle AOC is a right triangle, the Pythagorean Theorem says that |OC|^2 + |AO|^2 = |AC|^2.
Which of the following is an isosceles right triangle?
The Isosceles Right Triangle has one of the angles exactly 90 degrees and two sides, which are equal to each other. Since the two sides are equal which makes the corresponding angle congruent.
How do you classify a triangle by its vertices?
0:056:54Classifying Triangles with Coordinates - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd this one which is an angle we have to sort of sketch in a right triangle and say okay well it'sMoreAnd this one which is an angle we have to sort of sketch in a right triangle and say okay well it's going up four. And over five so we want to find this length. So this is a right triangle.
What is the theorem for isosceles triangles?
Theorem 1: Angles opposite to the equal sides of an isosceles triangle are also equal. Proof: Consider an isosceles triangle ABC where AC = BC. We need to prove that the angles opposite to the sides AC and BC are equal, that is, ∠CAB = ∠CBA. We first draw a bisector of ∠ACB and name it as CD. Now in ∆ACD and ∆BCD we have,
What are the properties of a triangle?
You may have already learnt about the properties and types of triangles. One of the special types of a triangle is the isosceles triangle. An isosceles triangle is a triangle which has two equal sides, no matter in what direction the apex (or peak) of the triangle points. Some pointers about isosceles triangles are: 1 It has two equal sides. 2 It has two equal angles, that is, the base angles. 3 When the third angle is 90 degree, it is called a right isosceles triangle.
What is the theorem 2 of the triangle?
Theorem 2: Sides opposite to the equal angles of a triangle are equal. Proof: In a triangle ABC, base angles are equal and we need to prove that AC = BC or ∆ABC is an isosceles triangle. Construct a bisector CD which meets the side AB at right angles. Now in ∆ACD and ∆BCD we have,
What is the special type of triangle?
One of the special types of a triangle is the isosceles triangle. An isosceles triangle is a triangle which has two equal sides, no matter in what direction the apex (or peak) of the triangle points. Some pointers about isosceles triangles are: It has two equal sides. It has two equal angles, that is, the base angles.
What is the third angle of a triangle called?
When the third angle is 90 degree, it is called a right isosceles triangle.
Why do isosceles triangles require special consideration?
Proofs involving isosceles triangle s often require special consideration because an isosceles triangle has several distinct properties that do not apply to normal triangles. ( More about triangle types) Therefore, when you are trying to prove that two triangles are congruent, and one or both triangles, ...
What is the isosceles triangle theorem?
Isosceles Triangle Theorems. The Base Angles Theorem. If two sides of a triangle are congruent, then the angles opposite those sides are congruent. Converse of the Base Angles Theorem. The converse of the base angles theorem, states that if two angles of a triangle are congruent, then sides opposite those angles are congruent. Proof 1.
What is the angle of an I sosceles triangle?
An i sosceles triangle has two congruent sides and two congruent angles. The congruent angles are called the base angles and the other angle is known as the vertex angle. ∠ BAC and ∠ BCA are the base angles of the triangle picture on the left. The vertex angle is ∠ ABC.
Answer
Answer: Use the distance formula to see if at least two sides are congruent.
New questions in Mathematics
Kay-anna and Amari are making gift bags for Kay-anna's cousin's birthday party. Each bag will have 4 pieces of candy and two small toys. Kay-anna went … to the store with $20 to buy the candy. She found bags that cost $3.79 and have 26 pieces of candy in each.
What formula is used to find the missing coordinate of a right angled triangle?
We use the distance formula and Pythagoras theorem to calculate the missing coordinate of a right-angled triangle.
How Do You Calculate the Area of A Triangle in Coordinate Geometry?
In coordinate geometry, if we need to find the area of a triangle, we use the coordinates of the three vertices. Consider ▵ABC as given in the figure below with vertices A (x 1, y 1 ), B (x 2 2, y 2 2 ), and C (x 3 3, y 3 3 ). In this figure, we have drawn perpendiculars AE, CF, and BD from the vertices of the triangle to the horizontal axis. Notice that three trapeziums are formed: BAED, ACFE, and BCFD.
How to find area of a triangle?
The area of the triangle is the space covered by the triangle in a two-dimensional plane. The formula for the area of a triangle is (1/2) × base × altitude. Let's find out the area of a triangle in coordinate geometry. 1.
What are the three points in a triangle?
Consider these three points: A (−2,1), B (3,2), C (1,5). If you plot these three points in the plane, you will find that they are non-collinear, which means that they can be the vertices of a triangle, as shown below:
Can the area of a triangle be negative?
The area of a triangle cannot be negative. In case we get the answer in negative terms, we should consider the numerical value of the area, without the negative sign. To find the area of a triangle in coordinate geometry, we need to find the length of three sides of a triangle using the distance formula. If three points A (x 1 1 ,y 1 1 ), B (x 2 2 ...