
What are the three Pythagorean identities?
the list of Pythagorean identities Let's explore the Pythagorean identities. The first of these three states that sine squared plus cosine squared equals one. The second one states that tangent squared plus one equals secant squared. For the last one, it states that one plus cotangent squared equals cosecant squared.
How to solve Pythagorean identities?
- Start with this first Pythagorean Identity.
- Divide each term by sin2θ.
- We know and .
- Substitute and simplify.
How many ways are there to prove the Pythagorean theorem?
How many ways are there to prove the Pythagorean Theorem? This theorem states that in a right-angled triangle, the square of the hypotenuse is equal to the sum of each other sides square. There are many proofs which have been developed by a scientist, we have estimated up to 370 proofs of the Pythagorean Theorem.
What are Pythagorean identities?
Pythagorean identities are identities in trigonometry that are extensions of the Pythagorean theorem. Pythagorean identities are useful for simplifying trigonometric expressions. These identities are especially used to write expressions such as a sine or cosine function as double angle formulas.
How do you prove the Pythagorean identities?
The Pythagorean identity tells us that no matter what the value of θ is, sin²θ+cos²θ is equal to 1. We can prove this identity using the Pythagorean theorem in the unit circle with x²+y²=1.
How do you prove an identity equation?
To prove that an equation is an identity, we need to apply known identities to show that one side of the equation can be transformed into the other. To prove that an equation is not an identity, we need to find one input at which the two sides of the equation have different values.
What is the easiest way to prove trigonometric identities?
0:0110:55Trigonometry - Proving trig identities - YouTubeYouTubeStart of suggested clipEnd of suggested clipThere's lots of little connections that might take you from side a to side B. And knowing thoseMoreThere's lots of little connections that might take you from side a to side B. And knowing those identities will make it a little bit easier to recognize maybe what you can swap out.
How do you know if a trigonometric equation is an identity?
To check to whether an equation is an identity we can compare graphs of (left side of the equation) and (right side of the equation). If the two graphs agree, the equation is an identity. If the two graphs are not the same, the equation is not an identity.
How would you prove that an equation is not an identity?
0:1010:22Prove Equation is NOT an Identity 1 - YouTubeYouTubeStart of suggested clipEnd of suggested clipYou need to do do is show that for at least one value you plug in for the variable. The two sides doMoreYou need to do do is show that for at least one value you plug in for the variable. The two sides do not equal the same number.
What can be used to show that an equation is not an identity?
The easiest way to tell whether or not any equation is an identity is by graphing the difference of both sides of the equation.
How do you verify identities in precalculus?
1:4910:25Verifying Trigonometric Identities ❖ Pre-Calculus - YouTubeYouTubeStart of suggested clipEnd of suggested clipSomething like this that this is equal to the cosine squared of X that comes from that PythagoreanMoreSomething like this that this is equal to the cosine squared of X that comes from that Pythagorean identity we've got one that says sine squared X plus cosine squared.
What is identity in quadratic equation?
An identity equation is an equation that is always true for any value substituted into the variable. For example, 2 ( x + 1 ) = 2 x + 2 2(x+1)=2x+2 2(x+1)=2x+2 is an identity equation.
What is Pythagorean identity?
Pythagorean identities are identities in trigonometry that are extensions of the Pythagorean theorem . Pythagorean identities are useful for simplifying trigonometric expressions. These identities are especially used to write expressions such as a sine or cosine function as double angle formulas. Here, we will learn about the Pythagorean identities and we will learn to derive them from the Pythagorean theorem. Then, we will look at some practice exercises where we will apply these identities.
How to derive the Pythagorean identities?
We can derive the Pythagorean identities using the unit circle. Recall that the unit circle is a circle with a radius of 1. In this triangle, the x -coordinates are represented by and the y -coordinates are represented by as shown in the following diagram:
How many identities can we derive from Pythagorean identity?
This is the main Pythagorean identity. Using this identity, we can derive two additional identities.
What is the hypotenuse of a right triangle?
We see that the legs of the right triangle in the unit circle have the values of and . Also, the hypotenuse of the triangle is the radius of the circle, which is equal to 1. Therefore, using the Pythagorean theorem, we have:
Is a cotangent reciprocal?
Now, we know that . Also, since the cotangent is the reciprocal of the tangent, this means that . Using these values, we have:
Can we use the second and third Pythagorean identities?
We can use the second and third Pythagorean identities. In addition, we remember that the secant is equal to and the cosecant is equal to . Using this, we have:
What is the Pythagorean identity?
The Pythagorean identity tells us that no matter what the value of θ is, sin²θ+cos²θ is equal to 1. We can prove this identity using the Pythagorean theorem in the unit circle with x²+y²=1. Created by Sal Khan.
How many Pythagorean trig identities are there?
Is there an alternative way to prove the Pythagorean trig identities (there are three of them)?
What does the pink line on the x axis mean?
but cos is the x value and sin the y value. The pink line connects the point you want to find with the x axis (it's a kind of projection of that point on the line), while the green line connects the point with the y axis... hope this helps!
What does it mean when an exponent is on a trig function?
When the exponent is on the trig function, it means to raise the output of the function to the power. However, this solution can also lead to confusion with the inverse trig functions. We write inverse sine as sin^-1 (x). However, raising anything to the -1 is the same as dividing 1 by it.
What is the Pythagorean theorem?
Learn more... The Pythagorean Theorem allows you to work out the length of the third side of a right triangle when the other two are known. It is named after Pythagoras, a mathematician in ancient Greece. The theorem states that the sum of the squares of the two sides of a right triangle equals the square of the hypotenuse: a2 + b2 = c2.
Which theorem only applies to right triangles?
Remember, the Pythagorean Theorem only applies to right triangles. Arrange the triangles so that they form a square with sides a+b. With the triangles placed in this way, they will form a smaller square (in green) inside the larger square with four equal sides of length c, the hypotenuse of each triangle. The larger square has sides of length a+b .
What is a congruent triangle?
Congruent triangles are ones that have three identical sides. Designate the legs of length a and b and hypotenuse of length c. The Pythagorean Theorem states that the sum of squares of the two legs of a right triangle is equal to the square of the hypotenuse, so we need to prove a2 + b2 = c2 . Remember, the Pythagorean Theorem only applies ...
What is the hypotenuse of the original triangles?
The hypotenuse of the original triangles is now the diagonal of the two rectangles formed by the triangles.
How to find the area of a triangle?
First, find the area of each one and then add all three together. Because two of the triangles are identical, you can simply multiply the area of the first triangle by two: 2A1 = 2 (½bh) = 2 (½ab) = ab. The area of the third triangle is A2 = ½bh = ½c*c = ½c2. The total area of the trapezoid is A1 + A2 = ab + ½c2.
How to make a trapezoid congruent?
Divide the trapezoid into three right triangles, two of which are congruent. Divide the base of the triangle into lengths a and b so that two right triangles of lengths a, b, and c is formed. The third triangle will have two sides of length c and a hypotenuse of length d.
What is Pythagorean identity?
Pythagorean identities are equations that write the Pythagorean Theorem in terms of the trig functions.
Why are Pythagorean identities useful?
The Pythagorean identities can be very useful for simplifying complicated trig statements and equations. Memorize them now, and you can save yourself a lot of time down the road!
Why can you add two denominators together?
You can easily add these two together because the denominators are the same.

What Are The Pythagorean Identities?
How to Derive The Pythagorean Identities?
- We can derive the Pythagorean identities using the unit circle. Recall that the unit circle is a circle with a radius of 1. In this triangle, the x-coordinates are represented by cos(θ) and the y-coordinates are represented by sin(θ)as shown in the following diagram: We see that the legs of the right triangle in the unit circle have the values of...
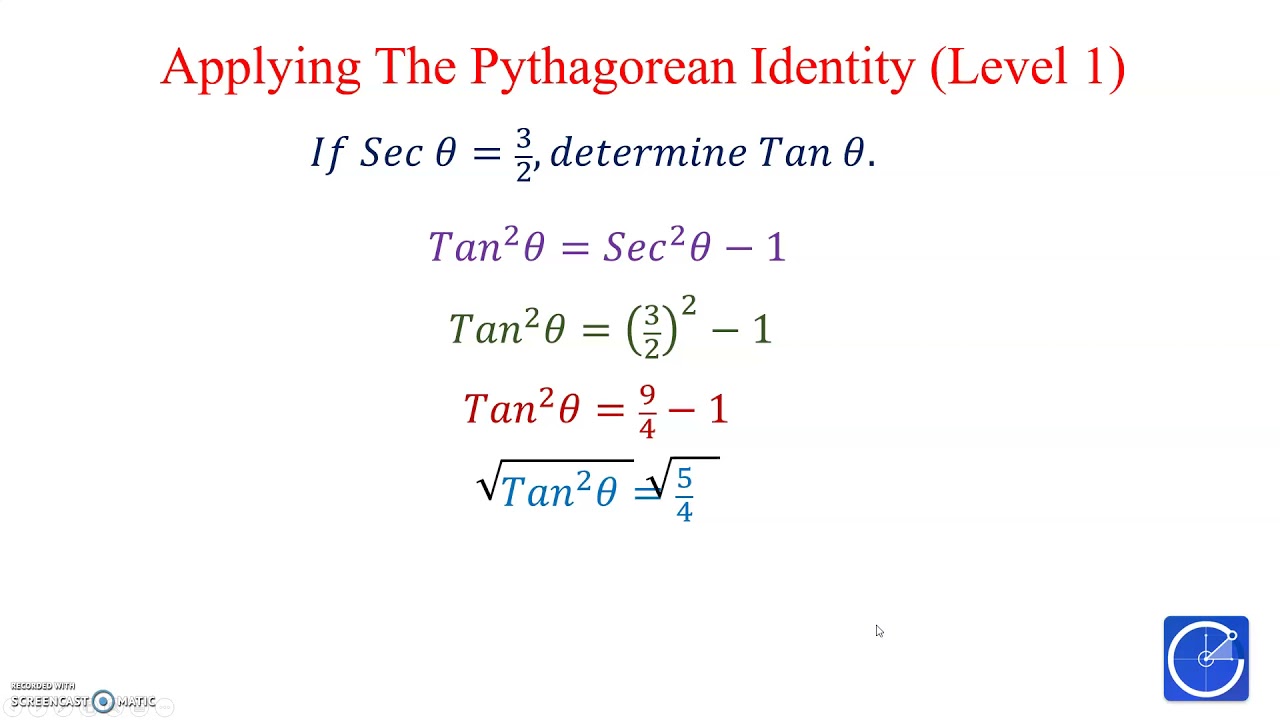
Examples with Answers of The Pythagorean Identities
- The Pythagorean identities derived above are used to solve the following exercises. Try to solve the exercises yourself before looking at the answer.
See Also
- Interested in learning more about trigonometric identities? Take a look at these pages: 1. Reciprocal Identities – Formulas and examples 2. Quotient Identities – Formulas and Examples 3. Formulas for the Trigonometric Identities 4. Fundamental Trigonometric Identities