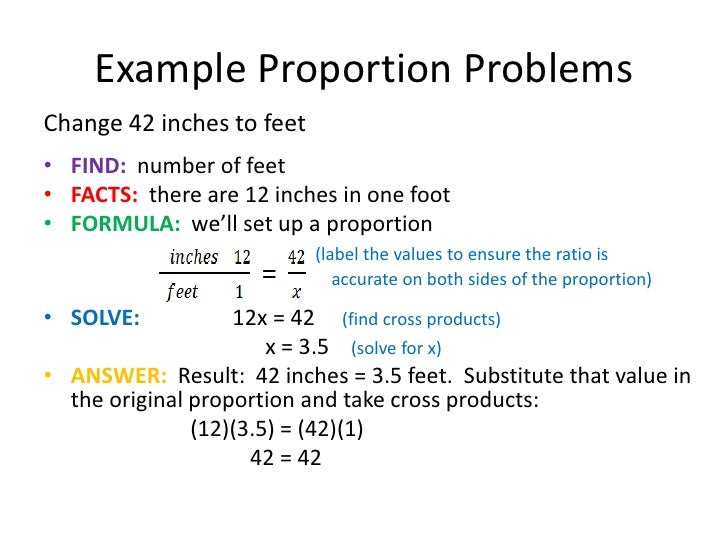
When solving proportion word problems remember to have like units in the numerator and denominator of each ratio in the proportion. Examples: Biologist tagged 900 rabbits in Bryer Lake National Park. At a later date, they found 6 tagged rabbits in a sample of 2000. Estimate the total number of rabbits in Bryer Lake National Park.
- Identify the known ratio and the unknown ratio.
- Set up the proportion.
- Cross-multiply and solve.
- Check the answer by plugging the result into the unknown ratio.
How to solve word problems using proportions?
To solve a problem that requires using proportion, we usually follow this process:
- Assign a variable to the unknown quantity
- Set up a proportion.
- Solve the proportion by cross-multiplying and dividing
How do you solve proportion word problems?
- Use the information in the problem to set up two ratios comparing the same quantities.
- Set the ratios equal creating a proportion.
- Use cross multiplication to solve for the unknown in the proportion.
How do you solve ratio word problems?
To solve ratio problems involving totals, we take these steps:
- Name the unknowns using variables.
- Set up a ratio box with totals using the given information.
- Use the ratio box to set up a proportion.
- Solve the proportion using cross multiplication.
What are some examples of ratio word problems?
Ratio problems: Two-term Ratios. Example 1: In a bag of red and green sweets, the ratio of red sweets to green sweets is 3:4. If the bag contains 120 green sweets, how many red sweets are there? Solution: Step 1: Assign variables: Let x = red sweets. Write the items in the ratio as a fraction. Step 2: Solve the equation.
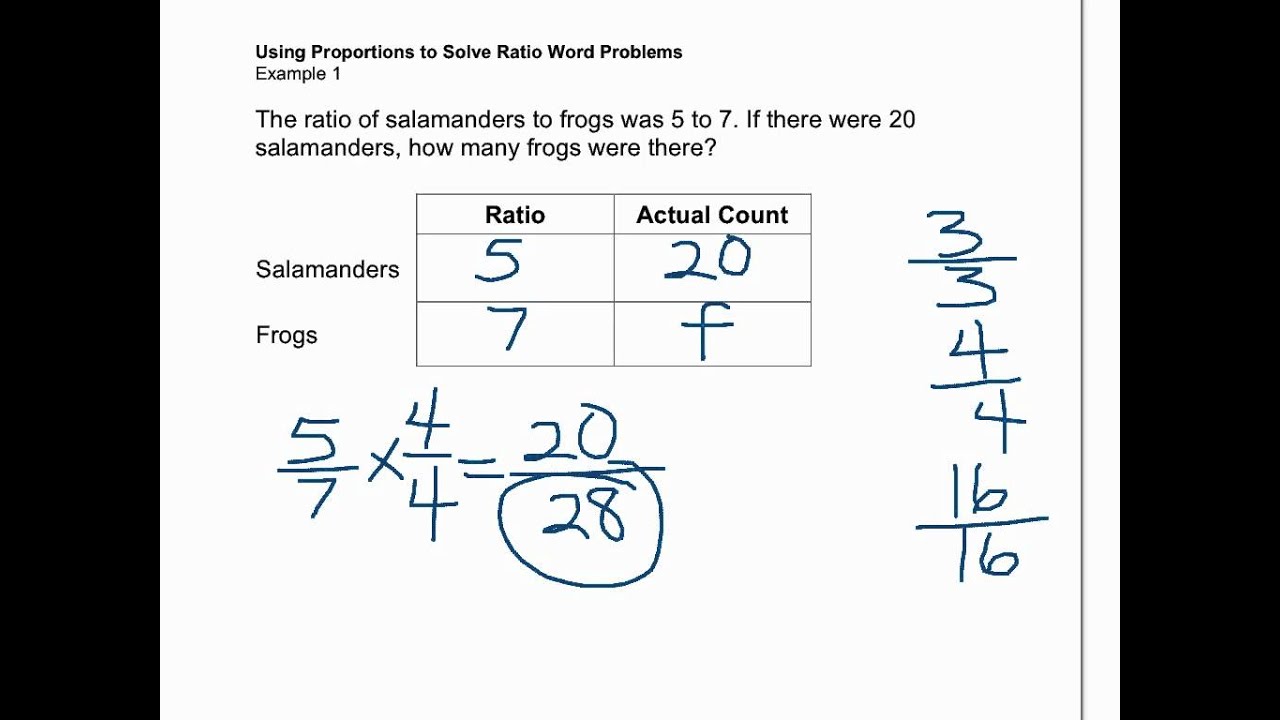
How do you solve ratios and proportions step by step?
1:3419:30Solving Ratio & Proportion Equations - [6-3-5] - YouTubeYouTubeStart of suggested clipEnd of suggested clipNow in order to solve this what we have to do is get the the unknown number x by itself on one sideMoreNow in order to solve this what we have to do is get the the unknown number x by itself on one side of an equal sign any equation that's all we're trying to do but the letter x here is just a
How do you solve a proportion word problem step by step?
1:155:06Math Proportion word problems - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd when you do that you cancel and X is equal to 25. And that is going to be gallons. And it's aMoreAnd when you do that you cancel and X is equal to 25. And that is going to be gallons. And it's a little messy. So it will take 25m up 25 gallons to go 175 miles okay let's look at another example.
How do you find the ratio of a word?
0:045:03How to Solve Ratio Word Problems - YouTubeYouTubeStart of suggested clipEnd of suggested clipWell it's the same ratio. 3 to 6 can be reduced to 1 to 2 because both 3 & 6 are divisible by 3MoreWell it's the same ratio. 3 to 6 can be reduced to 1 to 2 because both 3 & 6 are divisible by 3 which is how we get 1/2. So even though these two ratios.
How do you solve ratios 6th grade?
2:296:076th Grade Math 6.3b, Comparing Ratios - YouTubeYouTubeStart of suggested clipEnd of suggested clipWe multiply the numerator. By the same factor that we multiplied the denominator.MoreWe multiply the numerator. By the same factor that we multiplied the denominator.
How do you solve a proportion equation?
3:217:28Solving Proportions 3 Methods - YouTubeYouTubeStart of suggested clipEnd of suggested clipLet's look at an example B here so X divided by 2 equals 5/8. Our goal is to find out what this X isMoreLet's look at an example B here so X divided by 2 equals 5/8. Our goal is to find out what this X is right so the opposite of dividing by 2 is to multiply by 2 that way those are going to cancel.
What is ratio and proportion examples?
A ratio is an ordered pair of numbers a and b, written a / b where b does not equal 0. A proportion is an equation in which two ratios are set equal to each other. For example, if there is 1 boy and 3 girls you could write the ratio as: 1 : 3 (for every one boy there are 3 girls)
How do you solve math word problems?
0:447:25easy system to solve word problems.wmv - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo we are going to use the acronym of a buck bu CK to remember how to break down a word problem. AndMoreSo we are going to use the acronym of a buck bu CK to remember how to break down a word problem. And I think buck is easy to remember because after all we all remember. Money.
How do you solve ratios in simplest form?
0:483:18What is the Simplest Form of a Ratio? | Don't Memorise - YouTubeYouTubeStart of suggested clipEnd of suggested clipWe divide the numerator as well as the denominator by the HCF. We get the simplest.MoreWe divide the numerator as well as the denominator by the HCF. We get the simplest.
What is proportion and examples?
A proportion is an equation in which two ratios are set equal to each other. For example, if there is 1 boy and 3 girls you could write the ratio as: 1 : 3 (for every one boy there are 3 girls) 1 / 4 are boys and 3 / 4 are girls. 0.25 are boys (by dividing 1 by 4)
How do you write a proportion in geometry?
1:187:19How to Geometry: 7.1. Ratios and Proportions - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo as an example if I said the ratio 3 to 5 or 3/5 is equal to 27 over X the ratio of 27 to X IMoreSo as an example if I said the ratio 3 to 5 or 3/5 is equal to 27 over X the ratio of 27 to X I could solve this I could solve this ratio.
How do you set up a proportion with percentages?
1:363:46Percents and Proportions - Set up Proportion - YouTubeYouTubeStart of suggested clipEnd of suggested clipOver 100 now you notice this sets up our proportion 16. Over 20 equals x over 100 which we could useMoreOver 100 now you notice this sets up our proportion 16. Over 20 equals x over 100 which we could use to solve for X to answer the question.
How do you set up a proportion in geometry?
2:474:12Proportions in Similar Triangles - Geometry - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo we have 5 times X is 5x equals. And we're multiplying 3. Times 15 which is 45. So now to solveMoreSo we have 5 times X is 5x equals. And we're multiplying 3. Times 15 which is 45. So now to solve for X we just divide both sides by 5.
How can the ratio of a mixture be expressed?
Describe the ratio of a mixture by comparing two of its ingredients. This could be 1 cup of butter to 2 cups of flour, for example, or 1 egg to 1 c...
How can a ratio be expressed?
A ratio can be represented in three ways. For example, the same comparison could be expressed as 4 boys to 7 girls, 4 boys : 7 girls, or as the fra...
How do we find a missing value in two equivalent ratios?
Express each ratio of two quantities by writing its numerical values as a fraction. Remember, that's one of the three ways to write a ratio. Find...
How to solve proportions with unknown ratio?
There are a few different methods we can use to solve proportions with an unknown ratio. However, the easiest and most fail-safe method is to cross-multiply and solve the resulting equation. For the last example, we would have:
How to find ratios?
Ratios are found all around us every day and are simply a comparison between two numbers (e.g., red jellybeans to yellow jellybeans). A proportion is a statement that allows you to find an unknown ratio from a known ratio. In the known ratio, you know both of the numbers. In the unknown ratio, you only know one of the numbers. To solve for the unknown number, set up a proportion with the known ratio on one side and the unknown ratio on the other, cross multiply, and solve the resulting equation. This method works every single time, so long as you have identified the known and unknown ratios correctly.
What Is a Proportion?
By itself, a ratio is limited to how useful it is. However, when two ratios are set equal to each other, they are called a proportion. For example, 1/2 is a ratio and 3/6 is also a ratio. If we write 1/2 = 3/6, we have written a proportion. We can also say that 1/2 is proportional to 3/6. In math, a ratio without a proportion is a little like peanut butter without jelly or bread.
How to use ratios in real life?
In math problems and in real life, if we have a known ratio comparing two quanti ties, we can use that ratio to predict another ratio, if given one half of that second ratio. In the example 1/2 = 3/?, the known ratio is 1/2. We know both terms of the known ratio. The unknown ratio is 3/?, since we know one term, but not the other (thus, it's not yet a comparison between two ratios). We only know one of the two terms in the unknown ratio. However, if we set them as a proportion, we can use that proportion to find the missing number.
How to solve for unknown ratio?
To solve for the unknown number, set up a proportion with the known ratio on one side and the unknown ratio on the other, cross multiply, and solve the resulting equation.
What is ratio in math?
A ratio is a comparison between two numbers. To keep it simple, we'll ignore the units (e.g., cost in dollars or weight in ounces) and focus just on the number part for a bit. For example, how does 3 compare to 6? Well, three is half of six. We can write ratios in one of three ways:
How many pounds of tomatoes are needed for a tomato plant?
You will need 8.75 pounds of tomatoes.
How to get original quantity from ratio?
From the above point, it is very clear that if we want to get original quantity from the ratio, we have to multiply both the terms of the ratio by the same number.
What is the increment ratio of 3/1?
Therefore, the increment ratio from '3/1' is 1 : 3.
What Is a Ratio?
Let's say a family is driving in a car at 60 miles per hour. What does that actually mean? The phrase "60 miles per hour" is a ratio that means the car would travel 60 miles in one hour. A ratio is a comparison between two numbers, often numbers of objects or measuring units. They surround us in our daily lives.
Ratio Problem Solving
Ratio problem solving means solving for missing values or even creating a new ratio from the information given. Keep in mind, the units are very important. They explain what the numbers mean and are necessary to compare the correct values. As we proceed, we'll use the ratio problem below to clarify understanding and solving for ratios.
How to Find Ratios in Word Problems
Word problems in math are common but sometimes confusing. Let's examine how to find ratios in a ratio word problem.
How to Solve Ratios
From the important information found in the word problem, write the ratios in their three different forms. Remember, the ratios just compare two numbers.
How to Do Ratios with Unknown Variables
Now that all the ratios have been found and simplified, it's time to answer the question asked. We want to know how much mix would be needed if 24 cups of water were used. To solve this, set up a proportion. A proportion states that two ratios are equal to each other.
Ratio Word Problems in Real Life
Imagine you are going to host a large dinner party for 20 people, including yourself. You have a lot of favorite recipes you want to make, but none of them are written to serve 20 people. Can you use your skills in solving ratio word problems to figure out the quantities you would need for the ingredients listed below?
Problems
1) Your recipe for chocolate chip cookies uses 1 cup of flour for 12 cookies. How much flour will you need in order to make 20 cookies?
Interesting ratio word problems
In a small business, 40 of the employees are men and 30 of the employees are women. What is ratio of women to men?
Hard ratio word problems
Example #4: Suppose the width of a soccer field 60 meters and the length is 100 meters. What is the ratio in simplest form of the length to the area of the field?
How to simplify ratios?
We can simplify ratios with the same strategy that we use to simplify fractions. In the ratio 36 45 we can divide the numerator and denominator by 9. 36 45 now becomes 4 5 or 4:5.
What is the ratio of 14 boys to 17 girls?
We can express this comparison of boys to girls as a ratio. 14 boys and 17 girls can be described as the ratio 14:17 , or the fraction 14 17. 14 and 17 do not have any factors in common, so 14:17 is in simplest form.
Why do we cross multiply?
Cross multiply in order to determine which pair of ratios are equivalent.
How to compare number of quarters to number of nickels?
We can compare the number of quarters to the number of nickels by setting up a ratio. There are 14 quarters and 7 nickels, so our ratio would be 14:7. Choice B says 14:7, but we should simplify when possible. 14:7 simplifies to 2:1.
Is 3:6 the same as 1:2?
Well, it’s the same ratio. 3:6 can be reduced to 1:2 because both 3 and 6 are divisible by 3, which is how we get 1 2. So, even though these two ratios look different, they are actually the same. Let’s take a look at a few word problems, and practice working through them. There are 7 kids in a classroom with green shirts, 8 with red shirts, ...
