-If there is only one squared term, it is a parabola -If one of the squared terms has a negative coefficient, it is a hyperbola -If the coefficients on x2 and y2 don't match but they still have coefficients that either both positive or both negative, it is a ellipse
What is the difference between a hyperbola and an ellipse?
-If one of the squared terms has a negative coefficient, it is a hyperbola -If the coefficients on x2 and y2 don't match but they still have coefficients that either both positive or both negative, it is a ellipse
How do you know if an equation is an ellipse?
-If the coefficients on x2 and y2 don't match but they still have coefficients that either both positive or both negative, it is a ellipse Compare the given general quadratic equation: 4x2 + 9y2 − 16x +18y − 11 = 0 with the standard form : ax2 + 2hxy + by2 + 2gx + 2f y +c = 0 we get
How do you find the standard form of a hyperbola?
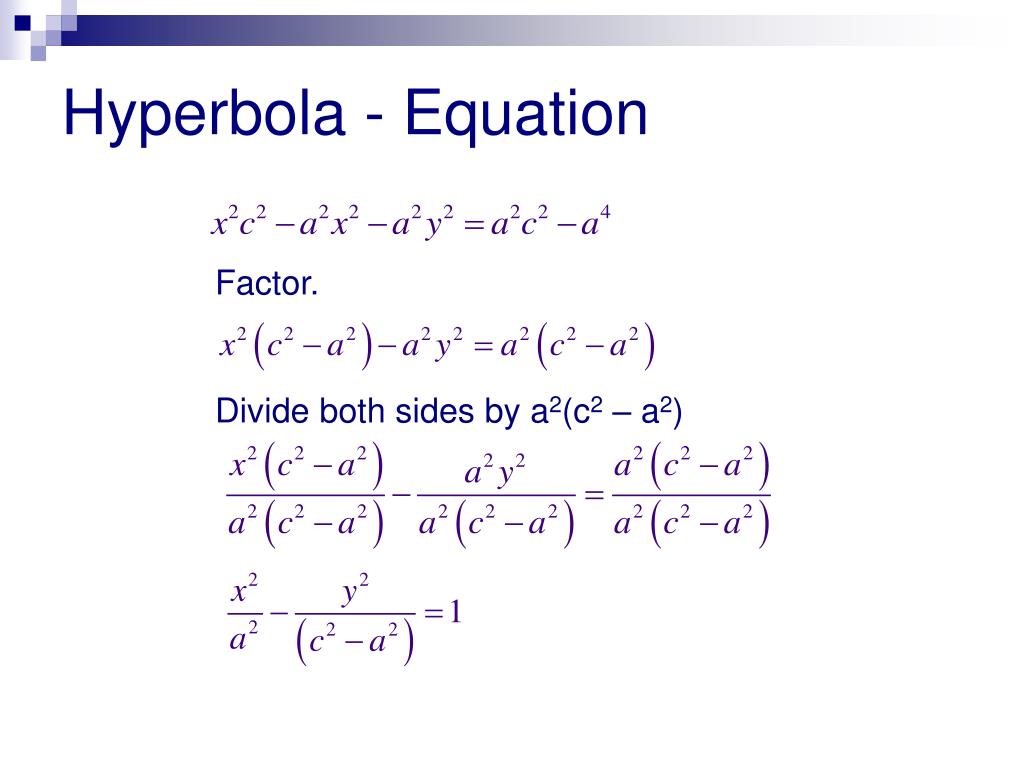
Therefore the equation of the ellipse becomes x2 a2 − y2 b2 = 1 x 2 a 2 − y 2 b 2 = 1. Finally, if the center of the hyperbola is moved from the origin to the point (h,k) ( h, k), we have the following standard form of a hyperbola. Consider the hyperbola with center (h,k) ( h, k), a horizontal major axis, and a vertical minor axis.
How do you find the eccentricity of a hyperbola?
The eccentricity of the parabola is greater than one; e > 1. If the principal axes are coinciding with the Cartesian axes, the general equation of the hyperbola is of the form: x 2/a 2 – y 2/b 2 = 1, where a is the semi-major axis and b is the distance from the center to either focus.
How do you identify if a equation is a ellipse?
How to identify an ellipse from the general equation of a conic section? Consider the general equation of the conic section Ax2+Bxy+Cy2+Dx+Ey+F = 0. If A and C are non zero and unequal, and have the same sign, then the given conic is an ellipse.
How do you know if something is a hyperbola?
If the squared x term and the squared y term are opposite signs (one is positive and one is negative), then you have a hyperbola. If the squared x term and the squared y term have the same constant multiplier (for example, 3x2 + 3y2), then you have a circle. The only other choice is an ellipse.
How can you tell the difference between a parabola and an ellipse?
If the plane intersects one of the pieces of the cone and its axis but is not perpendicular to the axis, the intersection will be an ellipse. To generate a parabola, the intersecting plane must be parallel to one side of the cone and it should intersect one piece of the double cone.
Can a hyperbola be an ellipse?
A hyperbola is related to an ellipse in a manner similar to how a parabola is related to a circle. Hyperbolas have a center and two foci, but they do not form closed figures like ellipses.
How do you prove an equation is a hyperbola?
Derivation of the Equation Therefore, a – (c/a)x becomes negative. Therefore, PF1 – PF2 = {a + x(c/a)} – {x(c/a) – a} = a + x(c/a) – x(c/a) + a = 2a. In this case, PF1 – PF2 = 2a. Hence, it is evident that any point that satisfies the equation x2/a2 – y2/b2 = 1, lies on the hyperbola.
What does hyperbola look like?
Hyperbolas consist of two vaguely parabola shaped pieces that open either up and down or right and left. Also, just like parabolas each of the pieces has a vertex. Note that they aren't really parabolas, they just resemble parabolas. There are also two lines on each graph.
What does an ellipse shape look like?
An ellipse is a circle that has been stretched in one direction, to give it the shape of an oval.
What are the 3 properties of an ellipse?
The following are the important properties of the Ellipse.Center: The point of intersection of the major axis and the minor axis is the center.Focus: The fixed point on the Ellipse is called the focus.Major Axis: The longest diameter of the Ellipse.Minor Axis: The shortest diameter of the Ellipse.More items...
What is the rule for a hyperbola?
The equation of a hyperbola written in the form (y−k)2b2−(x−h)2a2=1. The center is (h,k), b defines the transverse axis, and a defines the conjugate axis. The line segment formed by the vertices of a hyperbola. A line segment through the center of a hyperbola that is perpendicular to the transverse axis.
What is the rule of ellipse?
An ellipsis (plural: ellipses) is a punctuation mark consisting of three dots. Use an ellipsis when omitting a word, phrase, line, paragraph, or more from a quoted passage. Ellipses save space or remove material that is less relevant.
How do you classify a hyperbola?
3:525:08Learn how to classify conic sections - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd if you determine that one of them is negative their product is going to be less than zeroMoreAnd if you determine that one of them is negative their product is going to be less than zero therefore you have a hyperbola.
How do you identify a hyperbola and a parabola?
A parabola is defined as a set of points in a plane which are equidistant from a straight line or directrix and focus. The hyperbola can be defined as the difference of distances between a set of points, which are present in a plane to two fixed points, is a positive constant. A parabola has single focus and directrix.
What is a hyperbola example?
A hyperbola is the set of points in a plane whose distances from two fixed points, called its foci (plural of focus), has a difference that is constant. For example, the figure shows a hyperbola with foci at (-3,0) and (3,0). Its constant distance is 4, as shown by the point at (-3,-2.5).
What objects are hyperbola?
Lens, monitors, and optical glasses are of hyperbola shape.
What is the difference between an ellipse and a hyperbola?
What is the difference between Hyperbola and Ellipse? • Both ellipses and hyperbola are conic sections, but the ellipse is a closed curve while the hyperbola consists of two open curves. • Therefore, the ellipse has finite perimeter, but the hyperbola has an infinite length.
What is the eccentricity of the ellipse?
Eccentricity e is defined as the ratio between the distance from a focus to the arbitrary point ( PF2 ) and the perpendicular distance to the arbitrary point from the directrix ( PD ). It is also equal to the distance between the two foci and the semi-major axis: e = PF/PD = f/a
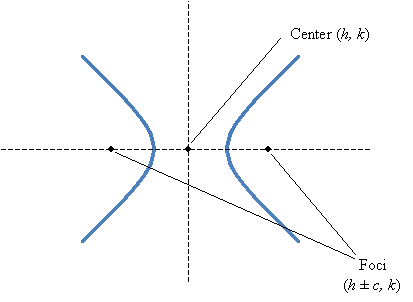
What is the term for the two disconnected curves shown in the figure?
The term hyperbola is referred to the two disconnected curves shown in the figure. Rather than closing like an ellipse the arms or the branches of the hyperbola continue to the infinity. The points where the two branches have the shortest distance between them are known as the vertices.
What is the line passing through the vertices?
The line passing through the vertices is considered as the major axis or the transverse axis, and it’s one of the principal axes of the hyperbola. The two foci of the parabola also lie on the major axis. The midpoint of the line between the two vertices is the center, and the length of the line segment is the semi-major axis.
What is the midpoint of a hyperbola?
The midpoint of the line between the two vertices is the center, and the length of the line segment is the semi-major axis. The perpendicular bisector of the semi-major axis is the other principal axis, and the two curves of the hyperbola are symmetric around this axis.
What is a conic section?
More precisely, a conic section is a curve obtained by intersecting a right circular conic surface with a plane surface. At different angles of intersection, different conic sections are given.
What is the line segment passing through the foci?
The line segment passing through the foci is known as the major axis, and the axis perpendicular to the major axis and passing through the center of the ellipse is known as the minor axis. The diameters along each axis are known as the transverse diameter and the conjugate diameter respectively.
What is the conic section of parabola?
Parabola Ellipse and Hyperbola come under the conic section topic. A conic section is the locus of a point that bears a fixed ratio from a particular point
What is the point of intersection of a conic section and its axis called?
Ans.2 The point of intersection of a conic section and its axis is called the vertex of the conic section. Q.3 State the standard forms of the parabola. Ans.3 The standard forms of the parabola are as follow;
What is the locus of a point that is equidistant from a fixed point named focus?
A parabola is defined as the locus of a point that is equidistant from a fixed point named focus and from a fixed straight line named the directrix.
What is hyperbola in math?
A hyperbola is defined as the locus of a point that travels in a plane such that the proportion of its distance from a fixed position (focus) to a fixed straight line (directrix) is constant and larger than unity i.e eccentricity e > 1.
What is the fixed point in a conic section?
The fixed point is termed as the focus of a conic section .
What is the major axis?
Major axis: Major axis is defined as the line joining the two vertices of an ellipse, starting from one side of the ellipse passing through the center, and ends to the other side. The Major Axis is also called the longest diameter.
What is the principal axis of an ellipse?
Principal Axis: It is the Line joining the two focal points/foci of ellipse/ hyperbola. Its midpoint is termed as the center of the curve. Major axis: Major axis is defined as the line joining the two vertices of an ellipse , starting from one side of the ellipse passing through the center, and ends to the other side.
What is the coordinates of the center of an ellipse?
For ellipse with horizontal major axis: (x-h)^2/a^2+ (y-k)^2/b^2=1, a>b, (h,k) being the ( x,y) coordinates of the center.
What are the standard forms for each function?
The following are the standard forms for each function: .. Circle: (x-h)^2+ (y-k)^2=r^2, (h,k) being the (x,y) coordinates of the center, r=radius. Ellipse: Two standard forms:
Is the form identical to the ellipse?
Also note the form is identical to that of the ellipse except for the negative sign.
General Equation of Conic For Second Degree
Parabola
- A parabola is defined as the locusof a point that is equidistant from a fixed point named focus and from a fixed straight line named the directrix.
Ellipse
- An ellipseis defined as the locus of a point that travels in a plane such that the ratio of its distance from an established point (focus) to a fixed straight position (directrix) is constant and less than unity i.e eccentricity e < 1. Principal Axis:It is the Line joining the two focal points/foci of ellipse/ hyperbola. Its midpoint is termed as t...
Hyperbola
- A hyperbolais defined as the locus of a point that travels in a plane such that the proportion of its distance from a fixed position (focus) to a fixed straight line (directrix) is constant and larger than unity i.e eccentricity e > 1.
Real-Life Applications of Conics
- Planets revolve around the sun in elliptical paths at the single focus.
- Mirrors employed to focus light rays at a point are parabolic.
- The route traversed by an object launched into the air and stretched arc of a rocket launch is parabolic.
- Telescopes use parabolic mirrors.