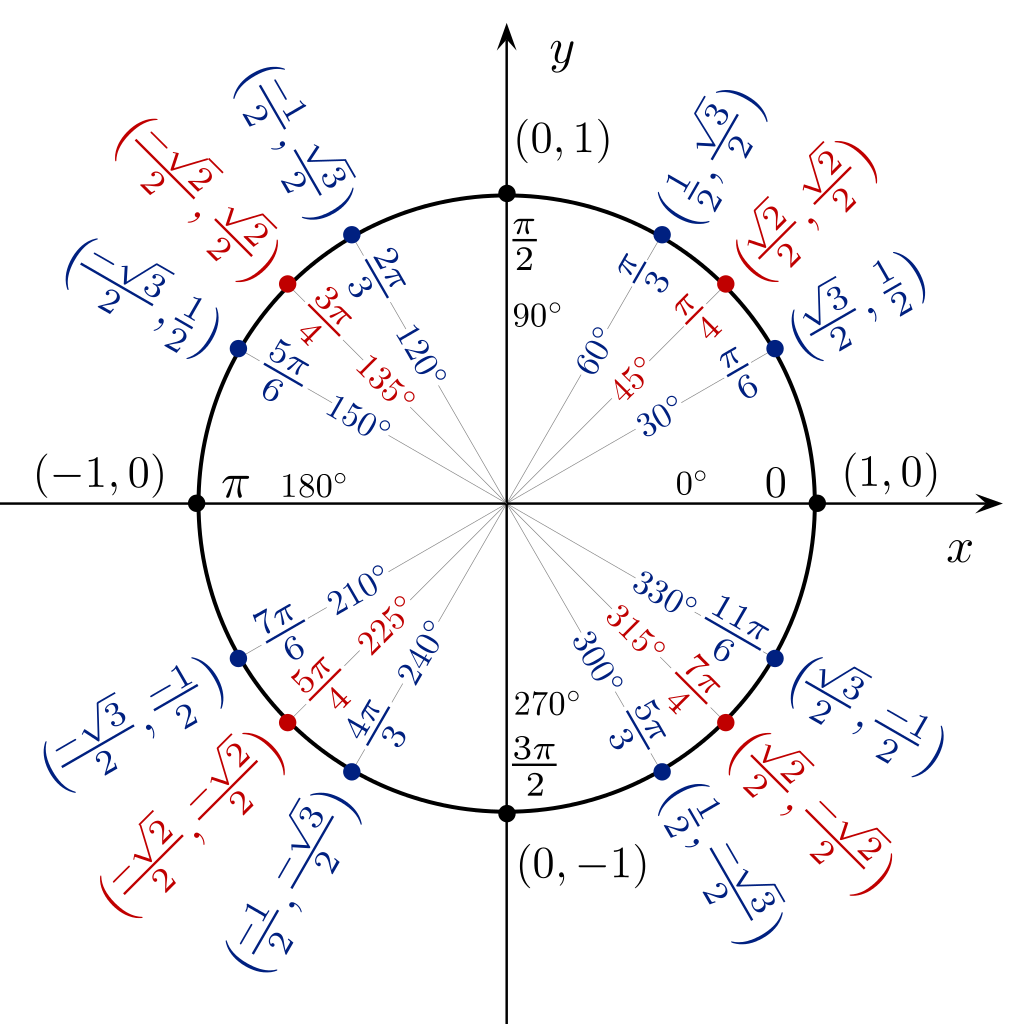
To help you remember the unit circle chart and apply it correctly, here are some useful tips:
- Focus On The Common Angles And Their Coordinates: To use the unit circle correctly, make sure to have the common angles at your fingertips. This means mastering their radians and degree and their coordinates. ...
- Make Sure To Grasp What Is Negative And Positive: in order to solve any trigonometric problem, it is crucial to know which y-coordinates and x-coordinates are negative. ...
What is a unit circle in calculus?
In mathematics, a unit circle is a circle of unit radius—that is, a radius of 1. Frequently, especially in trigonometry, the unit circle is the circle of radius 1 centered at the origin (0, 0) in the Cartesian coordinate system in the Euclidean plane.
How is the unit circle used in math?
If you're studying or preparing for trigonometry, you'll need to know the unit circle. This circle serves as an essential tool used to solve angular sines, cosine, and tangents, ultimately the lengths of triangles.
Is the a unit circle in AP calculus?
The unit circle is a circle with radius 1 that is representative of trigonometric values of the cosine and sine functions expressed in radians. While any value could be found on the unit circle, we will focus on values of common angles around the circle. How is the unit circle made?
How do you solve equations using the unit circle?
1:014:38Solve a Basic Trig Equation Using the Unit Circle and Reference TrianglesYouTubeStart of suggested clipEnd of suggested clipSo again our goal is to find angles on the interval from zero to two pi that have a cosine functionMoreSo again our goal is to find angles on the interval from zero to two pi that have a cosine function value of negative one half well on the unit circle the x coordinate is equal to cosine theta.
What is the fastest way to learn the unit circle?
To memorize the unit circle, use the acronym ASAP, which stands for "All, Subtract, Add, Prime." Each word represents a different quadrant in the unit circle. "All" corresponds with the top right quadrant in the circle, or the first quadrant.
How is the unit circle related to trigonometric functions?
The circumfrence of the unit circle is 2Π. An arc of the unit circle has the same length as the measure of the central angle that intercepts that arc. Also, because the radius of the unit circle is one, the trigonometric functions sine and cosine have special relevance for the unit circle.
Do I need to know unit circle for calculus?
If you're studying trig or calculus—or getting ready to—you'll need to get familiar with the unit circle. The unit circle is an essential tool used to solve for the sine, cosine, and tangent of an angle.
How much trig do you need to know for calculus?
In AP Calculus you should memorize the six trig function values above. from which we can derive other useful trig formulas. In the AP- world, the answer does not need to be rationalized (Hooray!). It is sometimes easier (at least for me) to recall trig values using triangles rather than using the unit circle.
Is there a lot of trig in calculus?
There are many important trig formulas that you will use occasionally in a calculus class. Most notably are the half-angle and double-angle formulas.
How do you find sin and cos on the unit circle?
Using the unit circle, the sine of an angle t equals the y-value of the endpoint on the unit circle of an arc of length t whereas the cosine of an angle t equals the x-value of the endpoint.
What is the importance of learning unit circle?
As stated above, the unit circle is helpful because it allows us to easily solve for the sine, cosine, or tangent of any degree or radian. It's especially useful to know the unit circle chart if you need to solve for certain trig values for math homework or if you're preparing to study calculus.
How can you use trigonometry in real life?
Trigonometry can be used to roof a house, to make the roof inclined ( in the case of single individual bungalows) and the height of the roof in buildings etc. It is used naval and aviation industries. It is used in cartography (creation of maps). Also trigonometry has its applications in satellite systems.
Why was the unit circle created?
To simplify computations, mathematicians like to fit an angle's triangle into a circle with radius r = 1. Because the number 1 is called "the unit" in mathematics, a circle with a radius of length 1 is called "the unit circle".
Where do unit circle values come from?
The general equation of a circle is (x - a)2 + (y - b)2 = r2, which represents a circle having the center (a, b) and the radius r. This equation of a circle is simplified to represent the equation of a unit circle. A unit circle is formed with its center at the point(0, 0), which is the origin of the coordinate axes.
Why is the unit circle important?
There are a number of advantages why you should grasp the concept: The main advantage of the unit circle is that it allows you to solve for cosine, tangent, and cosine of any radian or degree. That is very important, especially in your mathematics and physics classes.
Why do we use unit circles?
The unit circle helps to simplify learning mathematics without having to memorize a lot of concepts. In most of the cases, students are required to memorize over 15 angles as well as their values to correctly get the cosine, sine, and tangent of angles. But they do not need to memorize all of these when using the unit circle. They simply need to conceptualize its application.
What is the simplest way to solve ratios?
The unit circle is considered the simplest way to solve for these ratios. In this guide, we will dig deeper into the unit circle to establish what it is, how it works, how to remember, and how to use unit circle to solve trig problems.
What is trigonometric ratio?
The definition of trigonometric ratios operates within the limits of right angles. If you take the three interior angles of a triangle (right triangle), they always sum up to 180º. Besides, since one of the angles measures 90º, the remaining two must be acute.
Do you need to memorize trigonometry?
But they do not need to memorize all of these when using the unit circle. They simply need to conceptualize its application. Unlike other subjects, trigonometry is a broad subject. In real life, you will need it in careers such as construction, aerodynamics, shooting, and engineering.
Can you use the unit circle in calculus?
Are you in a calculus/trigonometry class, or planning to join one? If the answer is “yes,” it is prudent to understand and be able to use the unit circle. That is one of the most important mathematical tools for helping you to easily solve for cosine, sine, or tangent of an angle. But how does the unit circle work?
Can you use a calculator in calculus?
To demonstrate how it works, take a look at the problem below. Note that in most of the cases, you will not be allowed to use a calculator.
What is the unit circle in calculus?
The unit circle is an essential tool used to solve for the sine, cosine, and tangent of an angle.
Why is the unit circle useful?
As stated above, the unit circle is helpful because it allows us to easily solve for the sine, cosine, or tangent of any degree or radian. It's especially useful to know the unit circle chart if you need to solve for certain trig values for math homework or if you're preparing to study calculus. But how exactly can knowing the unit circle help you?
How to find the cosine of a right triangle?
We know that the cosine of an angle is equal to the length of the horizontal line, the sine is equal to the length of the vertical line, and the hypotenuse is equal to 1. Therefore, we can say that the formula for any right triangle in the unit circle is as follows: cos 2 θ + sin 2 θ = 1 2. Since 1 2 = 1, we can simplify this equation like this:
How to find the tangent of an angle?
In trig, to find the tangent of an angle θ (in either degrees or radians), you simply divide the sine by the cosine:
What is the cosine of a triangle?
On this triangle, the cosine is the horizontal line, and the sine is the vertical line. In other words, cosine = x-coordinate, and sine = y-coordinate. (The triangle’s longest line, or hypotenuse, is the radius and therefore equals 1.)
What is the radius of a unit circle?
The Unit Circle: A Basic Introduction. The unit circle is a circle with a radius of 1. This means that for any straight line drawn from the center point of the circle to any point along the edge of the circle, the length of that line will always equal 1. (This also means that the diameter of the circle will equal 2, ...
How to find the length of a line?
Here's what these lengths mean: 1 Short horizontal or vertical line =#N#1#N#2 2 Medium horizontal or vertical line =#N#√ 2#N#2 3 Long horizontal or vertical line =#N#√ 3#N#2
When the angle is close to zero, the tangent line is near vertical?
When the angle is close to zero the tangent line is near vertical and the distance from the tangent point to the x-axis is very short. As the angle nears 90 degrees the tangent line becomes nearly horizontal and the distance from the tangent point to the x-axis becomes remarkably long.
How many degrees are in a Pi radian?
Pi radians is equal to 180 degrees. They are two different ways of measuring angles. If you want to know why pi radians is half way around the circle, see this video: https://www.khanacademy.org/math/trigonometry/unit-circle-trig-func/intro-to-radians-trig/v/introduction-to-radians.
What is the tangent of a parabola at 45 degrees?
You are left with something that looks a little like the right half of an upright parabola. At negative 45 degrees the tangent is -1 and as the angle nears negative 90 degrees the tangent becomes an astronomically large negative value. This portion looks a little like the left half of an upside down parabola.
What is a unit circle?
In simple terms, the unit circle is a mathematical tool for making the use of angles and trigonometric functions easier. By understanding and memorizing "the unit circle" we are able to breeze through otherwise calculation-heavy problems, and make our lives a whole lot easier.
What quadrant is sine in?
We are therefore in the third quadrant. Thus, since sine gives us the y coordinate, and we are in the third quadrant, our answer will be negative!
How many practice questions are there in trigonometry?
We have over 250 practice questions in Trigonometry for you to master.
Is memorizing the unit circle easier than you think?
Memorizing the unit circle is actually much easier than you'd think, thanks to a couple little tricks:
Do you need sine to solve cosecant?
Since we're dealing with cosecant, it is important to recognize we will need to use sine values to solve using the equation for cosecant discussed earlier in trick 1. First, however, we need to figure out what quadrant we're in so we know whether our answer for sine will be positive or negative.
What is a Unit Circle?
A unit circle is typically drawn around the origin (0,0) of a X,Y axes with a radius of 1. For a straight line drawn from the circle’s centre point to a point along the circle’s edge, the length of that line is always 1. This also means that the circle’s diameter is equal to 2 because the diameter is equal to twice the length of the radius.
Understanding Its Use
As mentioned above, the unit circle allows you to quickly solve any order or radian sine, cosine, or tangent. Knowing the graph of the circle is especially useful if you need to solve a particular trigger value.
What is a unit circle?
The unit circle is a circle with radius 1 that is representative of trigonometric values of the cosine and sine functions expressed in radians. While any value could be found on the unit circle, we will focus on values of common angles around the circle.
How is a unit circle created?
How is the unit circle made? The unit circle is created so that the circle always has a radius of 1 and is centered at the origin of the coordinate plane. To find the various values of points on the unit circle, the two special right triangles are placed in the circle with one endpoint of the hypotenuse touching the origin and the other endpoint ...
What is the length of the hypotenuse of each special right triangle?
Therefore the length of the hypotenuse of each special right triangle is 1 (when we are dealing with the unit circle).
How to put a special right triangle into a unit circle?
Everything we do in calculus will be in radian measure. Remember, to go from degrees to radians, multiply by the conversion π radians / 180 °. Notice that the point on the unit circle, (x, y), corresponds with a width and height of a triangle.
What is the measure of the angle in radians?
Also notice that a point on the unit circle is not only (x, y), but also (cos θ, sinθ), where θ is the measure of the angle in radians. For the picture above, this tells us that (cos (π/4), sin (π/4)) = (√2/2, √2/2). So we can deduce that cos (π/4) = √2/2 and that sin(π/4) = √2/2.
Which way does the unit circle rotate?
We talk about angles of the unit circle always starting from the positive x-axis and rotating counterclockwise around the circle as shown with the green arrow above.
Do you need to submit a quiz to take a unit circle?
The quiz is not self grading, but will give you an idea of what types of questions I might ask about the unit circle in the future. You do not need to submit the quiz.
What is a unit circle?
The "Unit Circle" is a circle with a radius of 1.
What can we measure with radius?
Because the radius is 1, we can directly measure sine, cosine and tangent.
How to find lengths of x and y?
We can use the equation x2 + y2 = 1 to find the lengths of x and y (which are equal to cos and sin when the radius is 1 ):
What is tan in math?
Well, tan = sin/cos, so we can calculate it like this:
Which theorem states that the square of the long side equals the sum of the squares of the other?
Pythagoras' Theorem says that for a right angled triangle, the square of the long side equals the sum of the squares of the other two sides:
Do you have to remember sin, cos and tan?
You should try to remember sin, cos and tan for the angles 30 °, 45 ° and 60 °. Yes, yes, it is a pain to have to remember things, but it will make life easier when you know them, not just in exams, but other times when you need to do quick estimates, etc.