
Probability and the Law of Large Numbers Theoretical and experimental probabilities are linked by the Law of Large Numbers. This law states that if an experiment is repeated numerous times, the relative frequency, or experimental probability, of an outcome will tend to be close to the theoretical probability of that outcome.
Why is the law of large numbers important in statistics?
So the law of large numbers doesn't just try to determine the average, but it can also determine expected probabilities for other results. The law of large numbers is all about probability, so it is important to understand probability. Probability is how likely something is to happen.
What is the law of relative frequency in statistics?
This law states that if an experiment is repeated numerous times, the relative frequency, or experimental probability, of an outcome will tend to be close to the theoretical probability of that outcome. Here the relative frequency is the quotient of the number of times an outcome occurs divided by the number of times the experiment was performed.
What are the two versions of the law of large numbers?
There are two main versions of the law of large numbers. They are called the weak and strong laws of the large numbers. The difference between them is mostly theoretical. In this section, we state and prove the weak law of large numbers (WLLN).
What are the weak and strong laws of large numbers?
There are two main versions of the law of large numbers. They are called the weak and strong laws of the large numbers. The difference between them is mostly theoretical. In this section, we state and prove the weak law of large numbers (WLLN). The strong law of large numbers is discussed in Section 7.2.
Why is the law of large numbers an important concept in probability and statistics?
The result becomes closer to the expected value as the number of trials is increased. The law of large numbers is an important concept in statistics because it states that even random events with a large number of trials may return stable long-term results.
What does the law of large numbers tell us?
law of large numbers, in statistics, the theorem that, as the number of identically distributed, randomly generated variables increases, their sample mean (average) approaches their theoretical mean.
What does the law of large numbers suggest about random events?
According to the law, the average of the results obtained from a large number of trials should be close to the expected value and tends to become closer to the expected value as more trials are performed.
What does law mean in probability?
In probability theory, the law (or formula) of total probability is a fundamental rule relating marginal probabilities to conditional probabilities. It expresses the total probability of an outcome which can be realized via several distinct events, hence the name.
How is probability related to expected value?
The basic expected value formula is the probability of an event multiplied by the amount of times the event happens: (P(x) * n).
Where do we use large numbers in real life?
Examples of large numbers describing everyday real-world objects include:The number of cells in the human body (estimated at 3.72 × 1013)The number of bits on a computer hard disk (as of 2022, typically about 1013, 1–2 TB)The number of neuronal connections in the human brain (estimated at 1014)More items...
What is the law of large numbers and does it change your thoughts about what will occur on the next toss?
The law of large numbers is a principle of probability according to which the frequencies of events with the same likelihood of occurrence even out, given enough trials or instances. As the number of experiments increases, the actual ratio of outcomes will converge on the theoretical, or expected, ratio of outcomes.
What is an example of law of large numbers?
The law of large numbers states that as a sample size becomes larger, the sample mean gets closer to the expected value. The most basic example of this involves flipping a coin. Each time we flip a coin, the probability that it lands on heads is 1/2.
What does it mean to converge in probability?
Properties. Since F(a) = Pr(X ≤ a), the convergence in distribution means that the probability for Xn to be in a given range is approximately equal to the probability that the value of X is in that range, provided n is sufficiently large.
Which type of mathematical laws are frequently used in the concept of probability?
The law of large numbers connects observed relative frequency with the mathematical concept of probability, and has been proved with increasingly refined bounds on the true probability.
What are the 4 laws of probability?
The Four Probability Rules P(A or B)=P(A)+P(B)−P(A and B) In set notation, this can be written as P(A∪B)=P(A)+P(B)−P(A∩B). Whenever an event is the complement of another event, the Complementary Rule will apply. Specifically, if A is an event, then we have the following rule.
How many types of law of probability are there?
Probability Rules There are three main rules associated with basic probability: the addition rule, the multiplication rule, and the complement rule.
How does a casino benefit from the law of large numbers?
The law is basically that if one conducts the same experiment a large number of times the average of the results should be close to the expected value. Furthermore, the more trails conducted the closer the resulting average will be to the expected value. This is why casinos win in the long term.
What is the law of large numbers quizlet?
law of large numbers. A principle stating that the larger the number of similar exposure units considered, the more closely the losses reported will equal the underlying probability of loss.
Demonstrating the Law of Large Numbers
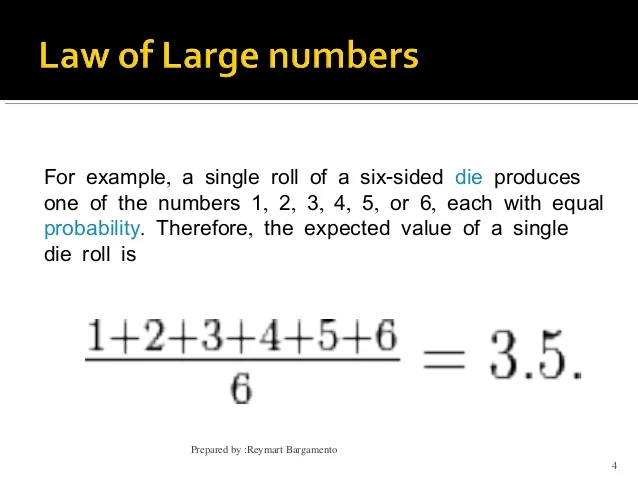
When a fair die is tossed, the likelihood that the number on the top face of the die will be 2 is because only one of the six numbers on the die is a 2. So the theoretical probability of rolling a two is or about 17 percent.
Misconceptions about Probability
If a coin is flipped once and it lands heads up, does that mean it will land tails up next time? Certainly not. The Law of Large Numbers does not apply to any individual flip of the coin, but rather to the long-run behavior. If the coin landed heads up nine times in a row, it cannot be assured that the next flip will show tails.
Bibliography
Berlinghoff, William P., et al. A Mathematics Sampler, 4th ed. New York: Ardsley House Publishers, Inc., 1996.
KERRICH'S COIN TOSSES
Mathematician John Kerrich tossed a coin 10,000 times while interned in a prison camp in Denmark during World War I.
What is the law of large numbers?
In statistics and probability theory, the law of large numbers is a theorem that describes the result of repeating the same experiment a large number of times.
Why is the law of large numbers important?
The law of large numbers is an important concept in statistics. Basic Statistics Concepts for Finance A solid understanding of statistics is crucially important in helping us better understand finance. Moreover, statistics concepts can help investors monitor.
Why is theorem of random events only used with large number of trials?
because it states that even random events with a large number of trials may return stable long-term results. Note that the theorem deals only with a large number of trials while the average of the results of the experiment repeated a small number of times might be substantially different from the expected value.
What is a Fibonacci number?
Fibonacci Numbers Fibonacci Numbers are the numbers found in an integer sequence discovered/created by mathematician, Leonardo Fibonacci. The sequence is a series of numbers. Hypothesis Testing Hypothesis Testing is a method of statistical inference.
What is the law of large numbers?
The law of large numbers states that an observed sample average from a large sample will be close to the true population average and that it will get closer the larger the sample.
Who discovered the law of large numbers?
In the 16th century, mathematician Gerolama Cardano recognized the Law of Large Numbers but never proved it. In 1713, Swiss mathematician Jakob Bernoulli proved this theorem in his book, Ars Conjectandi. It was later refined by other noted mathematicians, such as Pafnuty Chebyshev, founder of the St. Petersburg mathematical school.
Can the law of large numbers be applied to a variety of subjects?
In statistical analysis, the law of large numbers can be applied to a variety of subjects. It may not be feasible to poll every individual within a given population to collect the required amount of data, but every additional data point gathered has the potential to increase the likelihood that the outcome is a true measure of the mean .
Is the law of large numbers the same as the law of averages?
The Law of Large Numbers is not to be mistaken with the Law of Averages, which states that the distribution of outcomes in a sample (large or small) reflects the distribution of outcomes of the population.
Law of Large Numbers Definition
When a single experiment is performed, sometimes the results may show the true average, or actual results, but there is also the chance that it will show an outlier, or another result that is within the normal range, but different from the actual results.
Law of Large Numbers Statistics
The law of large numbers is very important in statistics. Different formulas have been developed, for different purposes, to determine the minimum number of times a trial needs to be repeated in order to use specific statistical tools and models. It is all about probability and determining the probability of an event occurring.
What is Probability
The law of large numbers is all about probability, so it is important to understand probability. Probability is how likely something is to happen. It can be stated as a decimal or a fraction. It is also sometimes stated as a percentage. The probability that a coin will land on heads is one out of two. This can be written as:
Demonstrating The Law of Large Numbers
- When a fair die is tossed, the likelihood that the number on the top face of the die will be 2 is because only one of the six numbers on the die is a 2. So the theoretical probability of rolling a two is or about 17 percent. If a die is tossed six times, a 2 may be rolled more than once or not at all; hence, the percentage of times that a 2 is roll...
Misconceptions About Probability
- If a coin is flipped once and it lands heads up, does that mean it will land tails up next time? Certainly not. The Law of Large Numbers does not apply to any individual flip of the coin, but rather to the long-run behavior. If the coin landed heads up nine times in a row, it cannot be assured that the next flip will show tails. The probability that the next flip of the coin will be head…
Bibliography
- Berlinghoff, William P., et al. A Mathematics Sampler, 4th ed. New York: Ardsley House Publishers, Inc., 1996. Consortium for Mathematics and Its Applications. For All Practical Purposes: Introduction to Contemporary Mathematics, 4th ed. New York: W. H. Freeman and Company, 1997. Devlin, Keith. Life by the Numbers.New York: John Wiley & Sons, Inc., 1998. Moore, David …
Kerrich's Coin Tosses
- Mathematician John Kerrich tossed a coin 10,000 times while interned in a prison camp in Denmark during World War I. At various stages of the experiment, the relative frequency would climb or fall below the theoretical probability of 0.5, but as the number of tosses increased, the relative frequency tended to vary less and stay near 0.5, or 50 percent. For example, Kerrich reco…
What Is The Law of Large numbers?
- The law of large numbers, in probability and statistics, states that as a sample size grows, its mean gets closer to the average of the whole population. In the 16th century, mathematician Gerolama Cardano recognized the Law of Large Numbers but never proved it. In 1713, Swiss mathematician Jakob Bernoulli proved this theorem in his book, Ars Conje...
Understanding The Law of Large Numbers
- In statistical analysis, the law of large numbers can be applied to a variety of subjects. It may not be feasible to poll every individual within a given population to collect the required amount of data, but every additional data point gathered has the potential to increase the likelihood that the outcome is a true measure of the mean. In business, the term "law of large numbers" is sometim…
The Law of Large Numbers and Statistical Analysis
- If a person wanted to determine the average value of a data set of 100 possible values, he is more likely to reach an accurate average by choosing 20 data points instead of relying on just two. For example, if the data set included all integers from one to 100, and sample-taker only drew two values, such as 95 and 40, he may determine the average to be approximately 67.5. If he continu…
Law of Large Numbers and Business Growth
- In business and finance, this term is sometimes used colloquially to refer to the observation that exponential growth rates often do not scale. This is not actually related to the law of large numbers, but may be a result of the law of diminishing marginal returns or diseconomies of scale. For example, in January 2020, the revenue generated by Walmart Inc. was recorded as $523.9 bi…