How do you calculate the angle of a triangle?
a/sin A = b/sin B = c/sin C Here, A, B, and C are the angles of a triangle and a, b, and c are their respective opposite sides. How do you solve angles in math? then How do you find the angle of a number? Divide the number of minutes by 60 and add to the number of degrees. So, for example, 12° 28′ is 12 + 28/60 which equals 12.467°.
How many degrees in one angle of a triangle?
Types of triangles by angle. One angle is 90 degrees. Each of the three angles measure less than 90 degrees. One angle is greater than 90 degrees. Triangles classified by side and angles.
What are the degrees for each angle of a triangle?
The interior angles of a triangle always add up to 180° while the exterior angles of a triangle are equal to the sum of the two interior angles that are not adjacent to it. Another way to calculate the exterior angle of a triangle is to subtract the angle of the vertex of interest from 180°.
How much is each angle in a triangle?
The most basic fact about triangles is that all the angles add up to a total of 180 degrees. The angle between the sides can be anything from greater than 0 to less than 180 degrees. The angles can't be 0 or 180 degrees, because the triangles would become straight lines. (These are called degenerate triangles ).

What type of angle is a triangle?
As the name suggests, a “triangle” is a three-sided polygon having three angles. The sum of all interior angles of a triangle will always add up to 180 degrees. This is called the angle sum property of triangle....Six Types of Triangles.Based on their SidesBased on their AnglesEquilateral TriangleRight Triangle2 more rows•Aug 20, 2020
Do all triangles have angles?
The three interior angles of a triangle will always have a sum of 180°. A triangle cannot have an individual angle measure of 180°, because then the other two angles would not exist (180°+0°+0°). The three angles of a triangle need to combine to 180°.
How many angles are in a triangle?
three anglesThe sum of the three angles of any triangle is equal to 180 degrees.
What is a triangle called?
A triangle is a 3-sided polygon sometimes (but not very commonly) called the trigon. Every triangle has three sides and three angles, some of which may be the same.
How are triangles classified?
If in a triangle, all three sides are unequal, then it is called a Scalene Triangle. If in a triangle, any two sides are equal, then it is called an Isosceles Triangle. If in a triangle, all three sides are equal, then it is called an Equilateral Triangle.
What are the rules for angles of a triangle?
The sum of interior angles in a triangle is 180°.To prove a + b + c = 180 ∘ , firstly draw a line parallel to one side of the triangle.a + d + e = 180 ∘ (angles on a straight line add up to 180°)So a + b + c = 180 ∘ .
What's an angle in math?
Angle Definition in Maths In Plane Geometry, a figure which is formed by two rays or lines that shares a common endpoint is called an angle. The word “angle” is derived from the Latin word “angulus”, which means “corner”. The two rays are called the sides of an angle, and the common endpoint is called the vertex.
What is a true triangle?
A true triangle is a shape that has three sides and three angles. The lengths of two of the sides must add up to a number greater than the third side, and the three angles must add up to 180°.
What are the three angles?
In geometry, there are three types of angles:acute angle-an angle between 0 and 90 degrees.right angle-an 90 degree angle.obtuse angle-an angle between 90 and 180 degrees.straight angle-a 180 degree angle.
Why is it called triangle?
A polygon is a closed plane figure with three or more straight sides. Polygons each have a special name based on the number of sides they have. For example, the polygon with three sides is called a triangle because “tri” is a prefix that means “three.” Its name also indicates that this polygon has three angles.
How many types of angles are there?
In geometry, there are various types of angles, based on measurement. The names of basic angles are Acute angle, Obtuse angle, Right angle, Straight angle, reflex angle and full rotation.
What are the 7 types of triangles?
To learn about and construct the seven types of triangles that exist in the world: equilateral, right isosceles, obtuse isosceles, acute isosceles, right scalene, obtuse scalene, and acute scalene.
What is the 30 60 90 triangle rule?
What is the 30 60 90 Triangle rule? The 30-60-90 triangle rule is for finding the the lengths of two sides when one side is given. The shorter side is opposite the 30 degree angle, the longer side is opposite the 60 degree angle, and the hypotenuse is opposite the 90 degree angle.
What is a unique triangle?
A unique triangle is a polygon that does not have any equivalent. It means that there is no other triangle that has the same dimensions and shape. Those trigonal figures are said to be unique that can be created in only one way. All triangles of this sort are congruent.
How many angles are there?
In Maths, there are mainly 5 types of angles based on their direction. These five angle types are the most common ones used in geometry.
How many exterior angles are there in a triangle?
An exterior angle of a triangle is equal to the sum of the opposite interior angles. Every triangle has six exterior angles (two at each vertex are equal in measure). The exterior angles, taken one at each vertex, always sum up to 360°. An exterior angle is supplementary to its adjacent triangle interior angle.
What is the sum of angles in a triangle?
From the theorem about sum of angles in a triangle, we calculate that γ = 180°- α - β = 180°- 30° - 51.06° = 98.94°
What is an exterior angle?
An exterior angle is supplementary to its adjacent triangle interior angle.
Why are the angles denoted with the same Greek letters congruent?
Look at the picture: the angles denoted with the same Greek letters are congruent because they are alternate interior angles. Sum of three angles α, β, γ is equal to 180°, as they form a straight line. But hey, these are three interior angles in a triangle! That's why α + β + γ = 180°.
What to do if the angle isn't between the given sides?
If the angle isn't between the given sides, you can use the law of sines. For example, assume that we know a, b, α:
Do you have to go from the top to the bottom?
Tip: You don't need to go from the top to the bottom.
What are the two known angles of a triangle?
Two known angles of a triangle are 37° 37 ° and 24° 24 °. What is the missing angle?
How to find the interior angles of a triangle?
Our formula for this is a + b + c = 180° a + b + c = 180 ° where a a, b b, and c c are the interior angles of any triangle.
How to find the missing measurement of a triangle?
You can do this one of two ways: Subtract the two known angles from 180° 180 °. Plug the two angles into the formula and use algebra: a + b + c = 180° a + b + c = 180 °.
What is the proof of a straight line?
And look, they form a straight line! A straight line measures 180° 180 °. This is the same type of proof as the parallel lines proof. The three angles of any triangle always add up to 180° 180 °, or a straight line.
What is the simplest polygon?
A triangle is the simplest possible polygon. It is a two-dimensional (flat) shape with three straight sides forming an interior, closed space. It has three interior angles. One of the earliest concepts to learn in geometry is that triangles have interior angles adding up to 180° 180 °.
How to label a triangle?
Label the inside corners (the vertices that form interior angles) with three letters, like R− A − T R - A - T. Cut the triangle out, leaving a little border around it so you can still see all three edges
What is the formula for 180 degrees?
Now that we've labeled our angles, we have a formula we can refer to for the angles. It is a + b + c = 180° a + b + c = 180 °, which tells us that if we add up all of our angles, they will always equal 180.
What is the relationship between the sides and angles in a triangle?
Rule 3: Relationship between measurement of the sides and angles in a triangle: The largest interior angle and side are opposite each other. The same rule applies to the smallest sized angle and side, and the middle sized angle and side.
What are the properties of a triangle?
Properties of Triangles. Triangles are one of the most fundamental geometric shapes and have a variety of often studied properties including: Rule 1: Interior Angles sum up to 180 0. Rule 2: Sides of Triangle -- Triangle Inequality Theorem : This theorem states that the sum of the lengths of any 2 sides of a triangle must be greater ...
What is the total degree of all interior angles?
No matter how you position the three sides of the triangle, the total degrees of all interior angles (the three angles inside the triangle) is always 180°. This property of a triangle's interior angles is simply a specific example of the general rule for any polygon's interior angles . Interior Angles of Triangle Worksheet.
What is the sum of all 3 angles in a triangle?
This may be one the most well known mathematical rules- The sum of all 3 interior angles in a triangle is 180 ∘ . As you can see from the picture below, if you add up all of the angles in a triangle the sum must equal 180 ∘ .
What is the largest interior angle?
the largest interior angle is opposite the largest side. the smallest interior angle is opposite the smallest side. the middle-sized interior angle is opposite the middle-sized side. To explore the truth of the statements you can use Math Warehouse's interactive triangle , which allows you to drag around the different sides ...
Do isosceles have a smallest side?
(All right, the isosceles and equilateral triangle are exceptions due to the fact that they don't have a single smallest side or, in the case of the equilateral triangle, even a largest side.
What is a triangle?
A triangle is a two-dimensional shape, in Euclidean geometry, which is seen as three non-collinear points in a unique plane. Table of contents: Definition. Angles. Properties. Types. Scalene Triangle. Isosceles Triangle. Equilateral Triangle.
What are the exterior angles of a triangle?
When we extend the sides of the triangle in the outward direction, then the three exterior angles formed are ∠4, ∠5 and ∠6, which are consecutive to ∠1, ∠2 and ∠3, respectively.
What is the most important property of a triangle?
The most important property of a triangle is that the sum of the internal angles of a triangle is equal to 180 degrees . This property is called angle sum property of triangle. If ABC is a triangle, then it is denoted as ∆ABC, where A, B and C are the vertices of the triangle.
What are the three categories of triangles?
On the basis of measurement of the angles, triangles are classified into three categories: 1 Acute Angle Triangle 2 Right Angle Triangle 3 Obtuse Angle Triangle
What is the name of the triangle with 3 sides?
As we discussed in the introduction, a triangle is a type of polygon, which has three sides, and the two sides are joined end to end is called the vertex of the triangle. An angle is formed between two sides. This is one of the important parts of geometry.
How many angles does an obtuse triangle have?
An obtuse triangle has any of its one angles more than 90°.
How many degrees are there in an equilateral triangle?
An equilateral triangle has all three sides equal to each other. Due to this all the internal angles are of equal degrees, i.e. each of the angles is 60°
How to find the measure of an angle in a triangle?
If we add all three angles in any triangle we get 180 degrees. So, the measure of angle A + angle B + angle C = 180 degrees. This is true for any triangle in the world of geometry. We can use this idea to find the measure of angle (s) where the degree measure is missing or not given.
What is the measure of a triangle?
We know that the sum of the measures of any triangle is 180 degrees. Using the fact that angle A + angle B + angle C = 180 degrees , we can find the measure of angle C.
What angle is 40 + 60?
Once you're comfortable with this sort of problem you'll be able to say "okay, 40 + 60 =100, so the other angle has to be 80 !" and it's much quicker.
Can you add angles to get a sum?
Just like regular numbers, angles can be added to obtain a sum, perhaps for the purpose of determining the measure of an unknown angle. Sometimes we can determine a missing angle because we know that the sum must be a certain value. Remember -- the sum of the degree measures of angles in any triangle equals 180 degrees. Below is a picture of triangle ABC, where angle A = 60 degrees, angle B = 50 degrees and angle C = 70 degrees.
What Type of Angle?
Triangles can also have names that tell you what type of angle is inside:
What is the meaning of the scalene triangle?
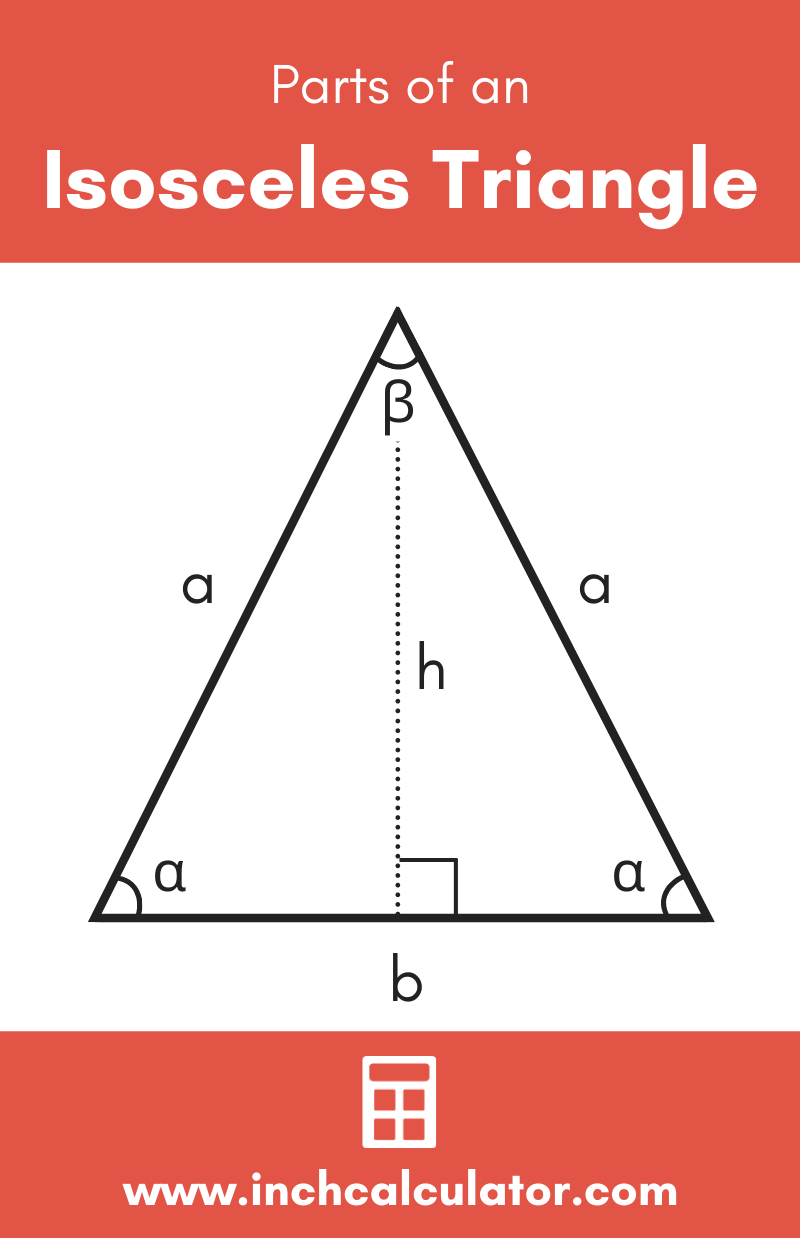
Isosceles: means "equal legs", and we have two legs, right? Also i SOS celes has two equal "S ides" joined by an " O dd" side. Scalene: means "uneven" or "odd", so no equal sides.
What are the names of the three types of triangles?
Equilateral, Isosceles and Scalene . There are three special names given to triangles that tell how many sides (or angles) are equal. There can be 3, 2 or no equal sides/angles:
Can base be any side?
The base can be any side, Just be sure the "height" is measured at right angles to the "base":
What is the circumcenter of a triangle?
The center of this circle, where all the perpendicular bisectors of each side of the triangle meet , is the circumcenter of the triangle, and is the point from which the circumradius is measured. The circumcenter of the triangle does not necessarily have to be within the triangle.
How to find the inradius of a triangle?
In a triangle, the inradius can be determined by constructing two angle bisectors to determine the incenter of the triangle. The inradius is the perpendicular distance between the incenter and one of the sides of the triangle. Any side of the triangle can be used as long as the perpendicular distance between the side and the incenter is determined, since the incenter, by definition, is equidistant from each side of the triangle.
What is a triangle in which all sides have equal lengths?
For example, a triangle in which all three sides have equal lengths is called an equilateral triangle while a triangle in which two sides have equal lengths is called isosceles. When none of the sides of a triangle have equal lengths, it is referred to as scalene, as depicted below.
What is a triangle with vertices?
A triangle is usually referred to by its vertices. Hence, a triangle with vertices a, b, and c is typically denoted as Δabc. Furthermore, triangles tend to be described based on the length of their sides, as well as their internal angles.
What is the unit of an angle?
Angle Unit: degree ° radian. A triangle is a polygon that has three vertices. A vertex is a point where two or more curves, lines, or edges meet; in the case of a triangle, the three vertices are joined by three line segments called edges. A triangle is usually referred to by its vertices. Hence, a triangle with vertices a, b, ...
What is circumradius in math?
circumradius =. a. #N#2sin (A) Where a is a side of the triangle, and A is the angle opposite of side a. Although side a and angle A are being used, any of the sides and their respective opposite angles can be used in the formula. #N#.
How to find the exterior angle of a triangle?
Another way to calculate the exterior angle of a triangle is to subtract the angle of the vertex of interest from 180°. The sum of the lengths of any two sides of a triangle is always larger than the length of the third side. Pythagorean theorem: The Pythagorean theorem is a theorem specific to right triangles.
