The SSA congruence rule states that if two sides and an angle not included between them are respectively equal to two sides and an angle of the other then the two triangles are equal. However, with this congruence rule, two triangles are not said to be congruent since the sides of the two triangles may not be on the same corresponding sides.
Does SSA prove congruence?
Given two sides and non-included angle (SSA) is not enough to prove congruence. You may be tempted to think that given two sides and a non-included angle is enough to prove congruence. But there are two triangles possible that have the same values, so SSA is not sufficient to prove congruence. How do you write a congruence statement for a triangle?
Why is SSA called the ambiguous case?
Working with the third option of SSA, however, leaves the door open for several different situations and solutions to occur. For this reason, SSA is referred to as the Ambiguous Case. Ambiguous means open to two or more interpretations. SSA: If two sides and the non-included angle are given, three situations may occur.
Does SSA prove similarity?
While two pairs of sides are proportional and one pair of angles are congruent, the angles are not the included angles. This is SSA, which is not a similarity
Is it true triangles satisfying SSA are congruent?
We can say that two triangles are congruent if any of the SSS, SAS, ASA, or AAS postulates are satisfied. In this case, we know that two corresponding angles are congruent (∠ B ≅ ∠ Y and ∠ C ≅ ∠ Z) and corresponding segments not in between the angles are congruent ( AB ≅ XY ).
Why SSA is not congruent?
The SSA congruence rule is not possible since the sides could be located in two different parts of the triangles and not corresponding sides of two triangles. The size and shape would be different for both triangles and for triangles to be congruent, the triangles need to be of the same length, size, and shape.
Is SSA always congruent?
If two sides and the following angle (SSA) are congruent between two triangles, that does NOT always mean the triangles are congruent. There are sometimes two ways to arrange the remaining side and angles!.
Is SSA a similarity theorem?
Explain. While two pairs of sides are proportional and one pair of angles are congruent, the angles are not the included angles. This is SSA, which is not a similarity criterion.
Can SAA be congruent?
Angle-Angle-Side (AAS or SAA) Congruence Theorem: If two angles and a non-included side in one triangle are congruent to two corresponding angles and a non-included side in another triangle, then the triangles are congruent.
What is a congruent triangle?
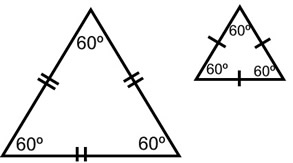
Congruent triangles are triangles that have the same size and shape. This means that the corresponding sides are equal and the corresponding angles are equal. We can tell whether two triangles are congruent without testing all the sides and all the angles of the two triangles. In this lesson, we will consider the four rules to prove triangle ...
What is the SSS postulate?
SSS Postulate: If there exists a correspondence between the vertices of two triangles such that three sides of one triangle are congruent to the corresponding sides of the other triangle, the two triangles are congruent. SAS Postulate: If there exists a correspondence between the vertices of two triangles such that the two sides and ...
What is the SSS rule?
Side-Side-Side (SSS) Rule. Side-Side-Side is a rule used to prove whether a given set of triangles are congruent. The SSS rule states that: If three sides of one triangle are equal to three sides of another triangle, then the triangles are congruent. In the diagrams below, if AB = RP, BC = PQ and CA = QR, then triangle ABC is congruent ...
What are the four rules of congruence?
In this lesson, we will consider the four rules to prove triangle congruence. They are called the SSS rule, SAS rule, ASA rule and AAS rule . In another lesson, we will consider a proof used for right triangles called the Hypotenuse Leg rule. As long as one of the rules is true, it is sufficient to prove that the two triangles are congruent.
