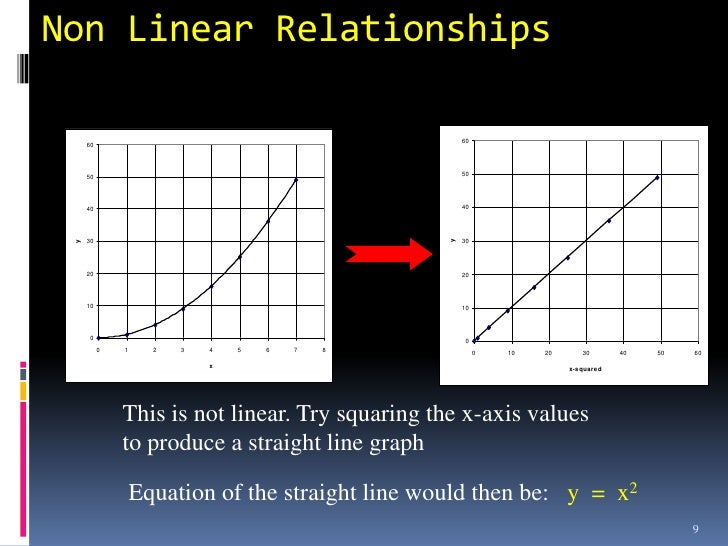
Proportional relationships are relationships between two variables where their ratios are equivalent. Another way to think about them is that, in a proportional relationship, one variable is always a constant value times the other. That constant is know as the "constant of proportionality".
How to tell if a relationship is proportional?
How to Determine Whether Two Variables Are Directly Proportional
- Method 1 Method 1 of 4: Rewriting the Equation of the Line Download Article. Understand direct proportion. ...
- Method 2 Method 2 of 4: Using a Set of Points Download Article. Identify the x-coordinates of the first two points. ...
- Method 3 Method 3 of 4: Using a Graph Download Article. ...
- Method 4 Method 4 of 4: Completing Sample Problems Download Article. ...
How do you know if a relationship is proportional?
The best way to show and explain direct proportional relationships is by graphing two sets of related quantities. If the relation is proportional, the graph will form a straight line that passes through the origin. What two things make a graph proportional? A graph of a proportional relationship is a straight line that passes through the origin.
How to solve proportional relationships?
Proportional relationships can be described by equations of the form y = kx, where k is the constant of proportionality or, when rates are involved, the unit rate. The y variable is what we are trying to find.
How do you graph a proportional relationship?
Graphs of a proportional relationship always start at the (0, 0) point and are always a straight line. Calculate a second point, draw a straight line through the two points, and you have the graph.

What is a proportional relationship example?
Now, we're going to consider an example of proportional relationship in our everyday life: When we put gas in our car, there is a relationship between the number of gallons of fuel that we put in the tank and the amount of money we will have to pay. In other words, the more gas we put in, the more money we'll pay.
How do you know if it is a proportional relationship?
You can tell if a table shows a proportional relationship by calculating the ratio of each pair of values. If those ratios are all the same, the table shows a proportional relationship.
How do you find a proportional relationship in 7th grade?
1:594:36What is a Proportional Relationship? | 7th Grade | Mathcation.comYouTubeStart of suggested clipEnd of suggested clipFor our proportional relationships equation in order to determine if this is proportional we have toMoreFor our proportional relationships equation in order to determine if this is proportional we have to figure out if we are multiplying by the same amount to go from the x column to the y.
What is a proportional relationship for kids?
0:061:57Math Shorts Episode 12 - Proportional Relationships - YouTubeYouTubeStart of suggested clipEnd of suggested clipProportional relationships two quantities are said to be directly proportional if they vary at theMoreProportional relationships two quantities are said to be directly proportional if they vary at the same.
How do you identify a proportional relationship on a graph?
To determine whether x and y have a proportional relationship, see if the line through these points passes through the origin, (0, 0). The points are on a line that passes through the origin. So, x and y have a proportional relationship.
What is not proportional?
NON-PROPORTIONAL. If two quantities are proportional, then they have a constant ratio. If the ratio is not constant, the two quantities are said to be non-proportional. We will make tables and look at the relationship between the variables to determine proportionality.
How do you find the proportional relationship between two fractions?
Trying to figure out if two ratios are proportional? If they're in fraction form, set them equal to each other to test if they are proportional. Cross multiply and simplify. If you get a true statement, then the ratios are proportional!
What does proportional mean in math?
In mathematics, proportionality indicates that two quantities or variables are related in a linear manner. If one quantity doubles in size, so does the other; if one of the variables diminishes to 1/10 of its former value, so does the other.
How do you solve proportions?
To solve proportions, start by taking the numerator, or top number, of the fraction you know and multiplying it with the denominator, or bottom number, of the fraction you don't know. Next, take that number and divide it by the denominator of the fraction you know. Now you can replace x with this final number.
What is proportion 5th grade?
Proportion is explained majorly based on ratio and fractions. A fraction, represented in the form of a/b, while ratio a:b, then a proportion states that two ratios are equal.
What is an example of a non proportional relationship?
The distance a car can travel on a tank of gas or a full battery charge in an electric car depends on factors such as fuel capacity and the car's efficiency. This is described by a nonproportional relationship.
What does it mean for two things to be proportional?
When two quantities are proportional, it means that as one quantity increases the other will also increase and the ratio of the quantities is the same for all values. An example could be the circumference of a circle and its diameter, the ratio of the values would equal \pi.
How do you know if something is proportional or Nonproportional?
0:085:08Proportional vs. Non-Proportional Relationships - YouTubeYouTubeStart of suggested clipEnd of suggested clipThat if it's non proportional. It will not go through the origin. So as you can see with this blueMoreThat if it's non proportional. It will not go through the origin. So as you can see with this blue graph it does not go through the center of the graph it still crosses the x and y axis it.
Is Y 2x proportional or Nonproportional?
A straight line through the origin = proportional. equation is of the form y=kx (y=2x)....x(lbs)y(cost)12342 more rows
Is Y 5x 2 a proportional relationship?
No, it is Partial Variation.
How do you know if something is inversely proportional?
When two quantities are related to each other inversely, i.e., when an increase in one quantity brings a decrease in the other and vice versa then they are said to be inversely proportional. In this, if one variable decreases, the other increases in the same proportion.
What is proportional relationship?
A proportional relationship between two quantities is a collection of equivalent ratios, related to each other by a constant of proportionality. Proportional relationships can be represented in different, related ways, including a table, equation, graph, and written description.
What are some problems that can be solved using proportional reasoning?
There are many applications that can be solved using proportional reasoning, including problems with price increases and decreases, commissions, fees, unit prices, and constant speed.
What is 7.RP.A.2.A?
7.RP.A.2.A — Decide whether two quantities are in a proportional relationship, e.g., by testing for equivalent ratios in a table or graphing on a coordinate plane and observing whether the graph is a straight line through the origin.
Why is proportion important?
The proportion helps us to find how one quantity varies with the other.
What is proportion in math?
Proportion is a mathematical comparison between two numbers. Let's understand the proportionality in detail.
What is the relationship between two quantities?
This type describes the direct relationship between two quantities. In simple words, if one quantity increases, the other quantity also increases and vice-versa.
What is the representation of speed?
It is represented as y ∝ 1/x. For example, increasing the speed of the car will result in covering a fixed distance in less time.
Can we apply proportions to geography?
Similarly, We can apply the concepts of proportions to geography, comparing quantities in physics, dietetics, cooking, etc.
Criteria for Success
Identify the constant of proportionality in a graph by finding the value of y / x from coordinate point ( x, y) .
Tips for Teachers
Students reason abstractly as they represent proportional situations using tables and graphs, and interpret the information to identify the constant of proportionality and write an equation.
Problem Set
The following resources include problems and activities aligned to the objective of the lesson that can be used to create your own problem set.
Target Task
A souvenir shop sells magnets, 2 for $3. The graph below shows the proportional relationship.
What is proportional relationship?
A proportional relationship is where one ratio is the same as another ratio. For example, the ratio 1:2 is the same as 2:4. If you go ahead and divide both of these ratios, you'll get the same relationship 1:2.
How to get to the second number in proportional relationships?
So, in proportional relationships, you have to multiply one of the numbers by the same number each time to get to the second number .
How to find proportionality?
Now comes the math. Once you know your proportional relationship, what you always multiply or divide by, you can use a formula to help you find your equation. This equation is a very easy one: 1 The y stands for your second set of numbers 2 The x stands for your first set of numbers 3 The k is your constant of proportionality.
What is the constant of proportionality?
The constant of proportionality is what number you need to multiply each number in the first column to get to the number in the second column. For your ice cream shop, your constant of proportionality is what you always multiply with the number of ice cream cones to find how much you earned.
What is proportional relationship?
A proportional relationship is one in which two quantities vary directly with each other. We say the variable y varies directly as x if:
What happens to the ratio between x and y?
This means that as x increases, y increases and as x decreases, y decreases-and that the ratio between them always stays the same .