.PNG)
Here are the Laws (explanations follow):
| Law | Example |
| (x/y)n = xn/yn | (x/y) 2 = x 2 / y 2 |
| x-n = 1/xn | x -3 = 1/x 3 |
| x m/n = n√xm = ( n√x ) m | x 2/3 = 3√x2 = ( 3√x ) 2 |
| Law | Example |
|---|---|
| x-1 = 1/x | 4-1 = 1/4 |
| xmxn = xm+n | x2x3 = x2+3 = x5 |
| xm/xn = xm-n | x6/x2 = x6-2 = x4 |
| (xm)n = xmn | (x2)3 = x2×3 = x6 |
What are the 8 rules of exponents?
Rule #1: Multiplying Exponents With the Same Base. Rule #2: Dividing Exponents With the Same Base. Rule #3: Raising a Product to a Power. Rule #4: Raising a Quotient to a Power. Rule #5: Raising an Exponent to an Additional Power. This post is part of the series: Math Help for Exponents.
What are the laws for exponents?
What are the 6 laws of exponents?
- Rule 1 (Product of Powers)
- Rule 2 (Power to a Power)
- Rule 3 (Multiple Power Rules)
- Rule 4 (Quotient of Powers)
- Rule 5 (Power of a quotient)
- Rule 6 (Negative Exponents)
- Quiz.
What are the different rules of exponents?
What are the different rules of exponents? The rules of exponents are the 0 rule, the 1 rule, the power rule for exponents, the negative exponent rule, the product rule, and the quotient rule. They are the rules you use in order to simplify exponent problems and solve problems with exponents.
What are laws of exponents on rational numbers?
Laws of Exponents. Exponents are also called Powers or Indices. The exponent of a number says how many times to use the number in a multiplication. In this example: 8 2 = 8 × 8 = 64. In words: 8 2 could be called "8 to the second power", "8 to the power 2" or simply "8 squared"
What are the 8 laws of exponents with examples?
What are the 8 Laws of Exponents?Zero Exponent Law: a0 = 1.Identity Exponent Law: a1 = a.Product Law: am × an = a. m+nQuotient Law: am/an = a. m-nNegative Exponents Law: a-m = 1/a. mPower of a Power: (am)n = a. mnPower of a Product: (ab)m = amb. mPower of a Quotient: (a/b)m = am/b. m
What are the 7 rules of exponents with examples?
7 Rules for Exponents with ExamplesRULE 1: Zero Property. Definition: Any nonzero real number raised to the power of zero will be 1. ... RULE 2: Negative Property. ... RULE 3: Product Property. ... RULE 4: Quotient Property. ... RULE 5: Power of a Power Property. ... RULE 6: Power of a Product Property. ... RULE 7: Power of a Quotient Property.
What are the 5 laws of exponents?
Laws of ExponentsMultiplying Powers with same Base.Dividing Powers with the same Base.Power of a Power.Multiplying Powers with the same Exponents.Negative Exponents.Power with Exponent Zero.Fractional Exponent.
What are the 6 laws of exponents?
Rule 1 (Product of Powers)Rule 2 (Power to a Power)Rule 3 (Multiple Power Rules)Rule 4 (Quotient of Powers)Rule 5 (Power of a Quotient)Rule 6 (Negative Exponents)Quiz.Logarithms.More items...•
What are the 10 rules of exponents?
The important laws of exponents are given below:am×an = a. m+nam/an = a. m-n(am)n = a. mnan/bn = (a/b) na0 = 1.a-m = 1/a. ma 1 n = a n.
What are the 9 laws of exponents?
Laws of exponents:am × an = a. m+naman a m a n = am-n, m > n.(am)n = a. mn(am × bm) = (a × b) mambm a m b m = (ab ) ma0 = 1.a-n = 1an.
What is the first law of exponent?
Law of Exponents: The first law states that to multiply two exponential functions with the same base, we simply add the exponents.
How many laws of exponents are there in maths?
There are seven exponent rules, or laws of exponents, that your students need to learn. Each rule shows how to solve different types of math equations and how to add, subtract, multiply and divide exponents.
What are the laws of indices with examples?
Index laws are the rules for simplifying expressions involving powers of the same base number. = ( 3 √ 27)2 = (3)2 = 9. (2) Watch out for powers of negative numbers. For example, (−2)3 = −8 and (−2)4 = 16, so (−x)5 = −x5 and (−x)6 = x6.
What are laws of exponents Class 7?
When numbers with the same base are multiplied, the power of the product is equal to the sum of the powers of the numbers. If 'a' is a non-zero integer, and 'm' and 'n' are whole numbers then, am × an = am+n.
What is the 4th law of exponent?
The fourth law of exponents says that "any value other than zero brought to an exponent of zero is equal to one". To check this fourth law of exponents take a calculator and let's check with an example, five to the zero equals one, forty eight to the zero equals one.
How do you solve laws of exponents?
16:2725:57Exponent Rules of Algebra (Laws of Exponents, How to Multiply & Add ...YouTubeStart of suggested clipEnd of suggested clipTogether that's why it becomes X to the twelfth. Another example would be Z squared raised to theMoreTogether that's why it becomes X to the twelfth. Another example would be Z squared raised to the seventh power power raised to a power you keep the same base two times seven is 14.
What are laws of exponents Class 7?
When numbers with the same base are multiplied, the power of the product is equal to the sum of the powers of the numbers. If 'a' is a non-zero integer, and 'm' and 'n' are whole numbers then, am × an = am+n.
How can I learn exponents easily?
0:001:29Easy Way to Learn Exponents & Radicals in Math - YouTubeYouTubeStart of suggested clipEnd of suggested clipItself three times so 2 to the third power is the same thing as 2 times 2 times 2 which is 8. So youMoreItself three times so 2 to the third power is the same thing as 2 times 2 times 2 which is 8. So you take the exponent. And whatever the base is you multiply it by itself that.
What are the steps to solve a law of exponents?
16:2625:57Exponent Rules of Algebra (Laws of Exponents, How to Multiply & Add ...YouTubeStart of suggested clipEnd of suggested clipAnd the rule is power raised to a power you multiply the powers. X to the 12th power that's theMoreAnd the rule is power raised to a power you multiply the powers. X to the 12th power that's the final answer.
Q. What are exponents?
A. Exponents are the powers that are used to simplify the multiplication and division of repeated numbers.
Q. What are the parts of laws of exponent?
A. Laws of exponents comprises of two parts i.e., base and exponent. For example, 6 x 6 x 6 x 6 x 6 = 6 5 . Here, 6 is the base and 5 is the exponent.
Q. What are rules of exponents?
A. The rules of exponents are as follows: 1. am×an = am+n 2. am/an = am-n 3. (am)n = amn 4. an/bn = (a/b)n 5. a0 = 1 6. a-m = 1/am
Q. What is the example of rule am×an = am+n ?
This law of exponent is applicable if the product has the same bases. For example, 25 × 21 = 25+1 = 26.
What are the laws of exponents?
The laws of exponents tell us how to solve equations or simplify expressions that contain exponents. Exponents are values that tell us how many times we must multiply a number by itself. For example, if we have , this means that we multiply 5 by itself 3 three times:
How many exponent laws are there?
There are seven laws of exponents that help us simplify exponential expressions. Each law shows how to solve different types of mathematical operations such as adding, subtracting, multiplying, and dividing exponents. In the following laws, the letters a and b represent nonzero real numbers and m and n represent interger numbers:
What Are the Laws of Exponents?
Exponents are the powers that are used to simplify the multiplication and division of repeated numbers. Laws of exponents comprise two parts i.e., base and exponent. Exponents are used to representing the repeated multiplication of numbers by themselves.
What are exponents used for?
A. Exponents are the powers that are used to simplify the multiplication and division of repeated numbers.
Why do we use exponents in math?
Laws of exponents are used to simplify the problems in the field of science and mathematics. Students learn the laws of exponents in their secondary school in order to solve mathematical problems quickly. You can check NCERT Solutions for Class 7 Maths Chapter 13 for a better understanding of the concepts. We have provided detailed information on laws of exponents in this article. Read on to find out about definition, Exponent Rules with Examples.
What is the law of a-m?
a-m = 1/am: This law is applicable if the power has negative exponent. For example, 2-2 = 1/22
What is an/bn law?
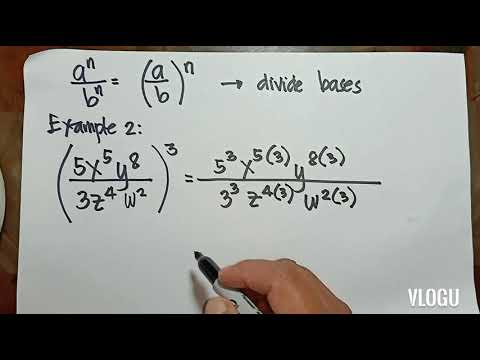
an/bn = (a/b)n : This law is applicable if the product is same with different powers. For example, 153/53 = (15/5)3= 33 = 27
What is the law of exponents?
Laws of Exponents. Exponents are also called Powers or Indices. The exponent of a number says how many times to use the number in a multiplication. So an Exponent saves us writing out lots of multiplies!
What are the first three laws of exponents?
The first three laws above ( x1 = x, x0 = 1 and x-1 = 1/x) are just part of the natural sequence of exponents. Have a look at this:
Where do we do the exponent?
We do the exponent at the top first, so we calculate it this way:
How to use the law of power of a quotient of exponents?
Using the law of power of a quotient of exponents, we copy the bases in the numerator and the denominator and multiply their exponents by the outer exponent:
How many factors are there with exponents?
Here we have three factors each with exponents. We simply have to copy each factor and multiply its exponent by the outer exponent:
When an exponential expression is raised to a power, do we copy the base and multiply the exponents?
When an exponential expression is raised to a power, we copy the base and multiply the exponents. Again, we assume that the base is nonzero and the exponents are whole numbers.
When we have a division of exponential expressions with the same base, do we copy the base and subtract the ex?
When we have a division of exponential expressions with the same base, we copy the base and subtract the exponent of the expression in the denominator from the exponent of the expression in the numerator.
Why are exponents important in algebra?
The laws of exponents are some of the most important laws in algebra because they can be part of most algebraic problems. Mastering the laws of basic exponents will make your study of algebra easier and more productive. In this article, we will learn about all the laws of exponents. We will use various examples to facilitate the mastery of these laws.
What is the first part of an exponential expression?
An exponential expression is made up of two parts. The first part is the base, which “carries” the exponent, which is the second part and is located in the upper right as can be seen in the illustration:
Where is the base with a negative exponent?
The base with a negative exponent is in the denominator. We can move it to the numerator and change the exponent from negative to positive:
What are the laws of exponents?
The laws of exponents, sometimes known as the rules of exponents, are the rules and properties of exponents you use when simplifying or evaluating expressions containing exponents. The laws of exponents include definitions for what fractional exponents, negative exponents, and even different exponential expressions mean.
How to simplify a negative exponent?
So, you can simplify a negative exponent by moving it from the numerator to the denominator of a fraction and then switching the exponent to a positive exponent. All the other numbers and coefficients stay the same. Keep in mind that the only number that moves is the number with the exponent.
Can you use subtraction to simplify exponents?
The same process holds true for the power of a quotient. If they have like bases , you can use subtraction to simplify the exponents.
Can you subtract exponents?
Note that you can't simply subtract or add exponents like that if you have exponents with different bases:
Can you multiply exponents together?
Finally, if you have an exponent on top of an exponent, you can multiply powers together to get a single exponent. This simply utilizes the other laws of exponents. For example, we simplify the uppermost exponent by multiplying the base (2³) by itself three times:
What is an exponent in math?
Exponents are defined as a number that tells how many times we have to multiply the base number. It is written above the right side of the base number. 1. 5 2 = “5 raised to the power of 2” or “5 squared.”. 2. 5 3 = “5 raised to the power of 3” or “10 cubed.”. The laws of exponents state the following rules to simplify the expressions.
When the numbers having the same base are multiplied, do you add the exponents?
Rule 1: When the numbers having the same base are multiplied, add the exponents.
What are Exponents?
Exponents are powers or indices. They are widely used in algebraic problems, and for this reason, its important to learn them so as to make studying of algebra easy. First of all, lets start by studying the parts of an exponential number.
When were exponents invented?
The history of exponents or powers is pretty old. In 9 th century , a Persian Mathematician Muhammad Musa introduced square of a number. Later in 15 th century, they introduced a cube of a number. The symbols to represent these indices are different, but the method of calculation was same.
How to change an exponent to a positive?
When an exponent is negative, we change it to positive by writing 1 in the numerator and the positive exponent in the denominator. The general forms of this law are: a -m = 1/a m a and (a/b) -n = (b/a) n
What is the difference between base and exponent?
The base is the first component of an exponential number. The base is basically a number or variable that is repeatedly multiplied by itself. Whereas the exponent is the second element which is positioned at the upper right corner of the base . The exponent specifies the number of times the base will be multiplied by itself.
What is the law of division of exponential numbers with the same base?
In the division of exponential numbers with same base, we need to do subtraction of exponents. The general forms of this law are: (a) m ÷ (a) n = a m – n and (a/b) m ÷ (a/b) n = (a/b) m – n
What is the general form of a zero exponent?
If the exponent is zero then you get 1 as the result. The general form is: a 0 = 1 and (a/b) 0 = 1
What is the general form of an exponential expression?
An exponential expression consists of two parts, namely the base, denoted as b and the exponent, denoted as n. The general form of an exponential expression is b n . For example, 3 x 3 x 3 x 3 can be written in exponential form as 3 4 where 3 is the base and 4 is the exponent.
What are exponents?
Exponents, also known as powers, are values that show how many times to multiply a base number by itself. For example, 43 is telling you to multiply four by itself three times. 43 = 4 × 4 × 4 = 64 The number being raised by a power is known as the base, while the superscript number above it is the exponent or power. Credit: To The Square Inch The equation above is said as “four to the power of three”. The power of two can also be said as “squared” and the power of three can be said as “cubed”. These terms are often used when finding the area or volume of various shapes. Writing a number in exponential form refers to simplifying it to a base with a power. For example, turning 5 × 5 × 5 into exponential form looks like 53. Exponents are a way to simplify equations to make them easier to read. This becomes especially important when you’re dealing with variables such as ‘𝒙’ and ‘𝑦’ — as 𝒙7 × 𝑦5 = ? is easier to read than (𝒙) (𝒙) (𝒙) (𝒙) (𝒙) (𝒙) (𝒙) (𝑦) (𝑦) (𝑦) (𝑦) (𝑦) = ?
How many exponent rules are there?
There are seven exponent rules, or laws of exponents, that your students need to learn. Each rule shows how to solve different types of math equations and how to add, subtract, multiply and divide exponents. Make sure you go over each exponent rule thoroughly in class, as each one plays an important role in solving exponent based equations.
How to solve an equation where a power is being raised by another power?
(𝒙3)3 = ? In equations like the one above, multiply the exponents together and keep the base the same . (𝒙3)3 = 𝒙9 Take a look at the expanded equation to see how this works:
What is the Quotient of Powers rule?
Quotient of powers rule — Subtract powers when dividing like bases. Power of powers rule — Multiply powers together when raising a power by another exponent. Power of a product rule — Distribute power to each base when raising several variables by a power.
How to make a number into a reciprocal?
To make a number into a reciprocal: Turn the number into a fraction (put it over one) Flip the numerator into the denominator and vice versa. When a negative number switches places in a fraction it becomes a positive number. The goal of equations with negative exponents is to make them positive.
How to turn a negative exponent into a positive?
When there is a number being raised by a negative exponent, flip it into a reciprocal to turn the exponent into a positive. Don’t use the negative exponent to turn the base into a negative. Credit: Thinglink We’ve talked about reciprocals before in our article, “How to divide fractions in 3 easy steps”. Essentially, reciprocals are what you multiply a number by to get the value of one. For example, to turn two into one, multiply it by ½. Now, look at this exponent example: 𝒙-2 = ? To make a number into a reciprocal:
How do exponents help you?
Rules of exponents in everyday life. Not only will understanding exponent properties help you to solve various algebraic problems , exponents are also used in a practical manner in everyday life when calculating square feet, square meters, and even cubic centimeters. Exponent rules also simplify calculating extremely large or extremely tiny ...
.PNG)
Summary of Laws of Exponents
Laws of Exponents – Examples with Answers
- The following examples apply the laws of exponents to simplify algebraic expressions. Try to solve the exercises yourself. However, each example has a detailed solution so that you can follow the reasoning used in each problem. Start now: Explore our additional Mathematics resources
Laws of Exponents – Practice Problems
- Test your knowledge of the laws of exponents with the following problems. Simplify or solve the expressions and select the correct answer. See the solved examples above if you need help.
See Also
- Interested in learning more about exponents? Take a look at these pages: 1. How to Solve Exponent Problems? 2. Examples of Negative Exponents 3. Examples of Fractional Exponents
Parts of Exponential Expressions
Summary of The Laws of Exponents
- In the following laws, a and b are nonzero real numbers, and m and nare integers: 1) Law of exponent zero: 2) Law of negative exponents: 3) Law of the product of exponents: 4) Law of the quotient of exponents: 5) Law of power of a power: 6) Law of power of a product: 7) Law of power of a quotient: Start now: Explore our additional Mathematics resources
Description of The Laws of Exponents with Examples
- 1) Law of exponent zero
Any number raised to the power of zero is equal to 1. - 2) Law of negative exponents
Any number that is raised to a negative exponent is not in its standard form. Therefore, we have to rewrite it. To achieve this, we move the base with the negative exponent to the opposite side of the fraction and then make the exponent positive.
See Also
- Interested in learning more about exponents? Take a look at these pages: 1. Fractional exponents – Rule and exercises 2. Negative Exponents in Fractions 3. Power of a Power Rule