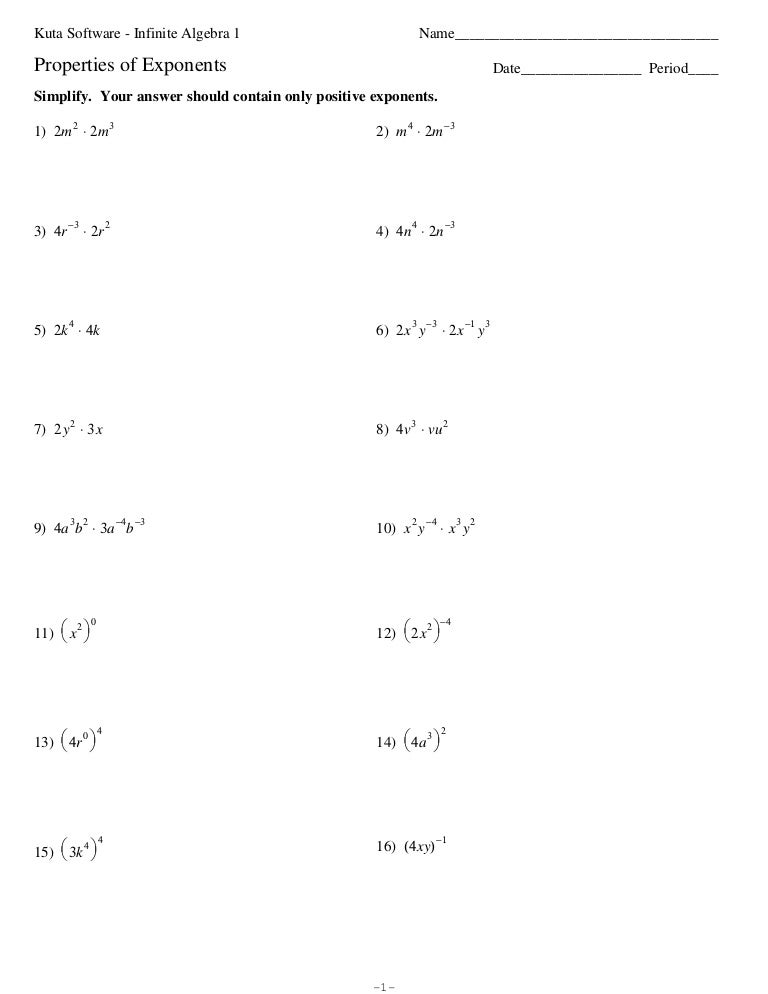
What are the 8 rules of exponents?
Rule #1: Multiplying Exponents With the Same Base. Rule #2: Dividing Exponents With the Same Base. Rule #3: Raising a Product to a Power. Rule #4: Raising a Quotient to a Power. Rule #5: Raising an Exponent to an Additional Power. This post is part of the series: Math Help for Exponents.
What are exponential properties?
The exponential function models exponential growth and has unique properties that make calculating calculus-type questions easier. The function has two important properties. First, the value of the function at 0 is 1 and second, the output of the function at any given point is equal to the rate of change at that point.
What is the multiplication property of exponents?
We multiplied the exponents. This leads to the Power Property for Exponents. Power Property for Exponents If a is a real number, and mandn are whole numbers, then (am)n = am · n To raise a power to a power, multiply the exponents. An example with numbers helps to verify this property. (32)3? = 32 · 3 (9)3? = 36 729 = 729 Example 6.22
What are the different rules of exponents?
What are the different rules of exponents? The rules of exponents are the 0 rule, the 1 rule, the power rule for exponents, the negative exponent rule, the product rule, and the quotient rule. They are the rules you use in order to simplify exponent problems and solve problems with exponents.

What are the 7 exponent laws?
Laws of ExponentsProduct of powers rule.Quotient of powers rule.Power of a power rule.Power of a product rule.Power of a quotient rule.Zero power rule.Negative exponent rule.
What are the 4 types of exponents?
Exponents can be observed in 4 different types namely, positive, negative, zero and rational/fractional. The number's value can be interpreted by using the exponent as the total number of times the base number has to be multiplied with the same base.
How do you use the properties of exponents step by step?
0:418:35Properties of exponents - YouTubeYouTubeStart of suggested clipEnd of suggested clipWe have an exponent of 2 and we can add that with our other exponent of 3. So if we simplify thisMoreWe have an exponent of 2 and we can add that with our other exponent of 3. So if we simplify this even further we have our base of X. And in our exponent. We have 2 plus 3 2 plus 3 is equal to 5.
What are the properties of exponents and how do they work?
When you raise a quotient to a power you raise both the numerator and the denominator to the power. When you raise a number to a zero power you'll always get 1. Negative exponents are the reciprocals of the positive exponents. The same properties of exponents apply for both positive and negative exponents.
What are the 5 rules of exponents?
Understanding the Five Exponent PropertiesProduct of Powers.Power to a Power.Quotient of Powers.Power of a Product.Power of a Quotient.
What are the 5 laws of exponents?
In Mathematics, there are different laws of exponents....The different Laws of exponents are:am×an = a. m+nam/an = a. m-n(am)n = a. mnan/bn = (a/b) na0 = 1.a-m = 1/a. m
What are the properties of exponents explain with example?
An exponent (also called power or degree) tells us how many times the base will be multiplied by itself. For example ', the exponent is 5 and the base is . This means that the variable will be multiplied by itself 5 times. You can also think of this as to the fifth power.
How many properties of exponents are there?
There are seven exponent rules, or laws of exponents, that your students need to learn. Each rule shows how to solve different types of math equations and how to add, subtract, multiply and divide exponents.
How do you simplify an exponent property?
3:4711:48Simplifying Exponents With Fractions, Variables, Negative ... - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo we need to multiply 3 times negative 5 is negative 15 x to the negative 15 is 1 over X to theMoreSo we need to multiply 3 times negative 5 is negative 15 x to the negative 15 is 1 over X to the positive 15. So this is the final answer for that problem now for the last one we need to subtract.
How do you find the properties of exponents?
0:1959:14Properties of Exponents - Algebra 2 - YouTubeYouTubeStart of suggested clipEnd of suggested clipPower you simply add the exponents. But now why is that let's use a simpler example x squared timesMorePower you simply add the exponents. But now why is that let's use a simpler example x squared times x cubed two plus three is five x squared means you're multiplying two x variables.
What are the rules of exponents?
The Power Rule for Exponents: (am)n = am*n. To raise a number with an exponent to a power, multiply the exponent times the power. Negative Exponent Rule: x–n = 1/xn. Invert the base to change a negative exponent into a positive.
What are the 6 laws of exponents?
Exponent rulesProduct of powers rule. When multiplying two bases of the same value, keep the bases the same and then add the exponents together to get the solution. ... Quotient of powers rule. ... Power of a power rule. ... Power of a product rule. ... Power of a quotient rule. ... Zero power rule. ... Negative exponent rule.
What is the exponent 4 called?
biquadrate; biquadratic; fourth power; quartic.
What is 4 by the power of 4?
256Answer: The value of 4 to the 4th power i.e., 44 is 256. Let us calculate the value of 4 to the 4th power i.e., 44.
How many exponents are there?
There are seven exponent rules, or laws of exponents, that your students need to learn. Each rule shows how to solve different types of math equations and how to add, subtract, multiply and divide exponents.
What is 4 the power of 2?
16Answer: 4 to the power of 2 can be expressed as 42 = 4 × 4 = 16. Let us proceed step by step.
What is an exponent in math?
Exponent is also known as the power of a number. It can be a whole number, fraction, negative number, or decimals. Let's learn more about exponents in this lesson. 1.
Why are exponents important?
Exponents are important because, without them, the products where a number is repeated by itself many times is very difficult to write. For example, it is very easy to write 5 7 instead of writing 5 × 5 × 5 × 5 × 5 × 5 × 5.
What does a negative exponent mean?
A negative exponent tells us how many times we have to multiply the reciprocal of the base. For example, if it is given that a-n, it can be expanded as 1/an. It means we have to multiply the reciprocal of a, i.e 1/a 'n' times. Negative exponents are used to write fractions with exponents.
How to solve decimal exponents?
It is slightly difficult to evaluate the correct answer of any decimal exponent so we find the approximate answer for such cases. Decimal exponents can be solved by first converting the decimal in fraction form.
What is a fractional exponent?
Fractional Exponents. If an exponent of a number is a fraction, it is known as a fractional exponent. Square roots, cube roots, n th root are all parts of fractional exponents. Number with power 1/2 is termed as the square root of the base. Similarly, numbers with power 1/3 is called cube root of the base. Some examples of fractional exponents are ...
When exponents are required to multiply multiplied, we first solve the numbers within parenthesis?
When exponents are required to multiply multiplied we first solve the numbers within parenthesis, the power outside the parenthesis is multiplied with every power inside the parenthesis. For example, (3x 2 y 3) 2 = 3 2 × x 2×2 × y 3×2 = 9x 4 y 6.
What is an exponent calculator?
An 'Exponent Calculator' is an online tool that finds the value for an exponential expression. Check now Cuemath's exponent calculator and find the value of an exponential expression for a given base and exponent value within a few seconds.
How to multiply exponentials with the same base?
Here's the formula: ( x ^ a ) ( x ^ b) = x ^ ( a + b ). When you multiply exponentials with the same base (notice that x and x are the same base), add their exponents (or powers).
What does quotient mean in math?
Remember, 'quotient' means 'division '.' The formula says ( x ^ a) / ( x ^ b) = x ^ ( a - b ). Basically, when you divide exponentials with the same base, you subtract the exponent (or powers).
How many exponent properties are there?
There are five main exponent properties, which are much like the order of operations in exponents, that give structure to simplifying expressions. Learn the formulas of the five exponent properties, including product of powers, power to a power, quotient of powers, power of a product, and power of a quotient. Updated: 10/24/2021
How to find the exponent of a power?
The formula says ( xy )^ a = ( x ^ a) and ( y ^ a ). When you have a product of a power, you give each base its own exponent. Think about it as distribution putting the exponent with each base.
What does it mean to enroll in a course?
Enrolling in a course lets you earn progress by passing quizzes and exams.
Do you have to be a Study.com member to unlock this lesson?
To unlock this lesson you must be a Study.com Member.
What does a negative exponent mean?from cuemath.com
A negative exponent tells us how many times we have to multiply the reciprocal of the base. For example, if it is given that a-n, it can be expanded as 1/an. It means we have to multiply the reciprocal of a, i.e 1/a 'n' times. Negative exponents are used to write fractions with exponents.
How to solve decimal exponents?from cuemath.com
It is slightly difficult to evaluate the correct answer of any decimal exponent so we find the approximate answer for such cases. Decimal exponents can be solved by first converting the decimal in fraction form.
How to do a decimal shift?from cuemath.com
Step 1: Put a decimal point after the first digit of the number from the right. If there is only one digit in a number excluding zeros, then we don't need to put decimal. Step 2: Multiply that number with a power of 10 such that the power will be equal to the number of times we shift the decimal point.
What is an exponent in math?from cuemath.com
Exponent is also known as the power of a number. It can be a whole number, fraction, negative number, or decimals. Let's learn more about exponents in this lesson. 1.
How do you use exponents in real life?from cuemath.com
In real life, we use the concept of exponents to write numbers in a simplified manner and in a short way. Repeated multiplication can be easily written with the help of exponents. Also, we use exponents to write larger numbers, for example, the distance of the moon from earth, the number of bacteria present on a surface, etc.
When exponents are required to multiply multiplied, we first solve the numbers within parenthesis?from cuemath.com
When exponents are required to multiply multiplied we first solve the numbers within parenthesis, the power outside the parenthesis is multiplied with every power inside the parenthesis. For example, (3x 2 y 3) 2 = 3 2 × x 2×2 × y 3×2 = 9x 4 y 6.
How to find the volume of a wardrobe?from cuemath.com
The volume of the wardrobe is, volume= lwh. So, by substituting the values, the volume of the wardrobe is x 5 × y 3 × x 8 = x 13 y 3 (using exponents formula = a m × a n = a (m+n) ). Therefore, the volume of the wardrobe is x 13 y 3 cubic units.
What is an Exponent in Math?
There are times in math when a number needs to be multiplied over and over. When that happens, one can end up with a long equation such as 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2 x 2, which is a hassle to write out and easy to lose one's place when trying to solve it. Mathematicians came up with the exponent to eliminate this problem.
Properties of Exponents
When it comes to working with exponents, there are several rules and properties of exponents that are used and are important to know. The following is a list of those properties and what each one means.
Additional Practice with Exponents
In the following practice problems, students will practice rewriting expressions involving exponents by using the properties of exponents. The skills developed while practicing using exponent properties will be necessary in future math courses. The problems will increase in difficulty and may involve more than one property of exponents.
Practice Problems
Use properties of exponents to simplify the expressions until they involve only one positive exponent. Then evaluate the expression using the definition of exponents.
What does an exponent represent?from ck12.org
When an exponent is a positive whole number, an exponent is a shorthand way to represent large quantities of multiplication. Exponents can represent any value that is multiplied by itself repeatedly (for example, an expression such as 5 · 5 · 5 · 5 ). Variables can have exponents just like real numbers. When the exponent is zero, negative, or fractional, there are certain rules we have to remember.
How to multiply powers with the same base?from ck12.org
To multiply powers with the same base, add the exponents together.
How to take a power of a product?from ck12.org
To take a power of a product, multiply the exponents together.
Properties of Exponents
The terms power and exponent are often used interchangeably in reference to a mathematical expression that means that a number will be multiplied by itself {eq}n {/eq} times.
Product of Powers Property
The product of powers property applies the multiplication properties of exponents as follows:
Power of a Power Property
The power of a power property applies the power properties of exponents as follows:
Quotient of Powers
The quotient of powers property applies the division properties of exponents as follows:
Power of a Product
The power of a product property applies the properties of exponents as follows:
Power of a Quotient
The power of a product property applies exponent properties similar to the ones applied to the power of a product.
What is the Power in Mathematics?
The term “power” in mathematics refers to the process of increasing a base number to its exponent. “Base number” and “exponent” are the two fundamental elements of powers. The exponent represents the number of times the base number is multiplied, while the base number is defined as a number that’s being multiplied by itself.
What is an Exponent?
Definition: The exponent of a number shows how many times we multiply the number itself. For example, \ (2^3\) indicates that we multiply 2 by 3 times. Its extended form is written as 2 × 2 × 2. Exponent is also known as numerical power. It could be a whole number, a fraction, a negative number, or decimals.
What are Negative Exponents?
Definition: A negative exponent is used when 1 is divided by repeated multiplication of a factor. Say, \ (\frac {1} {a}\) is given by \ (a^ {-1}\), where -1 is the exponent. If a number is raised to a negative exponent then it represents the reciprocal of it.
Exponent Symbol
For representing the exponent of the base number, we use the symbol ^. This symbol (^) is called a carrot. For example, 3 raised in 2 can be written 3 ^ 2. Thus, 3 ^ 2 = 3 × 3, which is 9. The table below shows the representations of a few number sentences using objects.
Exponents Properties
Exponents’ properties or rules of exponents are used to solve problems involving exponents ( exponents worksheets, 8th grade exponents worksheets ). These structures are also considered to be the rules of the main exponents which must be followed when resolving the exponents. The characteristics of the exponents are listed in the table.
What is the exponent of a number?
Recall an exponent describes the number of times a value is multiplied with itself. For example, six x ’s multiplied together is the same as “ x to the sixth power.”
How to multiply exponents?
This demonstrates two properties of exponents: 1 To multiply terms with exponents together make sure the bases are all the same, in this case they were all x, then add the exponents (s ee diagram 3 ). 2 When raising a term with an exponent to another exponent, multiply the powers together. For example:
What does it mean when a cubed is raised to the second power?
X-cubed raised to the second power means I am multiplying two x-cubes together.
What is the power of 4?
The power is an expression that shows repeated multiplication of the same number or factor. For example, in the expression 6 4, 4 is the exponent and 6 4 is called the 6 power of 4. That means, 6 is multiplied by itself 4 times. Learn more about exponents and powers here.
What is the zero rule?
Zero rule: Any number with an exponent zero is equal to 1. Example: 8 0 = 1, a 0 = 1. One Rule: Any number or variable that has the exponent of 1 is equal to the number or variable itself. Example: a 1 = a, 7 1 = 1.
What is the exponent of a number?
Exponent is defined as the method of expressing large numbers in terms of powers. That means, exponent refers to how many times a number multiplied by itself . For example 6 is multiplied by itself 4 times, i.e. 6 × 6 × 6 × 6. This can be written as 6 4. Here, 4 is the exponent and 6 is the base. This can be read as 6 is raised to the power 4.
What is the law of base?
Multiplication Law: Bases – multiplying the like ones; add the exponents and keep the base the same.
What are the laws of exponents?
Different laws of exponents are described based on the powers they bear. Multiplication Law : Bases – multiplying the like ones; add the exponents and keep the base the same. When bases are raised with power to another, multiply the exponents and keep the base the same.
What is the rule for a number with an exponent of zero?
Zero rule: Any number with an exponent zero is equal to 1.
What is the value of 2 with an exponent of 4?
2 with an exponent of 4 can be written as 2^4 and its value is 2 × 2 × 2 × 2 = 16.