Laws of Logarithms
Logarithm
In mathematics, the logarithm is the inverse operation to exponentiation. That means the logarithm of a number is the exponent to which another fixed value, the base, must be raised to produce that number. In simple cases the logarithm counts repeated multiplication. For ex…
Logarithm
In mathematics, the logarithm is the inverse operation to exponentiation. That means the logarithm of a number is the exponent to which another fixed value, the base, must be raised to produce that number. In simple cases the logarithm counts repeated multiplication. For ex…
What are the basics of logarithms?
- Common logs have a base of 10. (for example, log 10 x). ...
- Natural logs: These are logs with a base of e. e is a mathematical constant that is equal to the limit of (1 + 1/n) n as n approaches infinity, ...
- Other Logs: Other logs have the base other than that of the common log and the E mathematical base constant. ...
What is the power rule of logarithms?
The log rules could be expressed in less formal terms as:
- Multiplication can be turned outside the log into addition and versa can be turned.
- Division can be turned outside the log into a subtraction, and vice versa.
- An exponent can be moved as a multiplier outwards on all within a log, and vice versa.
How to calculate logarithms?
Calculating Logarithms By Hand W. Blaine Dowler June 14, 2010 Abstract This details methods by which we can calculate logarithms by hand. 1 De nition and Basic Properties A logarithm can be de ned as follows: if bx = y, then x = log b y. In other words, the logarithm of y to base b is the exponent we must raise b to in order to get y as the result.
What are the uses of logarithms?
- Electronic calculators have logarithms to make our calculations much easier.
- Logarithms are used in surveys and celestial navigation.
- Logarithms can be used to calculate the level of noise in decibels.
- Ratio active decay, acidity [PH] of a substance and Richter scale are all measured in logarithmic form.

What are the 7 laws of logarithms?
Descriptions of Logarithm RulesRule 1: Product Rule. The logarithm of the product is the sum of the logarithms of the factors.Rule 2: Quotient Rule. ... Rule 3: Power Rule. ... Rule 4: Zero Rule. ... Rule 5: Identity Rule. ... Rule 6: Inverse Property of Logarithm. ... Rule 7: Inverse Property of Exponent. ... Rule 8: Change of Base Formula.
What are the 4 laws of logarithmic functions?
Logarithm Rules or Log RulesThere are four following math logarithm formulas: ● Product Rule Law:loga (MN) = loga M + loga N. ● Quotient Rule Law:loga (M/N) = loga M - loga N. ● Power Rule Law:IogaMn = n Ioga M. ● Change of base Rule Law:
What are the laws of logarithms and examples?
Laws of logarithms These laws can be applied on any base, but during a calculation, the same base is used. Example: log 2 5 + log 2 4 = log 2 (5 × 4) = log 2 20. log 10 6 + log 10 3 = log 10 (6 x 3) = log 10 18.
What are the rules of logarithms?
The rules apply for any logarithm logbx, except that you have to replace any occurence of e with the new base b. The natural log was defined by equations (1) and (2)....Basic rules for logarithms.Rule or special caseFormulaQuotientln(x/y)=ln(x)−ln(y)Log of powerln(xy)=yln(x)Log of eln(e)=1Log of oneln(1)=02 more rows
What is the first law of logarithm?
First Law. log A + log B = log AB. This law tells us how to add two logarithms together. Adding log A and log B results in the logarithm of the product of A and B, that is log AB.
What are the 3 fundamental properties of logarithms?
Logarithm Base PropertiesProduct rule: am. an=a. m+nQuotient rule: am/an = a. m-nPower of a Power: (am)n = a. mn
What are the types of logarithms?
Two kinds of logarithms are often used in chemistry: common (or Briggian) logarithms and natural (or Napierian) logarithms. The power to which a base of 10 must be raised to obtain a number is called the common logarithm (log) of the number. The power to which the base e (e = 2.718281828.......)
How do you solve law of logarithms?
1:4110:04Laws of Logarithms - YouTubeYouTubeStart of suggested clipEnd of suggested clipThe similarity between this and the indc law that I showed you is here where x in these two numbersMoreThe similarity between this and the indc law that I showed you is here where x in these two numbers together x. And y when. We are timesing.
How do you prove laws of logarithms?
9:3416:38Understand Logarithm Rules & Laws of Logs - YouTubeYouTubeStart of suggested clipEnd of suggested clipYou see you take the logarithm of something with an exponent you can just take that exponent rightMoreYou see you take the logarithm of something with an exponent you can just take that exponent right out front and then multiply by the log of that of that base there.
What is a logarithm in simple terms?
A logarithm is the power to which a number must be raised in order to get some other number (see Section 3 of this Math Review for more about exponents). For example, the base ten logarithm of 100 is 2, because ten raised to the power of two is 100: log 100 = 2. because. 102 = 100.
What is logarithm used for?
Logarithms are the inverse of exponents. A logarithm (or log) is the mathematical expression used to answer the question: How many times must one “base” number be multiplied by itself to get some other particular number? For instance, how many times must a base of 10 be multiplied by itself to get 1,000?
Why do we use logarithms?
It lets you work backwards through a calculation. It lets you undo exponential effects. Beyond just being an inverse operation, logarithms have a few specific properties that are quite useful in their own right: Logarithms are a convenient way to express large numbers.
What is the function of logarithm?
Definition of logarithmic function : a function (such as y = loga x or y = ln x) that is the inverse of an exponential function (such as y = ax or y = ex) so that the independent variable appears in a logarithm.
How do you remember logarithmic rules?
1:014:30Rules of Logarithms | Don't Memorise - YouTubeYouTubeStart of suggested clipEnd of suggested clipIt equals n times log of a to the base B. The power of the argument is written here. So these areMoreIt equals n times log of a to the base B. The power of the argument is written here. So these are the three most important rules in logarithms.
What kind of function is a logarithmic function?
Logarithmic functions are the inverses of exponential functions. The inverse of the exponential function y = ax is x = ay. The logarithmic function y = logax is defined to be equivalent to the exponential equation x = ay.
How do you answer logarithmic functions?
0:0125:26Solving Logarithmic Equations - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo therefore x is equal to four you can also do this log of 16 divided by log of two if you typeMoreSo therefore x is equal to four you can also do this log of 16 divided by log of two if you type that in that's equal to four which is x using the change of base formula.
How many laws are there in logarithms?
The laws of logarithms allow us to rewrite logarithmic expressions to form more convenient expressions. There are seven main laws of logarithms. These seven laws are useful for expanding logarithms, condensing logarithms, and solving logarithmic equations. The laws apply to logarithms of any base, but the same base must be used to apply each law.
What is the power of a logarithm?
Remember that a logarithm is the power to which a number must be raised to obtain another number. For example, the base 10 logarithm of 100 is 2, since 10 raised to the power of 2 equals 100: The base is the number that is being raised to a power. We can use logarithms with any base.
What is the logarithm of the quotient of two quantities?
This law tells us that the logarithm of the quotient of two quantities is equal to the logarithm of the numerator minus the logarithm of the denominator:
What is the logarithm of an argument?
The logarithm of the argument (inside the parentheses), where the argument is equal to the base is equal to 1. Since the argument is equal to the base, b must be greater than zero, but not can be equal to 1.
Do laws apply to logarithms?
The laws apply to logarithms of any base , but the same base must be used to apply each law. Here, we will learn about the main laws of logarithms. In addition, we will lookt at several examples with answers to understand how to apply these laws to solve algebraic problems. ALGEBRA.
Can we simplify logarithms?
We have a product of factors inside the parentheses, so we can apply the product law to write each factor separately. We can simplify individual logarithms when possible. In this case we use the law of identity to simplify:
What are the rules of logarithms?
They are always applied under certain rules and regulations. Following rules needed to be remembered while playing with logarithms: Given that a n = b ⇔ log a b = n, the logarithm of the number b is only defined for positive real numbers.
Why is logarithm important?
It is important to note that the laws and rules of logarithms apply to logarithms of any base.
What is a logarithm with a base of 10?
Logarithms to the base of 10 are referred to as common logarithms. When a logarithm is written without a subscript base, we assume the base to be 10.
What is the purpose of logarithms in analyzing exponential processes?
A logarithm is used to calculate the payment period of a loan .
Why are logarithms taught in schools?
Although logarithms are taught in schools to simplify computation involving large numbers, they still have a significant role in our daily lives.
What is the correct answer for a logarithm argument?
Since argument of a logarithm cannot be negative, then the correct answer is x = 6.
What is the logarithm of a positive number to the same base?
The logarithm of any positive number to the same base is equal to 1.
What are the rules of logarithm?
Descriptions of Logarithm Rules. Rule 1: Product Rule. The logarithm of the product is the sum of the logarithms of the factors. Rule 2: Quotient Rule. The logarithm of the ratio of two quantities is the logarithm of the numerator minus the logarithm of the denominator. Rule 3: Power Rule. The logarithm of an exponential number is ...
What is the logarithm of an exponential number?
The logarithm of an exponential number is the exponent times the logarithm of the base.
What is the log of exponent rule?
Rule 6: Log of Exponent Rule (Logarithm of a Base to a Power Rule) The logarithm of an exponential number where its base is the same as the base of the log is equal to the exponent.
Why do we need to apply the quotient rule first?
You might notice that we need to apply the Quotient Rule first because the expression is in fractional form.
Can you use logarithm rules in reverse?
However, it’s okay to apply the Logarithm Rules in reverse! Notice that the log expression can be expressed as one or a single logarithmic number through the use of the Quotient Rule backward. Sounds like a plan.
When were logarithms invented?
Invented in the 17th century to speed up calculations, logarithms vastly reduced the time required for multiplying numbers with many digits.
What is the logarithm of a base?
logarithm, the exponent or power to which a base must be raised to yield a given number. Expressed mathematically, x is the logarithm of n to the base b if bx = n, in which case one writes x = log b n. For example, 2 3 = 8; therefore, 3 is the logarithm of 8 to base 2, or 3 = log 2 8. In the same fashion, since 10 2 = 100, then 2 = log 10 100. Logarithms of the latter sort (that is, logarithms with base 10) are called common, or Briggsian, logarithms and are written simply log n.
How did logarithms influence trigonometry?
The procedures of trigonometry were recast to produce formulas in which the operations that depend on logarithms are done all at once. The recourse to the tables then consisted of only two steps, obtaining logarithms and, after performing computations with the logarithms, obtaining antilogarithms.
Who published the logarithms of 1 to 20,000?
This change produced the Briggsian, or common, logarithm. Napier died in 1617 and Briggs continued alone, publishing in 1624 a table of logarithms calculated to 14 decimal places for numbers from 1 to 20,000 and from 90,000 to 100,000.
Who wrote the first logarithmic table?
Tables of logarithms were first published in 1614 by the Scottish laird John Napier in his treatise Description of the Marvelous Canon of Logarithm s. This work was followed (posthumously) five years later by another in which Napier set forth the principles used in the construction of his…
Can a logarithm be converted to a positive base?
Logarithms can also be converted between any positive bases (except that 1 cannot be used as the base since all of its powers are equal to 1), as shown in the table of logarithmic laws. Only logarithms for numbers between 0 and 10 were typically included in logarithm tables.
Is a logarithmic sine a number?
The logarithme, therefore, of any sine is a number very neerely expressing the line which increased equally in the meene time whiles the line of the whole sine decreased proportionally into that sine, both motions being equal timed and the beginning equally shift.
What is the logarithm of x raised to the power of y?
The logarithm of x raised to the power of y is y times the logarithm of x.
What is the base b logarithm?
The base b logarithm of a number is the exponent that we need to raise the base in order to get the number.
How to find inverse logarithm?
The inverse logarithm (or anti logarithm) is calculated by raising the base b to the logarithm y:
When is the base b real logarithm of x undefined?
The base b real logarithm of x when x<=0 is undefined when x is negative or equal to zero:
What are the properties of logarithms?
We have learned many properties in basic maths such as commutative, associative and distributive , which are applicable for algebra. In the case of logarithmic functions, there are basically five properties. Table of Contents: Logarithm Base Properties.
What is logarithm used for?
Logarithms are widely used for measuring the time taken by something to decay or grow exponentially, such as bacteria growth, radioactive decay, etc.
What is the logarithmic number of 10000?
The logarithmic number is associated with exponent and power, such that if x n = m, then it is equal to log x m=n. Hence, it is necessary that we should also learn exponent law . For example, the logarithm of 10000 to base 10 is 4, because 4 is the power to which ten must be raised to produce 10000: 10 4 = 10000, so log 10 10000 = 4.
How many properties are there in logarithmic functions?
In the case of logarithmic functions, there are basically five properties. The logarithmic number is associated with exponent and power, such that if x n = m, then it is equal to log x m=n. Hence, it is necessary that we should also learn exponent law .
Is logarithms outside of mathematics?
The application of logarithms is enormous inside as well as outside the mathematics subject. Let us discuss brief description of common applications of logarithms in our real life :
Do we need to revise the law of exponents before proceeding ahead?
Before we proceed ahead for logarithm properties, we need to revise the law of exponents, so that we can compare the properties.
Is log property similar to exponent?
As you can see these log properties are very much similar to laws of exponents. Let us compare here both the properties using a table:

Laws of Logarithm Definition
- Definition: The logarithmic expressions can be written in various ways, and there are a few specific laws called the laws of logarithms. That is \({b^v} = a\), which is expressed as \({\log _b}a = y\). In other words, this can be stated as the logarithm of a positive real number \(a\) to the ba…
Types of Logarithms
- You can see the two types of logarithm in most of the cases, and they are: 1. Common Logarithm 2. Natural Logarithm 1. Common Logarithm The common logarithm is also known as the base ten logarithms. It is written as \(p\log \log p\). So, when the logarithm is taken with respect to base \(10\), then we call it is the common logarithm. The base is sometimes not written in a commo…
Some Other Properties
- Few more properties of the logarithm functions are given below: 1. \({\log _b}b = 1\) 2. \({\log _b}1 = 0\) 3. \({\log _b}0 = \) 4. The logarithm of a negative number is not defined
How to Use The Logarithm Properties?
- We use the Logarithm properties to make the calculations simpler: Logarithms help us to convert the exponential form \({2^5} = 32\) into logarithmic form \({\log _2}32 = 5\) The logarithm property is helpful to write the product as the sum. \(\log \log 14 = \log \log (7 \times 2) = \log 7 + \log 2\) The logarithm property is utilised to write the division as the difference. \(\log 0.3 = \log …
Formulas of Logarithm
- There are a few of the formulas of logarithm given below: 1. \({\log _b}({\rm{mn}}) = {\log _b}(\;{\rm{m}}) + {\log _b}(n)\) 2. \({\log _b}\left( {\frac{m}{n}} \right) = {\log _b}(m) – {\log _b}(n)\) 3. \({\log _b}\left( {{x^y}} \right) = y{\log _b}(x)\) 4. \({{\mathop{\rm mlog}\nolimits} _b}(x) + {{\mathop{\rm nlog}\nolimits} _b}(y) = {\log _b}\left( {{x^m}{y^n}} \right)\) 5. \({\log _b}(m + n) = …
Solved Examples – Laws of Logarithm
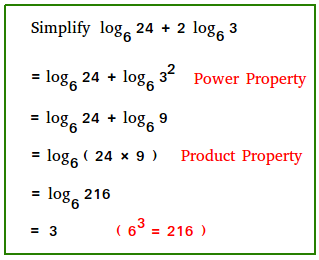
- Q.1. Evaluate the given expression: \({\log _2}8 + {\log _2}4\). Ans: Here, we will apply the rule, \({\log _2}8 + {\log _2}4 = {\log _2}(8 \times 4)\) \( = {\log _2}32\) Now, rewrite \(32\) in the exponential form to get the value of its exponent. \(32 = {2^5}\) So, we have, \({\log _2}32 = {\log _2}{2^5} = 5{\log _2}2 = 5 \times 1 = 5\) Hence, the required answer is \(5\). Q.2. Evaluate [{\log …
Summary
- In the given article, we have discussed what the logarithm of a number is, provided examples, and gave the definition for logarithm. We have concerned the rules and the properties of the logarithms with an example for each rule and property. Finally, we glanced at the types of common and natural logarithms—then provided a few formulas used in logarithms. We have als…
FAQs
- Q.1. How many types of logarithms are there? Ans: There are two types of logarithms, and they are given below: Common Logarithm The common logarithm is also known as the base ten logarithms. It is written as \(p\log \log p\) . So, when the logarithm is taken with respect to base \(10\), then we call it is the common logarithm. Example: \({10^2} = 100 \Rightarrow 100 = 2\) N…
Descriptions of The Laws of Logarithms
Laws of Logarithms – Examples with Answers
- The following examples apply the laws of logarithms described above. Each example has its respective solution to look at the reasoning used and the laws of logarithms applied.
Laws of Logarithms – Practice Problems
- Use the laws of logarithms to solve the following problems. Choose an answer and check it to see that you selected the correct one. If you need help, you can look at the solved examples above or the list of the laws of logarithms.
See Also
- Interested in learning more about logarithms? Take a look at these pages: 1. Exercises on Logarithms 2. Applications of Logarithms