6 Vital Measures of Central Tendency and Dispersion
- Mean. Mean provides a rough estimate of the most common value in a sample. ...
- Median. Median is one of the easiest measures of central tendency and dispersion to compute. ...
- Mode. Mode is the most occurring value of the data series. ...
- Range. ...
- Upper & Lower Quartiles. ...
- Variance And Standard Deviation. ...
- Conclusion. ...
What are the best measures of central tendency?
The Best Measure of Central Tendency
- Mean is the preferred measure of central tendency when data is normally distributed.
- Median is the best measure of central tendency when data is skewed.
- While dealing with nominal variables, the model is the best measure of central tendency.
What are the different measures of central tendency?
What are the Measures of Central Tendency?
- Mean. Mean is an approach that is generally used in Statistics. ...
- Median. The data of the middlemost observation that is achieved after modifying the data in ascending order is termed the median of the data.
- Mode. ...
- Relationship Between Mean, Median and Mode. ...
- Range. ...
What is the best measure of central tendency and why?
- If you have a symmetrical distribution of continuous data, all the three measures of central tendency hold good. ...
- If you have skewed distribution, the best measure of finding the central tendency is the median.
- If you have the original data, then both the median and mode are the best choice of measuring the central tendency.
How to calculate central tendency?
- x̄: sample mean
- ⅀ x: sum of all values in the sample data set
- n: number of values in the sample data set

What are the major measures of central tendency?
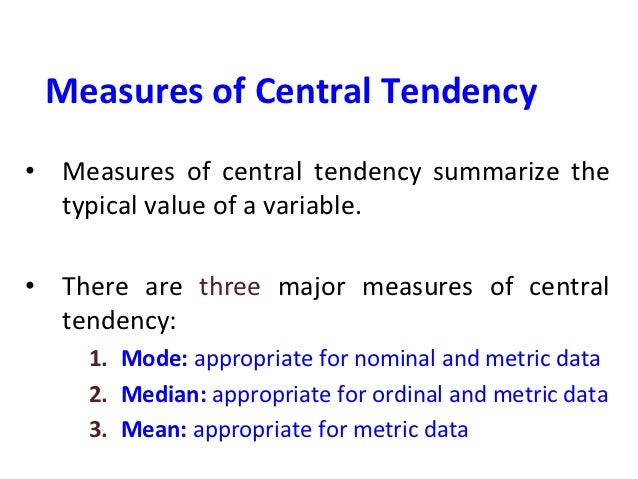
There are three main measures of central tendency: the mode, the median and the mean.
What is the best measure of central tendency and dispersion?
The mean is usually the best measure of central tendency to use when your data distribution is continuous and symmetrical, such as when your data is normally distributed.
What are the 3 main measures of dispersion?
Range, interquartile range, and standard deviation are the three commonly used measures of dispersion.
What is central tendency and dispersion?
Central tendency is described by median, mode, and the means (there are different means- geometric and arithmetic). Dispersion is the degree to which data is distributed around this central tendency, and is represented by range, deviation, variance, standard deviation and standard error.
Why is measures of central tendency and measures of dispersion important in research?
While measures of central tendency are used to estimate "normal" values of a dataset, measures of dispersion are important for describing the spread of the data, or its variation around a central value. Two distinct samples may have the same mean or median, but completely different levels of variability, or vice versa.
What are the measures of dispersion discuss each?
Measures of dispersion describe the spread of the data. They include the range, interquartile range, standard deviation and variance. The range is given as the smallest and largest observations. This is the simplest measure of variability.
What are the five measures of dispersion?
There are five most commonly used measures of dispersion. These are range, variance, standard deviation, mean deviation, and quartile deviation.
What is central tendency in statistics?
Central tendency is defined as “the statistical measure that identifies a single value as representative of an entire distribution.”[2] It aims to provide an accurate description of the entire data. It is the single value that is most typical/representative of the collected data.
What is dispersion and measures of dispersion?
Dispersion is the state of getting dispersed or spread. Statistical dispersion means the extent to which numerical data is likely to vary about an average value. In other words, dispersion helps to understand the distribution of the data.
What is measure of dispersion in statistics?
A measure of dispersion indicates the scattering of data. It explains the disparity of data from one another, delivering a precise view of their distribution. The measure of dispersion displays and gives us an idea about the variation and the central value of an individual item.
Why standard deviation is the best measure of dispersion?
Standard deviation is the best measures of dispersion, because it posseses most of the characterstics of an ideal measure of dispersion. 1. Most of the statistical theory is based on Standard Deviation. It helps to make comparison between variability of two or more sets of data.
What is the most stable measures of dispersion?
The standard deviation is the most widely used measure of dispersion. It takes all the items into consideration. It is more stable compared to other measures. However, it will be inflated by extreme items as is the mean.
Which measure of dispersion is the best and how class 11?
Standard Deviation is the best measure of dispersion as it satisfies the most essentials of the good measure of dispersion. The following points make Standard Deviation the best measure of dispersion: 1. Most of the statistical theory is based on Standard Deviation.
Which one is more appropriate to measure dispersion standard deviation or interquartile?
Standard Deviation (s) It is the better measure of dispersion compared to range and IQR because unlike range and IQR, the Standard deviation utilizes all the values in the data set in its calculation.
What is standard deviation?
The standard deviation is an extremely useful number, especially when accompanied by a measure of central tendency. If playback doesn't begin shortly, try restarting your device. Videos you watch may be added to the TV's watch history and influence TV recommendations.
What is the difference between sample statistic and population statistic?
The sample statistic is always derived from fewer than the total number of subjects in a population. The population statistic is always derived from measuring every single member of a subject population.
When did the term "statistic" come into use?
Statistics. Surprisingly, the term statistic first came into use as late as 1817. This is from the Oxford English Dictionary: statistic. A fact or a piece of data obtained from a study of a large quantity of numerical data.
Who is the last male rhinocerous?
On March 19th, 2018, the world’s last male northern white rhinocerous, Sudan, died at the age of 45. Two female rhinos remain: his daughter Najin and his granddaughter Fatu. If we want to find the average population weight of northern white rhinos, we would weigh Najin and Fatu, add their weights together, and divide by the number of rhinos we weighed: 2.
Is there a baby cockroach in my ear?
There might be a baby cockroach in your ear right now. Basically, there are a lot of f’ng cockroaches in the world. Okay then. Without major advances in technology, we will never know the population mean of the length of, say, American cockroaches.
How to find median?
You can find the median by arranging all the individual values in a dataset from smallest to largest and finding the middle value. If there are an odd number of values, the median is the middle value.
Why is central tendency important?
Takeaway: A measure of central tendency is useful because it provides us with a single value that describes the “center” of a dataset. This helps us understand a dataset much more quickly compared to simply looking at all of the individual values in the dataset.
What is central tendency?
A measure of central tendency is a single value that represents the center point of a dataset. This value can also be referred to as “the central location” of a dataset. In statistics, there are three common measures of central tendency:
What are the measures of central tendency?
Sometimes the data tends to cluster around the central value. The most common measures of central tendency are mean, median, and mode. In this section, we will learn how to calculate the three measures of central tendency. We will also see which kind of data is appropriate for which type of measure.
What is the purpose of measuring position?
Measures of Position. Measures of position are various techniques that are used to divide the data into equal groups. These values tell us the position of the value in the data set relative to other values. The data must be arranged in ascending order to compute the measures of position.
How do quartiles work?
Quartiles divide the data set into four equal parts. Different quartiles reflect the three values that divide the data into four equal parts. We denote the first quartile as and it represents 25% of the values less than it and 75% of the values greater than it. The second quartile is also known as median as it reflects 50% of the values above it and 50% below it. It is denoted as . The third quartile has 25% values greater than it and 75% values smaller than it. It is represented as .
How many segments does a decile have?
A decile splits the data set into ten equal segments. It is terminology in statistics that divides the data into ten intervals. It actually splits the data into ten equal segments on the number line.
What is the most repeated value in a data set?
The mode is the most repeated value in the data set. A data set can have one, two or multiple modes. A data set that has two modes is known as bimodal and the data set in which there are multiple modes is known as multi-modal data set. If all the elements in the data set are repeated with the same frequency, then the data has no mode.
How to find the arithmetic mean?
To calculate the arithmetic mean, enumerate all the values in the data set and divide the resulting value by the number of observations.
How to describe a joint distribution?
A partial description of the joint distribution of the data is provided here. Three aspects of the data are of importance, the first two of which you should already be familiar with from univariate statistics. These are: 1 Central Tendency: What is a typical value for each variable? 2 Dispersion: How far apart are the individual observations from a central value for a given variable? 3 Association: This might (or might not!) be a new measure for you. When more than one variable are studied together, how does each variable relate to the remaining variables? How are the variables simultaneously related to one another? Are they positively or negatively related?
What is sample statistic?
A sample statistic is a numerical characteristic of a sample. A sample statistic estimates the unknown value of a population parameter. Information collected from sample statistic are sometimes refered to as Descriptive Statistics.
What is population in statistics?
A population is the collection of all people, plants, animals, or objects of interest about which we wish to make statistical inferences (generalizations). The population may also be viewed as the collection of all possible random draws from a stochastic model; for example, independent draws from a normal distribution with a given population mean and population variance.
What is population parameter?
A population parameter is a numerical characteristic of a population. In nearly all statistical problems we do not know the value of a parameter because we do not measure the entire population. We use sample data to make an inference about the value of a parameter.
What are the most commonly used indices in describing the central tendency of a data set?
Mean, mode, and median are the most commonly used indices in describing the central tendency of a data set. If a data set is symmetric, then both the median and the mean of the data set coincide with each other. Given a data set, the mean is calculated by taking the sum of all the data values and then dividing it by the number of data.
What are the properties of indices?
In descriptive and inferential statistics, several indices are used to describe a data set corresponding to its central tendency, dispersion, and skewness: the three most important properties that determine the relative shape of the distribution of a data set.
What is the median in statistics?
The median is the data point found at the exact middle of the data set.
Is median a measure of central tendency?
Median is not affected by the presence of the outliers. Hence, median will serve as a better measure of central tendency in the presence of outliers. The mode is the most frequently occurring value in the set of data.

Mean
- Mean provides a rough estimate of the most common value in a sample. It is commonly known as the average of a data set. Calculating the mean of a data set is a simple process. A person can find the average by summing all the data values and dividing them by the total number of items. …
Mode
- Mode is the most occurring value of the data series. It gives the exact value present in the data set. There might be a different number of modes in a data set. One significant thing to understand in measures of central tendency and dispersion notes is that the mode of a sample can be zero when all the observations in the data occur only once or the same number of times. Formula A p…
Range
- Range is defined as the difference between the highest and the lowest value of a sample. It is the most time-efficient measure of central tendency & dispersion. Formula Range = Highest value – lowest value
Upper & Lower Quartiles
- A quartile is defined as the median or the middlemost value of two halves of a data set. The lower quartile measures the median of the lower half of the series while the other calculates the median of the upper half. Formula The formula is the same as the median. The only difference is that a person will need to identify the upper and lower half of the data, and then calculate the median o…
Variance and Standard Deviation
- These central tendency and dispersion measures calculate the variation and change of the data set and observations from its mean. Variance is the square of standard deviation. Formula Standard Deviation =√ (xi – m)^2/n Where xi means the ‘ith item value, ‘n’ refers to the number of observations, and ‘m’ means the average or mean.
Conclusion
- These statistics measures hold the most integral part of analyzing and interpreting a data set. From mean to standard deviation, every measure can give valuable insights to the audience. The most crucial benefit of using these measures is that a person can find the most common value, centre value, spread of data, etc., using simple formulae. It does not require any rocket science o…
Statistics
Populations vs. Samples
Measures of Central Tendency in A Population
Measures of Dispersion in A Population
Coming Up Next
- Now that you have a good idea of how measures of central tendency such as the mean, median, and mode, along with measures of dispersion such as the variance and the standard deviation can help you and your audience understand data without having to look at every data point, let’s move on to ways to understand descriptive statistics for non-scalar v...