Common Measures of Variance
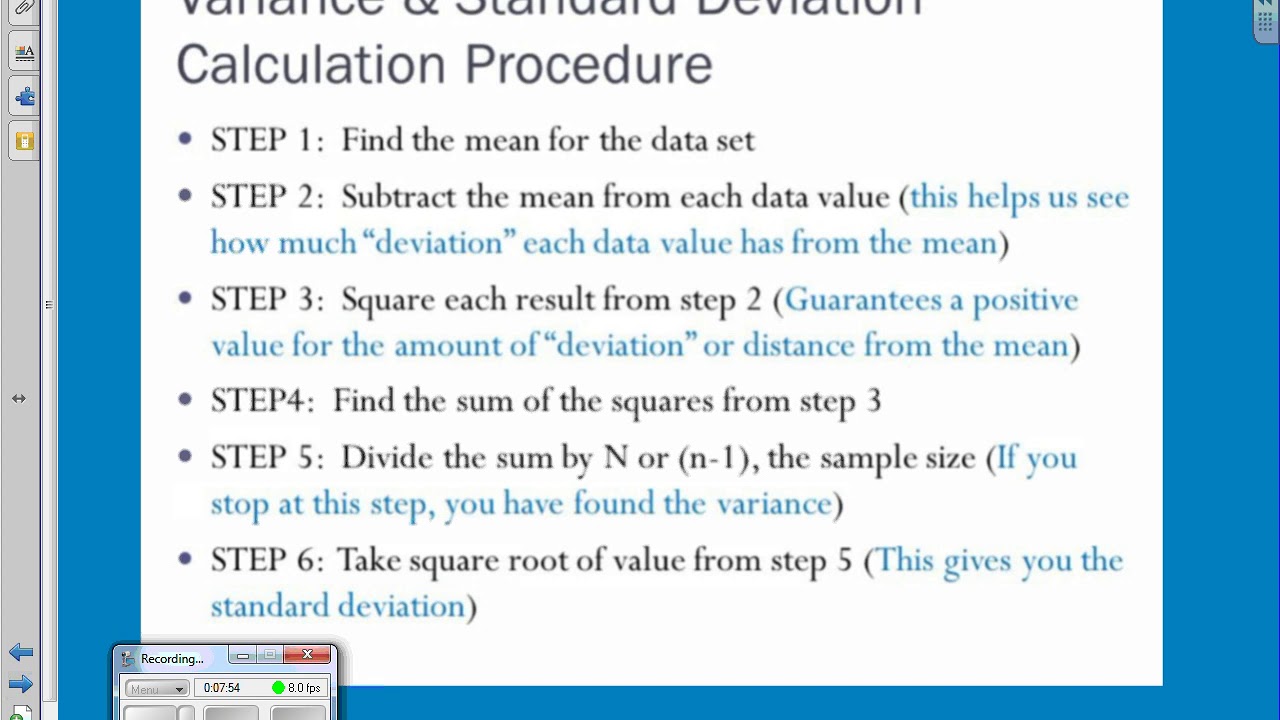
- Find the mean of the data
- Subtract the mean from each value to find the deviation from the mean
- Square the deviation from the mean
- Total the squares of the deviation from the mean
- Divide by the degrees of freedom (one less than the sample size)
- Range: the difference between the highest and lowest values.
- Interquartile range: the range of the middle half of a distribution.
- Standard deviation: average distance from the mean.
- Variance: average of squared distances from the mean.
What are the 4 measures of variability?
There are six steps for finding the standard deviation by hand:
- List each score and find their mean.
- Subtract the mean from each score to get the deviation from the mean.
- Square each of these deviations.
- Add up all of the squared deviations.
- Divide the sum of the squared deviations by n – 1 (for a sample) or N (for a population).
- Find the square root of the number you found.
Why is variance a poor measure of risk?
Variance is an important metric in the investment world. Variability is volatility, and volatility is a measure of risk. It helps assess the risk investors assume when they buy a specific asset and helps them determine whether the investment will be profitable.
What is the formula for calculating variance?
What is the Variance Formula? There are two formulas to calculate variance: Variance % = Actual / Forecast – 1. or. Variance $ = Actual – Forecast. In the following paragraphs, we will break down each of the formulas in more detail. Percent Variance Formula. As the name implies, the percent variance formula calculates the percentage difference between a forecast and an actual result.
What are two measures of variation?
- One is four minutes less than the average of five; four minutes is equal to two standard deviations.
- Binh's wait time of one minute is four minutes less than the average of five minutes.
- Binh's wait time of one minute is two standard deviations below the average of five minutes.

What are measures of variation?
Measures of variation in statistics are ways to describe the distribution or dispersion of your data. In other words, it shows how far apart data points are from each other. Statisticians use measures of variation to summarize their data.
What are the 3 measures of variation?
Measures of VariabilityRange.Interquartile range (IQR)Variance and Standard Deviation.
What are the measurement units of variance?
Variance: The variance (denoted σ2) represents the spread (the dispersion) of the repeated measurements either side of the mean. As the notation implies, the units of the variance are the square of the units of the mean value.
What are the 5 types of absolute measures of variation?
Measures of Absolute VariabilityRange – range.Interquartile Range – IQR.Quartile Deviation.Average Deviation.Standard Deviation – sd.
What is the best measure of variation?
Variability is most commonly measured with the following descriptive statistics:Range: the difference between the highest and lowest values.Interquartile range: the range of the middle half of a distribution.Standard deviation: average distance from the mean.Variance: average of squared distances from the mean.
What are the 3 measures of variability psychology?
Measures of Variability are statistics that describe the amount of difference and spread in a data set. These measures include variance, standard deviation, and standard error of the mean.
What is variance with example?
Variance is the average squared deviations from the mean, while standard deviation is the square root of the variance. Both measures reflect variability in distribution, but their units differ: Standard deviation is expressed in the same units as the original values (e.g., minutes or meters).
What is the range of variance?
The range is the difference between the high and low values. Since it uses only the extreme values, it is greatly affected by extreme values. The variance is the average squared deviation from the mean.
What is the variance in statistics?
In statistics, variance measures variability from the average or mean. It is calculated by taking the differences between each number in the data set and the mean, then squaring the differences to make them positive, and finally dividing the sum of the squares by the number of values in the data set.
What are the 4 measures of variability?
What are the 4 main measures of variability?Range: the difference between the highest and lowest values.Interquartile range: the range of the middle half of a distribution.Standard deviation: average distance from the mean.Variance: average of squared distances from the mean.
What are the 4 measures of dispersion?
Measures of dispersion describe the spread of the data. They include the range, interquartile range, standard deviation and variance. The range is given as the smallest and largest observations. This is the simplest measure of variability.
Which is not a measure of variation?
Interquartile rangeb.) Correct. The mean is a measure of center, not of variation or spread.
What are the measures of variation and why are they important?
An important use of statistics is to measure variability or the spread ofdata. For example, two measures of variability are the standard deviation andthe range. The standard deviation measures the spread of data from the mean orthe average score.
What are the measures of center and variation?
Two measures of center are mean and median. Spread describes the variation of the data. Two measures of spread are range and standard deviation.
Is mode a measure of variation?
Measures of central tendency tell us what is common or typical about our variable. Three measures of central tendency are the mode, the median and the mean. The mode is used almost exclusively with nominal-level data, as it is the only measure of central tendency available for such variables.
What are the properties of variation?
Variance Properties-Properties of Variance ExplainedIt is denoted by the symbol σ2. ... The variance can never be negative. ... The variance is equal to 0 only if the data values are ALL EQUAL to each other. ... The variance is independent of the “change in origin”. ... The variance is affected by the “change in scale”.More items...•
How to find variance in statistics?
In statistics, variance measures variability from the average or mean. It is calculated by taking the differences between each number in the data set and the mean , then squaring the differences to make them positive, and finally dividing the sum of the squares by the number of values in the data set.
What is variance in investing?
Investors can analyze the variance of the returns among assets in a portfolio to achieve the best asset allocation. In financial terms, the variance equation is a formula for comparing the performance of the elements of a portfolio against each other and against the mean.
Why is variance important?
Variability is volatility, and volatility is a measure of risk. It helps assess the risk that investors assume when they buy a specific asset and helps them determine whether the investment will be profitable.
Why do investors use standard deviation?
As noted above, investors can use standard deviation to assess how consistent returns are over time. In some cases, risk or volatility may be expressed as a standard deviation rather than a variance because the former is often more easily interpreted.
What does a large variance mean?
A large variance indicates that numbers in the set are far from the mean and far from each other. A small variance, on the other hand, indicates the opposite. A variance value of zero, though, indicates that all values within a set of numbers are identical. Every variance that isn’t zero is a positive number. A variance cannot be negative.
Why do statisticians use variance?
Statisticians use variance to see how individual numbers relate to each other within a data set, rather than using broader mathematical techniques such as arranging numbers into quartiles. The advantage of variance is that it treats all deviations from the mean as the same regardless of their direction.
Is variance a positive or negative number?
Every variance that isn’t zero is a positive number. A variance cannot be negative. That’s because it’s mathematically impossible since you can’t have a negative value resulting from a square. Variance is an important metric in the investment world. Variability is volatility, and volatility is a measure of risk.
What is variance in statistics?
Variance. The variance is the average squared deviation from the mean. It usefulness is limited because the units are squared and not the same as the original data. The sample variance is denoted by s2, it is an unbiased estimator of the population variance.
What is the coefficient of variation?
The coefficient of variation is expressed as a percent and describes the standard deviation relative to the mean. It can be used to compare variability when the units are different (the units will divide out, providing just a raw number).
What is the Pearson index of skewness?
Pearson's Index of Skewness. Pearson's index of skewness can be used to determine whether the data is symmetric or skewed. If the index is between -1 and 1, then the distribution is symmetric. If the index is no more than -1 then it is skewed to the left and if it is at least 1, then it is skewed to the right.
How many times is the range of a standard deviation?
The range rule of thumb says that the range is approximately four times the standard deviation. Alternatively, the standard deviation is approximately one-fourth the range. That means that most of the data lies within two standard deviations of the mean.
What is range in math?
The range is the difference between the high and low values. Since it uses only the extreme values, it is greatly affected by extreme values.
Range
Range is one of the simplest measures of variation. It is the lowest point of data subtracted from the highest point of data. For example, if your highest point is 10 and your lowest point is 3, then your range would be 7. The range tells you a general idea of how widely spread your data is.
Variance
Variance is the average squared variations of values from the mean. It compares every piece of value to the mean, which is why variance differs from the other measures of variation. Variance also displays the spread of the data set. Typically, the more spread out your data is, the larger the variance is.
Quartiles
Quartiles divide your data into four equal sections, or quarters. They divide the data in ascending order, meaning there are the lower two quartiles and the higher two quartiles.
Interquartile range
Interquartile range (IQR) refers to the middle of your data distribution or the middle of your four quartiles, meaning that the IQR is in the middle of the upper and lower quartiles. The IQR measures how the data is spread around the average. To find the IQR, you need to know the values of Q1 and Q3.
Standard deviation
Standard deviation is the average or standard distance between each point of data and the mean. It is the standard amount of variability in your data set. If you know the variance of your data set, then you can take the square root of that value to find the standard deviation.
Marketing analyst
A marketing analyst studies marketing trends to help decide what a company should sell to boost profits. Their roles could include researching consumer behavior, the current market or even competitor strategies. Marketing analysts can use variation statistics to analyze the variability of a company's sales to see what products sell the most.
Sales representative
A sales representative works closely with the sales team to support customers. They may process orders, provide price quotes or keep records of customers. Sales representatives can use variation statistics to analyze a variety of data sets they may have while working, such as sales records or the population of customers.
What is variance in investing?
Variance is a key concept used by investors to measure the risk and reward associated with a particular investment. Generally, it is used as a relative tool for performance comparison between assets in a data profile to achieve a superior asset allocation. .
How does variance affect data?
However, a variance may manipulate the data by squaring the numbers isolated from the mean value, which are referred to as outliers. Most investors prefer using the standard deviation to assess the consistency of returns since it is easily expressed rather than variance, which may be difficult to interpret.
What is the difference between standard deviation and variance?
The standard deviation shows the position of each value from the mean. Similarly, both standard deviation and variance demonstrate variability in a number set. However, a variance is indicated in larger units such as meters squared while the standard deviation is expressed in original units such as meters.
Why is variance more descriptive than standard deviation?
Due to the larger unit values of expression, a variance number becomes harder to interpret, with many traders opting for standard deviation as a key measure of variability. However, while making statistical analysis, a variance is preferred since it’s more descriptive about variability than the standard deviation.
What is large variability?
However, a minimum variance illustrates a close proximity of figures between each other and from the mean value. Identical values within a data set portray a zero variance. Similarly, every positive number indicates a non-zero variance since a square value cannot be negative.
What is mean in statistics?
Mean Mean is an essential concept in mathematics and statistics. In general, a mean refers to the average or the most common value in a collection of. and other values. Variance analysis may also be used to approximate population variability. Variance is expressed mathematically using the following formula:
Can variance be negative?
Variance cannot be negative because its squares are either positive or zero. For example: Var (X) ≥ 0. The variance of a constant value is equivalent to zero. Var (k) = 0. Variance remains invariant when a constant value is added to all the figures in the data set.
Definition
Variance is a measure of how data points differ from the mean. According to Layman, a variance is a measure of how far a set of data (numbers) are spread out from their mean (average) value.
Formula
As we know already, the variance is the square of standard deviation, i.e.,
Properties
The variance, var (X) of a random variable X has the following properties.
Variance and Standard Deviation
Standard deviation is the positive square root of the variance. The symbols σ and s are used correspondingly to represent population and sample standard deviations.
Frequently Asked Questions – FAQs
In statistics, variance is a measure of spread of values or observations from mean.
What is the measure of variation related to the interquartile range?
A measure of variation related to Interquartile range is Semi Interquartile range. It is the Interquartile range divided by 2. If your data is symmetrically distributed, then the median will lie exactly at the middle of Q1 and Q3. That is between 25th and 75th percentile. In such cases, the the Semi Interquartile range will be equal to the difference between median and 25th percentile, as well as the difference between median and 75th percentile.
Why is standard deviation used?
Thus, Standard Deviation can be used as a measure of variation which is easier to interpret and relate to the data set. The same rule of measure of variation as discussed earlier apply for standard deviation as well. Lower the Standard deviation, better the process.
Does central tendency provide complete information about process performance?
Central tendency alone does not provide complete information about process performance. It should always be looked at alongside variation in the process to get the complete picture. Now that we understand what variation is and why understanding variation is important, let us look at the measures of variation.
Does range give information about the data set?
Although range is fairly simple to understand and calculate, it does not give much information about the data set and the variation within. Since range depends entirely on the extreme values, it does not show how tightly or loosely the data is clustered around the center.
What is range in statistics?
Range is the simplest measure of variation. The range of a dataset is the difference between the highest value and the lowest value in the dataset. Range is also the most affected by outliers as it uses only the extreme values.
Is standard deviation a measure of variability?
Standard deviation is a good measure of variability for normal distributions or distributions that aren’t terribly skewed. Paired with mean this is a good way to describe the data. Variance is not used much as it is represented in squared units and is not an intuitive measure.
What are the factors of variability that need to be considered?
To get the ordinal level of measured data, the IQR (Interquartile Range) and the range (that have been discussed below) are the only factors of measures of variability that need to be considered.
Why do statisticians use variability?
Statisticians use measures of variability to check how far the data points are going to fall from the given central value. That is why statisticians consider variability to get the distribution of the values. The lower dispersion value shows the data points will be grouped nearer to the center.
Which measure is best for skewed distribution?
But it has been seen that variance and SD can easily influence by the outliers. The IQR is the best measure for skewed distribution.
Does variability matter in statistics?
Yes, it matters!! The lower variability considers being ideal as it provides better predictions related to the population. In contrast, the higher variability value considers to be less consistent. This will lead to making predictions much harder. See also The Basic Terminologies of Statistics You Should Know.
What is variance in statistics?
Variance is the average squared difference of the values from the mean . Unlike the previous measures of variability, the variance includes all values in the calculation by comparing each value to the mean. To calculate this statistic, you calculate a set of squared differences between the data points and the mean, sum them, and then divide by the number of observations. Hence, it’s the average squared difference.
What is a measure of variability?
A measure of variability is a summary statistic that represents the amount of dispersion in a dataset. How spread out are the values? While a measure of central tendency describes the typical value, measures of variability define how far away the data points tend to fall from the center. We talk about variability in the context of a distribution of values. A low dispersion indicates that the data points tend to be clustered tightly around the center. High dispersion signifies that they tend to fall further away.
When to use range to compare variability?
Consequently, use the range to compare variability only when the sample sizes are similar.
What is the difference between a standard deviation and a mean?
The standard deviation is the standard or typical difference between each data point and the mean. When the values in a dataset are grouped closer together , you have a smaller standard deviation. On the other hand, when the values are spread out more, the standard deviation is larger because the standard distance is greater.
Population vs Sample Variance
- Different formulas are used for calculating variance depending on whether you have data from a whole population or a sample.
Steps For Calculating The Variance
- The variance is usually calculated automatically by whichever software you use for your statistical analysis. But you can also calculate it by hand to better understand how the formula works. There are five main steps for finding the variance by hand. We’ll use a small data set of 6 scores to walk through the steps.
Why Does Variance Matter?
- Variance matters for two main reasons: 1. Parametric statistical tests are sensitive to variance. 2. Comparing the variance of samples helps you assess group differences.