
What are the 5 triangle theorem?
There are five ways to find if two triangles are congruent: SSS, SAS, ASA, AAS and HL.
How many theorems are there in triangles?
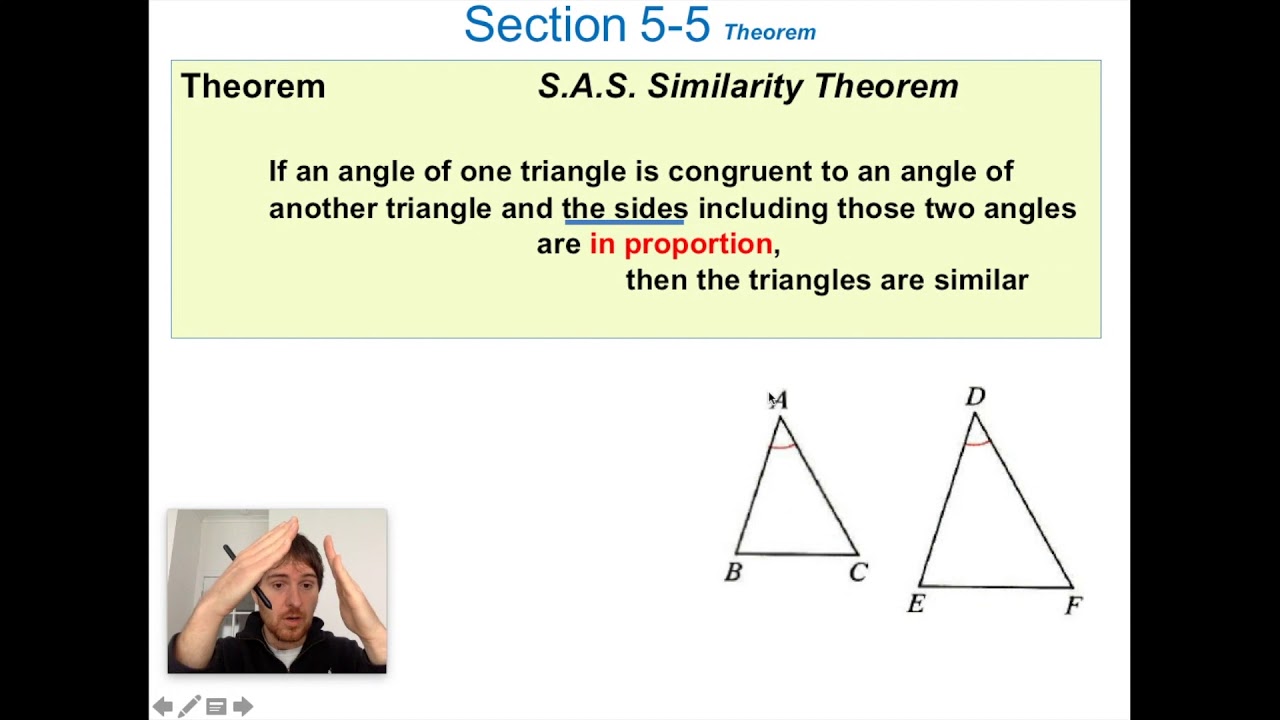
In total, there are 3 theorems for proving triangle similarity: AA Theorem. SAS Theorem. SSS Theorem.
What are the 4 triangle congruence theorems?
There are four commonly used congruence tests. The three sides of one triangle are respectively equal to the three sides of the other triangle. Two sides and the included angle of one triangle are respectively equal to two sides and the included angle of the other triangle.
What are the 3 triangle congruence theorems?
Triangles can be similar or congruent. Similar triangles will have congruent angles but sides of different lengths. Congruent triangles will have completely matching angles and sides.
What are the types of theorem?
In mathematics, the following few are the important types of theorems widely used in various branches of study:Pythagorean theorem.Sine rule.Cosine rule.Mean value theorem.Mid-point theorem.Triangle sum theorem.Isosceles theorem.Factor theorem.More items...•
What are the theorems of triangles Class 10?
Triangles Theorems for Class 10 Two triangles are similar if their corresponding angles are equal and corresponding sides are in the same ratio. In two triangles, if the corresponding angles are equal and corresponding sides are in the same ratio then the two triangles are similar.
What is SAS ASA SSS AAS?
Different rules of congruency are as follows. SSS (Side-Side-Side) SAS (Side-Angle-Side) ASA (Angle-Side-Angle) AAS (Angle-Angle-Side)
What is La theorem?
First, there's the LA theorem. This is the leg-acute theorem. It states that if the leg and an acute angle of one right triangle are congruent to the corresponding leg and acute angle of another right triangle, then the triangles are congruent.
What is SSS theorem?
1: Side-Side-Side (SSS) Theorem. Two triangles are congruent if three sides of one are equal respectively to three sides of the other (SSS=SSS).
How do you use AAS theorem?
The AAS Theorem says: If two angles and the non-included side of one triangle are congruent to the corresponding parts of another triangle, the triangles are congruent. Notice how it says "non-included side," meaning you take two consecutive angles and then move on to the next side (in either direction).
How can you tell AAS and ASA?
If two pairs of corresponding angles and the side between them are known to be congruent, the triangles are congruent. This shortcut is known as angle-side-angle (ASA). Another shortcut is angle-angle-side (AAS), where two pairs of angles and the non-included side are known to be congruent.
How many theorems prove the similarity of triangles?
Similar triangles are easy to identify because you can apply three theorems specific to triangles. These three theorems, known as Angle - Angle (AA), Side - Angle - Side (SAS), and Side - Side - Side (SSS), are foolproof methods for determining similarity in triangles.
1. What is Triangle Inequality?
Inequalities in triangles represent three factors. Firstly, if two sides of a triangle are not similar, the angle on the opposite of the longer sid...
2. How to Prove if Triangles are Similar?
To prove whether two triangles are similar or not, there are few theories. If all the sides are equal to two triangles, then they are identical. Al...
3. What is Congruence?
In Geometry, two figures are congruent when they have similar shapes and sizes. Moreover, two-point becomes congruent when one can transform into t...
4. Why should you read triangle theorems?
Triangle is a simple polygon with three sides and angles, but when you face numerous triangles at once or their types, it becomes slightly complex...
5. What are the benefits of using Vedantu to learn triangles?
Vedantu provides you with the best maths teachers; they break down most complex topics into simple points that are easy to swallow for the students...
6. How to study triangles to score more marks?
To score good marks in maths or any topic of it; only one thing that can help is practising a lot of numeral problems linked with topics. Vedantu h...
7. How to prove two triangles are similar?
To prove triangles are similar, you need to use the conditions explained in the triangle similarity theorem. This theorem has included three subtyp...
8. How do triangle theorems help to solve problems?
Solving a numerical problem in mathematics requires thorough knowledge of the concept. You have to read and practice solved examples rigorously to...
What is the most basic triangle theorem?
Probably the most basic among every triangle theorems, this one proves that all-three angles of this geometric figure constitute a total value of 180 degrees. 5. Triangle Inequality Theorem. Triangle inequality theorem suggests that one side of a triangle must be shorter than the other two.
What are the most popular theorems of triangles?
1. Pythagoras Theorem. Probably the most popular and widely discussed among triangle theorems is Pythagoras’ one.
How many sides does a triangle have?
A triangle is a polygon with three sides, three vertices, and three edges. An angle is formed when two sides meet, and a triangle contains three such angles where their total sum is 180 degree. It is one of the most fundamental figures of geometry, and it is a two dimensional one. Triangle theorems are associated with various subtypes ...
What are the properties of a triangle?
The properties of a triangle include the followings: 1 It has three sides, angles, and vertices 2 The sum of three interior angles are always 180 degree 3 The sum of two sides of this geometrical figure is greater than its third one 4 Area of the product of this figure’s height and the base is equal to twice of its area
What is the difference between an equilateral and an isosceles triangle?
An equilateral triangle is where all 3 sides are equal. An isosceles triangle is where 2 sides are equal. A scalene triangle is where no sides are equal. Since the definition of triangles and its types are now clear, students can now understand the theorems quicker.
What is the focus of the triangle similarity theorem?
Triangle Similarity Theorems. The focus of this theorem is to prove similarity between two triangles. It specifies conditions under which more than one triangle can be regarded as similar. It considers sides and angles to conclude, and once every condition is met, triangles are considered as the same.
How to find the third angle of a triangle?
Since the total value of three angles is 180 degrees, once the value of two angles is known, it is easy to find the third one by subtracting it from 180.
Triangles
Triangles are polygons contained in three sides, three vertices, and three edges. The three sides have three angles, which makes the central ideology of the triangle theorem revolve around the sides and angles of the triangle. The first basic theorem of Triangles is the Pythagoras theorem or Pythagorean theorem.
Properties of Triangle
A triangle has three sides, vertices, and edges. With that, it also possesses the following properties:
Types of Triangles
There are various types of triangles depending on the length of their side and the degree of the angle
Things to Remember
Triangle is categorized into Equilateral, Isosceles, and Scalene based on the length of sides and into Acute-Angled Triangle, Obtuse-Angled Triangle, and Right-Angled Triangle.
Sample Questions
Ques. In the right Δ ABC, the lengths of two of the sides, i.e. Base and perpendicular, are given. What is the size of the hypotenuse? (5 marks) a = 9 cm, b = 10 cm
What is the congruence of triangles?
Triangle Congruence Theorems (SSS, SAS, & ASA Postulates) Triangles can be similar or congruent. Similar triangles will have congruent angles but sides of different lengths. Congruent triangles will have completely matching angles and sides. Their interior angles and sides will be congruent.
What are the three parts of a triangle that must be congruent?
For the two triangles to be congruent, those three parts -- a side, included angle, and adjacent side -- must be congruent to the same three parts -- the corresponding side, angle and side -- on the other triangle, △ Y AK △ Y A K.
What does it mean when two triangles are congruent?
Congruence Definition. Two triangles are congruent if their corresponding sides are equal in length and their corresponding interior angles are equal in measure . We use the symbol ≅ ≅ to show congruence. Corresponding sides and angles mean that the side on one triangle and the side on the other triangle, in the same position, match.
What is the ASA theorem?
ASA Theorem (Angle-Side-Angle) The Angle Side Angle Postulate (ASA) says triangles are congruent if any two angles and their included side are equal in the triangles. An included side is the side between two angles.
What does it mean when a triangle has corresponding sides and angles?
Corresponding sides and angles mean that the side on one triangle and the side on the other triangle, in the same position, match. You may have to rotate one triangle, to make a careful comparison and find corresponding parts.
Which postulate says triangles are congruent?
Perhaps the easiest of the three postulates, Side Side Side Postulate (SSS) says triangles are congruent if three sides of one triangle are congruent to the corresponding sides of the other triangle. This is the only postulate that does not deal with angles.
How to check if a polygon is congruent?
Checking Congruence in Polygons. You can check polygon s like parallelograms, squares and rectangles using these postulates. Introducing a diagonal into any of those shapes creates two triangles. Using any postulate, you will find that the two created triangles are always congruent.
1. The Side-Splitter Theorem
To show this is true, draw the line BF parallel to AE to complete a parallelogram BCEF:
3. Area and Similarity
If two similar triangles have sides in the ratio x:y, then their areas are in the ratio x 2 :y 2
Example
These two triangles are similar with sides in the ratio 2:1 (the sides of one are twice as long as the other):
What is the theorem that holds that the longer side of a triangle will stand opposite the larger angle?
Given unequal angles , the theorem holds that the longer side of the triangle will stand opposite the larger angle, and that the larger angle will stand opposite the longer side. This inequality is helpful to prove triangles aren't congruent.
What happens if one side of a triangle is longer than the sum of the lengths of the other two?
Were one side of a triangle longer than the sum of the lengths of the other two, the triangle could not exist. As one side grows, the other two collapse toward that side until the altitude from the vertex opposite the growing side eventually becomes zero. This (an altitude of zero) would happen if the length of the one side was equal to the sum ...
How to find the exterior angle of a triangle?
1) The exterior angle at a given vertex is equal in measure to the sum of the two remote interior angles. These remote interior angles are those at the other two vertices of the triangle. 2) Knowing this, it follows that the measure of any exterior angle is always greater than the measure of either remote interior angle. The first fact (1), the equality, is useful for proving congruence; the second fact (2), the inequality, is useful for disproving congruence.
What are the two inequalities of a triangle?
It states that the length of a side of a triangle is always less than the sum of the lengths of the other two sides. Can you see why this must be true? Were one side of a triangle longer than the sum of the lengths of the other two , the triangle could not exist. As one side grows, the other two collapse toward that side until the altitude from the vertex opposite the growing side eventually becomes zero. This (an altitude of zero) would happen if the length of the one side was equal to the sum of the lengths of the other two. For this reason, the length of any side must be less than the sum of the lengths of the other sides.
Which theorem states that when sides or angles are equal, so are the sides or angles opposite them?
In essence, this theorem complements the theorem involving isosceles triangles, which stated that when sides or angles were equal, so were the sides or angles opposite them. The theorem about unequal pairs, though, goes a little farther.
What is the law of cosines?
Law of Cosines. If a, b and c are the lengths of the legs of a triangle opposite to the angles A, B and C respectively; then the law of cosines states: a 2 = c 2 + b 2 - 2bc cos A, solving for cos A, cos A = ( b 2 + c 2 - a 2 ) / 2bc. b 2 = a 2 + c 2 - 2ca cos B, solving for cos B, cos B = ( c 2 + a 2 - b 2 ) / 2ca.
Can you find the third angle of a triangle by specifying two angles?
Specifying the three angles of a triangle does not uniquely identify one triangle. Therefore, specifying two angles of a tringle allows you to calculate the third angle only. Given the sizes of 2 angles of a triangle you can calculate the size of the third angle. The total will equal 180° or π radians.
Which theorem states that triangles are congruent?
Three of these theorems do prove triangles to be congruent; SAS (Side-Angle-Side), ASA (Angle-Side-Angle), and SSS (Side-Side-Side). The AA ( Angle-Angle) Theorem states that two triangles with two congruent, corresponding angles are similar NOT necessarily congruent.
What happens if one side of a triangle is longer than the other side?
Prove that if one side of a triangle is longer than another side of the same triangle, then the angle opposite the longer side will be greater than the angle opposite the shorter side. Use the information that#N#displaystyle overline {AB }< overline {AC}#N#for triangle#N#displaystyle ABC#N#.
Triangles
- [Click Here for Sample Questions] Triangles are polygons contained in three sides, three vertices, and three edges. The three sides have three angles, which makes the central ideology of the triangle theorem revolve around the sides and angles of the triangle. The first basic theorem of Triangles is the Pythagoras theorem or Pythagorean theorem. Tr...
Properties of Triangle
- [Click Here for Sample Questions] A trianglehas three sides, vertices, and edges. With that, it also possesses the following properties: 1. Sums of the internal angle of a triangle make a sum of 180° 2. The total sum of the two sides of the triangle is more than the length of the third side 3. The area of the product of this figure’s height and the base is equal to twice its area 4. It is a regular …
Types of Triangles
- [Click Here for Sample Questions] There are various types of trianglesdepending on the length of their side and the degree of the angle
Things to Remember
- Triangle is categorized into Equilateral, Isosceles, and Scalene based on the length of sides and into Acute-Angled Triangle, Obtuse-Angled Triangle, and Right-Angled Triangle.
- In a given ΔABC, with Angle A, Angle B, and Angle C,
The Side-Splitter Theorem
The Angle Bisector Theorem
- To show this is true, we can label the triangle like this: 1. Angle BAD = Angle DAC = x° 2. Angle ADB = y° 3. Angle ADC = (180−y)° Both ABBD and ACDC are equal to sin(y)sin(x), so: ABBD = ACDC In particular, if triangle ABC is isosceles, then triangles ABD and ACD are congruent triangles And the same result is true: ABBD = ACDC
Area and Similarity
- Example:
These two triangles are similar with sides in the ratio 2:1 (the sides of one are twice as long as the other): What can we say about their areas? The answer is simple if we just draw in three more lines: We can see that the small triangle fits into the big triangle four times. So when the lengths … - The General Case:
Triangles ABC and PQR are similar and have sides in the ratio x:y We can find the areas using this formula from Area of a Triangle: Area of ABC = 12bc sin(A) Area of PQR = 12qr sin(P) And we know the lengths of the triangles are in the ratio x:y q/b = y/x, so: q = by/x and r/c = y/x, so r = cy/…