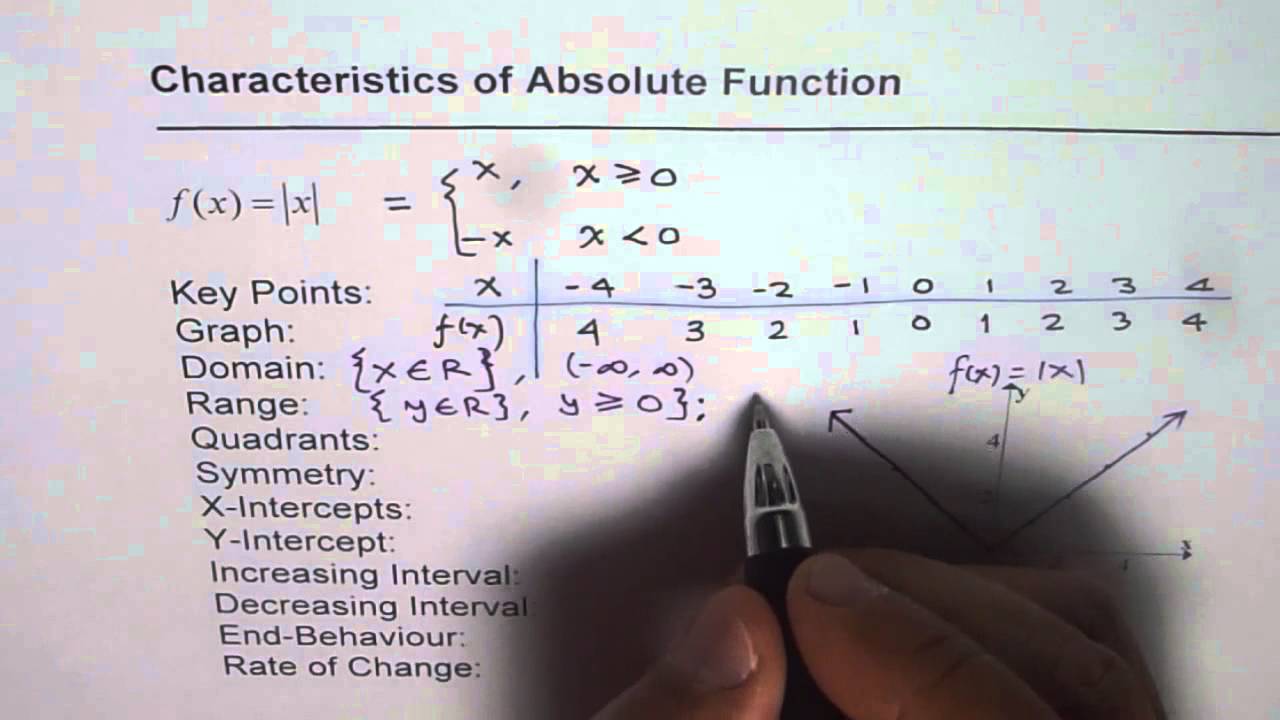
The following are some of the most important features of the absolute value function:
- In its most basic form, the absolute value function is f ( x) = | x |.
- Its domain is all real numbers.
- Its range is all real numbers greater than or equal to zero.
- Its graph is completely above the x -axis.
- Its graph is symmetric with respect to the y axis.
How do you write an absolute value function?
- 2x−5| = 9 | 2 x − 5 | = 9
- |1 −3t| = 20 | 1 − 3 t | = 20
- |5y−8| = 1 | 5 y − 8 | = 1
What is the absolute value of a function?
What is an Absolute Value Function?
- y = |x
- |y = |x + 5
- |y = |x – 10| + 9
How do you calculate absolute value?
Square both coefficients.
- Coefficients: (3, -4)
- Distance formula: 3 2 + ( − 4) 2 {\displaystyle {\sqrt {3^ {2}+ (-4)^ {2}}}}
- Square the coefficients: ' 9 + 16 {\displaystyle {\sqrt {9+16}}}
- Note: Review the distance formula if you're confused. Note now squaring both numbers makes them positive, effectively taking absolute value for you.
What are absolute value functions?
Key Concepts
- Applied problems, such as ranges of possible values, can also be solved using the absolute value function. ...
- The graph of the absolute value function resembles a letter V. ...
- In an absolute value equation, an unknown variable is the input of an absolute value function.
What are 2 things about absolute value?
The most common way to represent the absolute value of a number or expression is to surround it with the absolute value symbol: two vertical straight lines. |6| = 6 means “the absolute value of 6 is 6.” |–6| = 6 means “the absolute value of –6 is 6.” |–2 – x| means “the absolute value of the expression –2 minus x.”
What is a fact about absolute value?
absolute value, Measure of the magnitude of a real number, complex number, or vector. Geometrically, the absolute value represents (absolute) displacement from the origin (or zero) and is therefore always nonnegative. If a real number a is positive or zero, its absolute value is itself.
Why are absolute value functions important?
The absolute value function is commonly used to determine the distance between two numbers on the number line. Given two values a and b, then |a−b| will give the distance, a positive quantity, between these values, regardless of which value is larger.
How do you explain absolute value?
Definitions: The absolute value (or modulus) | x | of a real number x is the non-negative value of x without regard to its sign. For example, the absolute value of 5 is 5, and the absolute value of −5 is also 5. The absolute value of a number may be thought of as its distance from zero along real number line.
What is absolute value defined as?
Absolute value is a term used in mathematics to indicate the distance of a point or number from the origin (zero point) of a number line or coordinate system. This can apply to Scalar or vector quantities.
How is the absolute value function used in real life?
The absolute value is used in the real world to define the DIFFERENCE or change from one point to another. A good example I found was that if the everybody is going 55 mph and you are going 70 or 40 mph you will most likely get a ticket. It matters because the difference between you and everybody else is 15 mph.
What is a real life example of an absolute value function?
Absolute Value Functions Real World Applications For example, if your student walks to school and it is a 2 mile walk we would say he or she walked 2 miles. However, when the student walks home from school that night we would not say it's a (Negative) -2 mile walk.
What is absolute value used for in real life?
The absolute value is used in the real world to define the DIFFERENCE or change from one point to another. A good example I found was that if the everybody is going 55 mph and you are going 70 or 40 mph you will most likely get a ticket. It matters because the difference between you and everybody else is 15 mph.
What is the best way to describe the absolute value of 2?
The absolute value of a positive number like 2 is just equal to 2 since that's how far away it is from zero on the number line.
Why are absolute values always positive?
Absolute value is always positive. Since it's the distance a number is from 0, it would always be positive.
What is an absolute value of 4?
4The absolute value of 4 is 4 and –3 is 3. Subtract the smaller number from the larger and you get 4 – 3 = 1. The larger absolute value in the equation was 4 or a positive number so you give the result a positive result.
What is absolute value function?
An absolute value function is a function that contains an algebraic expression within absolute value symbols. Recall that the absolute value of a number is its distance from 0 on the number line.
How to find the axis of symmetry of a graph?
2. Plot the points on a coordinate plane and connect them. Observe that the graph is V-shaped. ( 1) The vertex of the graph is (0, 0). ( 2) The axis of symmetry ( x = 0 or y -axis) is the line that divides the graph into two congruent halves. ( 3) The domain is the set of all real numbers.
What is an absolute value equation?
An absolute value equation is an equation in which the unknown variable appears in absolute value bars. For example, Solutions to Absolute Value Equations. For real numbers and , an equation of the form with will have solutions when or If the equation has no solution.
Do absolute values always intersect the horizontal axis?
The graph of an absolute value function will intersect the vertical axis when the input is zero. No, they do not always intersect the horizontal axis. The graph may or may not intersect the horizontal axis, depending on how the graph has been shifted and reflected.
What are the characteristics of an absolute value function?
These characteristics are as follows: Its domain is all real numbers. Its range is all real numbers greater than or equal to zero. Its graph lies completely above the x -axis. Its graph is symmetric with respect to the y -axis.
What is the absolute value of a number?
Therefore, the absolute value of a number is the positive value of that number. For example, the absolute value of -5 is 5 and the absolute value of 5 is 5. It's really quite easy. You just use the positive value of the number.
tip for success
It can help to visualize the graph of an absolute value function as the graph of the identity function, f (x)= x f ( x) = x where, for all negative input, the function value is forced to be positive.
Example: Determine a Number within a Prescribed Distance
Describe all values x x within or including a distance of 4 from the number 5.
Example: Resistance of a Resistor
Electrical parts, such as resistors and capacitors, come with specified values of their operating parameters: resistance, capacitance, etc. However, due to imprecision in manufacturing, the actual values of these parameters vary somewhat from piece to piece even when they are supposed to be the same.
Try It
Students who score within 20 points of 80 will pass a test. Write this as a distance from 80 using absolute value notation.
Try It
Write the equation for the absolute value function that is horizontally shifted left 2 units, vertically flipped, and vertically shifted up 3 units.
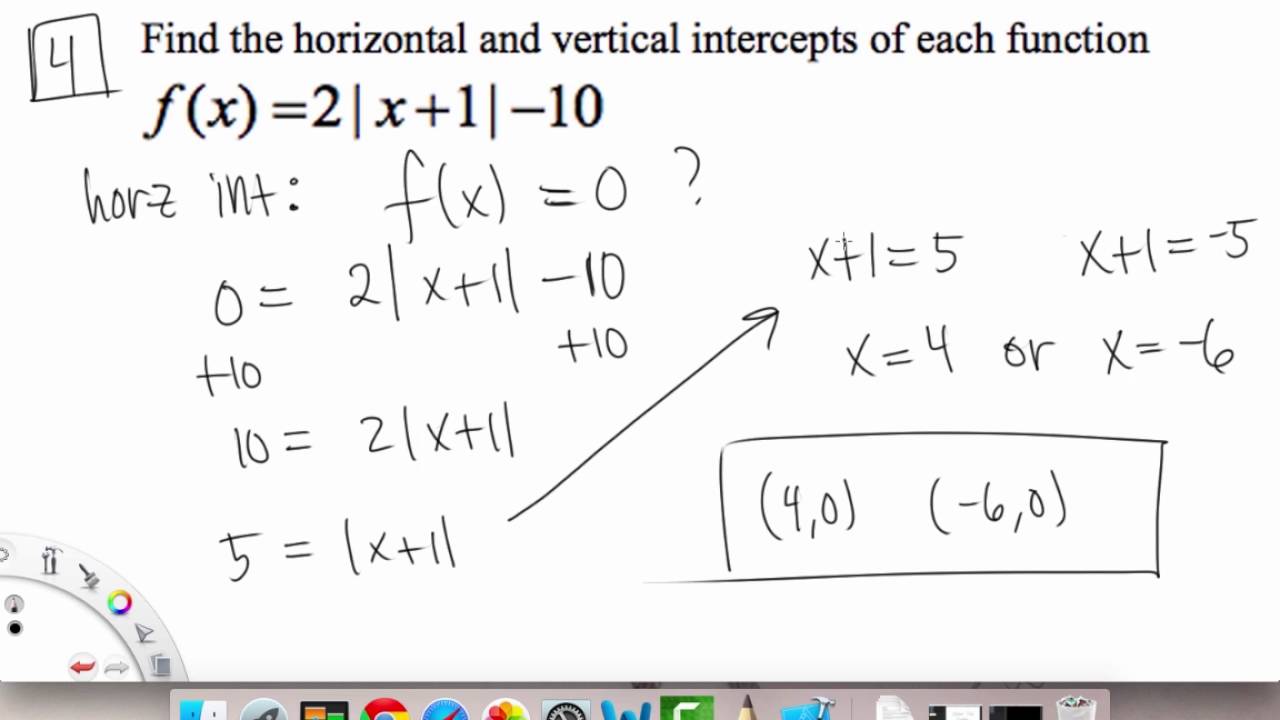
How To: Given the formula for an absolute value function, find the horizontal intercepts of its graph
Isolate the absolute value term. Use |A|= B | A | = B to write A =B A = B or −A = B − A = B, assuming B> 0 B > 0. Solve for x x.
tip for success
The places where a graph crosses the horizontal axis are sometimes called horizontal intercepts , x-intercepts, or zeros.
