Trigonometric Functions
| 1. | What Are Trigonometric Functions? |
| 2. | Trigonometric Functions Formulas |
| 3. | Trigonometric Functions Values |
| 3. | Trig Functions in Four Quadrants |
| 4. | Trigonometric Functions Graph |
How to evaluate trigonometric function?
- Find the reference angle for the given angle.
- Rewrite the original trig function with the reference angle.
- Affix the appropriate sign to the trig function (+ or -), depending which quadrant the angle terminates in.
- Evaluate the trig function.
How to find exact value of trig functions?
How to find the exact value of any trig function:Determine which quadrant the angle is in (sketch the angle if needed)If it's a quadrantal angle (0, pi/2, pi...
Which trigonometric functions always have values less than 1?
You found them by dividing the length of a leg by the hypotenuse. The hypotenuse is the longest side, so the numerator is less than the denominator. That means the output of the sine or cosine function is always less than 1. Keep in mind that the opposite side for one acute angle is the adjacent side of the other acute angle.
Which are the six functions in trigonometry?
These are:
- Cosecant (csc)
- Secant (sec)
- Cotangent (cot)

How do you find the value of trigonometric functions?
0:216:23Finding Trigonometric Function Values Given One Trig ... - YouTubeYouTubeStart of suggested clipEnd of suggested clipSide over the hypotenuse the length of the opposite over the length of the hypotenuse cosine ofMoreSide over the hypotenuse the length of the opposite over the length of the hypotenuse cosine of theta that's the adjacent length over the length of the hypotenuse.
What are the common trigonometric values?
The trig special angles (30, 45, 60, and 90) are in the first quadrant where everything is positive. In the second quadrant, tangent, cotangent, cosine and secant are negative, while sine and cosecant are positive.
What are the 6 trigonometric functions and their definitions?
Definitions of the six functionsSinesin x = o hThe three primary functionsCosinecos x = a hTangenttan x = o a
What are the six trig function values of?
For any right triangle, there are six trig ratios: Sine (sin), cosine (cos), tangent (tan), cosecant (csc), secant (sec), and cotangent (cot). Here are the formulas for these six trig ratios: Given a triangle, you should be able to identify all 6 ratios for all the angles (except the right angle).
How do you memorize trigonometric functions?
0:382:10Trick for Memorizing the Signs of Trig Functions ❖ All Students Take ...YouTubeStart of suggested clipEnd of suggested clipOne of those letters correspond. To going counterclockwise around our coordinate plank which is theMoreOne of those letters correspond. To going counterclockwise around our coordinate plank which is the way we number our quadrants anyway all students take calculus.
What is the value of 1 in trigonometry?
Values of Trigonometric RatiosAngles0∘90∘Cos θ10Tan θ0∞Cot θ∞0Sec θ1∞3 more rows
What are the 3 types of trigonometry?
The three basic functions in trigonometry are sine, cosine and tangent.
How do you solve 6 trig functions?
1:309:04Evaluate the Six Trigonometric Functions of the Angle - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo if we're here adjacent means next to that's going to be this side here that's 4 over theMoreSo if we're here adjacent means next to that's going to be this side here that's 4 over the hypotenuse which is five. So that's four-fifths for the cosine.
How do you evaluate 6 trig functions?
1:463:40Evaluate the six trigonometric functions for the given real numberYouTubeStart of suggested clipEnd of suggested clipFor tangent we put the Y over the X so I have a negative 1/2 divided by negative square root of 3/2.MoreFor tangent we put the Y over the X so I have a negative 1/2 divided by negative square root of 3/2.
Why there are only 6 trigonometric ratios?
There are only 6 trigonometric ratios because only 6 ratios can define ratios of all sides. For example, If you want ratio between perpendicular and hypotenuse there is sin. If you want ratio between perpendicular and base there is tan.
Which common trigonometric value is undefined?
Trigonometric functions are undefined when they represent fractions with denominators equal to zero. Cosecant is the reciprocal of sine, so the cosecant of any angle x for which sin x = 0 must be undefined, since it would have a denominator equal to 0. The value of sin (0) is 0, so the cosecant of 0 must be undefined.
How do you find common angles in trigonometry?
0:0617:25Unit Circle Finding Trig Values - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd this is the x-value. Cosine is adjacent over hypotenuse. So that's x over 1 sine is oppositeMoreAnd this is the x-value. Cosine is adjacent over hypotenuse. So that's x over 1 sine is opposite over hypotenuse. That's why over 1 and tangent is opposite over adjacent which is y over X.
How do you find the 6 trigonometric functions?
2:079:04Evaluate the Six Trigonometric Functions of the Angle - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo seeking is abbreviated SEC. And you can just take the reciprocal or flip that fraction over. SoMoreSo seeking is abbreviated SEC. And you can just take the reciprocal or flip that fraction over. So five over four and then the last one the tangent of theta we're using the opposite.
What is SOH CAH TOA?
"SOHCAHTOA" is a helpful mnemonic for remembering the definitions of the trigonometric functions sine, cosine, and tangent i.e., sine equals opposite over hypotenuse, cosine equals adjacent over hypotenuse, and tangent equals opposite over adjacent, (1) (2) (3) Other mnemonics include.
What is the value of sin 60 degrees?
The value of sin 60 degrees is given by: sin 60° = √3/2
Is the value of sin 45 degrees 1/2?
No, the value of sin 45° is not equal to 1/2, but its value is 1/√2.
Write the values of sin 30° and cos 60°.
The values of sin 30° and cos 60° are: sin 30° = 1/2 cos 60° = 1/2
How do you find the value of tan 45°?
tan 45° = sin 45°/cos 45° = (1/√2)/ (1/√2) = 1 Therefore, the value of tan 45° = 1.
What are the values of trigonometric functions?
The values of trigonometric functions for 0°, 30°, 45°, 60° and 90° are commonly used to solve trigonometry problems.
What is trigonometry value?
Trigonometry values are all about the study of standard angles for a given triangle with respect to trigonometric ratios. The word ‘trigon’ means triangle and ‘metron’ means ‘measurement’. It’s one of the major concepts and part of geometry, where the relationship between angles and sides of a triangle is explained.
What is trigonometric function?
In mathematics, the trigonometric functions (also called circular functions, angle functions or goniometric functions) are real functions which relate an angle of a right-angled triangle to ratios of two side lengths.
What are the reciprocals of trigonometric functions?
Their reciprocals are respectively the cosecant, the secant, and the cotangent, which are less used. Each of these six trigonometric functions has a corresponding inverse function (called inverse trigonometric function ), and an equivalent in the hyperbolic functions as well.
What are the points on a trigonometric plot?
Plot of the six trigonometric functions, the unit circle, and a line for the angle θ = 0.7 radians. The points labelled 1, Sec (θ), Csc (θ) represent the length of the line segment from the origin to that point. Sin (θ), Tan (θ), and 1 are the heights to the line starting from the x -axis, while Cos (θ), 1, and Cot (θ) are lengths along the x -axis starting from the origin.
How do derivatives of trigonometric functions result from those of sine and cosine?
The derivatives of trigonometric functions result from those of sine and cosine by applying quotient rule. The values given for the antiderivatives in the following table can be verified by differentiating them. The number C is a constant of integration .
What is Pythagorean identity?
The Pythagorean identity, is the expression of the Pythagorean theorem in terms of trigonometric functions. It is
What is the smallest period in trigonometric functions?
All trigonometric functions are periodic functions of period 2π. This is the smallest period, except for the tangent and the cotangent, which have π as smallest period. This means that, for every integer k, one has
When were trigonometric functions first used?
While the early study of trigonometry can be traced to antiquity, the trigonometric functions as they are in use today were developed in the medieval period. The chord function was discovered by Hipparchus of Nicaea (180–125 BCE) and Ptolemy of Roman Egypt (90–165 CE). The functions of sine and versine (1 - cosine) can be traced back to the jyā and koti-jyā functions used in Gupta period Indian astronomy ( Aryabhatiya, Surya Siddhanta ), via translation from Sanskrit to Arabic and then from Arabic to Latin. (See Aryabhata's sine table .)
What are Trigonometric Functions?
There are six basic trigonometric functions used in Trigonometry. These functions are trigonometric ratios. The six basic trigonometric functions are sine, cosine, secant, co-secant, tangent, and co-tangent. The trigonometric functions and identities are the ratio of sides of a right-angled triangle.
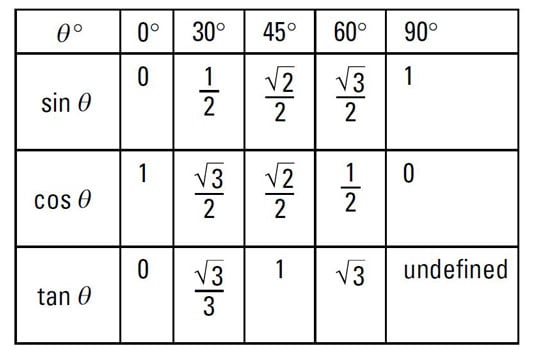
Principal Values of Trigonometric Functions
The trigonometric functions have a domain θ, which is in degrees or radians. Some of the principal values of θ for the different trigonometric functions are presented below in a table. These principal values are also referred as standard values and are frequently used in calculations.
Trigonometric Functions in Four Quadrants
The angle θ is an acute angle (θ < 90) and is measured with reference to the positive x-axis, in the anticlockwise direction. Further, these trigonometric ratios have different numeric signs (+ or -) in the different quadrants, which are based on the positive or negative axis of the quadrant.
Graphing Trigonometric Functions
The graphs of trigonometric functions have the domain value of θ represented on the horizontal x-axis and the range value represented along the vertical y-axis. The graph of Sinθ and Tanθ passes through the origin and the graphs of other trigonometric functions do not pass through the origin. The range of Sinθ and Cosθ is limited to [-1, 1].
Domain and Range of Trigonometric Functions
The value of θ represents the domain of the trigonometric functions and the resultant value is the range of the trigonometric function. The domain values of θ are in degrees or radians and the range is a real number value.
Trigonometric Function Formulas
The trigonometric functions formulas are broadly divided into reciprocal identities, Pythagorean formulas, sum and difference of identities, formulas for multiple and sub-multiple angles, sum and product of identities. All of these below formulas can be easily derived using the ratio of sides of a right-angled triangle.
Inverse Trigonometric Functions
Inverse trigonometric functions are the inverse ratio of the basic trigonometric ratios. Here the basic trigonometric function of Sin θ = x, can be changed to Sin -1 x = θ. Here x can have values in whole numbers, decimals, fractions, or exponents. For θ = 30° we have θ = Sin -1 (1/2).
What are the most common trigonometric functions?
Sine, cosine, and tangent are the most widely used trigonometric functions. Their reciprocals, though used, are less common in modern mathematics. Trigonometric functions are also called circular functions. There are two main ways in which trigonometric functions are typically discussed: in terms of right triangles and in terms of the unit circle. ...
What are the two ways trigonometric functions are discussed?
There are two main ways in which trigonometric functions are typically discussed: in terms of right triangles and in terms of the unit circle. The right-angled triangle definition of trigonometric functions is most often how they are introduced, followed by their definitions in terms of the unit circle.
What are the reciprocals of cosine and tangent?
Cosecant, secant, and cotangent are the reciprocals of sine, cosine, and tangent, respectively. As such, as long as we remember the definitions of sine, cosine, and tangent, we can take their reciprocals to determine the definitions of cosecant, secant, and cotangent.
What is the period of a cosine?
The sine, cosine, cosecant, and secant functions have a period of 2π. The tangent and cotangent functions have a period of π.
What is the periodic function of a trigonometric function?
Trigonometric functions are periodic functions. A periodic function is a function , f, in which some positive value, p, exists such that. f (x+p) = f (x) for all x in the domain of f, p is the smallest positive number for which f is periodic, and is referred to as the period of f.
How to determine the value of a trigonometric function in any other quadrant?
Once we determine the reference angle, we can determine the value of the trigonometric functions in any of the other quadrants by applying the appropriate sign to their value for the reference angle.
How many trigonometric functions are there in each quadrant?
Below is a table showing the signs of the 6 trigonometric functions in each quadrant.
What Are The Principal Value Of Trigonometric Functions?
The solutions of trigonometric functions, for which the θ value lies between 0 < θ < 2π, are called the principal values of the trigonometric functions. The value of the trigonometric function repeat after an interval of 2π. The following are the standard values of the trigonometric functions.
How To Find Principal Values Of Trigonometric Functions?
The principal values of trigonometric functions can be computed through a sequence of steps. First, we find the quadrant in which this principle value exists. Here θ is the acute angle and the principal values in the four quadrants can be obtained as follows.
General Solution Vs Principal Values Of Trigonometric Functions
The general solutions represent all the higher values of θ which can be obtained using the principal values of trigonometric functions.
Solved Examples on Principal Value Of Trigonometric Functions
Example 1: Find the principal value of the trigonometric function of Cosx = √3 2 3 2.
FAQs on Principal Value Of Trigonometric Function
The solutions of trigonometric functions, for which the θ value lies between 0 < θ < 2π, are called the principal values of the trigonometric functions. The value of the trigonometric function repeat after an interval of 2π. The principal value of trigonometric function cn be easily computed from the general solution of the trigonometric function.
Finding Exact Values of Trigonometric Ratios
Find the exact values indicated. What this means is don't use your calculator to find the value (which will normally be a decimal approximation). Keep everything in terms of surds (square roots). You will need to use Pythagoras' Theorem.
Finding Trigonometric Ratios Using Calculator
Suggestion: Go find the instruction book for your calculator. You are sure to need it in this section. Each calculator brand and model is a bit different - please don't expect your teacher to know how to use every model of every brand of calculator!
Finding Angles Given The Trig Ratio
We are now going to work the other way around. We may know the final trigonometeric ratio, but we don't know the original angle.
Steps to Find the Values of Trigonometric Functions Given Information About an Angle
Step 1: Complete the triangle for the given quadrant by substituting the known values from the trigonometric function given and using the Pythagorean Theorem.
Definitions & Equations for Finding the Values of Trigonometric Functions Given Information about an Angle
Trigonometric Functions: A function that takes an input of an angle and returns the ratio of side lengths for the right triangle. The basic functions are {eq}\sin (x) {/eq}, {eq}\cos (x) {/eq}, and {eq}\tan (x) {/eq}.
Overview
Algebraic values
The algebraic expressions for the most important angles are as follows:
(zero angle) (right angle)
Writing the numerators as square roots of consecutive non-negative integers, with a denominator of 2, provides an easy way to remember the values.
Such simple expressions generally do not exist for other angles which are rati…
Notation
Conventionally, an abbreviation of each trigonometric function's name is used as its symbol in formulas. Today, the most common versions of these abbreviations are "sin" for sine, "cos" for cosine, "tan" or "tg" for tangent, "sec" for secant, "csc" or "cosec" for cosecant, and "cot" or "ctg" for cotangent. Historically, these abbreviations were first used in prose sentences to indicate particular line segments or their lengths related to an arc of an arbitrary circle, and later to indicate …
Right-angled triangle definitions
If the acute angle θ is given, then any right triangles that have an angle of θ are similar to each other. This means that the ratio of any two side lengths depends only on θ. Thus these six ratios define six functions of θ, which are the trigonometric functions. In the following definitions, the hypotenuse is the length of the side opposite the right angle, opposite represents the side opposite the …
Radians versus degrees
In geometric applications, the argument of a trigonometric function is generally the measure of an angle. For this purpose, any angular unit is convenient, and angles are most commonly measured in conventional units of degrees in which a right angle is 90° and a complete turn is 360° (particularly in elementary mathematics).
However, in calculus and mathematical analysis, the trigonometric functions are generally regarde…
Unit-circle definitions
The six trigonometric functions can be defined as coordinate values of points on the Euclidean plane that are related to the unit circle, which is the circle of radius one centered at the origin O of this coordinate system. While right-angled triangle definitions allow for the definition of the trigonometric functions for angles between 0 and radians (90°), the unit circle definitions allow the domain …
In calculus
The modern trend in mathematics is to build geometry from calculus rather than the converse. Therefore, except at a very elementary level, trigonometric functions are defined using the methods of calculus.
Trigonometric functions are differentiable and analytic at every point where they are defined; that is, everywhere for the sine and the cosine, and, for the tange…
Basic identities
Many identities interrelate the trigonometric functions. This section contains the most basic ones; for more identities, see List of trigonometric identities. These identities may be proved geometrically from the unit-circle definitions or the right-angled-triangle definitions (although, for the latter definitions, care must be taken for angles that are not in the interval [0, π/2], see Proofs of trigonometric identities). For non-geometrical proofs using only tools of calculus, one may use …
Right Triangle Definition
Unit Circle Definition
Trigonometric Function Values For The Special Angles
Reference Angle
Trigonometric Functions Are Periodic Functions
Trigonometric Functions Are Odd Or Even
- An odd function is a function in which -f(x)=f(-x). It has symmetry about the origin. An even function is a function in which f(x)=f(-x) meaning that reflecting the graph across the y-axis will yield the same graph. Of the 6 trigonometric functions, sine, tangent, cosecant, and cotangent are odd functions. Cosine and secant are even functions. Ther...
Inverse Trigonometric Functions
Graphs of The Trigonometric Functions