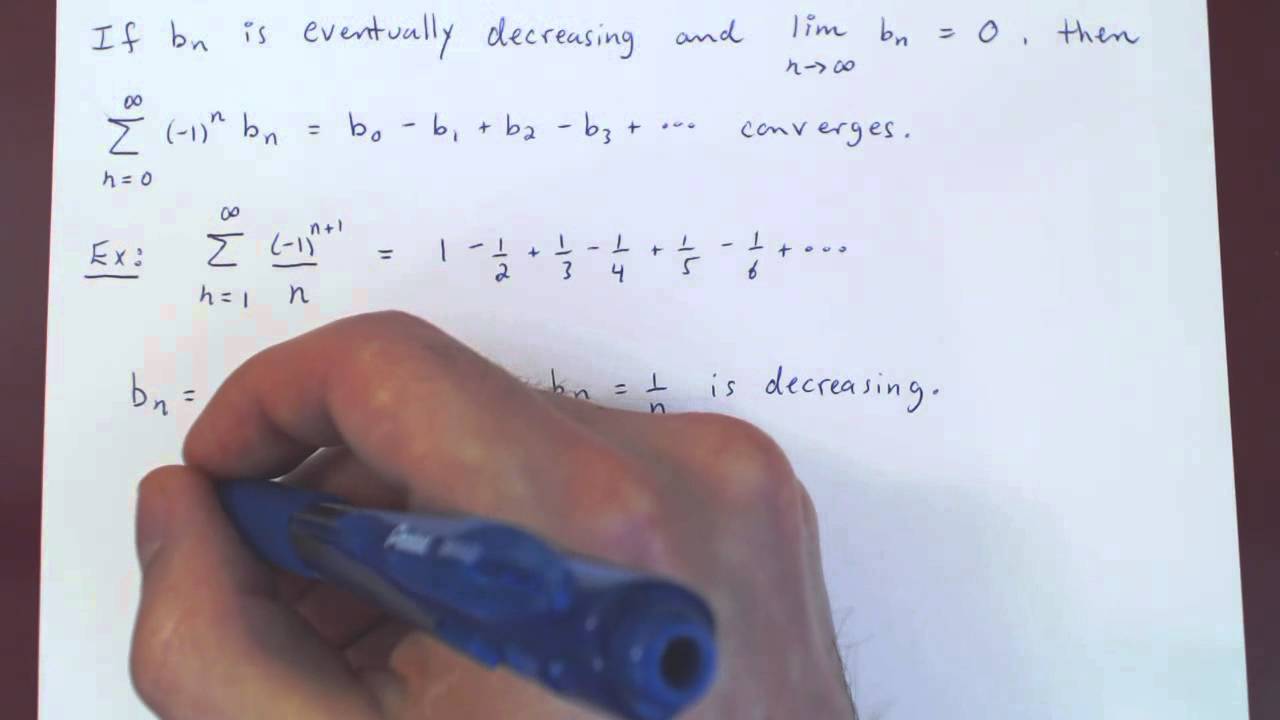
The alternating series test (or also known as the Leibniz test
Alternating series test
In mathematical analysis, the alternating series test is a method used to prove that an alternating series with terms that decrease in absolute value is a convergent series. The test was used by Gottfried Leibniz and is sometimes known as Leibniz's test, Leibniz's rule, or the Leibniz criterion.
What is the alternating series test for convergence?
The alternating series test for convergence lets us say whether an alternating series is converging or diverging. If you can’t be sure that 0 < a n + 1 < a n 0<a_ {n+1}<a_n 0 < a n + 1 < a n just by looking at the table, you can always take the derivative of a n a_n a n to double-check.
How do you know if a series is alternating?
In order to use the alternating series test, the series must be alternating. In other words, the series are in the form: ≤0. An alternating series is not limited to these two forms because the exponent on the (-1) can vary.
Why is the alternating series test applicable to a function?
The alternating series test is applicable because the series is alternating: the odd terms are positive and the even terms are negative. i. Check that is decreasing. The function is decreasing when its derivative is negative.
Is alternating series convergent or divergent?
We will also examine the convergence of alternating series by using a method called the alternating series test. The test requires two conditions, which is listed below. Keep in mind that if you cannot fulfill these conditions, that does not mean the alternating series is divergent. There is still a possibility that it is convergent.
What does the alternating series test prove?
In mathematical analysis, the alternating series test is the method used to show that an alternating series is convergent when its terms (1) decrease in absolute value, and (2) approach zero in the limit.
What defines an alternating series?
Definition of alternating series : a mathematical series in which consecutive terms are alternatively positive and negative.
Does alternating series test tell you about divergence?
No, it does not establish the divergence of an alternating series unless it fails the test by violating the condition limn→∞bn=0 , which is essentially the Divergence Test; therefore, it established the divergence in this case.
How do you identify alternating series?
0:0519:40Alternating Series Test - YouTubeYouTubeStart of suggested clipEnd of suggested clipNow you need to know what an alternating series is and it's basically a series that alternates inMoreNow you need to know what an alternating series is and it's basically a series that alternates in sign it's constantly changing from positive to negative and so forth so let me give an example of one.
Does alternating series converge or diverge?
Both conditions are met and so by the Alternating Series Test the series must converge. The series from the previous example is sometimes called the Alternating Harmonic Series.
How can you tell if an alternating series is absolutely convergent?
In an Alternating Series, every other term has the opposite sign. AST (Alternating Series Test) Let a1 - a2 + a3 - a4+... be an alternating series such that an>an+1>0, then the series converges. The error made by estimating the sum, Sn is less than or equal to an+1, i.e. E = |S - Sn| ≤ an+1.
Do alternating P series always converge?
The p-series rule (or p-series test) states that if p>1, then the series converge. Otherwise, the series diverge.
Does an alternating sequence converge?
The signs of the general terms alternate between positive and negative. Like any series, an alternating series converges if and only if the associated sequence of partial sums converges.
What happens if alternating series test fails?
(2) “If a given alternating series fails to satisfy one or more of the above three conditions, then the series diverges.” We need to realize the basic logic here: The contraposition of “If A is true, then B is true.” is “If B is false, then A is false.” These two statements are equivalent.
What does the ratio test tell us?
The ratio test states that: if L < 1 then the series converges absolutely; if L > 1 then the series diverges; if L = 1 or the limit fails to exist, then the test is inconclusive, because there exist both convergent and divergent series that satisfy this case.
Why does the alternating harmonic series converge?
Since the odd terms and the even terms in the sequence of partial sums converge to the same limit S , S , it can be shown that the sequence of partial sums converges to S , S , and therefore the alternating harmonic series converges to S .
Can you use nth term test for alternating series?
does not pass the first condition of the Alternating Series Test, then you can use the nth term test for divergence to conclude that the series actually diverges. Since the first hypothesis is not satisfied, the alternating series test does not apply.
What is an alternating series an alternating series is a whose terms are?
An alternating series is a series whose terms are al- ternately positive and negative. We look at a couple of examples. Example 1.2. (i) The series (−1)n is an alternating series - for each odd n it is negative and for each even n it is positive.
What is an alternating harmonic series?
The alternating harmonic series is the series. which is the special case of the Dirichlet eta function and also the. case of the Mercator series.
What does the word alternating means?
to interchange repeatedly and regularly with one another in time or place; rotate (usually followed by with): Day alternates with night. to change back and forth between conditions, states, actions, etc.: He alternates between hope and despair.
What is an alternating series an alternating series is a --- Select --- whose terms are?
An alternating series is a series whose terms are alternately positive and negative.
What is the alternating series test?
The alternating series test (also known as the Leibniz test), is type of series test used to determine the convergence of series that alternate. Keep in mind that the test does not tell whether the series diverges. In order to use this test, we first need to know what a converging series and a diverging series is.
What is divergent series?
A divergent series is an infinite series where the sum is infinity. For example, the series
What does the p-series rule state?
The p-series rule (or p-series test) states that if p>1, then the series converge. Otherwise, the series diverge. Notice that the series that we have is very similar to it.
What does it mean when the series diverge is 0?
It just means the test has failed, and you would have to use something else to test the convergence.
Does the numerator change?
Notice how the numerator never changes, but the denominator is getting bigger and bigger. As the denominator gets bigger, then the numbers itself get smaller. Hence we can conclude that the sequence is decreasing, and condition is fulfilled. Some teachers may not see this method as legitimate for more complicating questions (because it's harder to compare). In that case, look at the other methods.
Is an alternating series convergent?
An alternating series is not limited to these two forms because the exponent on the (-1) can vary. Now the alternating series test states that if the two following conditions are met, then the alternating series is convergent: Definition 6: Alternating Series Test. For the second condition, b n.
What is the alternating series test?
The alternating series test is one of the common convergence tests you’ll learn in your precalculus and calculus class. It’s also one of the easiest tests to apply, so it’ll come in handy when we’re left with a few choices in testing the convergence of a particular series.
How to tell if a series is an alternating series?
Step 1: Check if the series is an alternating series – they are normally of the forms, ∑ n = 1 ∞ ( − 1) n a n or ∑ n = 1 ∞ ( − 1) n + 1 a n .
What test confirms convergence?
To confirm the convergence of a particular series, the alternating series test plays a huge role. We’ll learn more about the process and conditions of this particular test in the next sections.
What happens when alternating series meets two conditions?
Step 3: If the alternating series meets the two conditions, the series is convergent.
When is a series convergent?
According to the alternating series test, if the alternating series’s terms continue to decrease and approach 0, the series is convergent.
Is 2 n less than or equal to a 1.?
By rewriting the expression the 2 n th partial sum, we can see that s 2 n is indeed less than or equal to a 1.
When we use the alternating series test, do we need to make sure that we separate the series?
When we use the alternating series test, we need to make sure that we separate the series a n a_n a n from the ( − 1) n (-1)^n ( − 1) n part that makes it alternating.
What does it mean when the derivative of a series is negative?
If you can’t be sure that 0 < a n + 1 < a n 0<a_ {n+1}<a_n 0 < a n + 1 < a n just by looking at the table, you can always take the derivative of a n a_n a n to double-check. If the derivative is negative, then you know the series is decreasing, which means that a n + 1 a_ {n+1} a n + 1 will always be less than a n a_n a n .
What is the alternating series test?
In mathematical analysis, the alternating series test is the method used to prove that an alternating series with terms that decrease in absolute value is a convergent series . The test was used by Gottfried Leibniz and is sometimes known as Leibniz's test, Leibniz's rule, or the Leibniz criterion .
What is it called when all ans are positive?
where either all an are positive or all an are negative, is called an alternating series.
Why is the alternating series test applicable?
1. The alternating series test is applicable because the series is alternating: the odd terms are positive and the even terms are negative.
Which theorem gives sufficient conditions for an alternating series to converge?
A theorem that gives sufficient conditions for an alternating series to converge is the Alternating Series Test. If the terms in the series converge monotonically down to zero, then the series converges. That is basically the statement of the AST. We can also estimate to any desired accuracy the value of the alternating series by applying the Alternating Series Estimation Theorem.
What happens to an object when conditions 1 and 2 are satisfied in the AST?
As long as conditions 1 and 2 are satisfied in the AST, the alternating series converges, and that object will slow down and settle into a fixed point.
What does it mean when a series converges?
If the series converges, it would mean that our balance settles in to a fixed number. Is this ideal for an investor? Not quite, but it could be worse if the series diverges. Let's first show that this series converges by the AST.
What happens after an odd number of movements?
That is after an odd number of movements, the object is at one meter (from the starting point), and after an even number of movements, it's back at its starting point. This is an example of an object that never settles into any particular spot along the one-dimensional line. Instead, it keeps bouncing back and forth from x = 0 and x = 1 along the one-dimensional axis.
What happens to the series of objects by the AST?
So by the AST, the series (2) converges, and the object's position settles in to a fixed point after all .
When is the last inequality true?
The last inequality is clearly true when n = 1, 2, 3,, ....., so (3) is valid for all n. Thus, condition (1) of the AST is satisfied. It remains to check condition (2) of the AST for this series. Observe that for all n,
