Modus tollens is a deductive argument form and a rule of inference used to make conclusions of arguments and sets of arguments. Modus tollens argues that if P is true then Q is also true. However, P is false. Therefore Q is also false. Modus tollens as an inference rule dates back to late antiquity where it was taught as part of Aristotelian logic.
What is modus tollens in math?
Modus Tollens In A Nutshell Modus tollens is a deductive argument form and a rule of inference used to make conclusions of arguments and sets of arguments. Modus tollens argues that if P is true then Q is also true. However, P is false. Therefore Q is also false.
What is the inference rule modus tollens?
It is an application of the general truth that if a statement is true, then so is its contrapositive. The form shows that inference from P implies Q to the negation of Q implies the negation of P is a valid argument. The history of the inference rule modus tollens goes back to antiquity.
What is an example of a modus tollens fallacy?
Example of modus tollens fallacy sentence: The modus tollens fallacyis a formal argument that concludes with the denial of the consequent. This fallacy takes place when someone assumes that: Premise 1: if A implies B Premise 2: not-B is observed. Conclusion: then A must be false.
What is the modus tollens rule for transposition?
Every use of modus tollens can be converted to a use of modus ponens and one use of transposition to the premise which is a material implication. For example: Likewise, every use of modus ponens can be converted to a use of modus tollens and transposition. The modus tollens rule can be stated formally as: stands for the statement "P implies Q".
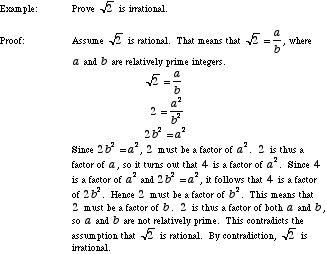
What is the example of modus tollens?
Modus PonensModus TollensIt is bright and sunny today.I will not wear my sunglasses.Therefore, I will wear my sunglasses.Therefore, it is not bright and sunny today.
What is an example of modus tollens in argument form?
This form of argument is called modus tollens (the mode that denies). E.g. All fish have scales. This salmon is a fish. Therefore, this salmon has scales.
How do I identify modus tollens?
0:001:49Modus Tollens - YouTubeYouTubeStart of suggested clipEnd of suggested clipExample if your king is in checkmate than you've lost the game. You have not lost the game thereforeMoreExample if your king is in checkmate than you've lost the game. You have not lost the game therefore your king is not in checkmate. Sounds reasonable you put in anything for a and B it.
What is the argument form for modus ponens?
The form of a modus ponens argument resembles a syllogism, with two premises and a conclusion: If P, then Q. P. Therefore, Q.
What are modus ponens and modus tollens examples?
ExamplesIt is a car. Therefore, it has wheels." ( Modus Ponens - CORRECT)It does not have wheels. Therefore, it is not a car." ( Modus Tollens - CORRECT)It has wheels. Therefore, it is a car." (Affirming the Consequent - INCORRECT.) ... It is not a car. Therefore, it does not have wheels." (
Is modus tollens a sound argument?
Any argument of this form is valid. But not every argument of this form is sound. For an argument to be sound, it must meet two requirements. First, it must be valid; second, it must have true premises.
What is universal modus tollens?
Universal Modus Tollens Sometimes one of the easiest methods to prove or disprove an argument is proof by contradiction - showing an argument is invalid by finding an example whereby the argument produces a contradiction.
Can a modus tollens argument have false premises?
FALSE. A valid argument can have false premises; and it can have a false conclusion. But if a valid argument has all true premises, then it must have a true conclusion.
Can a modus tollens argument have false premises and a true conclusion?
A valid argument can have false premises; and it can have a false conclusion. But if a valid argument has all true premises, then it must have a true conclusion.
How do modus ponens and modus tollens arguments differ?
6:158:44Logical Arguments - Modus Ponens & Modus Tollens - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo I think yes I think this is logically valid that modus ponens does indeed work and the intuitionMoreSo I think yes I think this is logically valid that modus ponens does indeed work and the intuition is proven by our truth. Table. Another very famous. And important a logical argument it is a form
What is modus ponens and modus tollens rule in fuzzy logic?
Modus ponens refers to inferences of the form A ⊃ B; A, therefore B. Modus tollens refers to inferences of the form A ⊃ B; ∼B, therefore, ∼A (∼ signifies “not”). An example of modus tollens is the following: Related Topics: hypothetical syllogism. See all related content →
What is the form of a modus tollens argument?
The form of a modus tollens argument resembles a syllogism, with two premises and a conclusion:
Who was the first to describe the argument form modus tollens?
The history of the inference rule modus tollens goes back to antiquity. The first to explicitly describe the argument form modus tollens was Theophrastus .
What is a modus tollen?
In propositional logic, modus tollens ( / ˈmoʊdəs ˈtɒlɛnz /) ( MT ), also known as modus tollendo tollens ( Latin for "method of removing by taking away" or, alternatively, "mode that by denying, denies") and denying the consequent, is a deductive argument form and a rule of inference.
Can modus tollens be converted to modus ponens?
Every use of modus tollens can be converted to a use of modus ponens and one use of transposition to the premise which is a material implication. For example:
What does "modus tollens" mean?
Modus tollens is a Latin term meaning "mode that denies by denying" or denying the consequent and is a valid argument form / rule of inference . Its logic is that if a statement is true, then so is its contra-positive (opposite). It can be stated formally as: P->Q,¬Q ∴ ¬P.
Why is modus tollens important in A/B testing?
The relevance of modus tollens logic in A/B testing is due to our goal of extracting as much as we can about a metric of interest from a particular test, including the relevant error bounds which serve as risk management devices and estimation uncertainty measurements which we can then present to stakeholders to assist in their decision-making process. Employing this argument shifts the burden of proof on whoever is willing to argue for the null hypothesis and thus gives data a central place.
What is the modus tollens?
The modus tollens is a formal logical argument that occurs when one attempts to draw an inference from two premises. The second premise, which denies the antecedent, must be true for the argument’s conclusion to follow logically. It is a type of logical fallacy that occurs when one reason in the following way: If A, then B. Not-B. Therefore, not-A
What does "modus ponens" mean?
The term “ modus ponens ” comes from Latin: it translates to “ Putting the limit .” or ‘modus’ meaning ‘way,’ ‘ponens’ meaning ‘to put,’ Thus, the name of this fallacy is mistake or error or misleading; one should not affirm anything when committing this error.
What is the logical fallacy of Modus Ponens?
Modus Ponens is a logical fallacy in which the conclusion of an argument follows from premises that are assumed to be true. It is a form of deductive reasoning, meaning that if the premise and the conclusion are both true, then it must be valid.
Why is the Modus Ponens fallacy committed?
This argument commits the modus ponens fallacy because it assumes that all men are mortal without providing any evidence for this claim.
What does "tollens" mean in Latin?
The name of the fallacy comes from Latin, where “ modus ” means method or way and “ tollens ” means denying. Thus the meaning of the phrase roughly translates as To deny by using a certain method.
What happens if A, then B, and A is true?
if A, then B, and A is true, therefore B must be true.
Is Modus Ponens a valid argument?
Modus Ponens is a valid form of argument, but it is also very commonly misused and misunderstood. It can be used to make an invalid syllogism.
What is a modus tollen?
In much the same way as modus ponens, modus tollens is a means of inferring a conclusion based on a conditional. Modus tollens essentially states, “if you have the first thing, then you also have the second thing. You do not have the second thing, so you do not have the first thing since you always have the second thing when you do have the first thing.” Here’s a simple example of modus tollens in action:
How does modus tollens work?
Modus tollens only works when the consequent (Q) follows from the antecedent (P) and the consequent (Q) is not present, which ensures that the antecedent (P) is also not present. In exactly the same way as modus ponens, modus tollens requires precisely consistent terms throughout the argument to maintain validity.
Why are modus ponens so powerful?
They are powerful because they are deductively valid, meaning (i) the premises contain all of the information necessary to determine the conclusion, and (ii) the conclusion absolutely follows from the premises. In short, modus ponens and modus tollens both provide argument forms that guarantee a true conclusion if the premises are true.
When citing modus ponens or modus tollens properly, what is the difference between true premises?
In other words, when citing modus ponens or modus tollens properly, true premises will never lead to a false conclusion. Both modus ponens and modus tollens require one premise to be in the form of a conditional. A conditional is simply an if-then statement, e.g. if I am human, then I am mortal. The “if” portion of the conditional is called ...
Is modus ponens valid?
In conclusion, both modus ponens and modus tollens are powerful, deductively valid argument forms, meaning they ensure that an argument’s conclusion follows from its premises; however, both fail to maintain their power through validity and quickly become fallacious if (i) their strict form is not upheld or (ii) the terms (P or Q) do not remain consistent throughout the argument. Additionally, care must be taken when placing the ‘not’ negation to ensure that the meaning of a proposition does not change.
What is a modus tollens?
In propositional logic, modus tollens ( /ˈmoʊdəs ˈtɒlɛnz/) (MT), also known as modus tollendo tollens ( Latin for "mode that by denying denies") and denying the consequent, is a deductive argument form and a rule of inference. Modus tollens takes the form of "If P, then Q. Not Q. Therefore, not P." It is an application of the general truth that if a statement is true, then so is its contrapositive. The form shows that inference from P implies Q to the negation of Q implies the negation of P is a valid argument.
What is the significance of modus ponens?
The significance of modus ponens is that it captures half of the meaning of implication, the elimination half.
What does "modus" mean in the dictionary?
FIRST WORD MODUS IS A NOUN AND IT'S PLURAL. MODUS COMES FROM THE WORD MODULATE, WHICH ESSENTIALLY MEANS, TO KEEP GOING UNTIL YOU ARE WHERE YOU SET OUT TO BE. IT SAYS TO HAVE AND END IN MIND. IT ALSO SAYS TO HAVE A CONTINUED PURPOSE (WHICH IS WHY MODULATE, GIVES YOU TEMPER ( TOWIT TEMPERAMENT). WHEN YOU LOOK AT MODUS, IT SAYS A METHOD OF PROCEDURE. PROCEDURE GIVES YOU PROCEED, AND THEY BOTH SAY TO KEEP GOING, THERE'S A SERIES, IT'S GRADUAL (AFTER WHILE YOU TEMPER). THESE WORDS ARE ALL POSITIVE PARTICLES. NEXT WORD IS TOLLENS, COMES FROM THE WORD AS WE KNOW AS TOLERATE. WHICH MEANS, WE KNOW THERE'S AN END, BUT WE SHOULDN'T LOOK FORWARD TO IT. IT SAYS THAT SOMETHINGS DONT NEED BOTHERING. THESE WORDS TOLLENS AND TOLERATE ARE BOTH POSITIVE PARTICLES, AND SAYS STOP STRESSING AND RELAX. PHILOSOPHY IS FREEWILL EMBODIED, WHICH SHOWS THERE'S NO LIMITS TO PHILOSOPHY, BUT IT'S GIVEN ONE.
What happens if a card has a vowel on one side?
If a card has a vowel on one side, it has an even number on its other side.
Why do we use problems?
We sometimes use problems that seem to be about the real world—we do this to make the problems more interesting and relevant, and to give us some insight into what the symbols mean. But the problems, ultimately are not in the real world—they are about a mathematical model of the real world, where we make a lot of simplifying assumptions. For example, the hard and fast absolute statement:
Who said "What the Tortoise said to Achilles"?
As simple and obvious as modus ponens is, Lewis Carroll had fun with it in his 1895 article "What the Tortoise said to Achilles". Page on platonicrealms.com
Is an argument valid if one of the premises is false?
Note that an argument can be valid, even if one of the premises is false. For example, the argument above doesn't say whether you do or don't have a current password.Maybe you do, and maybe you don't . But either way, the argument is still valid.

Overview
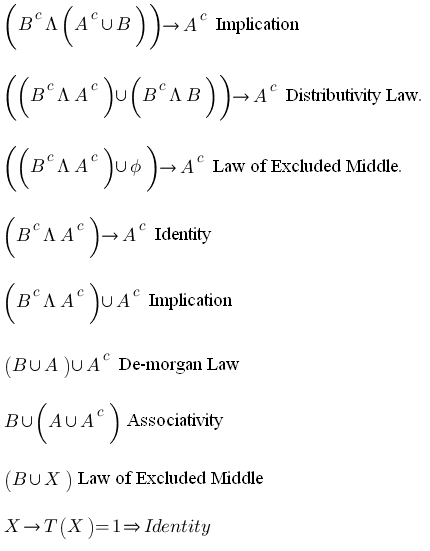
In propositional logic, modus tollens (MT), also known as modus tollendo tollens (Latin for "method of removing by taking away") and denying the consequent, is a deductive argument form and a rule of inference. Modus tollens takes the form of "If P, then Q. Not Q. Therefore, not P." It is an application of the general truth that if a statement is true, then so is its contrapositive. The form shows that inference from P implies Q to the negation of Q implies the negation of P is a valid arg…
Explanation
The form of a modus tollens argument resembles a syllogism, with two premises and a conclusion:
If P, then Q. Not Q. Therefore, not P.
The first premise is a conditional ("if-then") claim, such as P implies Q. The second premise is an assertion that Q, the consequent of the conditional claim, is not the case. From these two premis…
Relation to modus ponens
Every use of modus tollens can be converted to a use of modus ponens and one use of transposition to the premise which is a material implication. For example:
If P, then Q. (premise – material implication) If not Q, then not P. (derived by transposition) Not Q . (premise) Therefore, not P. (derived by modus ponens)
Likewise, every use of modus ponens can be converted to a use of modus tollens and transposit…
Formal notation
The modus tollens rule can be stated formally as:
where stands for the statement "P implies Q". stands for "it is not the case that Q" (or in brief "not Q"). Then, whenever "" and "" each appear by themselves as a line of a proof, then "" can validly be placed on a subsequent line.
The modus tollens rule may be written in sequent notation:
Correspondence to other mathematical frameworks
Modus tollens represents an instance of the law of total probability combined with Bayes' theorem expressed as:
,
where the conditionals and are obtained with (the extended form of) Bayes' theorem expressed as:
and .
See also
• Evidence of absence – Relevance fallacy
• Latin phrases
• Modus operandi – The habits of working
• Modus ponens – Rule of logical inference
Sources
• Audun Jøsang, 2016, Subjective Logic; A formalism for Reasoning Under Uncertainty Springer, Cham, ISBN 978-3-319-42337-1
External links
• Modus Tollens at Wolfram MathWorld