What is example of visual representation?
An image is a visual representation of something that depicts or records visual perception. For example, a picture is similar in appearance to some subject, which provides a depiction of a physical object or a person.
What is visual representation?
Visual representation is mainly the direct or symbolic reflection of something in the format of photos, the images, memes, graphics to represent people, things, a place, or a situation.
What are the four examples of visual representation?
The types of visual representations occur in six types: graphs, tables, maps, diagrams, networks and icons. The variations on these types can also be classified according to the way they convey information and the efficiency in which they provide information.
What is the meaning of representation in mathematics?
In mathematics, a representation is a very general relationship that expresses similarities (or equivalences) between mathematical objects or structures.
What's another word for visual representation?
What is another word for visual representation?representationgraphoutlineillustrationgraphicplotgraphic representationvisual aidscatter diagramblueprint45 more rows
What is an example of representation?
Representation is the act of speaking on someone's behalf, or depicting or portraying something. When a lawyer acts on behalf of a client, this is an example of representation. When you make a drawing of your mother that is meant to look like her, this is an example of a representation of your mother.
Why are visual representations important in math?
Teaching students to systematically use a visual representation to solve word problems has led to substantial improvements in math achievement for students with learning disabilities. Students who use visual representations to solve word problems are more likely to solve the problems accurately.
What is the importance of visual representations of ideas in studying mathematics?
Visual representation often helps in understanding a problem. Using visual representations leads to a better understanding and to improving special mathematical reasoning. The present paper presents an experiment involving university students who have been asked to solve a logical problem.
What is the important of a visual representation?
Visual representation helps us better understand quantitative data by using visual elements such as charts, graphs, colors, etc. This is because our brains can process visual information more easily.
What are examples of mathematical representations?
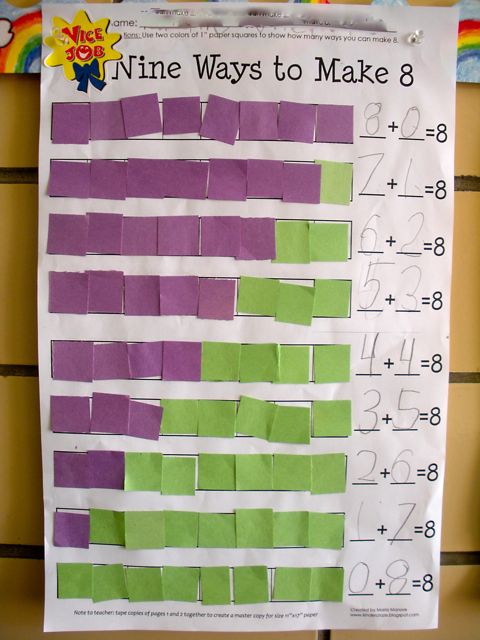
Examples of such conventional mathematical representations include base ten numerals, abaci, number lines, Cartesian graphs, and algebraic equations written using standard notation.
What are some ways to represent a problem in math?
A tape diagram is another way to represent information in a word problem. We are learning to use tape diagrams to solve problems that involve both multiplication and division. A tape diagram starts with a rectangle. The students must label the tape diagram using information from the problem.
Which different types of representations can be used in problem solving?
Commonly used representations are tables, strip diagrams, percent bars, and number lines. Strip diagrams are especially useful for comparisons; tables are helpful for ratio and proportion problems.
What is visual representation in art?
The term "representation" suggests a type of description or portrayal of someone or something. In the visual arts this implies that the art object depicts something other than or outside itself. In some cases the mode of representation is iconic and relies on ideas or symbols.
Why is visual representation important?
Visual representation helps us better understand quantitative data by using visual elements such as charts, graphs, colors, etc. This is because our brains can process visual information more easily.
What is visual representation process?
Visual representations range from simple maps and schematic diagrams to computer generated 2D and 3D visualizations. They differ themselves from other kinds of representations, e.g., sentential representations, in the fact that they make use of visual variables to convey the information to the user.
What is visual representation in art and design?
Visual representation in design is viewed here as a transaction between conceptual and visual knowledge, which enables the designer to immediately control, promote or evaluate specific characteristics of the design in progress.
Why is visual representation important in math?
Using visual representations helps give concrete structure to abstract concepts and, therefore, is a beneficial practice in math instruction for all students, particularly those with autism.
Can autistic students learn math?
Students with autism are, generally, concrete thinkers, which can make teaching math skills difficult. As math work becomes more abstract, how can educators help these students build problem solving and application skills? Jo Boaler, Professor of Mathematics Education at Stanford University, reports that brain evidence supports the use of visual approaches in mathematics and explains that when these approaches are used “mathematics changes for them, and they are given access to deep and new understandings” (Boaler, Chen, Williams, & Cordero, 2016)
Why is visual representation important in math?
Teaching students to systematically use a visual representation to solve word problems has led to substantial improvements in math achievement for students with learning disabilities. Students who use visual representations to solve word problems are more likely to solve the problems accurately.
Why do students use visual representations?
Students who use visual representations to solve word problems are more likely to solve the problems accurately . This was equally true for students who had LD, were low-achieving, or were average-achieving. Visual representations are flexible; they can be used across grade levels and types of math problems.
What is concrete representational abstract?
The Concrete-Representational-Abstract (CRA) framework helps students gain a conceptual understanding of a mathematical process, rather than just completing the algorithm (e.g., 2 + 4, 2x + y = 27). Systematically connecting concrete objects or visual representations to the abstract equation is a way to scaffold a student’s understanding. The components of the framework are: 1 Concrete —Students interact and manipulate three-dimensional objects, for example algebra tiles or other algebra manipulatives with representations of variables and units. 2 Representational — Students use two-dimensional drawings to represent problems. These pictures may be presented to them by the teacher, or through the curriculum used in the class, or students may draw their own representation of the problem. 3 Abstract — Students solve problems with numbers, symbols, and words without any concrete or representational assistance.
How do manipulatives help students?
The use of manipulatives really helps students see that conceptually, and it clicks a little more with them. Some of the things, though, that we need to remember when we’re using manipulatives is that it is important to give students a little bit of free time when you’re using a new manipulative so that they can just explore with them. We need to have specific rules for how to use manipulatives, that they aren’t toys, that they really are learning materials, and how students pick them up, how they put them away, the right time to use them, and making sure that they’re not distracters while we’re actually doing the presentation part of the lesson. One of the important things is that we don’t want students to memorize the algorithm or the procedures while they’re using the manipulatives. It really is just to help them understand conceptually. That doesn’t mean that kids are automatically going to understand conceptually or be able to make that bridge between using the concrete manipulatives into them being able to solve the problems. For some kids, it is difficult to use the manipulatives. That’s not how they learn, and so we don’t want to force kids to have to use manipulatives if it’s not something that is helpful for them. So we have to remember that manipulatives are one way to think about teaching math.
How to move students with disabilities to the abstract equation?
One promising practice for moving secondary students with mathematics difficulties or disabilities from the use of manipulatives and visual representations to the abstract equation quickly is the CRA-I strategy. In this modified version of CRA, the teacher simultaneously presents the content using concrete objects, visual representations of the concrete objects, and the abstract equation. Studies have shown that this framework is effective for teaching algebra to this population of students (Strickland & Maccini, 2012; Strickland & Maccini, 2013; Strickland, 2017).
Why don't teachers use manipulatives?
It is true we can’t walk around life with manipulatives in our hands. And I think one of the other reasons that a lot of schools or teachers don’t use manipulatives is because they’re very expensive. And so it’s very helpful if all of the teachers in the school can pool resources and have a manipulative room where teachers can go check out manipulatives so that it’s not so expensive. Teachers have to know how to use them, and that takes a lot of practice.
What is schematic representation?
More than simply a picture or detailed illustration, a visual representation—often referred to as a schematic representation or schematic diagram— is an accurate depiction of a given problem’s mathematical quantities and relationships.
What is Visual Mathematics?
Through decades of work with students, teachers, high-tech companies, politicians and others we have learned that people are excited and inspired when they see mathematics as pictures, not just symbols. For example, consider how you might solve 18 x 5, and ask others how they would solve 18 x 5. Here are some different visual solutions of this problem.
Why are visuals important in math?
Each of these visuals highlights the mathematics inside the problem and helps students develop understanding of multiplication. Pictures help students see mathematical ideas, which aids understanding. Visual mathematics also facilitates higher-level thinking, enables communication and helps people see the creativity in mathematics.
Why is visual math important?
Visual mathematics also facilitates higher-level thinking, enables communication and helps people see the creativity in mathematics. Mathematics is a subject that allows for precise thinking, but when that precise thinking is combined with creativity, openness, visualization, and flexibility, the mathematics comes alive.
How can teachers create mathematical excitement in classrooms?
Teachers can create such mathematical excitement in classrooms with any mathematics question by asking students for the different ways they see and can solve the problems and by encouraging discussion of different ways of seeing problems. For an example of visualizing algebra see here.
Does visual training improve math?
Additionally, they found that training students through visual representations improved students’ math performance significantly, even on numerical math, and that the visual training helped students more than numerical training.
Is visual math only needed for abstract math?
Despite the importance of visual mathematics at high levels of mathematics (and all other levels) there is a common perception that visual mathematics is only needed as a crutch for more abstract mathematics.
Why do we use representations in math?
In mathematical terms, we use the word representations as one way of labelling the many methods and strategies we may encounter. This definition of representations may be useful in understanding this further:
How can a mathematical problem be represented?
Put simply, a mathematical problem can be represented using concrete or physical materials, the problem can then be represented using a diagram or picture and the same problem can be represented in the abstract, using symbolic notation:
What is mastery in math?
The goal of maths mastery is for learners to get to a point where they no longer have to attend to certain functions as they solve a problem. In this example, we would want the children to just know that 28 can be broken into smaller parts or just know that 6 x 8 is 48. That way, they can concentrate on new ideas that are associated (in this case, with multiplying a 2-digit number by another 2-digit number).
Why is the use of language, written words and reading maths important?
The use of language, written words and reading maths is essential to a learner’s understanding. This idea is continually being developed and built upon. Revisiting these ideas again and again within a spiral curriculum is a key component of a maths mastery approach.
Why is variation important in representations?
Then, any variation in the representations acts as a support and challenge in a learner’s development of new mathematical ideas.
What is the first representation of the theatre?
The first representation is pictorial. It shows the array of seats in the theatre and connects this to a multiplication strategy using number bonds to break 28 into 10, 10 and 8, and 26 into 10, 10 and 6.
Does the next representation you see have pictorial support?
The next representation you see doesn’t have pictorial support . But because the previous example did, the link between the pictorial representation and the abstract representation has been provided. To further develop this link you can ask your learners:
Teaching Abstract Concepts to Concrete Thinkers
Seeing Is Understanding
- Using visual representations helps give concrete structure to abstract concepts and, therefore, is a beneficial practice in math instruction for all students, particularly those with autism. Research has shown that students who use concrete materials and visual representations develop more mathematical understandings and better apply these ideas to...
to Learn More
- Information on how to carry out the use of visual representations, as well as solutions to potential roadblocks, is available in the Institute of Education Science’s practice guide, Assisting Students Struggling with Mathematics: Response to Intervention (RtI) for Elementary and Middle Schools.
References
- Boaler, J., Chen, L., Williams, C., & Cordero, M. (2016). Seeing as Understanding: The Importance of Visual Mathematics for our Brain and Learning. Journal of Applied & Computational Mathematics,05(05). Fuchs, L. S., Powell, S. R., Hamlett, C. L., Fuchs, D., Cirino, P. T., & Fletcher, J. M. (2008). Remediating Computational Deficits at Third Grade: A Randomized Field Trial. Journa…