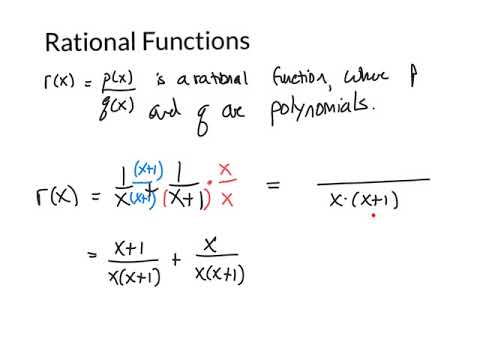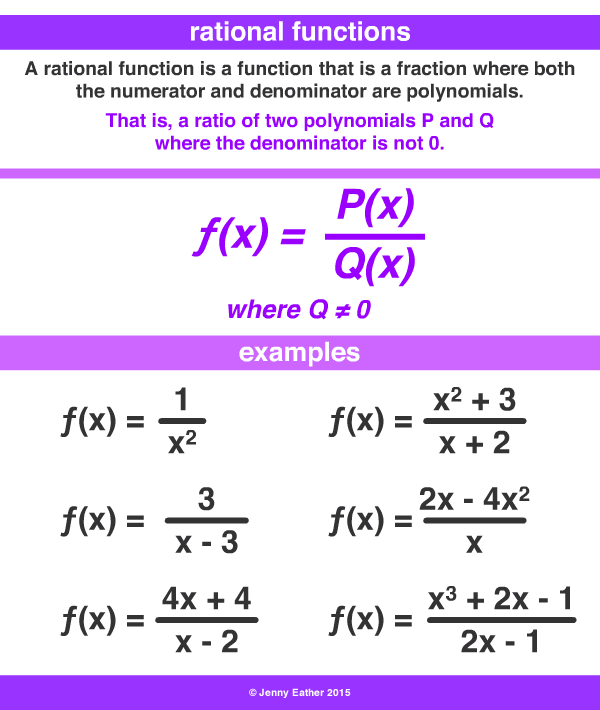
Below are some examples of rational functions:
- f ( x) = x 2 − 1 2 x + 1
- g ( x) = x 2 – 3 x + 2 x 2 – 4
- h ( x) = ( x + 1) 3 x + 5
Full Answer
How do you make a rational function?
A rational function is any function which can be written as the ratio of two polynomial functions, where the polynomial in the denominator is not equal to zero.Domain restrictions of a rational function can be determined by setting the denominator equal to zero and solving.
What is the standard form of a rational function?
Standard Notation The typical rational function has the form p(x)/q(x) where p and q are polynomials. p(x) is called the numerator and q(x) is called the denominator. the numerator is x 2 – 4 and the denominator is x 2 2 – 5x + 6. A polynomial is a rational functions with denominator 1.
What does a rational function look like?
What does a rational function look like? Here is a sketch of this graph. First, notice that the graph is in two pieces. Almost all rational functions will have graphs in multiple pieces like this. A rational function will be zero at a particular value of x only if the numerator is zero at that x and the denominator isn't zero at that x .
How to evaluate rational functions?
How to Evaluate a Rational Function: Example 1. Evaluate f (3) for {eq}f (x)=dfrac {x+2} {3x-8} {/eq} Step 1: Determine your input value for your function, this will be the value inside your ...

What is an example of rational function?
Examples of Rational Functions The function R(x) = (x^2 + 4x - 1) / (3x^2 - 9x + 2) is a rational function since the numerator, x^2 + 4x - 1, is a polynomial and the denominator, 3x^2 - 9x + 2 is also a polynomial.
What is considered a rational function?
A rational function is one that can be written as a polynomial divided by a polynomial. Since polynomials are defined everywhere, the domain of a rational function is the set of all numbers except the zeros of the denominator. Example 1. f(x) = x / (x - 3). The denominator has only one zero, x = 3.
How do you know if a function is rational?
A rational function is defined as the quotient of polynomials in which the denominator has a degree of at least 1 . In other words, there must be a variable in the denominator. The general form of a rational function is p(x)q(x) , where p(x) and q(x) are polynomials and q(x)≠0 .
What are the 3 rational functions?
Rational function is the ratio of two polynomial functions where the denominator polynomial is not equal to zero....Rational functions can have 3 types of asymptotes:Horizontal Asymptotes.Vertical Asymptotes.Oblique Asymptote.
What are 5 examples of rational equation?
Solving Rational Equations4/x + 5/2 = -11/x.5x/(x - 2) = 7 + 10/(x - 2)(3x - 2)/(x - 2) = 6/(x2 - 4) + 1.2/(x2 - x) = 1/(x - 1)3/(x + 2) = 6/(x - 1)
Why is it called a rational function?
A function that is the ratio of two polynomials. It is "Rational" because one is divided by the other, like a ratio.
What is not rational function?
You should know the following non-rational functions: Square root functions. Trigonometric functions. Exponential functions. Logarithmic functions.
How do you determine if an equation is rational or irrational?
What are rational and irrational numbers? Rational numbers are the numbers that can be expressed in the form of a ratio (i.e., P/Q and Q≠0) and irrational numbers cannot be expressed as a fraction. But both the numbers are real numbers and can be represented in a number line.
What are the 5 examples of rational inequality?
A rational inequality is an inequality that contains a rational expression. Inequalities such as 3 2 x > 1 , 2 x x − 3 < 4 , 2 x − 3 x − 6 ≥ x , 3 2 x > 1 , 2 x x − 3 < 4 , 2 x − 3 x − 6 ≥ x , and 1 4 − 2 x 2 ≤ 3 x 1 4 − 2 x 2 ≤ 3 x are rational inequalities as they each contain a rational expression.
What are the two functions used to form rational function?
A rational function is a function that is the quotient of two polynomial functions. Two basic rational functions are the reciprocal function, f(x)=1x, and the squared reciprocal function, g(x)=1x2. For both of these basic functions, the domain is all real numbers such that x≠0.
What is rational equation in math?
A rational equation is an equation containing at least one fraction whose numerator and denominator are polynomials, \frac{P(x)}{Q(x)}. Q(x)P(x). These fractions may be on one or both sides of the equation.
What are the differences of rational function and equation?
A rational expression is nothing more than a fraction in which the numerator and/or the denominator are polynomials. A rational equation is an equation containing at least one rational expression.
What is a simple rational function?
Rational functions are created by dividing polynomials by one another. A simple example of a rational function is the function: f(x) = 1/x
How do you write a rational function?
Rational functions are quotients of polynomial functions. The general way to write a quotient is as a fraction: to write one function (the numerato...
How do you know if a function is rational or not?
A rational function is the quotient of two polynomial functions. Any function that cannot be expressed in terms of polynomials is not a rational fu...
Why is it called rational function?
A rational function is an analog term of "rational number." Just as a rational number is a ratio (or fraction) of two simpler numbers (integers), a...
What is the formula of rational function?
Rational functions are a broad class of functions with no universal formula. However, all rational functions are quotients of polynomial functions,...
What is rational function?
A rational function is simply the ratio of polynomials. Any function of one variable, x, is called a rational function if, it can be represented as the following rational function formula: where p p and q q are polynomial functions of x x and q (x) ≠ 0 q (x) ≠ 0 .
What is the largest exponent in the numerator and denominator?
Now, the largest exponent in the numerator and denominator is 1 1 and so by the fact, there will be a horizontal asymptote at the line.
What are the properties of rational functions?
First off, we should probably define a vertical asymptote. A vertical asymptote at a value x is when the value of our function approaches either positive or negative infinity when we evaluate our function at values that approach x (but are not equal to x ).
What is the result of a common factor in the denominator?
If there are more instances of the common factor in the denominator, the result is a vertical asymptote.
Did we use the numerator?
You might be thinking. . . Wait, we didn't use the numerator! Were we supposed to? Well, we technically did use the numerator since we had to make sure there were no common factors between the numerator and denominator. But, when it comes to actually computing our vertical asymptotes, we only use the denominator!
Can we use the theorem of common factor?
We can't use the theorem since there is a common factor in both the numerator and the denominator.
Is R a rational function?
The function R ( x) = 1 / ( ( x - 1) ( x ^2 + 3)) is a rational function since the numerator , 1, is a polynomial (yes, a constant is still a polynomial) and the denominator, ( x - 1) ( x ^2 + 3), is also a polynomial (it's just in a factored form).
What Is a Rational Function?
A rational function is a quotient of two polynomial functions. Thus, to define a rational function, it is necessary to understand what a polynomial function is. A polynomial function is any function that can be written in the form
When is a rational function obtained?
where each {eq}a_i {/eq} is a real number. A rational function is obtained when one polynomial function is divided by another.
What happens if there is no common factor in a rational function?
But what if there are common factors between the numerator and denominator of a rational function? If a rational function has a common factor between the numerator and denominator - and the factor occurs more times in the numerator or exactly the same amount of times in the numerator and denominator, then the result is a hole in the graph where the factor equals zero. Solving where the factor equals zero will give the x coordinate of a hole and substituting this value into the rational function after all common factors have been "cancelled" will give the y coordinate of a hole. For example, the rational function R (x) = ( (x+1) (x-1))/ (x-1) has a common factor of x-1 in the numerator and denominator. x-1 =0 when x=1, so we have a hole at x=1. If we were to cancel the common factors, R (x) would look like R (x)=x+1. Substituting x=1 into the simplified version gives a y coordinate of 2. So we have a hole at the point (1,2)
How to find asymptotes of fractions?
In order to find asymptotes, first begin by factoring both sides of the fraction and canceling common factors ; these canceled factors will give rise to holes, as mentioned above. Then, inspect the reduced denominator. Any values of {eq}x {/eq} that cause this denominator to equal zero produce vertical asymptotes at that {eq}x {/eq}-value, instead of holes.
What is the result of a common factor in the denominator?
If there are more instances of the common factor in the denominator, the result is a vertical asymptote.
Do rational functions have asymptotes?
In addition, rational functions often (but not always!) have asymptotes. These will be revisited in a later section.
What is rational quotient?
It is the quotient or ratio of two integers, where the denominator is not equal to zero. Hence, the name rational is derived from the word ratio.
How many asymptotes can rational functions have?
Rational functions can have 3 types of asymptotes:
What happens when Q (x) = 1?
When Q (x) = 1, i.e. a constant polynomial function, the rational function becomes a polynomial function.
What Is A Rational Function?
A rational function is a fraction where the numerator and denominator are both polynomials. A rational function R (x) has the following form:
How many asymptotes does a rational function have?
A rational function cannot have two horizontal asymptotes. A rational function R (x) = P (x) / Q (x) has only one horizontal asymptote:
When can rational functions have no vertical asymptotes?
A rational function can have no vertical asymptotes when Q (x) has only imaginary or complex (not real) zeros.
What is the function of R if Q = 1?
If Q (x) = 1, then R (x) would equal P (x). In that case, R (x) is a polynomial function with coefficients in the same field as P (x).
What is the exponent of every term in P and Q?
So P (x) = x 2 + 4 and Q (x) = x 2 – 4. In this case, the exponent of every term in P (x) and Q (x) is even (either 2 or 0).
Is the graph of the rational function R (x) = 1 / x 2 (blue curves?
The graph of the rational function R (x) = 1 / x 2 ( blue curves) is not one-to-one, since the horizontal line y = 1 intersects it twice, at x = 1 and x = -1.
Can a rational function have even symmetry?
A rational function can have even symmetry, odd symmetry, or neither, depending on the exponents in the numerator and denominator polynomials.
How to find holes in a rational function?
Now that we understand the importance of understanding what the holes of rational functions represent, it’s time that we learn how to determine holes that a function may have.
What do holes represent?
What do these holes represent? These are the values or the coordinates that a function’s graph may pass through but are not defined by the function, hence, the unfilled dots.
Can a function pass through holes?
Here’s the graph of f ( x) along its holes and asymptotes. This confirms that the function’s curve can pass through these holes, but these values will not be part of the function’s domain and range.