BOUSSINESQ THEORY Boussinesq (1885) developed a theory to calculate stresses and deformation due to point load applied at the surface of semi infinite soil mass. Assumptions: 1. Soil mass is elastic continuum having a constant value of modulus of elasticity (E) i.e it obeys Hook’s law.
What are the assumptions of Boussinesq's theory formula?
Boussinesq's theory formula is based on following assumptions. The soil mass is semi-infinite, homogeneous and isotropic. The soil is weightless.
What is Boussinesq theory in civil engineering?
1. Boussinesq Theory for Vertical Stress due to Concentrated Load: Boussinesq in 1885 gave a solution for stress distribution in a homogeneous and isotropic subgrade subjected to a vertical concentrated load (point load) on the ground surface.
What did Boussinesq do?
In 1885, Boussinesq published equations to determine the state of stress in a subgrade material. He investigated the stresses in an elastic, semi-infinite, homogeneous, and isotropic soil solid medium. The assumed solid medium is loaded normally on its upper plane surface by a concentrated point load.
What is the vertical stress in Boussinesq theory?
The vertical stress computed by the Boussinesq theory for a point load is infinite when z = 0, that is, at the ground surface, where the load is applied. a. The soil is homogeneous and isotropic. b. The soil mass is semi-infinite; that is, it extends infinitely in all directions below a level surface.
What are the three assumptions of Boussinesq theory?
The soil mass is semi-infinite, homogeneous and isotropic. The soil has a linear stress-strain relationship, The soil is weightless. The load is a point load acting on the surface.
What is Boussinesq equation used for?
In coastal engineering, Boussinesq-type equations are frequently used in computer models for the simulation of water waves in shallow seas and harbours.
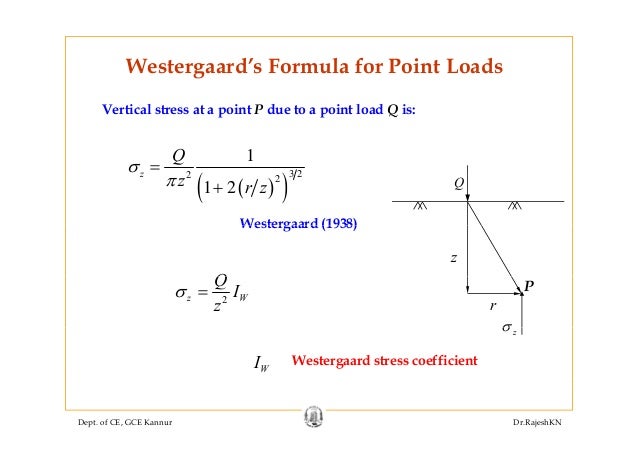
What is Westergaard theory?
Explanation: Westergaard's Theory Assumptions: Elastic medium of semi-infinite extent but containing numerous, closely spaced horizontal sheets of a negligible thickness of an infinite rigid material which permits only downward deformation as a whole without allowing it to undergo any lateral strain.
What is the basic difference between Boussinesq's theory and Westergaard's theory?
Westergaard's theory:- Considers the poisson's ratio, which varies from 0 to 0.5. In comparison to Boussinesq's hypothesis, the vertically stress value produced is smaller. In comparison to Boussinesq, Westergaard has a modest influence factor.
What is r in Boussinesq equation?
Boussinesq's Formula Equation r = the horizontal distance between an arbitrary point P below the surface and the vertical axis through the point load Q. z = the vertical depth of the point P from the surface.
What is oberbeck Boussinesq approximation?
The Oberbeck-Boussinesq approximation is the most widely employed theoretical scheme for the study of natural or mixed convection flows. However, the misunderstanding of this approximated framework is a possibility that may cause the emergence of paradoxes or, at least, incorrect conclusions.
What are the assumptions of Westergaard theory?
Westergaard's Theory Assumptions: Elastic medium of semi-infinite extent but containing numerous, closely spaced horizontal sheets of a negligible thickness of an infinite rigid material which permits only downward deformation as a whole without allowing it to undergo any lateral strain.
What is Westergaard equation?
The formula is presented below. Westergaard's Equation for Point Loads. If Q is the point load and σz is the vertical stress due to the point load, σz=Q2πz2×√(1−2μ)/(2−2μ)[(1−2μ)/(2−μ)+(r/z)2)]3/2=Qz2Iw. Here, μ= Poisson's Ratio.
What is 2 1 stress distribution method?
Vertical Ratio 2:1 Method For a non-rectangular footing, the stress is calculated by computing the area of the load at the surface. With increasing depth, the area over which the load is applied increases at a 2:1 ratio and the magnitude of the loading stress decreases correspondingly.
What is Boussinesq's influence factor?
It may be noted that the Boussinesq influence factor for vertical stress is a function of the ratio r/z and is independent of soil properties. It is maximum when r = 0, that is, below the concentrated load, and is equal to 0.4775. Values of IB are computed for different values of r/z and are presented in Table 8.1.
Which theory is applicable for layered soil?
Westergaard theory considers infinitely thin elastic layers of soil.
What are the components of settlement?
The total settlement of the ground consists of 3 components: immediate settlement (commonly referred to as elastic settlement, although this is a misnomer), consolidation settlement (or primary settlement) and creep settlement (or secondary settlement).
What is Westergaard equation?
The formula is presented below. Westergaard's Equation for Point Loads. If Q is the point load and σz is the vertical stress due to the point load, σz=Q2πz2×√(1−2μ)/(2−2μ)[(1−2μ)/(2−μ)+(r/z)2)]3/2=Qz2Iw. Here, μ= Poisson's Ratio.
How do you pronounce Boussinesq?
Re: How to pronounce Boussinesq? The standard pronouciation in English (and used by everyone O know) is Boo-zin-esk.
What is buoyancy driven flow?
Buoyancy-driven convection denotes a type of the heat transfer in a fluid, in which the fluid flow is driven solely by a density difference due to a temperature gradient. Consider a two-dimensional flow within a square cavity of solid walls, where gravity is acting in the direction.
What is Reynolds stress tensor?
In fluid dynamics, the Reynolds stress is the component of the total stress tensor in a fluid obtained from the averaging operation over the Navier–Stokes equations to account for turbulent fluctuations in fluid momentum.
What is the purpose of Boussinesq equations?
Boussinesq equations ( Boussinesq, 1871, 1872) are a set of nonlinear partial differential equations. Boussinesq equations incorporate frequency dispersion while the shallow water equations are not frequency-dispersive. Boussinesq equations are widely applied in numerical modeling in the field of coastal engineering to model water waves in shallow ...
What is the Boussinesq approximation?
The Boussinesq approximation applies if the Mach number of the flow is small, propagation of sound or shock waves is not considered, the vertical scale of the flow is not too large, and the temperature differences in the fluid are small. Then the density can be treated as a constant in both the continuity and the momentum equations, except in the gravity term. Properties of the fluid such as μ, k, and cp are also assumed constant. Omitting Coriolis accelerations, the set of equations corresponding to the Boussinesq approximation is: (4.9) and/or (4.10), (4.86) with g = – gez, (4.89), and ρ = ρ0 [1 – α (T – T0 )], where the z -axis points upward. The constant ρ0 is a reference density corresponding to a reference temperature T0, which can be taken to be the mean temperature in the flow or the temperature at an appropriate boundary. Applications of the Boussinesq set can be found in several places in this book, for example, in the analysis of wave propagation in a density-stratified medium, thermal instability, turbulence in a stratified medium, and geophysical fluid dynamics.
What are the effects of Boussinesq?
The Boussinesq equations include the effects of diffraction, refraction, reflection and wave-current interaction . Wind input and dissipation can be included in simplified forms as already indicated for Mild Slope Equation models. Triad interactions [see Section (7.2.7)] are represented by the quadratic terms. The inclusion of these terms is a particular strength of this class of model and they have been particularly successful in predicting the evolution of higher harmonics during shoaling ( Beji and Battjes, 1994; Madsen et al, 1997 ).
What was Boussinesq's guess?
Boussinesq’s guess was a “try-on”; but it is now sufficiently venerable to be called a “hypothesis” and even a “model.” The other just-mentioned pioneers all adopted it; indeed, it can be said that the purpose of their endeavors was to devise a means for predicting what value should be ascribed to the effective viscosity at any point within a turbulent flow.
Is Boussinesq a three dimensional equation?
Although wave simulation in such cases is perfectly described by the Navier-Stokes equations, currently it is extremely difficult to solve the full three-dimensional equations in complicated models.
Does Boussinesq change in appearance?
Under the Boussinesq approximation, Eq. (5.49) does not change in appearance, however, the gravitational and pressure terms are redefined to include the effects of temperature variation. To this end, let ˆ ρ denote the density under hydrostatic conditions. If the y axis is aligned with the vertical direction, we can write
Which hypothesis is related to the mean velocity gradient?
Boussinesq's hypothesis is that the turbulent stresses are related to the mean velocity gradients in almost the same way that the viscous stresses are related to the complete velocity gradients.
What is the essence of Boussinesq?
The essence of the Boussinesq approximation is that the difference in inertia is negligible but gravity is sufficiently strong to make the specific weight appreciably different between the two fluids.
What is the Boussinesq approximation?
In fluid dynamics, the Boussinesq approximation ( pronounced [businɛsk], named for Joseph Valentin Boussinesq ) is used in the field of buoyancy-driven flow (also known as natural convection ).
What are some examples of non-Boussinesq flows?
An example of a non-Boussinesq flow is bubbles rising in water. The behaviour of air bubbles rising in water is very different from the behaviour of water falling in air: in the former case rising bubbles tend to form hemispherical shells, while water falling in air splits into raindrops (at small length scales surface tension enters the problem and confuses the issue).
Is Boussinesq a common flow?
Boussinesq flows are common in nature (such as atmospheric fronts, oceanic circulation, katabatic winds ), industry ( dense gas dispersion, fume cupboard ventilation), and the built environment (natural ventilation, central heating ). The approximation is extremely accurate for many such flows, and makes the mathematics and physics simpler.
What is the classical Boussinesq equation?
The classical Boussinesq equation includes the weak nonlinear and dispersive effects; it can effectively improve the situation that the shallow water equation can't directly handle the dispersion wave. Its basic assumption is similar to that of the equation of shallow water long wave; it still doesn't consider the effects of nonlinearity and bottom friction during the transoceanic spreading, only added in the calculation process during the spreading on the coast and climbing. But because the classical Boussinesq equation model is limited to the weak nonlinear interaction, and in many practical cases, the nonlinear effect is very big, weak nonlinear Boussinesq equation model is no longer effective. For example, when a tsunami wave arrives at the shoal and enters into the shallow water, and then begins to climb on the beach, the wave would be at the state of rapid evolution, and the tsunami wave at this time has similar characteristics with the long wave generated by other conditions (such as tide). Wave is becoming steep or broken before it reaches the coast, or it rushes past coastal topography and is not broken. Therefore, the classical Boussinesq equation now is mainly used in the dispersion study of transoceanic spreading. In order to optimize the classical Boussinesq equation, Wei and others at the University of Delaware, United States, improved the classical Boussinesq equation model and developed a completely nonlinear Boussinesq equation model (Yao et al., 2007 ).
Why is the Boussinesq limit a temperature equation?
The basic reason for this is that in the Boussinesq limit, the thermal energy is much larger than the kinetic energy or the magnetic energy. The heat transport equation then becomes a temperature equation, because the dominant form of energy is the temperature-dependent internal heat energy. This is rather a drawback for the Boussinesq approximation, because the ohmic dissipation might be a significant player in the overall heat budget. In consequence, the last three terms on the right-hand side of eqn [30] can be bundled into a single space-dependent heat source term
What is phase-resolving wave model?
Phase-resolving models simulate wave processes based on conservation laws (mass and momentum). They are aimed at rapidly varying waves. For instance, waves that change rapidly as a function of local topography or the presence of structures (harbours) where strong diffraction and waves-wave interaction (triads) requires detailed modelling. These models describe the sea surface as function of time and therefore resolve individual waveforms, that is, each and every single wave in the model domain. Modelling requires at least five grid points per wavelength with a very fine grid in which spacing is normally around a few metres in order to capture and resolve all relevant wavelengths. The most common time-dependent phase-resolving wave models are based on the Boussinesq equation (see Chapter 7) and known as Boussinesq models, whereas stationary phase-resolving wave models are based on the Mild-Slope Equation (MSE). The Boussinesq equation is non-linear, and it is used (mainly in research situations) to study non-linear wave effects in shallow water. The MSE on the other hand is linear. These phase-resolving models incorporate processes of shoaling, refraction, diffraction, reflection, wave generation, bottom friction, wave-current interactions, wave breaking and run-up and wave-induced currents (see Chapters 4, 6 and 7 for full description of these processes).
What are the three regions of approximation for the long wave theory?
Under the assumption that depth is small compared to a horizontal length scale, there are three regions of approximation for the long-wave theory: (a) linear equations, (b) finite-amplitude equations, (c) Boussinesq e quations (Murty, 1977; Levin and Nosov, 2009 ). Three characteristic lengths determine which equation is most appropriate: water depth, D, wave length, λ, and wave amplitude, η. It has been shown that for very long waves, which is the case of tsunamis in the deep ocean, the speed of the wave is mainly controlled by the water depth as a first approximation:
Do ocean models solve Navier Stokes equations?
Present large-scale ocean models do not solve the Navier–Stokes and thermodynamic equations, which have unwanted complexity, such as a time step limited by the speed of sound. Instead, the Boussinesq, hydrostatic, traditional, and geoid simplification approximations are typical ( Griffies and Adcroft, 2008; Young, 2010; Chapter 20) although exceptions exist (e.g., McDougall et al., 2002; Mahadevan, 2006 ). The hydrostatic approximation ∇zP = − ρg is appropriate for large-aspect-ratio flow, and the background pressure ( P0 − ρ0gz) is hydrostatic, but the hydrostatic approximation will not be made to the vertical momentum equation so that the symmetries are more apparent.
Is the Boussinesq equation model weak?
But because the classical Boussinesq equation model is limited to the weak nonlinear interaction, and in many practical cases, the nonlinear effect is very big, weak nonlinear Boussinesq equation model is no longer effective.
Why is Boussinesq's equation used?
It is simpler than the Westergaard’s equation and since the vertical stress estimates are greater from the Boussinesq’s equation, it can only overestimate the loadings within the soil, and hence be a conservative one.
Which equation is derived from Boussinesq?
Boussinesq derived the equation of vertical stress and shear stress in a soil medium.
When did Boussinesq publish his equations?
In 1885, Boussinesq published equations to determine the state of stress in a subgrade material. He investigated the stresses in an elastic, semi-infinite, homogeneous, and isotropic soil solid medium. The assumed solid medium is loaded normally on its upper plane surface by a concentrated point load.
What is 2:1 stress?
2:1 approach is used to calculate an average or mean vertical stress taken at the layer existing at depth z. This technique follows the procedure that the stresses from the loaded edge points distribute at an angle 2 vertical to 1 horizontal.
What is Effective Stress?
When the stress is transferred from grain to grain at the point of contact via a soil mass is called effective stress. It is also known as intergranular stress. It is denoted by σ’.
What is Pore Water Stress?
When the pores of soil are filled with water and the pressure-induced tries to detach the grain then it is known as pore water stress or neutral stress. It is denoted by Uw.
What is Total Stress?
It is the summation of effective stress and pore water pressure. It is denoted by σt.
What is Boussinesq theory?
Boussinesq Theory for Vertical Stress due to Concentrated Load: Boussinesq in 1885 gave a solution for stress distribution in a homogeneous and isotropic subgrade subjected to a vertical concentrated load (point load) on the ground surface.
What is the influence factor of Boussinesq?
It may be noted that the Boussinesq influence factor for vertical stress is a function of the ratio r/z and is independent of soil properties. It is maximum when r = 0, that is, below the concentrated load, and is equal to 0.4775. Values of I B are computed for different values of r/z and are presented in Table 8.1. The vertical stress computed by the Boussinesq theory for a point load is infinite when z = 0, that is, at the ground surface, where the load is applied.
How to find vertical stress distribution?
The vertical stress distribution on a horizontal plane at any given depth, say z = 1 m, due to concentrated load, can be computed by substituting different values of r in Eq. (8.7) for the same depth z = 1 m. From Eq. (8.7),
Which theory gives the vertical stress a 50% higher value than that given by the Boussinesq theory?
Thus, Westergaard’s theory gives the vertical stress a 50% higher value than that given by the Boussinesq theory at any depth below the axis of the load.
What is the vertical stress of a square footing?
It approaches the average vertical stress at a depth of 0.2B. We know that the vertical stress at the edge of a square footing is about 50% of that at the center. Hence, the 2:1 distribution method underestimates vertical stress, especially for depths less than about 1.5B, where stresses are significant. Hence, the use of the 2:1 distribution method causes errors on the unsafe side.

Overview
In fluid dynamics, the Boussinesq approximation is used in the field of buoyancy-driven flow (also known as natural convection). It ignores density differences except where they appear in terms multiplied by g, the acceleration due to gravity. The essence of the Boussinesq approximation is that the difference in inertia is negligible but gravity is sufficiently strong to make the specific weight appreciably different between the two fluids. Sound waves are impossible/neglected when the Bo…
The approximation
The Boussinesq approximation is applied to problems where the fluid varies in temperature from one place to another, driving a flow of fluid and heat transfer. The fluid satisfies conservation of mass, conservation of momentum and conservation of energy. In the Boussinesq approximation, variations in fluid properties other than density ρ are ignored, and density only appears when it is multiplied by g, the gravitational acceleration. If u is the local velocity of a parcel of fluid, the conti…
Advantages
The advantage of the approximation arises because when considering a flow of, say, warm and cold water of density ρ1 and ρ2 one needs only to consider a single density ρ: the difference Δρ = ρ1 − ρ2 is negligible. Dimensional analysis shows that, under these circumstances, the only sensible way that acceleration due to gravity g should enter into the equations of motion is in the reduced gravity g′ where
Inversions
One feature of Boussinesq flows is that they look the same when viewed upside-down, provided that the identities of the fluids are reversed. The Boussinesq approximation is inaccurate when the dimensionless density difference Δρ/ρ is of order unity.
For example, consider an open window in a warm room. The warm air inside is less dense than the cold air outside, which flows into the room and down towards the floor. Now imagine the opp…
Further reading
• Boussinesq, Joseph (1897). Théorie de l'écoulement tourbillonnant et tumultueux des liquides dans les lits rectilignes a grande section. Vol. 1. Gauthier-Villars. Retrieved 10 October 2015.
• Kleinstreuer, Clement (1997). Engineering Fluid Dynamics An Interdisciplinary Systems Approach. Cambridge University Press. ISBN 978-0-52-101917-0.