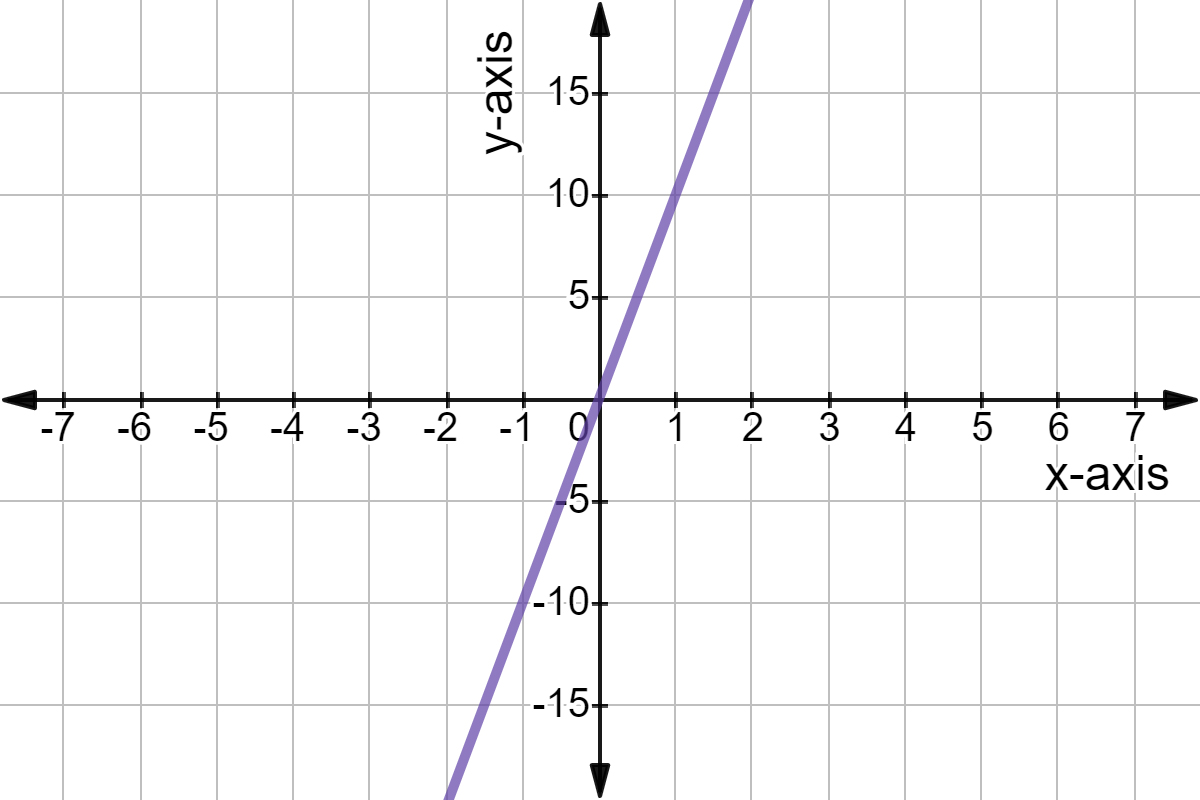
Is 5x a proportional relationship?
A relationship is a proportional relationship if its graph is a straight line. Example 1 : The equation y = 5x represents the relationship between the number of gallons of water used (y) and the number of minutes (x) for most shower heads manufactured before 1994.
Is Y 5x direct or inverse?
It can be observed that as x increases in value , so does y and hence the variation is not inverse, it is direct variation.
What is the constant of proportionality of Y?
The equation for the constant of proportionality is k = y/x. This is the same as the equation of the slope of a straight line that passes through the origin, m = y/x. Using the equation for the slope of the line, one can find the value of m, which will be the same as the value of k, or the constant or proportionality.
What is the constant of proportionality in the equation y 3x?
y results from something being multiplied by x , and the values are always proportional to one another. Notice that putting these points into a ratio of x-value to y-value results in 1:3 as a simplified version. So, the constant of proportionality is what x is being multiplied by to get y . In this case, it is 3 .
What is the constant of 5x?
Algebra Examples The constant of variation, k , is 5 .
How do you graph 5x?
0:012:37How to Graph y = 5x + 1 - YouTubeYouTubeStart of suggested clipEnd of suggested clipLet's graph the linear equation y equals five x plus one we'll do this two ways first we'll putMoreLet's graph the linear equation y equals five x plus one we'll do this two ways first we'll put values in for x.
What is the constant of proportionality of Y 2x?
Since the equation can be written in the form y=kx y = k x , y varies directly with x and k . The constant of variation, k , is −2 .
What is constant of proportionality 7th grade?
0:164:11What is the Constant of Proportionality? | 7th Grade | Mathcation.comYouTubeStart of suggested clipEnd of suggested clipIs the ratio between two proportional quantities this is typically shown using the equation y equalsMoreIs the ratio between two proportional quantities this is typically shown using the equation y equals k x or k equals y divided by x.
How do you write the constant of proportionality?
When two variables are directly or indirectly proportional to each other, then their relationship can be described as y = kx or y = k/x, where k determines how the two variables are related to one another. This k is known as the constant of proportionality.
What is the constant of proportionality of 3y 2x?
Since the equation can be written in the form y=kx y = k x , y varies directly with x and k . The constant of variation, k , is 23 .
What is the constant of proportionality of Y 12x?
12y = 12x, where y is the number of cards and x is the number of packages. This is a direct variation. The constant of proportionality is 12.
How do you find a constant value?
A constant function is a linear function whose general format is y = mx + k, where m and k are constants. Thus, a constant function which is f(x) = k (or) y = k can be written as y = 0x + k. Comparing this equation with the slope-intercept form y = mx+b, we get its slope to be m = 0.
What is the inverse of 5x?
Cancel the common factor of 5 5 . Cancel the common factor. Since f−1(f(x))=x f - 1 ( f ( x ) ) = x and f(f−1(x))=x f ( f - 1 ( x ) ) = x , then f−1(x)=x5 f - 1 ( x ) = x 5 is the inverse of f(x)=5x f ( x ) = 5 x .
Does y 5x represent a direct variation?
Yes, y=5x is a direct variation and the constant of variation is 5 .
How do you know if it is direct or inverse proportion?
In direct proportion, if one quantity is increased or decreased then the other quantity increases or decreases, respectively. But in indirect or inverse proportion, if one quantity increases then other quantity decreases and vice-versa.
Is Y 5x 0 a direct variation?
This is a direct variation , as y increases , x increases.
Answer
The equation for direct variation is y = kx where k is the constant of proportionality
New questions in Mathematics
Answer this plzz ! Will give brainliest ! Perform the indicated operations using order of operations rules. (ANSWER WITH WORK)