Examples of Irrational Numbers (With Lists)
- Irrational Number – Definition Any real number that is not rational is irrational. ...
- Prime Square Roots We can use prime numbers to find irrational numbers. ...
- Logarithms of Primes ...
- Sum of Rational & Irrational ...
- Product of Rational Irrational ...
- Infinite Continued Fraction ...
- π – A Ratio that is not Rational ...
- The Number e – sum of infinite Quotients ...
Full Answer
What are some examples of irrational numbers?
What are some real life examples of irrational numbers?
- Pi, which begins with 3.14, is one of the most common irrational numbers.
- e, also known as Euler’s number, is another common irrational number.
- The Square Root of 2, written as √2, is also an irrational number.
What numbers are irrational numbers?
What is the Irrational Number?
- List of Irrational Numbers. The irrational numbers consist of Pi, Euler’s number, Golden ratio, and many others. ...
- Properties. The irrational numbers are the subsets of the real numbers. ...
- Set of Irrational Numbers. All the square roots that are not perfect square are irrational numbers. ...
- Irrational Numbers on Number Line. ...
- Solved Examples. ...
- Summary. ...
- FAQs. ...
What's the difference between rational and irrational numbers?
The difference between Rational and Irrational numbers is as follows:
| Rational Numbers | Irrational Numbers |
| Rational numbers refer to a number that ... | An irrational number is one that can’t b ... |
| Rational numbers are expressed in fracti ... | Irrational numbers cannot be expressed i ... |
| Rational numbers are perfect squares | Irrational Numbers are Surds |
| Rational numbers are finite or recurring ... | Irrational Numbers are non-finite or non ... |
How to approximate irrational numbers?
- Count up until you hit a square root that works.
- Count down until you hit a square root that works.
- Square root the high and low number, then graph their points on a number line.
- Your estimate should be somewhere between those two numbers. Example: Estimate √5 and then graph your estimate on the number line

What is irrational numbers and examples?
An irrational number is a type of real number which cannot be represented as a simple fraction. It cannot be expressed in the form of a ratio. If N is irrational, then N is not equal to p/q where p and q are integers and q is not equal to 0. Example: √2, √3, √5, √11, √21, π(Pi) are all irrational.
What defines an irrational number?
An irrational number is a number that cannot be expressed as a fraction for any integers and. . Irrational numbers have decimal expansions that neither terminate nor become periodic. Every transcendental number is irrational.
What are 10 examples of irrational numbers?
These are listed below: √2, √3, √5, √7, √11, √13 … √9949, √9967, and √9973. Now we can create infinite irrationals using these and the multiplication rule....See the lists of such numbers below:List A: 2√2, 3√2, 4√2, …List B: 2π, 3π, 4π, …List C: 2log35, 3log35, 4log35, …
How can you tell if a number is rational or irrational?
A rational number is the one which can be represented in the form of P/Q where P and Q are integers and Q ≠ 0. But an irrational number cannot be written in the form of simple fractions. ⅔ is an example of a rational number whereas √2 is an irrational number.
What is an irrational number for dummies?
a nonrepeating or nonterminating decimal but not as a fraction, the decimal goes on forever without repeating. EXAMPLES: Phi, pi and the square root of any prime number are irrational numbers.
What is the most famous irrational number?
PiAnd on Pi Day — March 14, or 3/14 — we love to celebrate the world's most famous irrational number, pi, whose first 10 digits are 3.141592653. As the ratio of a circle's circumference to its diameter, pi is not just irrational, meaning it can't be written as a simple fraction.
What are the three famous irrational numbers?
4 Famous Irrational Numbers Pi describes the ratio of a circle's circumference in relationship to its diameter. ... Phi Φ (pronounced fie) describes the ratio of line segments divided in a specific way. ... The square root of 2 is another well-known irrational number.More items...
Is 7/5 A irrational numbers?
Hence, it is proved that 7 √ 5 Is irrational number.
What is an irrational number? Give an example.
An irrational number is a type of real number which cannot be represented as a simple fraction. It cannot be expressed in the form of a ratio. If N...
Are integers irrational numbers?
Integers are rational numbers but not irrational. All the integers whether they are positive or negative or zero can be written in the form of p/q....
Is an irrational number a real number?
Yes, an irrational number is a real number and not a complex number, because it is possible to represent these numbers in the number line.
What are the five examples of irrational numbers?
There are many irrational numbers that cannot be written in simplified form. Some of the examples are: √8, √11, √50, Euler’s Number e = 2.718281,...
What are the main irrational numbers?
The most common irrational numbers are: Pi (π) = 22/7 = 3.14159265358979… Euler’s Number, e = 2.71828182845904… Golden ratio, φ = 1.618033988749...
What is an irrational number?
Irrational Numbers. Irrational numbers are those real numbers that cannot be represented in the form of a ratio. In other words, those real numbers that are not rational numbers are known as irrational numbers. Hippasus, a Pythagorean philosopher, discovered irrational numbers in the 5th century BC.
What are the properties of irrational numbers?
Given below are some of the properties of irrational numbers: Irrational numbers consist of non-terminating and non-recurring decimals. These are real numbers only. When an irrational and a rational number are added, the result or their sum is an irrational number only.
What is rational and irrational worksheet?
Rational and irrational numbers worksheets can provide a better understanding of why rational and irrational numbers are part of real numbers. Rational and irrational numbers worksheets include a variety of problems and examples based on operations and properties of rational and irrational numbers. It consists of creative and engaging fun activities where a child can explore end-to-end concepts of rational and irrational numbers in detail with practical illustrations.
When an irrational and a rational number are added, the result or their sum is an i?
When an irrational and a rational number are added, the result or their sum is an irrational number only. For an irrational number x, and a rational number y, their result, x+y = an irrational number.
How many irrational numbers are there between root 2 and root 3?
We can have infinitely many irrational numbers between root 2 and root 3. A few examples of irrational numbers between root 2 and root 3 are 1.575775777 ..., 1.4243443..., and 1.686970...
Is pi a decimal?
ㄫ (pi) is an irrational number. π=3⋅14159265… The decimal value never stops at any point. Since the value of ㄫ is closer to the fraction 22/7, we take the value of pi as 22/7 or 3.14 (Note: 22/7 is a rational number.)
Is a set of irrational numbers closed?
The set of irrational numbers is not closed under the multiplication process, unlike the set of rational numbers. The addition or the multiplication of two irrational numbers may be rational; for example, √2 × √2 = 2. Here, √2 is an irrational number.
What is the irrational number?
e, also known as Euler's number, is another common irrational number. The number is named for Leonard Euler, who first introduced e in 1731 in a letter he wrote; however, he had started using the number in 1727 or 1728. e is a universal number.
What are some examples of irrational numbers?
Common Examples of Irrational Numbers. Pi, which begins with 3.14 , is one of the most common irrational numbers. Pi is determined by calculating the ratio of the circumference of a circle (the distance around the circle) to the diameter of that same circle (the distance across the circle).
Can an irrational number be expressed as a ratio?
An irrational number cannot be expressed as a ratio between two numbers and it cannot be written as a simple fraction because there is not a finite number of numbers when written as a decimal. Instead, the numbers in the decimal would go on forever, without repeating.
Is the Golden Ratio irrational?
Many other square roots and cubed roots are irrational numbers; however, not all square roots are. The Golden Ratio, written as a symbol, is an irrational number that begins with 1.61803398874989484820...
Is the square root of 2 irrational?
The Square Root of 2, written as √2, is also an irrational number. The first part of this number would be written as 1.41421356237…but the numbers go on into infinity and do not ever repeat, and they do not ever terminate. A square root is the opposite of squaring a number, meaning that the square root of two times the square root of two equals two.
Prime Square Roots
We can use prime numbers to find irrational numbers. For example, √5 is an irrational number. We can prove that the square root of any prime number is irrational. So √2, √3, √5, √7, √11, √13, √17, √19 … are all irrational numbers.
Logarithms of Primes
The logarithm of a prime number with a prime base, like log 35 or log 72, is irrational. See the proof below:
Sum of Rational & Irrational
Adding a rational number to an irrational number is an easy way to create new irrational numbers. See the lists of numbers created using this method:
Product of Rational Irrational
What works for the sum of a rational and an irrational number, works for their product also. This provides yet another method to create examples of irrational numbers. See the lists of such numbers below:
Infinite Continued Fraction
This is one of the better ways to represent irrational numbers. It takes the form:
π – A Ratio that is not Rational
The number Pi originated from geometry. It is the ratio of the circumference and the diameter of a circle. It remains constant, independent of the size of the circle.
The Number e – sum of infinite Quotients
The number e is a recent discovery compared to Pi. Jacob Bernoulli was trying to compute a continuously compounded interest growth in the 17th century. In a nutshell, he was evaluating (1 + 1/n) n, as n grows to infinity. The graph below plots of values of this expression along the y-axis and n along the x-axis.
What are some examples of natural numbers?
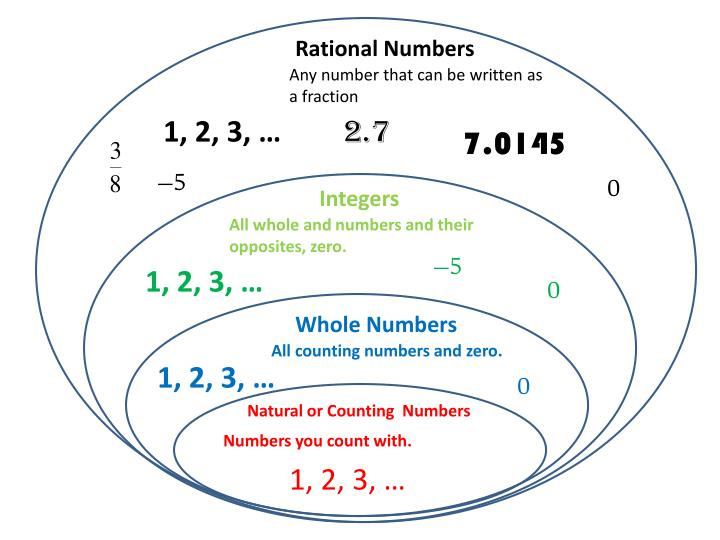
Let's start with some basic examples. When you first learn to count, say, the number of fingers that you have, you use natural, or whole numbers: 1, 2, 3, 4, 5 … and so on. These are natural numbers. Whole numbers are also numbers without fractions, but whole numbers include 0 while natural numbers do not.
What is the ratio of a circle's circumference to its diameter?
Pi is the ratio of a circle's circumference to its diameter. Its decimals go on infinitely long. You may know the first couple digits as 3.14.
What is the decimal expansion of 1/3?
Rational numbers can also be divided into two types: those that have a terminating decimal expansion and those that have a repeating decimal expansion. A decimal expansion when a fraction is converted into a decimal number. The decimal expansion of 1/2 is 0.5. The decimal expansion of 1/3 is 0.333.
Can rational numbers be expressed as fractions?
A rational number can be expressed as a fraction.
Is a square root rational or irrational?
Depending on whether you are able to write them as a fraction, some square roots and cube roots are rational and some are irrational.
Is 0 a whole number?
Rational numbers, on the other hand, can be split into rational numbers with repeating decimals and rational numbers with non-repeating decimals. Natural numbers and whole numbers are the smallest subsets, and 0 is considered a whole number but not a natural number .
