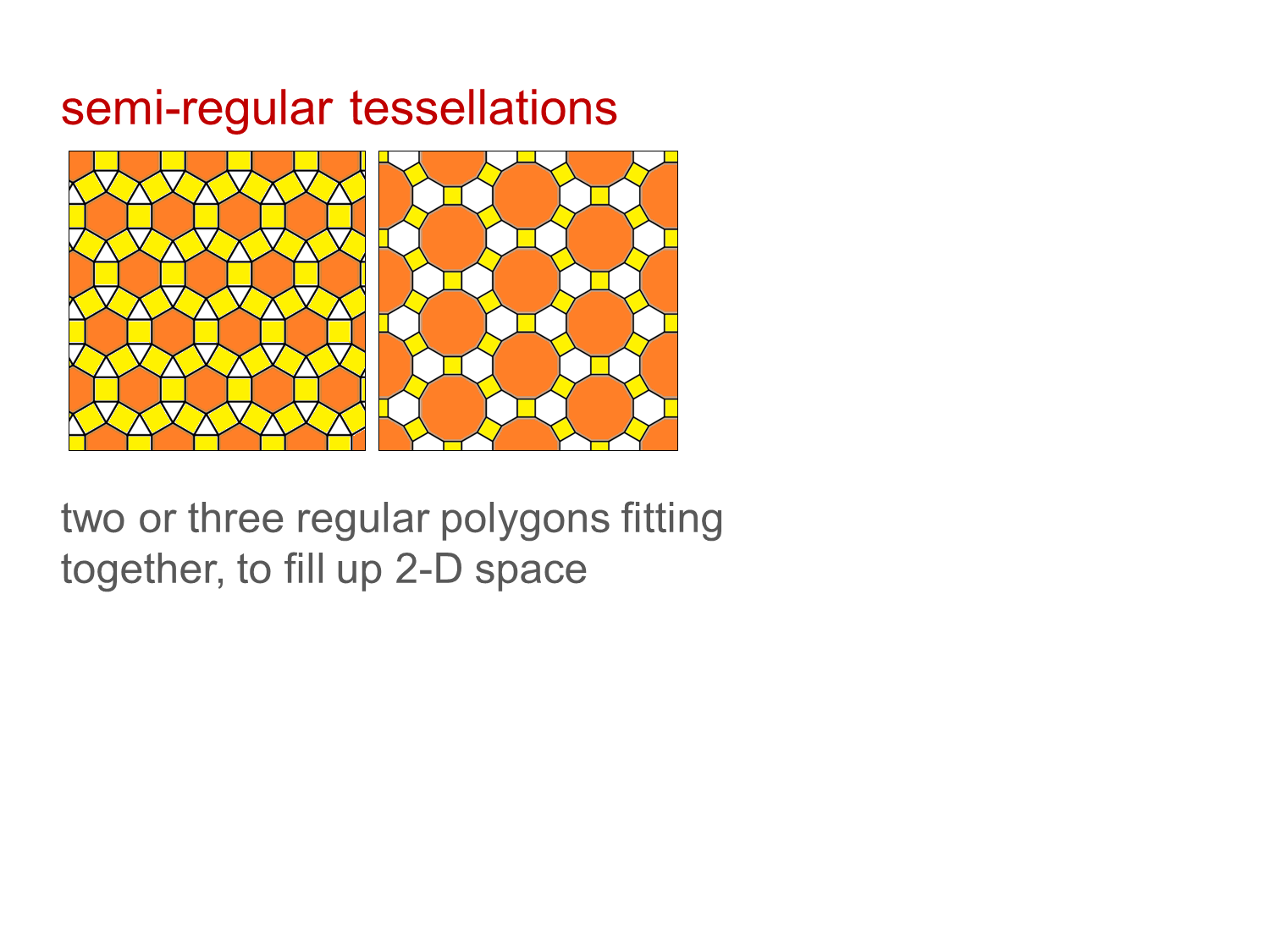
There are three different types of tessellations ( source ):
- Regular tessellations are composed of identically sized and shaped regular polygons.
- Semi-regular tessellations are made from multiple regular polygons. ...
- Meanwhile, irregular tessellations consist of figures that aren't composed of regular polygons that interlock without gaps or overlaps. ...
What is a semi-regular tessellation?
In the example given above of a regular tessellation of hexagons, next to the vertex there are a total of three polygons and each of them has six sides, so this tessellation is called "6.6.6". When two or three types of polygons share a common vertex, then a semi-regular tessellation is formed.
What is the difference between regular and semi-regular polygons?
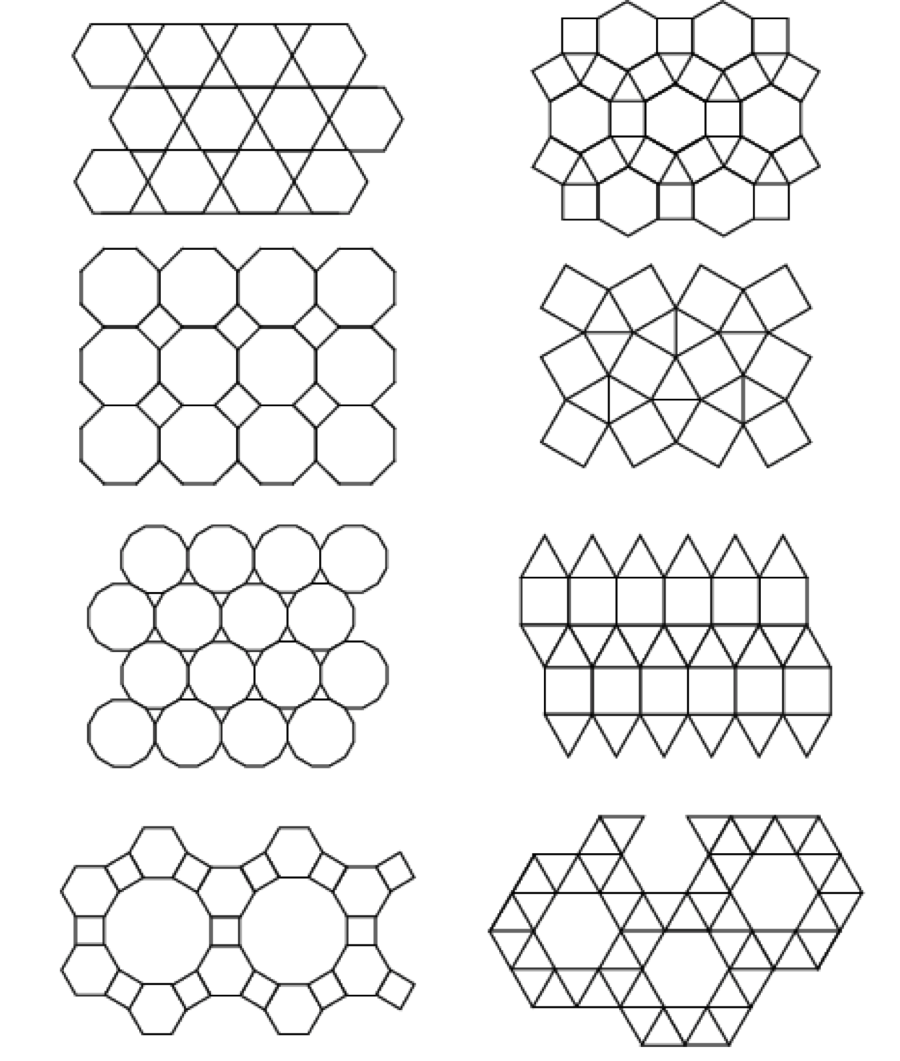
A regular polygon is a polygon where all the sides and angles are the same. All of the polygons in a semi-regular tessellation must be the same length for the pattern to work. As we said earlier, semi-regular tessellations are special because there are only eight of them.
How many regular tessellations are there?
The three regular tessellations are for convenience included here as special cases of semi-regular ones. An example of a semi-regular tessellation is that with triangle–triangle–square–triangle–square in cyclic order, at each vertex.
What is a tessellation in geometry?
A tessellation is a pattern of a shape or shapes in geometry that repeat. There are as many tessellations as you can imagine. Semi-Regular Tessellations. A semi-regular tessellation is made up of two or more regular polygons that are arranged the same at every vertex, which is just a fancy math name for a corner.

What is the difference between semi tessellation and regular tessellation?
Regular tessellations of the plane by two or more convex regular polygons such that the same polygons in the same order surround each polygon vertex are called semiregular tessellations, or sometimes Archimedean tessellations.
What is semi-regular tessellations?
A semi-regular tessellation is one consisting of regular polygons of the same length of side, with the same 'behaviour' at each vertex. By this we mean that the polygons appear in the same order (though different senses are allowed) at each vertex.
What is a semi-regular tessellation how many semi-regular tessellations are possible?
There are 8 semi-regular tessellations in total. We know each is correct because again, the internal angle of these shapes add up to 360. For example, for triangles and squares, 60 \times 3 + 90 \times 2 = 360.
What's regular about tessellations?
A regular tessellation is one made using only one regular polygon. A semi-regular tessellation uses two or more regular polygons. Triangles and squares, for example, form regular tessellations and octagons and squares for a semi-regular tessellation.
What are the 3 different types of tessellation?
There are three types of regular tessellations: triangles, squares and hexagons.
What does semi regularly mean?
semiregular (not comparable) Somewhat regular; occasional.
Why are there only 3 regular tessellations?
Which regular polygons will tessellate on their own without any spaces or overlaps? Equilateral triangles, squares and regular hexagons are the only regular polygons that will tessellate. Therefore, there are only three regular tessellations.
Why are there only 8 semi regular tessellations?
Answer and Explanation: The reason there are only eight semi-regular tessellations has to do with the angle measures of various regular polygons. Each vertex of a tessellation must contain 360° total in order for there to be no gaps or spaces between the shapes.
How many regular tessellations are there?
three regular tessellationsTherefore, there are only three regular tessellations (composed of the hexagon, square, and triangle), illustrated above (Ghyka 1977, p.
Which is not a regular tessellation?
A non-regular tessellation is a group of shapes that have the sum of all interior angles equaling 360 degrees. There are again, no overlaps or gaps, and non-regular tessellations are formed many times using polygons that are not regular.
How do you know if a regular polygon will tessellate?
How do you know that a figure will tessellate? If the figure is the same on all sides, it will fit together when it is repeated. Figures that tessellate tend to be regular polygons.
What only 3 shapes can tessellate?
Only three regular polygons(shapes with all sides and angles equal) can form a tessellation by themselves—triangles, squares, and hexagons.
Why are there only 8 semi regular tessellations?
Answer and Explanation: The reason there are only eight semi-regular tessellations has to do with the angle measures of various regular polygons. Each vertex of a tessellation must contain 360° total in order for there to be no gaps or spaces between the shapes.
What shapes Cannot Tessellate?
Shapes That Do Not Tessellate Circles or ovals, for example, cannot tessellate. Not only do they not have angles, but you can clearly see that it is impossible to put a series of circles next to each other without a gap. See? Circles cannot tessellate.
1. What Shapes Cannot Tessellate?
There are shapes that are unable to tessellate by themselves. Circles, for example, cannot tessellate. Not only do they not have angles, but it is...
2. Is Tessellation Math or Art?
Tessellation, or tiling, is the covering of the plane by closed shapes, called tiles, without gaps or overlaps 17, page 157. Tessellations have man...
3. What does Tessellate Mean?
A tessellation is a pattern created with identical shapes that fit together with no gaps. Regular polygons tessellate if the interior angles of the...
4. What are the 3 Types of Tessellations?
There are three types of tessellations: Translation, Rotation, and Reflection.
5. Can tessellations overlap?
A tessellation is a sample of shapes repeated to fill a plane. The shapes of Tessellations do not overlap.
6. What are the number of varieties of tessellations present?
There are 3 types of normal tessellations: triangles, squares and hexagons.
7. What are the main features of tessellations?
The key functions of tessellations are that there should be no gaps or overlaps in shapes. The identical discern (or institution of figures) come c...
What is a pattern of shapes that fit perfectly together?
Tessellation. A pattern of shapes that fit perfectly together! A Tessellation (or Tiling) is when we cover a surface with a pattern of flat shapes so that there are no overlaps or gaps.
What is a regular tessellation?
A regular tessellation is a pattern made by repeating a regular polygon.
How to name a tessellation?
To name a tessellation, go around a vertex and write down how many sides each polygon has, in order ... like "3.12.12".
Can curved shapes be tessellations?
And some people allow curved shapes (not just polygons) so we can have tessellations like these:
Can you try tessellation?
You can try it too - maybe you will invent a new tessellation!
How to tell if a pattern is semi-regular?
First, identify the types of polygons in the pattern. There are regular triangles (shown in green) and regular hexagons, or 6-sided figures (shown in grey). So far, the definition of a semi-regular tessellation is working because there are at least two regular polygons. Now, see if they are arranged the same way at every vertex and if all of the sides of all of the shapes are the same length.
What is a tessellation pattern?
A tessellation is a pattern of a shape or shapes in geometry that repeat. There are as many tessellations as you can imagine.
What is a regular polygon?
A regular polygon is a polygon where all the sides and angles are the same. All of the polygons in a semi-regular tessellation must be the same length for the pattern to work. As we said earlier, semi-regular tessellations are special because there are only eight of them.
How many semi-regular tessellations are there?
Lesson Summary. A tessellation is a geometric pattern of shapes that repeats itself. There are a lot of tessellations; however, there are only eight semi-regular-tessellations. A semi-regular tessellation is made up of two or more regular polygons, which have equal sides and angles that are arranged the same at every vertex.
How many siblings does Barry have?
Tessellations in Real Life. Barry has five brothers and sisters. All six siblings asked their mother if each one of them could have a cookie. There were only five cookies. That meant that one person wasn't going to get a cookie. Barry said he would trade his cookie for one dollar. A dollar is a lot for a cookie, ...
What do adults say to you?
Often adults will tell you things over and over. 'Look both ways before crossing the street.' ' Don't get in a car with someone without my permission.' and 'Eat your vegetables.' Well, just like your parents, semi-regular tessellations repeat too!
What did Barry say he would trade for?
Barry said he would trade his cookie for one dollar. A dollar is a lot for a cookie, but one of his brothers gave it to him anyway. Why did his brother pay so much for a cookie? Well, there weren't many of them, so each one was very important, just like semi-regular tessellations.
Triominoes
A triomino is a flat L shape made from 3 square tiles. A chess board is marked into squares the same size as the tiles and just one square, anywhere on the board, is coloured red. Can you cover the board with trionimoes so that only the square is exposed?
LOGO Challenge 5 - Patch
Using LOGO, can you construct elegant procedures that will draw this family of 'floor coverings'?
LOGO Challenge - Triangles-squares-stars
Can you recreate these designs? What are the basic units? What movement is required between each unit? Some elegant use of procedures will help - variables not essential.
What are The Three Types of Tessellations?
There are three types of tessellations that you’re going to come across, and they are as follows:
What is a Tessellation Pattern?
A tessellation pattern, also called tiling, is when a number of different shapes fit together perfectly on a flat plane. There are three types that we have already discussed, and it is linked closely to hyperbolic geometry.
What About Fractals?
A fractal is made by repeating a mathematical fraction repeatedly, so it cannot be created by hand. You’re not going to see any artists publishing fractal-based geometric artwork any time soon. The closest thing we’re going to get to that is one of the numerous Twitter accounts that post-AI-generated fractal artwork.
What is the difference between fractal and tessellation?
To boil it all down, the primary contrast between them is that a tessellation is much more closely linked to the artistic world, whereas a fractal is far more mathematic in nature.
What is a semi-regular tessellation?
Semi-regular tessellations: When two or three different polygonal shapes share a common vortex, it is called a semi-regular tessellation. There are nine different types of semi-regular tessellations that can be created by using various shapes at various lengths, such as combining triangles, hexagons, and squares.
When was the first tessellation made?
Escher produced his first tessellation in 1925. At the time it was a new and fresh entry into the art world and featured a series of interlocking lions, the first of a many number of works to use animals to divide the plane.
Where can you find fractals?
When it comes to real-life applications and examples of fractals, you’re much more likely to find them in nature than you are in anything man-made. You can see fractal designs in a number of different types of plants and wildlife. Certain types of trees, broccoli, and certain cactuses all fit under the umbrella here.
Answer
Answer: Semi-regular tessellations are made of more than one kind of regular polygon. There are only 8 semi-regular tessellations.
New questions in Mathematics
the whether the givenm statements below illustrate quadratic inequality in one variable or not.you may translate into mathematics symbol to justify yo …