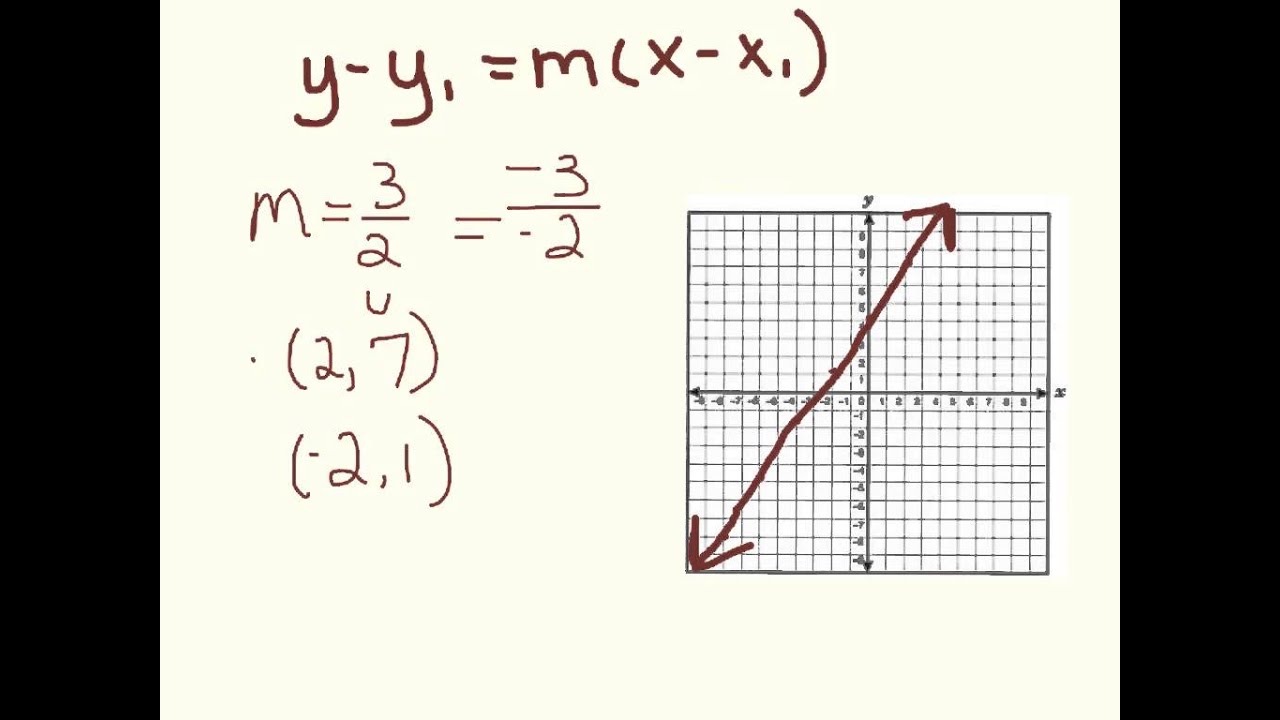
Important Notes on Linear Functions:
- A linear function is of the form f (x) = mx + b and hence its graph is a line.
- A linear function f (x) = mx + b is a horizontal line when its slope is 0 and in this case, it is known as a constant function.
- The domain and range of a linear function f (x) = ax + b is R (all real numbers) whereas the range of a constant function f (x) = b ...
...
Identifying a Linear Function.
| x | y |
|---|---|
| 13 | 55 |
How do you solve a linear equation?
The methods for solving linear equations are given below:
- Substitution method
- Elimination method
- Cross multiplication method
- Graphical method
How do you calculate linear function?
Solving Linear Functions
- Substitute the value of f ( x) into the problem.
- Isolate the variable. In this case, you add 1 to both sides to isolate the variable term by using the opposite operation to move the constant term across ...
- Continue to isolate the variable. ...
- Because this is a linear function, it's appropriate to format the final answer in function form. ...
How to identify a linear equation?
Identifying Basic Linear Equations. Step 1: Read through the given equations to see how many variables are present. If the equation is between more than 2 variables (i.e. y and x), this equation ...
How do you calculate linear equations?
To solve a linear equation with fraction, follow these steps:
- Make any complex fraction into a simple fraction
- Find the LCM of all denominators
- Multiply the equation with the LCM of the denominator
- Cancel out the fractions as all the denominators can be divided by the LCM value
- Solve the final linear equation using any of the methods explained here
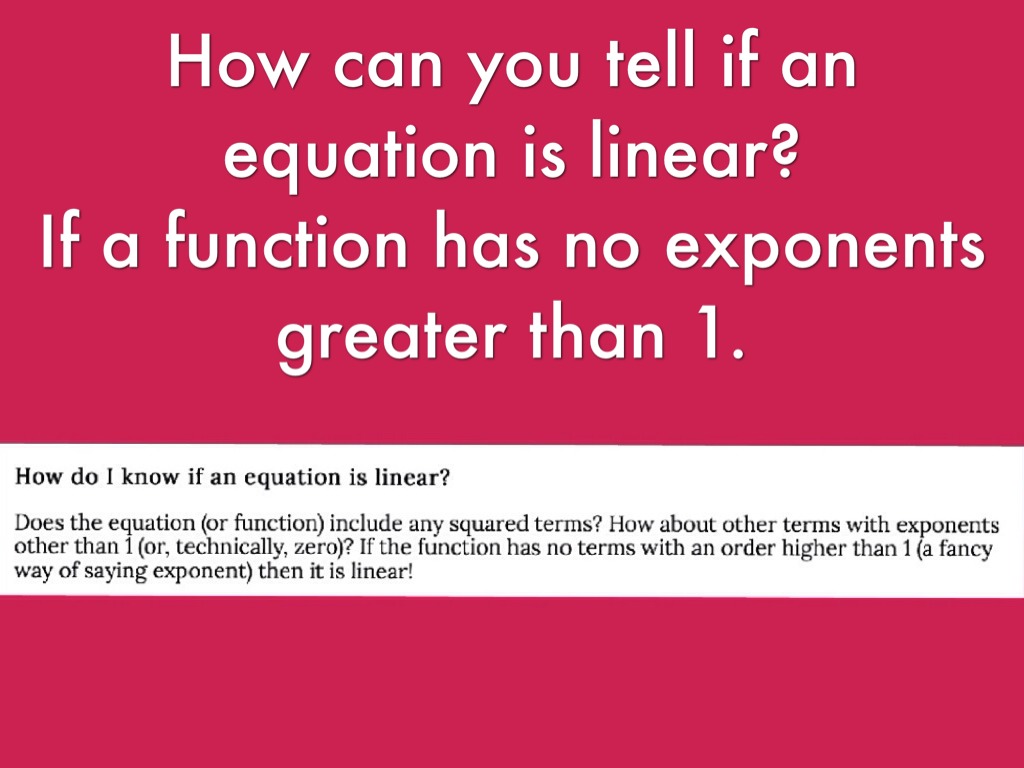
How do you tell if a linear equation is a function?
Locate the exponent with the highest degree out of the terms. That exponent is the degree of the polynomial. If it is one, it is a linear equation. Because the highest power of "x" in y = 7/5 - (6/5)x is 1, it is a linear function.
What is a linear function simple definition?
Definition of linear function 1 : a mathematical function in which the variables appear only in the first degree, are multiplied by constants, and are combined only by addition and subtraction.
What is a Linear Function?
A linear function is a function which forms a straight line in a graph. It is generally a polynomial function whose degree is utmost 1 or 0. Although the linear functions are also represented in terms of calculus as well as linear algebra. The only difference is the function notation. Knowing an ordered pair written in function notation is necessary too. f (a) is called a function, where a is an independent variable in which the function is dependent. Linear Function Graph has a straight line whose expression or formula is given by;
What is a function that is not linear?
A function which is not linear is called nonlinear function. In other words, a function which does not form a straight line in a graph. The examples of such functions are exponential function, parabolic function, inverse functions, quadratic function, etc. All these functions do not satisfy the linear equation y = m x + c.
How to verify a linear function?
Using the table, we can verify the linear function, by examining the values of x and y. For the linear function, the rate of change of y with respect the variable x remains constant. Then, the rate of change is called the slope.
What is a variable in math?
Variable: A symbol that shows a quantity in a math expression. Linear function: If each term is either a constant or It is the product of a constant and also (the first power of) a single variable, then it is called as an algebraic equation. Function: A function is a relation between a set of inputs and a set of permissible outputs.
How to Solve Linear Equations?
Similarly, if we multiply or divide the same number on both sides of an equation, it is correct. We bring the variables to one side of the equation and the constant to the other side and then find the value of the unknown variable. This is the way to solve a linear equation with one variable. Let us understand this with the help of an example.
What is the value of the variable that makes a linear equation true?
The values of the variable that makes a linear equation true are called the solution or root of the linear equation. The solution of a linear equation is unaffected if the same number is added, subtracted, multiplied, or divided into both sides of the equation.
How to tell if an equation is linear or quadratic?
Whereas, quadratic equations have one variable which is raised to the second power. The general form of a quadratic equation is expressed as, y = ax 2 + bx + c. Another difference between the two equations is that a linear equation forms a straight line whereas a quadratic equation forms a parabola.
How to solve linear equations in two variables?
For example, 6x + 2y + 9 = 0 is a linear equation in two variables. There are various ways to solve linear equations in two variables like the Graphical Method, the Substitution Method, the Cross Multiplication Method, the Elimination Method and the Determinant Method. After solving these, we get the solutions for both the variables.
How many variables can be in a linear equation?
Linear Equations are a wide variety of equations altogether. There can be linear equations in one variable, linear equations in two variables, and so on. In every equation, one thing remains constant: The highest (and the only) degree of all variables in the equation should be 1. Other than that, constants (zero degree variables) can be there.
Why is a graph of a linear equation called a linear equation?
The graph of a linear equation in one variable x forms a vertical line parallel to the y-axis and vice-versa, whereas the graph of a linear equation in two variables x and y forms a straight line. The reason an equation of degree one is called a linear equation is that its geometrical representation is a straight line. Given above are a few examples of how we plot linear equations on a graph.
What is the standard form of a linear equation in one variable?
The standard form of a linear equation in one variable is of the form ax + b = 0.
Why are linear functions easy to spot?
Choose whichever method you find easier. Lesson Summary. Linear functions are easy to spot because they all have to graph into a straight line. They can have a maximum of two variables, where all of the variables are in the numerator and none have exponents or powers.
What is linear function?
A linear function is any function that graphs to a straight line. What this means mathematically is that the function has either one or two variables with no exponents or powers. If the function has more variables, the variables must be constants or known variables for the function to remain a linear function. {"error":true,"iframe":true}.
What is the third item in a function?
The third item is that the function must graph to a straight line. Any kind of a curve disqualifies the function.
How to identify a linear function?
To identify linear functions, you can create a checklist of several items the function must meet. The first item the function must satisfy is that it must have either one or two real variables. If another variable is present, it must be a known variable or constant. For example, the function C = 2 * pi * r is a linear function because only ...
What does it mean to enroll in a course?
Enrolling in a course lets you earn progress by passing quizzes and exams.
How to calculate the cost of coffee?
1. If 1 cup of coffee is purchased, the total cost is $2.00. If 2 cups are purchased, the total cost is 2*$2.00 = $4.00. If 3 cups are purchased, the total cost is 3*$2.00 = $6.00. If 4 cups are purchased, the total cost is 4*$2.00 = $8.00. Plotting the points on a graph, using x for the number of cups and y for the total cost in dollars, we have:
How many points can you use to plot a function?
If you know that a function is linear, you can plot the graph using just two points. If you are unsure, you can use three or four points to double check.
How to find the y intercept of a linear function?
Given a linear function in slope-intercept form, {eq}y=mx+b {/eq }, the y-intercept is given by {eq} (0, b) {/eq}. Since the y-intercept occurs on the y-axis, the x-coordinate of this intercept will always be zero. Thus, substituting zero into the equation gives {eq}y=b {/eq} which is the y-intercept.
What is the difference between x and y intercepts?
The x-intercept is the point where the graph crosses the x-axis, while the y-intercept is the point at which the graph crosses the y-axis. But, how are they found from the equation of a linear function? First, look at a linear function in slope-intercept form.
What can be used to determine output given input and input given output?
Finally, the linear function graph can be used to determine output given input and input given output.
What happens if the slope is undefined?
If the slope is undefined, then the graph of the line will be a vertical line. As noted before, vertical lines are not functions and have undefined slopes.
Why does the graph have a straight line?
This equation generates a straight line because the power of x is always one demonstrating that there is only one x variable. As long as the exponent of x is one, the graph is a straight line. Any other values will yield nonlinear curves.
How to find the y intercept?
To find the y-intercept, find the point at which the graph crosses the y-axis. In this case, the point is {eq} (0, 1) {/eq}.
What is a non linear function?
What is a linear function? Well, a linear function is any function that, when graphed, is a straight line. A non-linear function is any function that is not linear. The general form of a linear function that appears is called the slope-intercept form:
What is linear function?
Ans. When two or more different notions have a distinct relation between them, they are referred to as linear function. This mathematical term can be found in calculus as well as other related areas where it is represented with a straight line. Its formula shows the relationship between x-axis, y-axis and its slope. Its slope can be positive, negative, undefined or zero.
What is the difference between a linear and a nonlinear function?
The most evident difference between a linear and a nonlinear function is when their values are put on a graph the former forms a straight line while the latter forms a curve.
What is the importance of graphing data?
Graphical representation of data is not just an important aspect of mathematics but a key element in the field of economics as well. The linear function is one such topic that will be present in both subjects. This entire topic revolves around a single equation that represents the increase and decrease of a variable when the other variables are kept constant.
How to verify a table?
By utilising the table, one can verify it by checking the values assigned to x and y. In this type of function the rate at which y changes in respect to x remains constant. That means
When a line goes up from left to right, is it increasing?
In contrast, if the goes down from left to right, it is decreasing or b ˂ 0. If the line is vertical, its slope is said to be undefined, and when it is horizontal, its slope is zero.
Is y a dependent variable?
Here ‘y’ represents the Y-axis in the graph and is a dependent variable as its value depends on the value of ‘x’. In contrast, ‘x’ here is an independent variable and represents the X-axis. In this equation, ‘a’ is a constant and when ‘x=0’ it is also the value of ‘y’. Thus ‘a’ is also called the y-intercept in this equation. The other constant in this equation is b’’, and its value is never 0.
Is slope constant or variable?
Ans. The slope in this type of function is constant. In mathematics, the steepness and direction both are described by the slope of a line. Its line’s direction can be increasing or decreasing, vertical or horizontal depending upon its value.
Why is it called the identity function?
There is a special linear function called the "Identity Function": It is called "Identity" because what comes out is identical to what goes in:
Is f always equal to a constant?
No matter what value of "x", f (x) is always equal to some constant value.
How to Solve Linear Equations?
Now let us learn how to solve linear equations or line equations in one variable, in two variables and in three variables with examples. Solving these equations with step by step procedures are given here.
What is the maximum order of an equation?
An equation having the maximum order of 1 is known as a Linear equation. Below are some examples of linear equations in 1 variable, 2 variables and 3 variables: Linear Equation in One variable. Linear Equation in Two variable. Linear Equation in Three variable.
What is linear equation?
Linear equations are those equations that are of the first order. These equations are defined for lines in the coordinate system . Linear equations are also first-degree equations as it has the highest exponent of variables as 1.
What is the most common form of linear equations?
The most common form of linear equations is in slope-intercept form, which is represented as;
What does the equality sign mean in math?
Equality sign denotes that the expressions on either side of the ‘equal to’ sign are equal. Since the equation is balanced, for solving it certain mathematical operations are performed on both sides of the equation in a manner that it does not affect the balance of the equation. Here is the example related to the linear equation in one variable.
What is the intercept form of a line?
A line which is neither parallel to x-axis or y-axis nor it pass through the origin but intersects the axes in two different points, represents the intercept form. The intercept values x 0 and y 0 of these two points are nonzero and forms an equation of the line as:
What is the slope of a line?
So basically the slope shows the rise of line in the plane along with the distance covered in the x-axis. The slope of the line is also called a gradient.
What is a Linear Model?
A linear model is one that represents the relationship between two quantities and where the degree of the equation is 1. The most basic linear equation demonstrates the relationship between (x,y), which can then be used to create a table or a graph on the coordinate plane or to evaluate at a certain x or y value.
Linear Model Equation
where y represents the output value, m represents the slope or rate of change, x represents the input value, and b represents the constant or the starting amount.
Linear Model Examples
Example 1: Divya is making cookies for her school bake sale. She pays $25 for the ingredients, and sells the cookies for $2 each. What is the linear model that represents this scenario?

Linear Equations in Standard Form
Linear Equations in One Variable
- A linear equation in one variable is an equation in which there is only one variable present. It is of the form Ax + B = 0, where A and B are any two real numbers and x is an unknown variable that has only one solution. It is the easiest way to represent a mathematical statement. This equation has a degreethat is always equal to 1. A linear equatio...
Linear Equations in Two Variables
- A linear equation in two variables is of the form Ax + By + C = 0, in which A, B, C are real numbers and x and y are the two variables, each with a degree of 1. If we consider two such linear equations, they are called simultaneous linear equations. For example, 6x + 2y + 9 = 0 is a linear equation in two variables. There are various ways of solving linear equations in two variables lik…
How to Solve Linear equations?
- An equation is like a weighing balance with equal weights on both sides. If we add or subtract the same number from both sides of an equation, it still holds true. Similarly, if we multiply or divide the same number on both sides of an equation, it is correct. We bring the variables to one side of the equation and the constant to the other side and then find the value of the unknown variable. …