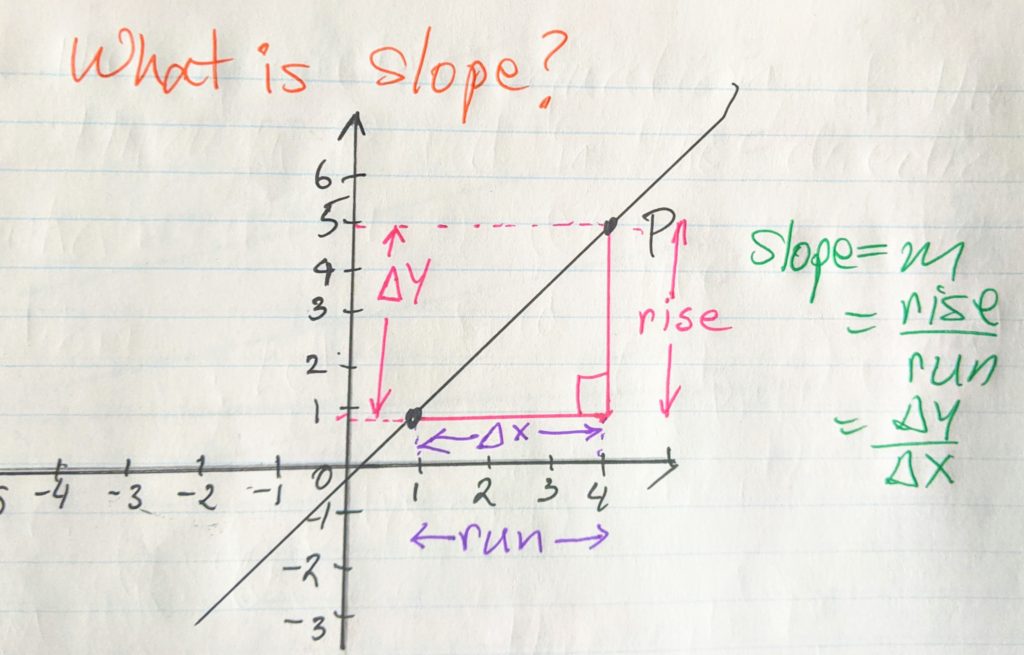
What does the meaning of slope mean?
1 : ground that forms a natural or artificial incline. 2 : upward or downward slant or inclination or degree of slant. 3 : the part of a continent draining to a particular ocean Alaska's North Slope.
What is slope with example?
Whenever the equation of a line is written in the form y = mx + b, it is called the slope-intercept form of the equation. The m is the slope of the line. And b is the b in the point that is the y-intercept (0, b). For example, for the equation y = 3x – 7, the slope is 3, and the y-intercept is (0, −7).
How do I solve for slope?
1:513:15How to Solve for Slope & Y-Intercept - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo we're going to start with the formula again y equals MX plus B and we're going to substitute someMoreSo we're going to start with the formula again y equals MX plus B and we're going to substitute some values in. So I can pick whichever. Point I want to use and most people like to choose the one with
How do you calculate slope?
0:133:50Calculating the Slope of a Line on a Graph - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd how do you calculate the slope. Well i'm mr h and i have some answers for you slope refers toMoreAnd how do you calculate the slope. Well i'm mr h and i have some answers for you slope refers to the steepness of a line on a graph. It is the rise divided by the run the rise is the change in the y-
What is a real life example of slope?
Some real life examples of slope include: in building roads one must figure out how steep the road will be. skiers/snowboarders need to consider the slopes of hills in order to judge the dangers, speeds, etc. when constructing wheelchair ramps, slope is a major consideration.
What are the 4 types of slopes?
0:041:28Review: The 4 Types of Slope of a Line (⤢⤡⟷↕) - YouTubeYouTubeStart of suggested clipEnd of suggested clipHey everyone thanks for joining us on this very short lesson on the different types of slope. WhichMoreHey everyone thanks for joining us on this very short lesson on the different types of slope. Which we are going to look at from a skatepark. Now we want to develop the skill of being able to identify
How do you explain slope to a child?
1:547:34Understanding Slope (Simplifying Math) - YouTubeYouTubeStart of suggested clipEnd of suggested clipIt's often called the rise. Over the run or how much it changes up and down over how much it changesMoreIt's often called the rise. Over the run or how much it changes up and down over how much it changes side to side in this case 2 over 1 that's the slope. That is the slope for the line that's. It.
What is slope of a curve?
The concept of a slope is central to differential calculus. For non-linear functions, the rate of change varies along the curve. The derivative of the function at a point is the slope of the line tangent to the curve at the point, and is thus equal to the rate of change of the function at that point.
1. What is a Zero Slope Line Equation?
Ans. A zero slope line is a perfectly flat, and straight-line running along the horizontal axis of a cartesian plane. The equation of a zero line i...
2. What Does the Negative Slope Mean?
Ans. The negative slope means that the relation of two variables is negatively related i.e. when the value of the variable x increases, the value o...
3. What Does the Positive Slope Mean?
Ans. The positive slope means that the relation of two variables is positively related i.e. when the value of the variable x increases, the value o...
What is slope in math?
The slope is a measure of the steepness of a line, or a section of a line, connecting two points. In this lesson, you will use several different formulas for slope and learn how those formulas relate to the steepness of a line. Updated: 11/27/2019. Create an account.
When is slope a bigger number?
Slope is a bigger number when a line looks flatter.
What does negative slope mean?
Well, negative slope means that the line is slanting downward from the left to the right. When you think of a ski slope, you tend to think of traveling downward because you ski downward no matter which way the slope is facing.
How to tell how steep a slope is?
It is the combination of the two. In fact, the ratio of the height to the width (the height divided by the width) tells you the slope.
How to know if a line is steep or flat?
Steepness. One way to think of the slope of a line is by imagining a roof or a ski slope. Both roofs and ski slopes can be very steep or quite flat. In fact, both ski slopes and roofs, like lines, can be perfectly flat (horizontal). You would never find a ski slope or a roof that was perfectly vertical, but a line might be.
What is a line segment?
It is also possible that you might be given a line segment, which is a section of a line that has a beginning and an end. Or, you might be given two points and you are expected to draw (or imagine) the line segment between them. In all these situations, finding the slope works the same way.
Is slope the same everywhere on a graph?
When graphing on it, a line may not start at zero as in the ski slope examples. In fact, a line goes on forever at both ends. The slope of a line, however, is exactly the same everywhere on the line.
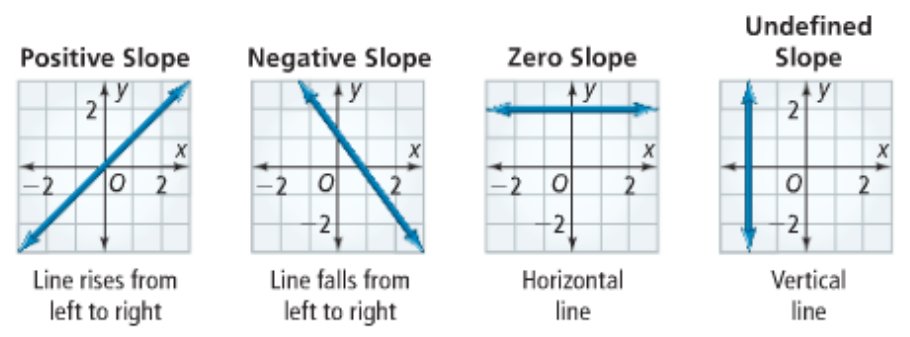
How many types of slopes are there?
There are four types of slope you can encounter. A slope can be
What does it mean when you go uphill?
As you go uphill, you may feel like you are spending lots of energy to get yourself to move. The steeper the hill, the harder it is for you to keep yourself moving. Keeping this fact in mind, by definition, the slope is the measure of the steepness of a line.
Have A Great Problem About Slope?
Do you have great problems about slope? Share them here with the solutions!
What is slope in math?
The slope is the ratio of change in the y-axis and change in the x-axis.
How to find slope?
What is a Slope? 1 A line that extends from left to right has a positive run and positive rise, and also yielding a positive slope i.e. m > 0 2 A line that declines from left to right has a negative run and negative fall, and also yielding a negative slope i.e. m < o 3 Horizontal lines have a zero positive slope, as they have zero rise and a positive run. 4 The slope is undefined if the line is vertical as the vertical line has zero rise and any amount of run.
What Does the Slope of a Velocity Time Graph Give?
When the velocity and time are graphed on the y - axis and the x-axis respectively, then the slope of the velocity-time graph gives the acceleration of the object.
What is a zero slope line?
Ans. A zero slope line is a perfectly flat, and straight-line running along the horizontal axis of a cartesian plane. The equation of a zero line is one where the x values changes but the y values remain constant. Therefore, the equation for a zero line is given as y = b, where the slope of the line is m (m = 0).
What is the equation of a line with gradient 5 passing through (4,1)?
Therefore, the equation of a line with gradient 5, passing through ( 4,1) is y = 5x - 19
What does a slope with a greater absolute value mean?
A slope with a greater absolute value represents a steeper line. The direction of a line either rises, falls, and is horizontal or vertical. A line that extends from left to right has a positive run and positive rise, and also yielding a positive slope i.e. m > 0.
How to find the general equation of a straight line?
The general equation of a straight line is represented in the form of y = mx + c, where m is the gradient and coordinates of the y-intercepts are (0,c).
What is the slope of a line?
In mathematics, the slope or gradient of a line is a number that describes both the direction and the steepness of the line. Slope is often denoted by the letter m; there is no clear answer to the question why the letter m is used for slope, but its earliest use in English appears in O'Brien (1844) who wrote the equation of a straight line as "y = mx + b" and it can also be found in Todhunter (1888) who wrote it as " y = mx + c ".
How to find slope?
Slope is calculated by finding the ratio of the "vertical change" to the "horizontal change" between (any) two distinct points on a line. Sometimes the ratio is expressed as a quotient ("rise over run"), giving the same number for every two distinct points on the same line. A line that is decreasing has a negative "rise". The line may be practical - as set by a road surveyor, or in a diagram that models a road or a roof either as a description or as a plan.
How to describe steepness of a road?
One is by the angle between 0° and 90° (in degrees), and the other is by the slope in a percentage. See also steep grade railway and rack railway .
What is the slope of a nonlinear function?
The concept of a slope is central to differential calculus. For non-linear functions, the rate of change varies along the curve. The derivative of the function at a point is the slope of the line tangent to the curve at the point, and is thus equal to the rate of change of the function at that point.
What is the slope of a 45° rising line?
Thus, a 45° rising line has a slope of +1 and a 45° falling line has a slope of −1.
What is parallel line?
Two lines are parallel if and only if they are not the same line (coincident) and either their slopes are equal or they both are vertical and therefore both have undefined slopes. Two lines are perpendicular if the product of their slopes is −1 or one has a slope of 0 (a horizontal line) and the other has an undefined slope (a vertical line).
What is an angle of 100 %?
where angle is in degrees and the trigonometric functions operate in degrees. For example, a slope of 100 % or 1000 ‰ is an angle of 45°.
What is slope in math?
One of the most important things to understand about lines is the definition of slope. Slope is the 'steepness' of the line, also commonly known as rise over run . We can calculate slope by dividing the change in the y-value between two points over the change in the x-value.
What is slope in algebra?
of algebra is the idea of slope. And slope just refers to. how steep a line is. And that's kind of the basic definition. That's something that I help -- or helps. me remember what slope means. There's a whole lot of fancy notation. that goes along with it. And we'll get into that in just a second.
How to find slope of a line?
We can calculate slope by dividing the change in the y-value between two points over the change in the x-value.
What does slope mean in math?
Slope expresses the steepness and direction of the line. It represents how steep a line is.
What is the slope of a line between two points?
Therefore, Slope, m = Rise/Run.
How to Find Slope of a line on a Graph?
In the given figure, if the angle of inclination of the given line with the x-axis is θ then, the slope of the line is given by tan θ . Hence, there is a relation between the lines and angles. In this article, you will learn various formulas related to the angles and lines.
What is the slope of a line whose equation is y = -2x + 7?
The slope of the line whose equation is y = -2x + 7 is -2.
How to find the slope of a straight line?
The slope of a straight line between two points says (x1,y1) and (x2,y2) can be easily determined by finding the difference between the coordinates of the points. The slope is usually represented by the letter ‘m’.
How to find the inclination of two parallel lines?
Consider two parallel lines given by l1 l 1 and l2 l 2 with inclinations α and β respectively. For two lines to be parallel their inclination must also be equal i.e. α=β. This results in the fact that tan α = tan β. Hence, the condition for two lines with inclinations α, β to be parallel is tan α = tan β.
What happens when the slope is negative?
If the value of slope is negative, then the line goes done in the graph as we move along the x-axis.
Overview
- In mathematics, the slope or gradient of a line is a number that describes both the direction and the steepness of the line. Slope is often denoted by the letter m; there is no clear answer to the question why the letter m is used for slope, but its earliest use in English appears in O'Brien (1844) who wrote the equation of a straight line as "y = mx + b" and it can also be found in Todhunter (1…
Definition
- The slope of a line in the plane containing the x and y axes is generally represented by the letter m, and is defined as the change in the y coordinate divided by the corresponding change in the x coordinate, between two distinct points on the line. This is described by the following equation: m = Δ y Δ x = vertical change horizontal change = rise run. {\displaystyle m={\frac {\Delta y}{\Delta …
- The distance of travel (distance along the slope or hypotenuse of triangle) is obtained from the Pythagorean theorem equation: (hypotenuse distance)2 = 2472 + 2802. Distance along the slope is equal to 373m.
- The slope of a line is defined as its rise (the amount that it travels up or down on a graph as it moves from point to point) divided by its run (the amount that it travels left to right between those same two points). If the slope of the line doesn't travel up or down, however, the slope ends up being zero divided by the run of the line. As zero divided by any number is still zero, the overall sl…
- An undefined slope is characterized by a straight, vertical line on the graph with the X coordinate points having no existing value of slope. In this situation, the difference between the two X points equals to zero. The X coordinate, being the denominator, will yield an undefined answer despite the value of the numerator. It is a rule that anything decided by zero is an undefined value since …
Example
- As an example, assume that you have a line with a zero slope that crosses the y axis at the point (0,6). When you put the slope and the y intercept into the linear equation, you end up with y = 0x + 6, which can then be simplified to y = 6. To graph this, locate 6 on the y axis and draw a horizontal line across the graph at that point. It may seem odd to think about graphing an undefined slope. …
- Suppose a line runs through two points: P(1,2) and Q(13,8). By dividing the difference in y-coordinates by the difference in x-coordinates, one can obtain the slope of the line:The slope is .As another example, consider a line which runs through the points (4, 15) and (3, 21). Then, the slope of the line is...
Horizontal And Vertical Lines
- Sometimes, we will see equations whose graphs are horizontal lines. These are graphs in which y remains constant -- that is, in which y1 - y2 = 0 for any two points on the line: We will also see equations whose graphs are vertical lines. These are graphs in which x remains constant -- that is, in which x1 - x2 = 0 for any two points on the line:
- Since horizontal lines have no steepness, their slopes are equal to zero. Imagine a line that passes through the points (2, 3) and (4, 3). The 'change in y' is zero, since 3 - 3 = 0. Zero divided by any other number equals zero, so that is the slope.For vertical lines, the slope is undefined. For instance, a line that passes through the points (5, 7) and (5, 3) would have a 'change in x' of zero…
Calculus
- The concept of a slope is central to differential calculus. For non-linear functions, the rate of change varies along the curve. The derivative of the function at a point is the slope of the line tangent to the curve at the point, and is thus equal to the rate of change of the function at that point. If we let Δx and Δy be the distances (along the x and y axes, respectively) between two poi…
Algebra And Geometry
- Examples
For example, consider a line running through the points (2,8) and (3,20). This line has a slope, m, of ( 20 − 8 ) ( 3 − 2 ) = 12. {\displaystyle {\frac {(20-8)}{(3-2)}}\;=12.}
- The greater the absolute value of a slope, the steeper the line. A horizontal line has slope 0, a 45° rising line has a slope of +1, and a 45° falling line has a slope of -1. A vertical line's slope is undefined meaning it has \"no slope.\"The angle θ a line makes with the positive x axis is closely related to the slope m via the tangent function:andTwo lines are parallel if and only if their slope…
Practice Problems
- 1. Find the slope of a line that passes through the points (2, 1) and (6, 3).2. Find the slope of a line with the equation y = 1/2x + 3.3. Find the slope of a line that passes through the points (4, -2) and (6, -2).4. Find the slope of a line with the equation 5x + y = 7.
Analysis
- In a zero slope, the numerator is zero. This means that the Y points (Y1 and Y2) produce a difference of zero between the variables. Zero divided by any non-zero denominator will result in zero. This also results in a straight, horizontal line on the graph that neither climbs nor descends along the X axis. Between the two points, Y is not changing but X is increasing. The line is draw…
Statistics
- In statistical mathematics, the gradient of the line of best fit for a given distribution of data which is linear, numerical, and free of outliers, is usually written as b = r s y s x {\displaystyle b={\frac {rs_{y}}{s_{x}}}}, where b {\displaystyle b} is defined as the gradient (in statistics), r {\displaystyle r} is Pearson's correlation coefficient, s y {\displaystyle s_{y}} is the standard deviation of the y-val…
Usage
- Notice that the slope of a line is easily calculated by hand using small, whole number coordinates. The formula becomes increasingly useful as the coordinates take on larger values or decimal values.
- The run or the horizontal distance between two points is found by using the map's scale bar. Using a ruler we can measure the scale bar of Google Maps at bottom left corner. 17mm or 1.7cm on the map is equal to 100m in the real world. Again using a ruler, the next step is measuring the horizontal distance between point A and point B on the map: 42mm or 4.2cm. Calculating the re…
- The slope, lines, and points are plotted on a chart with integers (both positive and negative) on both the X and Y axis. The zero is placed in the center of the graph and lies in the intersection of both the Y and X axis. The system used to denote where the lines are drawn is the Cartesian system. The slope is often used in mathematical word problems especially linear equations. Slo…