
What are some uses of logarithms?
We can use laws and rules of logarithms to perform the following operations:
- Changing logarithmic functions to exponential form.
- Addition
- Subtraction
- Multiplication
- Division
- Expanding and condensing
- Solving logarithmic equations.
What are the basics of logarithms?
- Common logs have a base of 10. (for example, log 10 x). ...
- Natural logs: These are logs with a base of e. e is a mathematical constant that is equal to the limit of (1 + 1/n) n as n approaches infinity, ...
- Other Logs: Other logs have the base other than that of the common log and the E mathematical base constant. ...
What is the power rule of logarithms?
The log rules could be expressed in less formal terms as:
- Multiplication can be turned outside the log into addition and versa can be turned.
- Division can be turned outside the log into a subtraction, and vice versa.
- An exponent can be moved as a multiplier outwards on all within a log, and vice versa.
How to use logarithms?
Use smaller chart for precise numbers.
- Stay in same row
- Find small column header with fourth digit of n
- Add this to previous value
- Example: log 10 (31.62) → row 31, small column 2 → cell value 2 → 4997 + 2 = 4999.

Why do we use logarithms in math?
Logarithmic functions are important largely because of their relationship to exponential functions. Logarithms can be used to solve exponential equations and to explore the properties of exponential functions.
What is the use of logarithms in real life?
Logarithms are used for measuring the magnitude of earthquakes. Logarithms are used for measuring the noise levels in dBs (decibels). They are used to measure the pH level of chemicals. Logarithms are used in radioactivity, mainly to detect the half life of a radioactive element.
What is the purpose of studying logarithm?
Why are we studying logarithms? As you just learned, logarithms reverse exponents. For this reason, they are very helpful for solving exponential equations.
What jobs use logarithms?
Business.Computers & Technology.Criminal Justice.Education.Engineering & Science.Humanities.Nursing & Healthcare.Psychology.More items...
How logarithm helped in making life easier?
Logarithms rescue us from having to process both large numbers as well as extremely small numbers.
How do you explain logarithms?
2:583:33Logarithms, Explained - Steve Kelly - YouTubeYouTubeStart of suggested clipEnd of suggested clipIt's easier to remember logarithms log base B of some number N equals P by repeating the base raisedMoreIt's easier to remember logarithms log base B of some number N equals P by repeating the base raised to what power equals the number the base raised to what power equals the number the base raised to
What is a logarithm in simple terms?
A logarithm is the power to which a number must be raised in order to get some other number (see Section 3 of this Math Review for more about exponents). For example, the base ten logarithm of 100 is 2, because ten raised to the power of two is 100: log 100 = 2.
Why is it important to study exponential and logarithmic functions?
In particular, the study of exponential and logarithmic functions is essential since a) they are necessary concepts for Mathematics courses at the university such as 2 / 14 Calculus, Differential Equations and Complex Analysis (Weber, 2002) ; b) they are considered pivotal for learning several physical and social ...
What does logarithm tell us?
The logarithm tells us what the exponent is!
Who invented the word "logarithm"?
"Logarithm" is a word made up by Scottish mathematician John Napier (1550-1617), from the Greek word logos meaning "proportion, ratio or word" and arithmos meaning "number", ... which together makes "ratio-number" !
What does negative logarithm mean?
A negative logarithm means how many times to divide by the number.
What decimal is logarithm?
All of our examples have used whole number logarithms (like 2 or 3), but logarithms can have decimal values like 2.5, or 6.081, etc.
What does log mean in math?
Mathematicians use "log" (instead of "ln") to mean the natural logarithm. This can lead to confusion:
Is a logarithm written without a base?
Sometimes a logarithm is written without a base, like this:
What is logarithm used for?
These find its applications in surveying and celestial navigation purposes. They are also used in calculations such as measuring the loudness (decibels), the intensity of the earthquake regarding Richter scale, in radioactive decay, to find the acidity (pH= -log10 [H+]), etc.
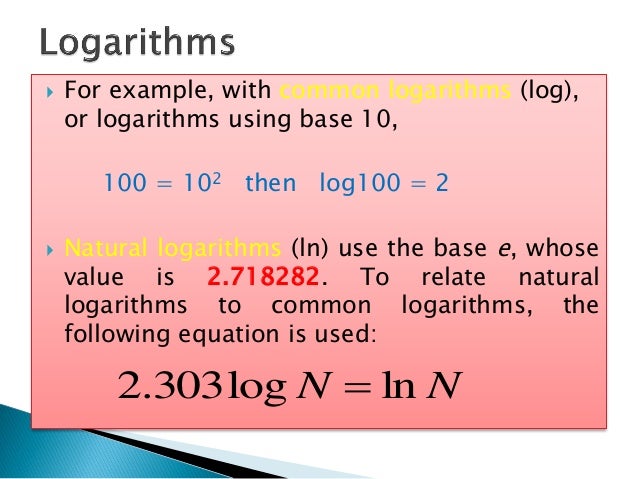
What is the common logarithm?
Common Logarithm. The common logarithm is also called the base 10 logarith ms. It is represented as log10 or simply log. For example, the common logarithm of 1000 is written as a log (1000). The common logarithm defines how many times we have to multiply the number 10, to get the required output. For example, log (100) = 2.
What is the most convenient way to express large numbers?
It is the most convenient way to express large numbers. A logarithm has various important properties that prove multiplication and division of logarithms can also be written in the form of logarithm of addition and subtraction.
What is logarithm exponentiation?
In simple words, Logarithms are the inverse process of the exponentiation. In this article, we are going to have a look at the definition, properties, and examples of logarithm in detail.
What is the power to which a number must be raised to get some other values?
A logarithm is defined as the power to which a number must be raised to get some other values. In other words, it gives the answer to the question “How many times a number is multiplied to get the other number?”. The logarithm of a number is expressed as
What are the rules for logarithms?
There are certain rules based on which logarithmic operations can be performed. The names of these rules are: 1 Product rule 2 Division rule 3 Power rule/Exponential Rule 4 Change of base rule 5 Base switch rule 6 Derivative of log 7 Integral of log
What is the base 3 logarithm of 27?
Therefore, the base 3 logarithm of 27 is 3.
What is the logarithm of a given number?
That means the logarithm of a given number x is the exponent to which another fixed number, the base b, must be raised, to produce that number x. In the simplest case, the logarithm counts the number of occurrences of the same factor in repeated multiplication; e.g. since 1000 = 10 × 10 × 10 = 103, the "logarithm base 10" of 1000 is 3, or log10 (1000) = 3. The logarithm of x to base b is denoted as logb (x), or without parentheses, logb x, or even without the explicit base, log x, when no confusion is possible, or when the base does not matter such as in big O notation .
How to calculate logarithms?
Logarithms are easy to compute in some cases, such as log10 (1000) = 3. In general, logarithms can be calculated using power series or the arithmetic–geometric mean, or be retrieved from a precalculated logarithm table that provides a fixed precision. Newton's method, an iterative method to solve equations approximately, can also be used to calculate the logarithm, because its inverse function, the exponential function, can be computed efficiently. Using look-up tables, CORDIC -like methods can be used to compute logarithms by using only the operations of addition and bit shifts. Moreover, the binary logarithm algorithm calculates lb (x) recursively, based on repeated squarings of x, taking advantage of the relation
How to get logb graph?
The graph of the logarithm function logb (x) (blue) is obtained by reflecting the graph of the function bx (red) at the diagonal line ( x = y ).
How to find logarithm of a product?
The logarithm of a product is the sum of the logarithms of the numbers being multiplied; the logarithm of the ratio of two numbers is the difference of the logarithms. The logarithm of the p -th power of a number is p times the logarithm of the number itself; the logarithm of a p -th root is the logarithm of the number divided by p. The following table lists these identities with examples. Each of the identities can be derived after substitution of the logarithm definitions#N#x = b log b x {displaystyle x=b^ {log _ {b}x}}#N#or#N#y = b log b y {displaystyle y=b^ {log _ {b}y}}#N#in the left hand sides.
What is the logarithm of a decibel?
For example, the decibel is a unit of measurement associated with logarithmic-scale quantities. It is based on the common logarithm of ratios —10 times the common logarithm of a power ratio or 20 times the common logarithm of a voltage ratio. It is used to quantify the loss of voltage levels in transmitting electrical signals, to describe power levels of sounds in acoustics, and the absorbance of light in the fields of spectrometry and optics. The signal-to-noise ratio describing the amount of unwanted noise in relation to a (meaningful) signal is also measured in decibels. In a similar vein, the peak signal-to-noise ratio is commonly used to assess the quality of sound and image compression methods using the logarithm.
What is the base of logarithm 10?
The logarithm base 10 (that is b = 10) is called the decimal or common logarithm and is commonly used in science and engineering. The natural logarithm has the number e (that is b ≈ 2.718) as its base; its use is widespread in mathematics and physics, because of its simpler integral and derivative.
What is the natural logarithm of t?
The natural logarithm of t is the shaded area underneath the graph of the function f(x) = 1/x (reciprocal of x ).
Why are logarithms useful?
Historically, they were also useful because of the fact that the logarithm of a product is the sum of the logarithms and sums are easier to calculate by hand (or to estimate by overlapping rulers as in a slide rule).
What is a logarithm?
Logarithms are a convenient way to express large numbers. (The base-10 logarithm of a number is roughly the number of digits in that number, for example.)
What are some examples of things that decay logarithmically?
For example, hot objects cool down, cold objects warm up. Things in motion experience friction and drag and gradually slow down.
What is the power of a logarithm?
In Logarithms, the power is raised to some numbers (usually, base number) to get some other number. It is an inverse function of exponential function. We know that Mathematics and Science constantly deal with the large powers of numbers, logarithms are most important and useful.
What is the logarithmic function to the base called?
The logarithmic function to the base e is called the natural logarithmic function and it is denoted by log e.
Can we have logarithmic function with fractional base?
We also can have logarithmic function with fractional base.
What are some examples of logarithms?
Sigh. We're at the typical "logarithms in the real world" example: Richter scale and Decibel. The idea is to put events which can vary drastically (earthquakes) on a single scale with a small range (typically 1 to 10). Just like PageRank, each 1-point increase is a 10x improvement in power. The largest human-recorded earthquake was 9.5; the Yucatán Peninsula impact, which likely made the dinosaurs extinct, was 13.
How many digits are in a logarithm?
Logarithms count the number of multiplications added on, so starting with 1 (a single digit) we add 5 more digits ( 10 5) and 100,000 get a 6-figure result. Talking about "6" instead of "One hundred thousand" is the essence of logarithms. It gives a rough sense of scale without jumping into details.
What does "math in the real world" mean?
Finding "math in the real world" means encountering ideas in life and seeing how they could be written with notation. Don't look for the literal symbols!
When dealing with a series of multiplications, do logarithms help "count" them?
When dealing with a series of multiplications, logarithms help "count" them, just like addition counts for us when effects are added.
Is logarithm everywhere?
Logarithms are everywhere. Ever use the following phrases?
Is logarithm so common?
Surprised that logarithms are so common? Me too. Most attempts at Math In the Real World (TM) point out logarithms in some arcane formula, or pretend we're geologists fascinated by the Richter Scale. "Scientists care about logs, and you should too. Also, can you imagine a world without zinc ?"
History
What Are logarithms?
- A logarithm is defined as the power to which a number must be raised to get some other values. It is the most convenient way to expresslarge numbers. A logarithm has various important properties that prove multiplication and division of logarithms can also be written in the form of logarithm of addition and subtraction. “The logarithm of a positive...
Logarithm Types
- In most cases, we always deal with two different types of logarithms, namely 1. Common Logarithm 2. Natural Logarithm
Logarithm Rules and Properties
- There are certain rules based on which logarithmic operations can be performed. The names of these rules are: 1. Product rule 2. Division rule 3. Power rule/Exponential Rule 4. Change of base rule 5. Base switch rule 6. Derivative of log 7. Integral of log Let us have a look at each of these properties one by one
Logarithmic Formulas
- logb(mn) = logb(m) + logb(n) logb(m/n) = logb(m) – logb(n) Logb(xy) = y logb(x) Logbm√n = logbn/m m logb(x) + n logb(y) = logb(xmyn) logb(m+n) = logbm + logb(1+nm) logb(m – n) = logbm + logb(1-n/m) Also check:
Logarithms Examples
- Example 1: Solve log 2(64) =? Solution: since 26= 2 × 2 × 2 × 2 × 2 × 2 = 64, 6 is the exponent value and log 2(64)= 6. Example 2: What is the value of log10(100)? Solution: In this case, 102 yields you 100. So, 2 is the exponent value, and the value of log10(100)= 2 Example 3: Use of the property of logarithms, solve for the value of x for log3 x= log3 4+ log37 Solution: By the additio…
Overview
In mathematics, the logarithm is the inverse function to exponentiation. That means the logarithm of a given number x is the exponent to which another fixed number, the base b, must be raised, to produce that number x. In the simplest case, the logarithm counts the number of occurrences of the same factor in repeated multiplication; e.g. since 1000 = 10 × 10 × 10 = 10 , the "logarithm bas…
Motivation
Addition, multiplication, and exponentiation are three of the most fundamental arithmetic operations. The inverse of addition is subtraction, and the inverse of multiplication is division. Similarly, a logarithm is the inverse operation of exponentiation. Exponentiation is when a number b, the base, is raised to a certain power y, the exponent, to give a value x; this is denoted
Definition
Given a positive real number b such that b ≠ 1, the logarithm of a positive real number x with respect to base b is the exponent by which b must be raised to yield x. In other words, the logarithm of x to base b is the unique real number y such that .
The logarithm is denoted "logb x" (pronounced as "the logarithm of x to base b", "the base-b logarithm of x", or most commonly "the log, base b, of x").
Logarithmic identities
Several important formulas, sometimes called logarithmic identities or logarithmic laws, relate logarithms to one another.
The logarithm of a product is the sum of the logarithms of the numbers being multiplied; the logarithm of the ratio of two numbers is the difference of the logarithms. The logarithm of the p-th power of a number is p times the logarithm of the number itself; the logarithm of a p-th root is th…
Particular bases
Among all choices for the base, three are particularly common. These are b = 10, b = e (the irrational mathematical constant ≈ 2.71828), and b = 2 (the binary logarithm). In mathematical analysis, the logarithm base e is widespread because of analytical properties explained below. On the other hand, base-10 logarithms are easy to use for manual calculations in the decimal number syste…
History
The history of logarithms in seventeenth-century Europe is the discovery of a new function that extended the realm of analysis beyond the scope of algebraic methods. The method of logarithms was publicly propounded by John Napier in 1614, in a book titled Mirifici Logarithmorum Canonis Descriptio (Description of the Wonderful Rule of Logarithms). Prior to Napier's invention, there had been other techniques of similar scopes, such as the prosthaphaeresis or the use of tables of pro…
Logarithm tables, slide rules, and historical applications
By simplifying difficult calculations before calculators and computers became available, logarithms contributed to the advance of science, especially astronomy. They were critical to advances in surveying, celestial navigation, and other domains. Pierre-Simon Laplace called logarithms
"...[a]n admirable artifice which, by reducing to a few days the labour of many …
Analytic properties
A deeper study of logarithms requires the concept of a function. A function is a rule that, given one number, produces another number. An example is the function producing the x-th power of b from any real number x, where the base b is a fixed number. This function is written as f(x) = b . When b is positive and unequal to 1, we show below that f is invertible when considered as a function …