
Logarithmic Functions
| 1. | What are Logarithmic Functions? |
| 2. | Domain and Range of Log Functions |
| 3. | Logarithmic Graph |
| 4. | Graphing Logarithmic Functions |
| 5. | Properties of Logarithmic Functions |
What are the asymptotes of a logarithmic function?
The inverse of a logarithmic function is an exponential function and vice versa. logarithm: The logarithm of a number is the exponent by which another fixed value, the base, has to be raised to produce that number. asymptote: A line that a curve approaches arbitrarily closely. Asymptotes can be horizontal, vertical or oblique.
What is the zero of the logarithmic function?
log p 1 = 0. Other properties of logarithmic functions include: The bases of an exponential function and its equivalent logarithmic function are equal. The logarithms of a positive number to the base of the same number are equal to 1. log a a = 1. Logarithms of 1 to any base are 0. log a 1 = 0. Log a 0 is undefined
What is the domain and range for the function?
- The domain and range of a function is the set of all possible inputs and outputs of a function respectively.
- The domain and range of a function y = f (x) is given as domain= {x ,x∈R }, range= {f (x), x∈Domain}.
- The domain and range of any function can be found algebraically or graphically.
How do you find the domain of a linear function?
Determining the Domain and Range Modeled by a Linear Function. To determine the domain of a given situation, identify all possible x -values, or values of the independent variable. To determine the range of a given situation, identify all possible y -values, or values of the dependent variable. Example 1. A clown at a birthday party can blow up ...

What is the range for logarithmic functions?
set of all real numbersHence, the range of a logarithmic function is the set of all real numbers. Thus: The domain of log function y = log x is x > 0 (or) (0, ∞). The range of any log function is the set of all real numbers (R)
How do you find the range of a logarithmic equation?
2:265:23Finding the domain and range of a logarithmic function - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd the range is the y-coordinate of the lowest. Point to the y-coordinate of the highest.MoreAnd the range is the y-coordinate of the lowest. Point to the y-coordinate of the highest.
Do all logarithmic functions have the same range?
The domain of logarithmic functions is equal to all real numbers greater or less than the vertical asymptote. The range of exponential functions is always equal to all real numbers since we have no restrictions on the output values.
How do you determine the domain and range of a logarithmic function?
Finding the Domain of a Logarithmic Function In the last section we learned that the logarithmic function y=logb(x) is the inverse of the exponential function y=bx. So, as inverse functions: The domain of y=logb(x) is the range of y=bx: (0,∞). The range of y=logb(x) is the domain of y=bx: (−∞,∞).
How find the range of a function?
Overall, the steps for algebraically finding the range of a function are:Write down y=f(x) and then solve the equation for x, giving something of the form x=g(y).Find the domain of g(y), and this will be the range of f(x). ... If you can't seem to solve for x, then try graphing the function to find the range.
What is the domain of all logarithmic functions?
The domain of a logarithmic function is real numbers greater than zero, and the range is real numbers.
What is the range of exponential function?
The domain of exponential functions is all real numbers. The range is all real numbers greater than zero. The line y = 0 is a horizontal asymptote for all exponential functions. When a > 1: as x increases, the exponential function increases, and as x decreases, the function decreases.
What is a function range and domain?
Domain and Range. The domain of a function is the set of values that we are allowed to plug into our function. This set is the x values in a function such as f(x). The range of a function is the set of values that the function assumes. This set is the values that the function shoots out after we plug an x value in.
What is the range of E X?
(0,∞)The domain of exp(x) is all real numbers and the range is (0,∞).
How do you find the domain and range of a logarithmic asymptote?
0:004:19Determine the Domain, Range, and Asymptote of a Log Function yYouTubeStart of suggested clipEnd of suggested clipAnd b can't be one the log equation y equals log base b of x is equivalent to the exponentialMoreAnd b can't be one the log equation y equals log base b of x is equivalent to the exponential equation b raised to the power of y equals x the domain is the open interval from zero to infinity.
What is the range of this exponential function?
The range is all real numbers greater than zero. The line y = 0 is a horizontal asymptote for all exponential functions. When a > 1: as x increases, the exponential function increases, and as x decreases, the function decreases.
What is the range of E X?
(0,∞)The domain of exp(x) is all real numbers and the range is (0,∞).
How many parts does a logarithm have?
Usually a logarithm consists of three parts.
What is the domain of a logarithm?
Fact to be known about domain of logarithm functions. A very important fact that we have to know about the domain of a logarithm to any base is, "A logarithmic function is defined only for positive values of argument". For example, if the logarithmic function is. y = log10x,
What is the power of a logarithm?
In Logarithms, the power is raised to some numbers (usually, base number) to get some other number. It is an inverse function of exponential function. We know that Mathematics and Science constantly deal with the large powers of numbers, logarithms are most important and useful.
What is the logarithmic function to the base called?
The logarithmic function to the base e is called the natural logarithmic function and it is denoted by log e.
Can we have logarithmic function with fractional base?
We also can have logarithmic function with fractional base.
What Are Logarithmic Functions?
The logarithmic function is derived from an exponential function. Some of the non-integral exponent values can be calculated easily with the use of logarithmic functions. Finding the value of x in the exponential expressions 2 x = 8, 2 x = 16 is easy, but finding the value of x in 2 x = 10 is difficult. Here we can use logarithmic functions to transform 2 x = 10 into logarithmic form as log210 = x l o g 2 10 = x and then find the value of x. The logarithm counts the numbers of occurrences of the base in repeated multiples. The formula for transforming an exponential function into a logarithmic function is as follows.
How to find logarithmic function graph?
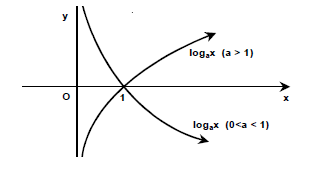
The graph of logarithmic function y = log x can be obtained by plotting the logx value on the x-axis and the y value on the y-axis. The graph of logx does not pass through the origin, and the graph is similar to the square root graph.
What is the derivation of a logarithmic function?
The derivation of the logarithmic function gives the slope of the tangent to the curve representing the logarithmic function. The functions within the logarithm can be a simple variable or a trigonometric ratio. The derivative taken for the logarithmic function gives the inverse of the logarithmic function as an answer. The formula for the derivative of the logarithmic function is as follows.
What is the exponent of a logarithm?
The logarithm of any number X if interpreted as an exponential form, is the exponent to which the base of the logarithm should be raised, to obtain the number X. Here we shall aim at knowing more about logarithmic functions, type of logarithms, the graph of the logarithmic function, and the properties of logarithms.
How to find the domain of a logarithmic function?
The domain of a logarithm function is similar to a square root function. The domain of the logarithmic function is plotted on the x-axis and the range is plotted with respect to the y-axis. Since the logarithm of 1 to any base ( logn1 = 0 log n#N##N#1 = 0) is equal to 0, the logarithmic function at the domain value of 1 cuts the x-axis. Also, the logarithm of zero does not exist, and hence the graph does not pass through the origin.
What are the two types of logarithms?
The logarithms are broadly classified into two types, based on the base of the logarithms. We have natural logarithms and common logarithms . Natural logarithms are logarithms to the base 'e', and common logarithms are logarithms to the base of 10. Further logarithms can be calculated with reference to any base, but are often calculated for the base of either 'e' or '10'. The natural logarithms are written as loge x log e#N##N#x , and the common logarithms are written as log10x log 10#N##N#x. To obtain the value of x from natural logarith ms, it is equal to the power to which e has to be raised to obtain x, ie e1.6.9 = 5 e 1.6.9 = 5.
What is the value of e in logarithms?
The value of e = 2.718281828459, but is often written in short as e = 2.718. Also, the above formulas help in the interconversion of natural logarithms and common logarithms.
What Is The Range Of A Logarithmic Function?
The range of the basic logarithmic function f (x) = log b (x) is the set of all real numbers. We can see this by picking a y value in the range (any real number) and solving for x:
What are the most important things to remember about logarithmic functions?
So, what are the most important things to remember about logarithmic functions? The function logb(x) has no y intercept and no horizontal asymptote, but it has a vertical asymptote at x = 0. It does not have symmetry, but it is the mirror image of its inverse function bx reflected across the line y = x. Its domain is positive real numbers and its range is all real numbers.
Do Logarithmic Functions Have Horizontal Asymptotes?
A logarithmic function does not have a horizontal asymptote. However, it does have a vertical asymptote (more on this shortly).
What is the vertical asymptote of a logarithmic function?
A logarithmic function has a vertical asymptote. The basic logarithmic function f (x) = log b (x) for b > 0 has its vertical asymptote at x = 0.
Why are logarithms used in science?
Logarithms are often used in science to model things like sound (decibels), earthquake strength (Richter scale), and pH (for the strength of acids or bases). Logarithmic functions have some interesting properties when graphed.
What is the function of f (x)?
f (x) = logb(x) [the definition of the basic logarithmic function]
Does a logarithmic function have symmetry?
A logarithmic function itself does not have symmetry. However, there is some symmetry to be found if you consider a logarithmic function f (x) = log b (x) and its inverse function f -1 (x) = b x (the exponential function with a base of b).
What is the domain of a logarithmic function?
(Since the logarithmic function is the inverse of the exponential function, the domain of logarithmic function is the range of exponential function , and vice versa.) 3. The function is continuous and one-to-one. 4.
What is the natural logarithm?
The logarithm with base e is called the natural logarithm. It is denoted by ln x. The natural logarithmic function, y = ln x, is the inverse of the natural base exponential function, y = e x.
What is the inverse of exponential function?
Observe that the logarithmic function f ( x) = log b x is the inverse of the exponential function g ( x) = b x. It has the following properties.
What is the domain of a logarithmic function?
Therefore, the domain of the logarithmic function y = log b x is the set of positive real numbers and the range is the set of real numbers.
What is the inverse of log 2?
A simple logarithmic function y = log 2 x where x > 0 is equivalent to the function x = 2 y . That is, y = log 2 x is the inverse of the function y = 2 x .
What is the graph of the basic exponential function?
In general, the graph of the basic exponential function y = a x drops from ∞ to 0 when 0 < a < 1 as x varies from − ∞ to ∞ and rises from 0 to ∞ when a > 1 .
What is the domain of a function?
Recall that the domain of a function is the set of input or x -values for which the function is defined, while the range is the set of all the output or y -values that the function takes.
Is the domain of a function set of real numbers?
The function is defined for all real numbers. So, the domain of the function is set of real numbers.
Is logarithmic function inverse of exponential?
Remember that since the logarithmic function is the inverse of the exponential function, the domain of logarithmic function is the range of exponential function, and vice versa.
Name of The Parts of A Logarithm
Fact to Be Known About Domain of Logarithm Functions
- A very important fact that we have to know about the domain of a logarithm to any base is, "A logarithmic function is defined only for positive values of argument" For example, if the logarithmic function is y = log10x, then the domain is x > 0 or (0, +∞)
Domain of Y = Log₁₀
- In the logarithmic function y = log10(x), the argument is 'x'. From the fact explained above, argument must always be a positive value. So, the values of x must be greater than zero. Therefore, the domain of the above logarithmic function is x > 0 or (0, +∞)
Some More Stuff on Domain of Logarithmic Functions
- Let us consider the logarithmic functions which are explained above. y = log10(x) y = log10(x+a) y = log10(x-a) y = log10(kx) y = log10(kx+a) y = log10(kx-a) Domain is already explained for all the above logarithmic functions with the base '10'. In case, the base is not '10' for the above logarithmic functions, domain will remain unchanged. For example, in the logarithmic function y …
Range of Logarithmic Functions
- The table shown below explains the range of y = log10(x). That is, "All Real Numbers" Here, we may think that if the base is not 10, what could be the range of the logarithmic functions? Whatever base we have for the logarithmic function, the range is always "All Real Numbers" For the base other than '10', we can define the range of a logarithmic f...