What is the difference between central angle and arc length?
Dec 14, 2015 · Since arc UF is 20 degrees, central angle FVU is 20 degrees. Likewise, central angle UVN is equal to 180 degrees based upon the arc it intercepts. As we examine the diagram further, we can see that there is an angle relationship that is important to recognize: angle UVF + angle FVN = 180 degrees.
What is the relationship between angles and arcs in a circle?
The arc measure equals the corresponding central angle measure, in radians. That's why radians are natural: a central angle of one radian will span an arc exactly one radius long. What is the arc length formula? Calculate the arc length according to the formula above: L = r * Θ = 15 * π/4 = 11.78 cm . Calculate the area of a sector: A = r² * Θ / 2 = 15² * π/4 / 2 = 88.36 cm² . You can …
What is the relationship between arc length and radius in radians?
Feb 10, 2020 · What is the relationship between an arc and its central angle? Central angles are subtended by an arc between those two points, and the arc length is the central angle of a circle of radius one (measured in radians). The central angle is …
What are the theorems about arcs and central angles?
It is the central angle's ability to sweep through an arc of 360 degrees that determines the number of degrees usually thought of as being contained by a circle. Central angles are angles formed by any two radii in a circle. The vertex is the center of the circle. In Figure 1, ∠ AOB is a central angle. Figure 1 A central angle of a circle. Arcs. An arc of a circle is a continuous portion of …

What is the relationship between the central angle and arc?
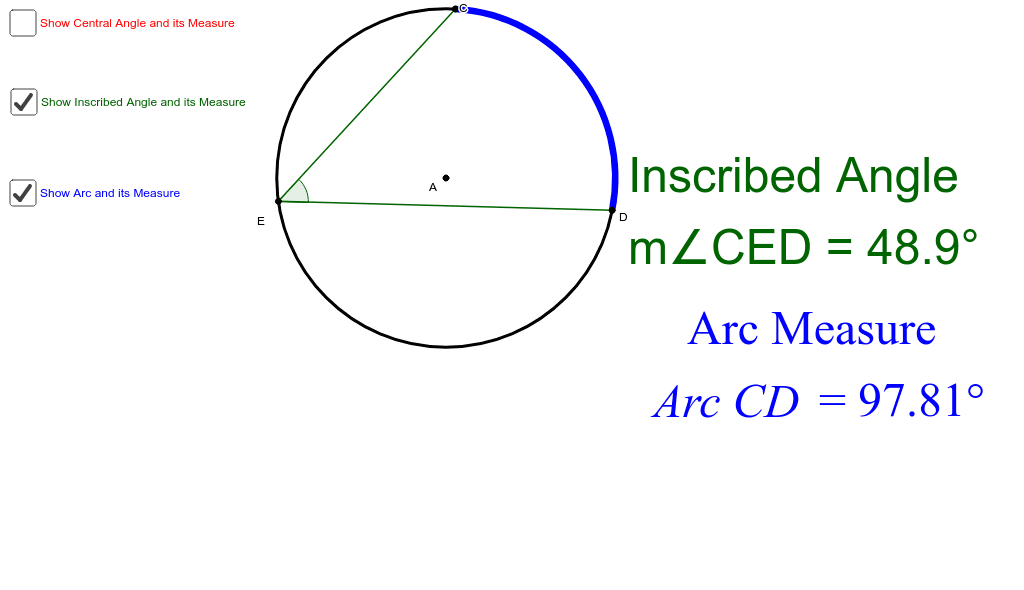
The measure of the central angle is the same as the measure of the arc it intercepts. Inscribed Angles: Angles with the vertex located on the circumference of the circle. The measure of the inscribed angle is half the measure of the arc it intercepts.
What is the relationship between a central angle and its arc Quizizz?
What is the relationship between a central angle and its arc? The angle is half of the arc.
What is the relationship between a central angle and its opposite arc?
A central angle of a circle is defined as the angle whose vertex totally lies on the center of the circle. Also, the opposite arc formed is known as the corresponding arc related to the given central angle.
Is a central angle equal to its arc?
1:3113:44Central Angles, Circle Arcs, Angle Measurement, Major Arcs vs Minor ...YouTubeStart of suggested clipEnd of suggested clipSo make sure you know the difference between a segment and the measure of an arc. Now arc Eady is itMoreSo make sure you know the difference between a segment and the measure of an arc. Now arc Eady is it a minor arc or major arc a minor arc has an angle that's less than 180 a major art has an angle
What is the relationship between two tangents that share a common endpoint outside of the circle?
If two tangent segments to a circle share a common endpoint outside the circle, then the two segments are congruent.
What is the relationship between arc and inscribed angles of a circle?
Inscribed angles are angles whose vertices are on a circle and that intersect an arc on the circle. The measure of an inscribed angle is half of the measure of the intercepted arc and half the measure of the central angle intersecting the same arc. Inscribed angles that intercept the same arc are congruent.
Which angles intercept the same arc?
Inscribed angles that intercept the same arc are congruent. This is called the Congruent Inscribed Angles Theorem and is shown below.Feb 24, 2012
What is the relationship between the measure of a central angle and the measure of its intercepted arc?
What is the relationship between central angles and their arcs? The measure of a central angle is equal to the measure of its intercepted arc. A chord is a segment that has its endpoints on a circle.
What is the central angle equal to?
A central angle is an angle with its vertex at the center of a circle, with its sides containing two radii of the circle. In the figure above, ∠PZQ,∠QZR , and ∠RZP are central angles. Sum of Central Angles: The sum of the measures of the central angles of a circle with no points in common is 360° .
How do you find the arc angle of a central angle?
A central angle is defined as the angle subtended by an arc at the center of a circle. The radius vectors form the arms of the angle. A central angle is calculated using the formula: Central Angle = Arc length(AB) / Radius(OA) = (s × 360°) / 2πr, where 's' is arc length, and 'r' is radius of the circle.
Interior Intersections
If two secants or chords intersect inside a circle, then the measure of the angle formed is equal to half the sum of the measures of the intercepted arcs.
On the Circle Intersections
If a secant and a tangent intersect at the point of tangency, then the measure of each angle formed is equal to half the measure of its intercepted arc.
Exterior Intersections
If secants and/or tangents intersect on the exterior of a circle, then the measure of the angle formed is equal to half the difference of the intercepted arcs.

Interior Intersections
- If two secants or chords intersect inside a circle, then the measure of the angle formed is equal to half the sum of the measures of the intercepted arcs. In the diagram above, m∠1 = 1/2 ⋅ (m∠arc AD + m∠arc BC) m∠2 = 1/2 ⋅ (m∠arc AB + m∠arc CD) Example 1 : Find m∠AED. Solution : m∠AED = 1/2 ⋅ (m∠arc AD + m∠arc BC) Substitute. m∠AED = 1/2 ⋅ (45°+ 10...
on The Circle Intersections
- If a secant and a tangent intersect at the point of tangency, then the measure of each angle formed is equal to half the measure of its intercepted arc. In the diagram above, m∠1 = 1/2 ⋅ m∠arc AB m∠2 = 1/2 ⋅ m∠arc ACB Example 4 : Find m∠DEG. Solution : m∠DEG = 1/2 ⋅ m∠arc EHG Substitute. m∠DEG = 1/2 ⋅ 308° m∠DEG = 154° Example 5 : Find m∠arc KNM. Solution : F…
Exterior Intersections
- If secants and/or tangents intersect on the exterior of a circle, then the measure of the angle formed is equal to half the difference of the intercepted arcs.
Two Tangents
- m∠A = 1/2 ⋅ (m∠arc BDC - m∠arc BC) Example 6 : Find m∠KLM. Solution : m∠KLM = 1/2 ⋅ (m∠arc JN - m∠arc KM) Substitute. m∠KLM = 1/2 ⋅ (140°- 66°) m∠KLM = 1/2 ⋅ 74° m∠KLM = 37° Example 7 : Find m∠BCD. Solution : In the circle above, m∠arc BD + m∠arc DE + m∠arc EA = 90° Substitute. m∠arc BD + 94°+ 67°= 180° m∠arc BD + 161°= 180° Subtract 161° from each sid…