
A square root transformation can be useful for:
- Normalizing a skewed distribution
- Transforming a non-linear relationship between 2 variables into a linear one
- Reducing heteroscedasticity of the residuals in linear regression
- Focusing on visualizing certain parts of your data
What are the steps in solving square roots?
Use Square Roots in Applications
- Read the problem.
- Identify what we are looking for. The speed of a car.
- Name what we are looking for. Let s = the speed.
- Translate into an equation by writing the appropriate formula. Substitute the given information.
- Solve the equation. Round to 1 decimal place.
- Check the answer in the problem. ...
- Answer the question with a complete sentence.
How do you calculate calculate square root?
Visitor comments
- Estimate the square root to at least 1 digit.
- Divide this estimate into the number whose square root you want to find.
- Find the average of the quotient and the divisor. The result becomes the new estimate.
How do you approximate the square root?
You'll first have to keep in mind the steps to finding square roots and they are:
- Estimate: Get as close as possible to the number you're trying to square root by finding two perfect square roots that gives a close number.
- Divide: Divide your number by one of the square roots you've chosen from the previous step.
- Average: Take the average of step 2 and the root.
What is a square root, and how is it used?
Why its importnt and how we use in real life?
- square root → 2nd degree equations → functions & graphing
- square root → Pythagorean theorem → trigonometry
- square root → fractional exponents → functions & graphing
- square root → irrational numbers → real numbers If you play video games you use square roots, because the square root function is used in collision detection for polygon objects. ...
When can I always use the square root method?
This method is generally used on equations that have the form ax2 = c or (ax + b)2 = c, or an equation that can be re-expressed in either of those forms. To solve an equation by using the square root property, you will first isolate the term that contains the squared variable.
How do you know when you can use the square root method instead of factoring?
Choosing the Best Method to Use If there's a common factor, divide both sides of the equation by that number to simplify the situation. If b = 0 (no bx term), go to the square root method. (if c is positive, there are no solutions). If c = 0, then one of your solutions is x = 0.
Can we use square root method in all quadratic equation?
Not all quadratic equations are solved by immediately taking the square root. Sometimes we have to isolate the squared term before taking its root. For example, to solve the equation 2 x 2 + 3 = 131 2x^2+3=131 2x2+3=1312, x, squared, plus, 3, equals, 131 we should first isolate x 2 x^2 x2 .
How do you use the square root method?
1:182:29Solving Quadratic Equations by the Square Root Method - YouTubeYouTubeStart of suggested clipEnd of suggested clipAlways use plus or minus. So on the right side of the equation. We have plus or minus root 18MoreAlways use plus or minus. So on the right side of the equation. We have plus or minus root 18 finally always break down your square root as much as possible 18 is 2 times 9 and 9 is 3 times 3.
When should you use the quadratic formula method?
This formula is very helpful for solving quadratic equations that are difficult or impossible to factor, and using it can be faster than completing the square. The Quadratic Formula can be used to solve any quadratic equation of the form ax2 + bx + c = 0.
How do you know which factoring method to use?
0:1311:58Choosing a Factoring Method - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd that's what we should look for first is there anything that I can pull out of the entireMoreAnd that's what we should look for first is there anything that I can pull out of the entire polynomial to make it simpler. That's where we should start then.
How do you know when to use factoring?
Factoring is usually faster and less prone to arithmetic mistakes (if you are working by hand). If the coefficient of x2 and the coefficient with no x element have relatively few factors, time invested in attempting to factor the quadratic is usually worthwhile.
How can square roots be used to solve quadratic equations?
Key Strategy in Solving Quadratic Equations using the Square Root Method. The general approach is to collect all x2 terms on one side of the equation while keeping the constants to the opposite side. After doing so, the next obvious step is to take the square roots of both sides to solve for the value of x.
How can you use square roots to solve quadratic equations?
Extracting Square RootsStep 1: Express the quadratic equation in standard form.Step 2: Factor the quadratic expression.Step 3: Apply the zero-product property and set each variable factor equal to 0.Step 4: Solve the resulting linear equations.
Can all quadratic equations be solved by quadratic formula?
In algebra, all quadratic problems can be solved by using the quadratic formula.
Where can we use quadratic equations in real life?
Quadratic equations are used in many real-life situations such as calculating the areas of an enclosed space, the speed of an object, the profit and loss of a product, or curving a piece of equipment for designing.
Can all quadratic equations be solved by factoring?
No, not all quadratic equations can be solved by factoring. This is because not all quadratic expressions, ax2 + bx + c, are factorable.
Can you square root a negative number?
Negative numbers doesn't have real square roots since a square is either positive or 0.
How do you know if an equation is quadratic or not?
In other words, if you have a times the square of the expression following b plus b times that same expression not squared plus c equal to 0, you have an equation that is quadratic in form. If we substitute what is in the ( ) with a variable like t, then the original equation will become a quadratic equation.
What equation is not quadratic?
An equation is claimed to be not quadratic when there are more than two solutions in an equation that satisfy it. Basically, the absence of the square term in the equation makes it non-quadratic.
Which among the methods can be used to solve all types of quadratic equations?
Answer and Explanation: The method that can be used to solve all quadratic equations is the quadratic formula.
What is the most convenient method in solving the quadratic equation?
Completing the square – can be used to solve any quadratic equation. It is a very important method for rewriting a quadratic function in vertex form. Quadratic formula – is the method that is used most often for solving a quadratic equation.
Which among the methods can be used to solve all types of quadratic equations?
Answer and Explanation: The method that can be used to solve all quadratic equations is the quadratic formula.
What is the difference between completing the square and quadratic formula?
When you complete the square, you change the equation so that the left side of the equation is a perfect square trinomial. That's just a fancy way of saying that completing the square is a technique that transforms your quadratic equation from an equation that can't be factored into one that can.
Which of the four methods can be used to solve any type of quadratic equations?
Answer: There are various methods by which you can solve a quadratic equation such as: factorization, completing the square, quadratic formula, and graphing. These are the four general methods by which we can solve a quadratic equation.
What is a square root in Maths?
A square root is a value that gives the original number when multiplied by itself.
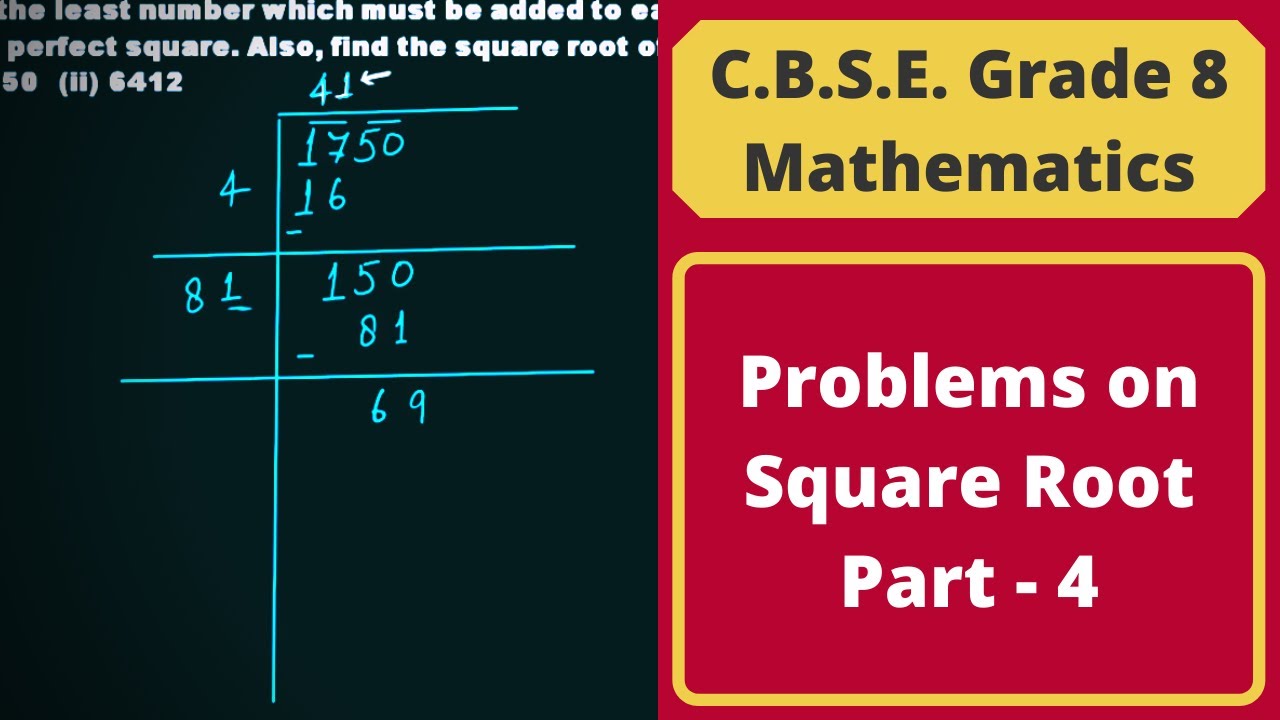
How to find square root?
To find the square root, we can use four methods: Prime factorisation, Repeated Subtraction, Long division and Estimation method.
What is the meaning of this symbol ‘√’?
‘√’ is a radical symbol used to denote square root of a number.
What are squares and square roots?
The square root is just the opposite process of squaring a number. Suppose we square a number n, such as n^2, then the square root of n^2 is equal...
How to find the square root of perfect squares?
By using prime factorisation, we can find the square root of perfect squares.
How to find the square root of imperfect squares?
By using the long division method, we can find the square root of imperfect squares.
How many square root operations do you need to solve for x?
Since the x -term is being raised to the second power twice, that means, I need to perform two square root operations in order to solve for x.
How many values of x can satisfy the original quadratic equation?
The solutions to this quadratic equations are x = 4, x = - ,4, x = 2, and x = - ,2. Yes, we have four values of x that can satisfy the original quadratic equation.
How to eliminate parentheses in math?
So my first step is to eliminate both of the parentheses by applying the distributive property of multiplication. Once they are gone, I can easily combine like terms. Keep the {x^2} terms to the left, and constants to the right. Finally, apply square root operation in both sides and we’re done!
Is there an x squared term after the first square root?
There’s an x -squared term left after the first application of square root.
How do Find Square Root of Numbers?
To find the square root of any number, we need to figure out whether the given number is a perfect square or an imperfect square. If the number is a perfect square, such as 4, 9, 16, etc., then we can factorize the number by prime factorisation method . If the number is an imperfect square, such as 2, 3, 5, etc., then we have to use a long division method to find the root.
What is the square root symbol?
The square root symbol is usually denoted as ‘ √’. It is called a radical symbol. To represent a number ‘x’ as a square root using this symbol can be written as:#N#‘ √x ‘
How to solve a radical equation?
It is also called the radical equation. To solve the radical equation, we need to follow the below steps: Isolate the square root to one of the sides (L.H.S or R.H.S).
What is the radical symbol for the square root of a number?
Suppose, x is the square root of y, then it is represented as x=√y or we can express the same equation as x2 = y. Here,’√’is the radical symbol used to represent the root of numbers. The positive number, when multiplied by itself, represents the square of the number. The square root of the square of a positive number gives the original number.
How many times is the square root of 25?
Since, the subtraction is done for 5 times, hence the square root of 25 is 5.
What are the properties of a square root?
Some of the important properties of the square root are as follows: If a number is a perfect square number, then there exists a perfect square root. If a number ends with an even number of zeros (0’s), then it can have a square root. The two square root values can be multiplied.
What is the square root of a negative number?
Note: The square root of a negative number represents a complex number.
How many square roots does every positive number have?
Note that every positive number has two square roots: a positive square root and a negative square root. For example, both and , when squared, equal . Therefore, this equation has two solutions.
What is the inverse of taking the square?
The inverse operation of taking the square is taking the square root. However, unlike the other operations, when we take the square root we must remember to take both the positive and the negative square roots.
Why is it important to keep things factored in quadratic equations?
Therefore, it's actually helpful in quadratic equations to keep things factored, because this allows us to take the square root.
When do you need a square root transformation?
Another situation where you might need a square root transformation is when the distribution of the residuals (a.k.a. error terms) of a regression model is not normal. In this case, using a square root transformation on the outcome variable (dependent variable) may yield normally distributed residuals.
What happens when you apply a square root transformation to a variable?
When you apply a square root transformation to a variable, high values get compressed and low values become more spread out. Log transformation does the same thing but more aggressively.
How to normalize left skewed distributions?
In order to normalize left skewed distributions, you can try a quadratic, cube or exponential transformation.
How to fix nonlinear relationship between X and Y?
One solution to fix a non-linear relationship between X and Y, is to try a log or square root transformation.
Does square root transformation fix skews?
The square root transformation will not fix all skewed variables. Variables with a left skew, for instance, will become worst after a square root transformation. As discussed above, this is a consequence of compressing high values and stretching out the ones on the lower end.
When to use parametric hypothesis test?
When you want to use a parametric hypothesis test (especially if you have a small sample size), you need to ensure that the variable under study is normally distributed.
Should you mention any transformation before plotting?
Be careful, however, to clearly mention and defend any transformation or change you make to your data before plotting. Otherwise, you may be accused of misleading your readers.
What does completing the square do?
Both the quadratic formula and completing the square will let you solve any quadratic e quation. ( In this post, we’re specifically focusing on completing the square.) When you complete the square, you change the equation so that the left side of the equation is a perfect square trinomial. That’s just a fancy way of saying that completing the square is a technique that transforms your quadratic equation from an equation that can’t be factored into one that can.
Why is it important to practice quadratics?
That’s why it’s important to work as many quadratic equations as you need to in order to feel comfortable solving these types of problems. Luckily for you, completing the square can be used to solve any quadratic equation, so as long as the practice questions are quadratics, you can use them!
What Is a Quadratic Equation?
In order to understand how to complete the square, you first have to know how to identify a quadratic equation. That’s because completing the square only applies to quadratic equations!
What is factoring in math?
Factoring, on the other hand, involves breaking the quadratic equation into two linear equations that are both equal to zero. Unfortunately, trying to factor this equation doesn’t result in two linear equations! Both the quadratic formula and completing the square will let you solve any quadratic equation.
What is the missing number in a square called?
(This missing number is called the constant. ) By doing that, we’ll be able to factor the equation like normal.
Why do we add 9 to the right side of an equation?
So because we’re adding 9 to our equation to make it a perfect square, we also have to add 9 to the right side of the equation to keep things balanced. If you forget to add the new constant to the right side of the equation, you won’t get the right answer!
Can you see quadratics on the SAT?
It’s pretty much a guarantee that you’ll see quadratic equations on the SAT and ACT. But they can be tricky to tackle, especially since there are multiple methods you can use to solve them.
When to use square root?
Now using the square root (e.g., sqrt (x)) is a transformation that has a moderate effect on distribution shape. It is generally used to reduce right skewed data. Finally, the square root can be applied on zero values and is most commonly used on counted data.
Why does np.sqrt have value error?
More exactly, if you get the “ValueError: Data must be positive” while using either np.sqrt (), np.log () or SciPy’s boxcox () it is because your dependent variable contains negative numbers. To solve this, you can reverse the distribution.
How do you convert left skewed data?
One way to handle left (negative) skewed data is to reverse the distribution of the variable. In Python, this can be done using the following code:
What are some examples of statistical tests that can be used to test whether data is normally distributed?
For example, we can carry out statistical tests of normality such as the Shapiro-Wilks test. It is worth noting, however, that most of these tests are susceptible for the sample size. That is, even small deviations from normality will be found using e.g. the Shapiro-Wilks test.
Why is it important to have a normal shape?
That the data we have is of normal shape (also known as following a Bell curve) is important for the majority of the parametric tests we may want to perform. This includes regression analysis, the two-sample t-test, and Analysis of Variance that can be carried out in Python, to name a few.
