Rational and Irrational numbers both are real numbers but different with respect to their properties. A rational number is the one which can be represented in the form of P/Q where P and Q are integers and Q ≠ 0.
How can you tell if numbers rational or irrational?
Rational Numbers: The real numbers which can be represented in the form of the ratio of two integers, say P/Q, where Q is not equal to zero are called rational numbers. Irrational Numbers: The real numbers which cannot be expressed in the form of the ratio of two integers are called irrational numbers.
Can a real number be an irrational number?
Therefore, no (real) number could be simultaneously a rational number and an irrational number, just as no subset A of a non-empty set X could have anything in common with its own complementary set X-A, because, by definition, what is in X-A is not in A, and what is in A is not in X-A.
How can you tell if a number is irrational?
Let’s summarize a method we can use to determine whether a number is rational or irrational. If the decimal form of a number. stops or repeats, the number is rational. does not stop and does not repeat, the number is irrational.
Do real numbers include irrational numbers?
We know that real numbers include rational numbers and irrational numbers. Thus, there does not exist any real number that is neither rational nor irrational. It simply means that if we pick up any number from R, it is either rational or irrational. Any number which can be defined in the form of a fraction p/q is called a rational number.

Are all irrational numbers real?
In Mathematics, all the irrational numbers are considered as real numbers, which should not be rational numbers. It means that irrational numbers cannot be expressed as the ratio of two numbers.
Is rational always a real number?
The number is rational (it's written as a ratio of two integers) but it's also real. All rational numbers are also real numbers. The correct answer is rational and real numbers, because all rational numbers are also real.
Are all numbers real numbers?
Real numbers are, in fact, pretty much any number that you can think of. This can include whole numbers or integers, fractions, rational numbers and irrational numbers. Real numbers can be positive or negative, and include the number zero.
What are some examples of irrational numbers?
Examples of Irrational Numbers 1 5/0 is an irrational number, with the denominator as zero. 2 π is an irrational number which has value 3.142…and is a never-ending and non-repeating number. 3 √2 is an irrational number, as it cannot be simplified. 4 0.212112111…is a rational number as it is non-recurring and non-terminating.
What is rational number?
Rational numbers are numbers which can be expressed as a fraction and also as positive numbers, negative numbers and zero. It can be written as p/q, where q is not equal to zero. The word rational is derived from the word ‘ratio’, which actually means a comparison of two or more values or integer numbers and is known as a fraction.
What is the difference between rational and irrational numbers?
Rational numbers are the numbers that can be expressed in the form of a ratio (P/Q & Q≠0) and irrational numbers cannot be expressed as a fraction. But both the numbers are real numbers and can be represented in a number line.
What are some rules based on arithmetic operations such as addition and multiplication performed on the rational number
Here are some rules based on arithmetic operations such as addition and multiplication performed on the rational number and irrational number. #Rule 1: The sum of two rational numbers is also rational. #Rule 2: The product of two rational number is rational.
Can irrational numbers be written as fractions?
Now, let us elaborate, irrational numbers could be written in decimals but not in the form of fractions, which means it cannot be written as the ratio of two integers. Irrational numbers have endless non-repeating digits after the decimal point. Below is an example of the irrational number: Example: √8 =2.828….
What is rational number?
Solution: A rational number is any real number that can be written as a fraction whose numerator and denominator are integers (in decimal form, this means a number that is either a terminating or repeating decimal). Thus, 0, 1, 0.11111..., and are rational.
What are the two classes of real numbers?
We can further divide the real numbers into two distinct classes: rational numbers and irrational numbers. A rational number is any real number that can be expressed exactly as a fraction whose numerator is an integer and whose denominator is a non-zero integer.
What are some examples of irrational numbers?
Examples of irrational numbers include and π. Rational numbers and irrational numbers are mutually exclusive: they have no numbers in common. Furthermore, they span the entire set of real numbers; that is, if you add the set of rational numbers to the set of irrational numbers, you get the entire set of real numbers.
Is a rational number a decimal?
In decimal form, a rational number is either a terminating decimal (such as 2, 1.375, and –0.5) or a repeating decimal (such as 0.3333...). An irrational number, on the other hand, cannot be written as a fraction with an integer numerator and denominator. Irrational numbers in decimal form are nonrepeating, nonterminating decimals.
What is the form of a complex number?
In general, a complex number has the form a + bi, where a and b are real numbers. ( a is called the real part and b is called the imaginary part.) Because i is not a real number, complex numbers cannot generally be placed on the real line (except when b is equal to zero). Complex numbers must be treated in many ways like binomials;
What is the real number that represents a particular point on a line called?
The real number corresponding to a particular point on the line is called a coordinate.
Is infinity a real number?
Note carefully, however, that infinity (typically written as ∞) is not a real number--it simply represents the fact that the real line extends indefinitely. In the positive (right) direction, the real line extends toward +∞ (positive infinity); in the negative (left) direction, it extends toward –∞ (negative infinity).
What are real numbers?
Real numbers include the sets of Integers, Natural Numbers, Whole numbers, Rational and irrational numbers. Complex numbers are one of the example of non-real numbers. Simply, we can say that the set of rational and irrational numbers together are called real numbers.
When were fractions first used?
Around 1000 BC, the term fractions were used by the Egyptians but the use of Irrational numbers can be seen in the Vedic “Shulba Sutras” in, c. 600 BC, it was the first use of irrational numbers.
What did mathematicians do in the Middle Ages?
In the Middle ages, the development of algebra by Muslim mathematicians allowed irrational numbers to be treated as algebraic objects. Middle Eastern mathematicians also merged the concepts of " number " and " magnitude " into a more general idea of real numbers, criticized Euclid's idea of ratios, developed the theory of composite ratios, and extended the concept of number to ratios of continuous magnitude. In his commentary on Book 10 of the Elements, the Persian mathematician Al-Mahani (d. 874/884) examined and classified quadratic irrationals and cubic irrationals. He provided definitions for rational and irrational magnitudes, which he treated as irrational numbers. He dealt with them freely but explains them in geometric terms as follows:
How are irrational numbers expressed?
Like all real numbers, irrational numbers can be expressed in positional notation, notably as a decimal number. In the case of irrational numbers, the decimal expansion does not terminate, nor end with a repeating sequence.
What is the real number that cannot be expressed as a ratio of integers?
Real number that cannot be expressed as a ratio of integers. The number √ 2 is irrational. In mathematics, the irrational numbers (from in- prefix assimilated to ir- (negative prefix, privative) + rational) are all the real numbers which are not rational numbers. That is, irrational numbers cannot be expressed as the ratio of two integers.
Is the square root of 2 irrational?
The square root of 2 was the first number proved irrational, and that article contains a number of proofs. The golden ratio is another famous quadratic irrational number. The square roots of all natural numbers which are not perfect squares are irrational and a proof may be found in quadratic irrationals .
Who discovered the existence of irrational numbers?
The first proof of the existence of irrational numbers is usually attributed to a Pythagorean (possibly Hippasus of Metapontum ), who probably discovered them while identifying sides of the pentagram. The then-current Pythagorean method would have claimed that there must be some sufficiently small, indivisible unit that could fit evenly into one of these lengths as well as the other. However, Hippasus, in the 5th century BC, was able to deduce that there was in fact no common unit of measure, and that the assertion of such an existence was in fact a contradiction. He did this by demonstrating that if the hypotenuse of an isosceles right triangle was indeed commensurable with a leg, then one of those lengths measured in that unit of measure must be both odd and even, which is impossible. His reasoning is as follows:
Is a decimal expansion a rational number?
Conversely, a decimal expansion that terminates or repeats must be a rational number. These are provable properties of rational numbers and positional number systems, and are not used as definitions in mathematics. Irrational numbers can also be expressed as non-terminating continued fractions and many other ways.
Does decimal expansion repeat?
The decimal expansion of an irrational number never repeats or terminates (the latter being equivalent to repeating zeroes), unlike any rational number. The same is true for binary, octal or hexadecimal expansions, and in general for expansions in every positional notation with natural bases.

Definitions
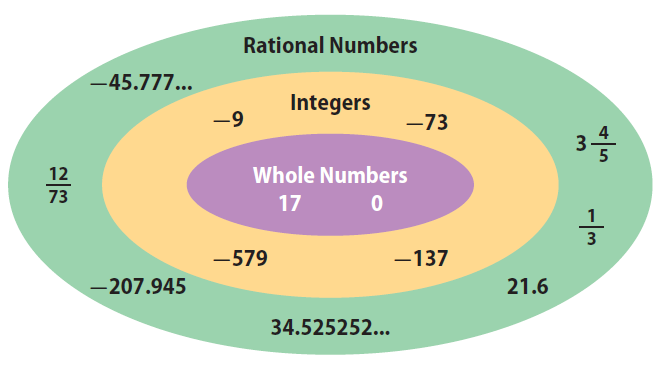
How to Classify Rational and Irrational numbers?
- Let us seehow to identify rational and irrational numbers based on the given set of examples. As per the definition,rational numbers include all integers, fractions and repeating decimals. For every rational number, we can write them in the form of p/q, where p and q are integer values.
Properties of Rational and Irrational Numbers
- Here are some properties based on arithmetic operations such as addition and multiplication performed on the rational number and irrational number. 1: The sum of two rational numbers is also rational. Example: 1/2 + 1/3 = (3+2)/6 = 5/6 2: The product of two rational numbers is rational. Example: 1/2 x 1/3 = 1/6 3: The sum of two irrational numbers ...
Solved Problems
- Q.1:Find any 5 rational numbers between 5 and 6. Solution: We need to find 5 rational numbers between 5 and 6. So, multiply and divide the numbers 5 and 6 by 5 + 1, i.e., 6. That means, 5 = 5 × (6/6) = 30/6 6 × (6/6) = 36/6 Therefore, five rational numbers between 5 and 6 are 31/6, 32/6, 33/6, 34/6, and 35/6. Q.2:Classify the following as rational and irrational numbers. √12, √16, √5, 0.944…