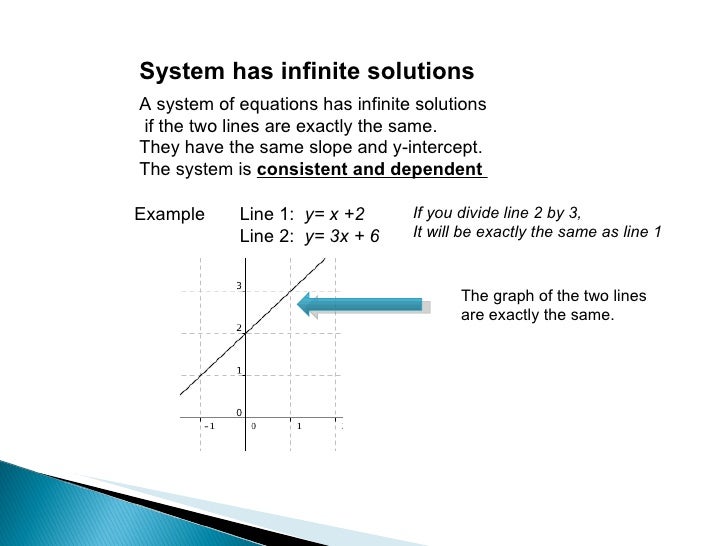
There are a few ways to tell when a linear system in two variables has infinite solutions:
- Solve the system – if you solve the system and get an equation that is always true, regardless of variable value (such as 1 = 1), then there are infinite solutions.
- Look at the graph – if the two lines are the same (they overlap, or intersect everywhere on the line), then there are infinite solutions to the system.
How do you know if an equation has infinite solutions?
A system of two linear equations in two variables has infinite solutions if the two lines are the same. From an algebra standpoint, we get an equation that is always true if we solve the system. Visually, the lines have the same slope and same y-intercept (they intersect at every point on the line).
Why are there infinite solutions to the linear system?
Since the slopes are the same and the y-intercepts are the same, the equations represent the same line. So, they will intersect at every point on the line. This means that there are infinite solutions to the linear system we started with. The lines are horizontal, so they both have the same slope (m = 0).
How do you know if a system of equations is consistent?
If the two lines have the same y-intercept and the slope, they are actually in the same exact line. In other words, when the two lines are the same line, then the system should have infinite solutions. It means that if the system of equations has an infinite number of solution, then the system is said to be consistent.
How do you know if an equation has a solution?
A system of linear equations in two variables has a solution when the two lines intersect in at least one place. If the two lines have the same slope and the same y-intercept, then the two equations are equivalent, and they represent the same line (so there are infinitely many solutions, since every point on the line is a solution).
What is infinite solution?
When we graph a linear system with infinite solutions, do we get two lines that overlap?
When Does A System Of Linear Equations Have No Solution?
How many solutions does a system of three equations have?
What happens to a system of equations in 3 variables?
What does it mean when two lines intersect?
When two lines are parallel, what is the solution of a system of two linear equations?
See 2 more
How do you know if the system of equations has infinite solutions?
If the two lines have the same y-intercept and the slope, they are actually in the same exact line. In other words, when the two lines are the same line, then the system should have infinite solutions.
How do you tell if a system has no solution or infinitely many?
Give the condition for a system of linear equations that has no solution. If a1/a2 = (b1/b2) ≠ (c1/c2), then there will be no solution.
How can you tell how many solutions a system has?
A system of two equations can be classified as follows: If the slopes are the same but the y-intercepts are different, the system has no solution. If the slopes are different, the system has one solution. If the slopes are the same and the y-intercepts are the same, the system has infinitely many solutions.
Is 0 0 infinite or no solution?
If you solve this your answer would be 0=0 this means the problem has an infinite number of solutions. For an answer to have no solution both answers would not equal each other.
How do you know if a system has a solution or not?
2:537:29One Solution, No Solution, or Infinitely Many Solutions - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd also four x minus y is equal to eighteen determine if there's one solution no solution or manyMoreAnd also four x minus y is equal to eighteen determine if there's one solution no solution or many solutions. If it's consistent or inconsistent dependent or independent.
What qualifies as no solution?
The constants are the numbers alone with no variables. If the coefficients are the same on both sides then the sides will not equal, therefore no solutions will occur.
What equation has infinitely many solutions?
The equation 2x + 3 = x + x + 3 is an example of an equation that has an infinite number of solutions.
What is the condition for no solution?
Condition for No Solution: If (a1/a2) = (b1/b2) ≠ (c1/c2), then this will result in no solution.
Define a Linear equation.
A Linear equation is an equation that has one or more variables having degree one.
Give an example of a Linear equation in two variables.
An example of a Linear equation in two variables: 2x + 3y + 4 = 0.
Give the condition for a system of linear equations that has no solution.
If a 1 /a 2 = (b 1 /b 2 ) ≠ (c 1 /c 2 ), then there will be no solution.
Give the condition for a system of linear equations that has infinitely many solutions.
If (a 1 /a 2 ) = (b 1 /b 2 ) = (c 1 /c 2 ), then there will be infinitely many solutions.
What are Infinite Solutions?
The number of solutions of an equation depends on the total number of variables contained in it. Thus, the system of the equation has two or more equations containing two or more variables. It can be any combination such as
What does it mean when a system of equations has an infinite number of solutions?
It means that if the system of equations has an infinite number of solution, then the system is said to be consistent. As an example, consider the following two lines. These two lines are exactly the same line. If you multiply line 1 by 5, you get the line 2. Otherwise, if you divide the line 2 by 5, you get line 1.
How to solve systems of equations?
To solve systems of an equation in two or three variables, first, we need to determine whether the equation is dependent, independent, consistent, or inconsistent. If a pair of the linear equations have unique or infinite solutions, then the system of equation is said to be a consistent pair of linear equations.
How many types of solutions are there to an equation?
Depending on the number of equations and variables, there are three types of solutions to an equation. They are
When can an equation have infinitely many solutions?
An equation can have infinitely many solutions when it should satisfy some conditions. The system of an equation has infinitely many solutions when the lines are coincident, and they have the same y-intercept. If the two lines have the same y-intercept and the slope, they are actually in the same exact line. In other words, when the two lines are ...
When two lines have the same y intercept and slope, what is the system?
If the two lines have the same y-intercept and the slope, they are actually in the same exact line. In other words, when the two lines are the same line, then the system should have infinite solutions. It means that if the system of equations has an infinite number of solution, then the system is said to be consistent.
What is the same line if you multiply by 5?
These two lines are exactly the same line. If you multiply line 1 by 5, you get the line 2. Otherwise, if you divide the line 2 by 5, you get line 1.
What type of equations allow an undetermined number of solutions?
Any system of equations that is underdetermined and consistent may be said to allow an undetermined number of solutions. A system of linear equations of this type will have a continuous infinity of solutions in Euclidean space.
Is arithmetic inconsistent?
That arithmetic, and therefore all of mathematics, is fundamentally inconsistent.
Can you express the solution of a linear system of simultaneous equations?
If you mean a system of linear simultaneous equations, in particular an underdetermined but consistent system, then you could express the solution in terms of parameters. It is often convenient to set one of the variables equal to the parameter, although it isn’t always possible with any given variable.
Can you make an equation arbitrarily long?
For example: “add 1 to both sides of the equation” and “subtract 1 from both sides of the equation”. Therefore, any proof you have, you can make arbitrarily long by including many pairs of
Can a mathematical framework have cancelling steps?
Having said all that , it is possible to produce a mathematical framework that doesn’t have cancelling steps. The simplest example is a null-logical framework that doesn’t have any logical steps at all. In such a case, all you know are the set of given axioms, and nothing else. In other words, a statement is true if it’s an axiom, and nothing else is provable. In this degenerate framework, there is exactly one (trivial) proof for all provable statements.
Do you need multiple parameters?
The other thing to keep in mind is that you might need multiple parameters. The example here might be a+b=0, c+d=0 where by setting a=s and c=t it is easy to show that a=s, b=-s, c=t, d=-t for any pair of values s and t. The number of parameters you need is always equal to the system’s degeneracy, the difference between the number of unknowns and the number of equations in the system.
What is infinite solution?
Solve the system – if you solve the system and get an equation that is always true, regardless of variable value (such as 1 = 1) , then there are infinite solutions.
When we graph a linear system with infinite solutions, do we get two lines that overlap?
That is, they intersect at every point on the line, since the two equations are equivalent and give us the same line.
When Does A System Of Linear Equations Have No Solution?
A system of two linear equations in two variables has no solution when the two lines are parallel.
How many solutions does a system of three equations have?
Of course, a system of three equations in three variables has infinite solutions if the planes intersect in an entire line (or an entire plane if all 3 equations are equivalent).
What happens to a system of equations in 3 variables?
A system of equations in 3 variables will have infinite solutions if the planes intersect in an entire line or in an entire plane.
What does it mean when two lines intersect?
If the two slopes are the same and the y-intercepts are the same, then the two lines are equivalent, meaning they intersect at all points on the line and there are infinite solutions to the linear system .
When two lines are parallel, what is the solution of a system of two linear equations?
A system of two linear equations in two variables has no solution when the two lines are parallel.
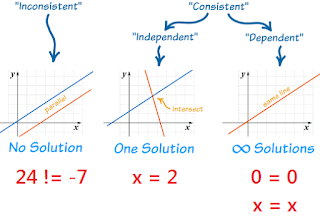
What Are Infinite Solutions?
- The number of solutions of an equation depends on the total number of variables contained in it. Thus, the system of the equation has two or more equations containing two or more variables. It can be any combination such as 1. 2 equations in 3 variables 2. 6 equations in 4 variables, and so on Depending on the number of equations and variables, there are three types of solutions to an …
Equations with Infinite Solutions
- To solve systems of an equation in two or three variables, first, we need to determine whether the equation is dependent, independent, consistent, or inconsistent. If a pair of the linear equations have unique or infinite solutions, then the system of equation is said to be a consistent pair of linear equations. Thus, suppose we have two equations in two variables as follows: a1x + b1y = …
Conditions For Infinite Solution
- An equation can have infinitely many solutions when it should satisfy some conditions. The system of an equation has infinitely many solutions when the lines are coincident, and they have the same y-intercept. If the two lines have the same y-intercept and the slope, they are actually in the same exact line. In other words, when the two lines are t...