
Solving Systems of Equations by Substitution Examples (One Solution)
- 1. Rewrite one of the equations to isolate one variable. ...
- 2. In the other equation, substitute the value of your isolated variable in for that variable. ...
- 3. Solve this second equation for the other variable. ...
- 4. Substitute your numerical value into one of the two original equations and solve for the other variable. ...
- 5. Check your work either. ...
How do you use substitution?
Substitution is a great way to solve a system of equations! In this tutorial, you'll see how the substitution method is used to solve a system of equations involving both a linear and a quadratic equation! How Do You Evaluate an Algebraic Expression?
What is a quadratic equation in disguise?
What makes these super equations quadratic equations in disguise is that by making one substitution, we can turn it into a quadratic equation that we can easily solve. Let's take a look at how we can go ahead and solve the equation x^4 - 3x^2 + 2.
How to solve a system of linear equations?
There are many different ways to solve a system of linear equations. In this tutorial, you'll see how to solve a system of linear equations by substituting one equation into the other and solving for the variable. Then, see how to use that variable value to find the value of the other variable.
Can you go through algebra without seeing quadratic equations?
You can't go through algebra without seeing quadratic equations. The graphs of quadratic equations are parabolas; they tend to look like a smile or a frown. There's also a bunch of ways to solve these equations!
What makes super equations quadratic equations in disguise?
Why are there disguised quadratics?
What kind of super equations can we expect to see?
About this website
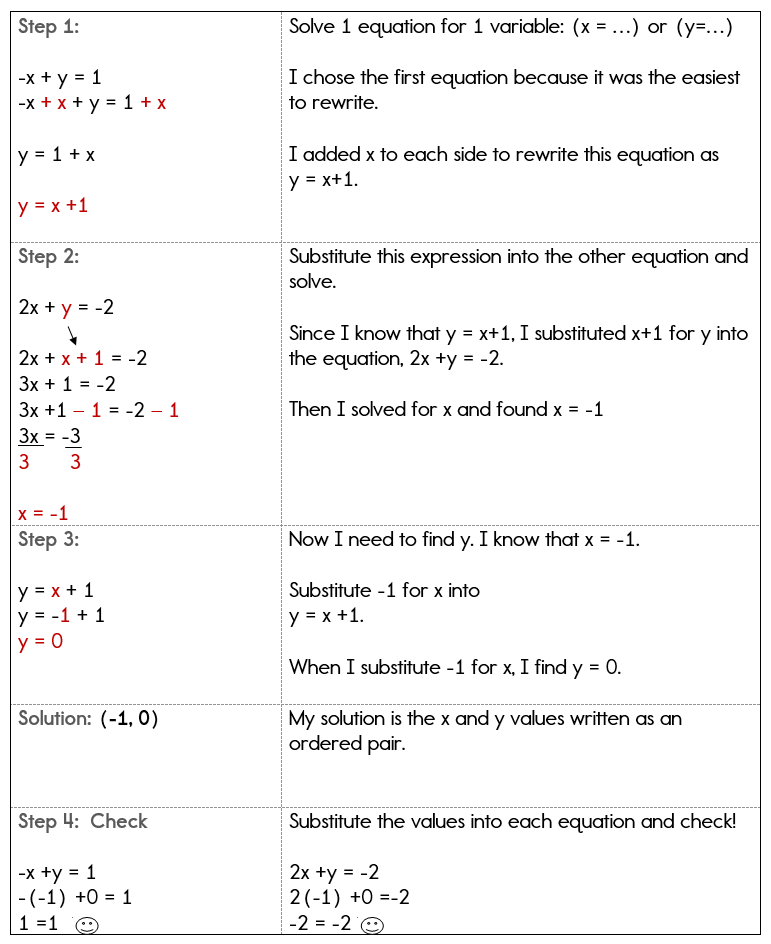
How do you solve by substitution step by step?
Steps to Solving by Substitution:Step One→ Solve one equation for either x or y.Step Two→ Substitute the expression from step one into the 2nd equation.Step Three→ Solve the second equation for the given variable.Step Four→ Plug you solution back into the first equation.Step Five→ Write your solution as a point.
How do you find the substitution solution?
SOLVE A SYSTEM OF EQUATIONS BY SUBSTITUTION.Solve one of the equations for either variable.Substitute the expression from Step 1 into the other equation.Solve the resulting equation.Substitute the solution in Step 3 into one of the original equations to find the other variable.Write the solution as an ordered pair.More items...•
What is an example of substitution method?
Substitution Method Examples In this way, we can find out the value of the unknown variables x and y using the substitution method. Example 2: Solve the pair of linear equations: 4x + 6y = 10 and 2x – 3y = 8 using Substitution method.
What do you mean by method of substitution?
The substitution method can be defined as a way to solve a linear system algebraically. The substitution method works by substituting one y-value with the other. To put it simply, the method involves finding the value of the x-variable in terms of the y-variable.
How do you do substitution in Algebra 1?
0:3113:45Learn Algebra 1 Substitution Method - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd we've got let's see 11x minus 20 add the 20 to both sides. Get the variable by itself divide byMoreAnd we've got let's see 11x minus 20 add the 20 to both sides. Get the variable by itself divide by 11 because we just want to get X by itself so x equals 5 now that's great.
How do you solve three equations with substitution?
16:1519:08Solve a 3 Variable System by Substitution - YouTubeYouTubeStart of suggested clipEnd of suggested clipWe'll multiply equation three by negative one and then we'll add in equation.MoreWe'll multiply equation three by negative one and then we'll add in equation.
How do you do u substitution for integrals?
1:3521:35How To Integrate Using U-Substitution - YouTubeYouTubeStart of suggested clipEnd of suggested clipAnd then u raised to the third power and then u divided by two x. So here we can cancel x four xMoreAnd then u raised to the third power and then u divided by two x. So here we can cancel x four x divided by two x is two.
What is substitution in geometry?
The substitution property in geometry states that if a geometric figure such as a line segment, triangle, angle, etc. is congruent to another geometric figure, and a geometric problem is given to determine the value of the unknown, then we can substitute the value of one with another and solve the problem.
Solving Quadratic Equations By Substitution Worksheet
Quadratic equations free worksheets powerpoints and other resources for gcse doingmaths maths openalgebra com solving in form practice questions answers cazoomy systems of kuta linear by substitution using the formula go teach handcrafted teachers factoring quadratics mathematics education college math edboost Quadratic Equations Free Worksheets Powerpoints And Other Resources For Gcse ...
Quadratic in Form (U-substitution) | math15fun.com
Example: Solve the equation. Solution: The equation is similar to a quadratic. It has 3 terms and one exponent is twice the other. Since the equation is quadratic in form, use substitution to solve the equation.
10.5 Solving Quadratic Equations Using Substitution
Factor the binomial .. Here, it would be a lot easier if the expression for factoring was .. First, let , which leaves the factor of .. easily factors out to .. Now that the substituted values are factored out, replace the with the original .This turns into .. The factored and terms can be recognized as the difference of cubes.. These are factored using and .
What makes super equations quadratic equations in disguise?
What makes these super equations quadratic equations in disguise is that by making one substitution, we can turn it into a quadratic equation that we can easily solve. Let's take a look at how we can go ahead and solve the equation x^4 - 3x^2 + 2. To help us figure out what kind of substitution to make, we can ask ourselves, 'how can we change the equation so it becomes like the regular quadratic equations that we are so familiar with'? Looking at our equation, we see that if we make the substitution u = x^2, then our equation will turn back to normal. We are substituting with whatever the disguise is. We use another letter for our substitution so that we don't get confused with the problem's variable. Let's see what happens when we make this substitution. Our equation, x^4 - 3x^2 + 2, turns into u^2 - 3u + 2. Hey, that's a normal quadratic equation! We know how to solve this.
Why are there disguised quadratics?
We know these are disguised quadratics because by making one substitution, we can turn the super equation into a normal quadratic equation that we can easily solve. After we make the substitution, we solve the quadratic equation as we normally do using the skills we have already learned, such as factoring.
What kind of super equations can we expect to see?
What kind of super equations can we expect to see? We can expect to see equations such as x^4 + 3x^2 + 2 or even (x - 2)^2 - 4 (x - 2) - 12. These are super equations because we see that they are disguised quadratics. The x^4 + 3x^2 + 2 can be rewritten as (x^2)^2 + 3 (x^2) + 2. Our quadratic has the x^2 disguise on. The (x - 2)^2 - 4 (x - 2) - 12 has the (x - 2) disguise on. Once we've discovered the disguise, we see that they still follow the quadratic form of ax^2 + bx + c.
What makes super equations quadratic equations in disguise?
What makes these super equations quadratic equations in disguise is that by making one substitution, we can turn it into a quadratic equation that we can easily solve. Let's take a look at how we can go ahead and solve the equation x^4 - 3x^2 + 2. To help us figure out what kind of substitution to make, we can ask ourselves, 'how can we change the equation so it becomes like the regular quadratic equations that we are so familiar with'? Looking at our equation, we see that if we make the substitution u = x^2, then our equation will turn back to normal. We are substituting with whatever the disguise is. We use another letter for our substitution so that we don't get confused with the problem's variable. Let's see what happens when we make this substitution. Our equation, x^4 - 3x^2 + 2, turns into u^2 - 3u + 2. Hey, that's a normal quadratic equation! We know how to solve this.
Why are there disguised quadratics?
We know these are disguised quadratics because by making one substitution, we can turn the super equation into a normal quadratic equation that we can easily solve. After we make the substitution, we solve the quadratic equation as we normally do using the skills we have already learned, such as factoring.
What kind of super equations can we expect to see?
What kind of super equations can we expect to see? We can expect to see equations such as x^4 + 3x^2 + 2 or even (x - 2)^2 - 4 (x - 2) - 12. These are super equations because we see that they are disguised quadratics. The x^4 + 3x^2 + 2 can be rewritten as (x^2)^2 + 3 (x^2) + 2. Our quadratic has the x^2 disguise on. The (x - 2)^2 - 4 (x - 2) - 12 has the (x - 2) disguise on. Once we've discovered the disguise, we see that they still follow the quadratic form of ax^2 + bx + c.
