What does Euclid's 5th postulate say?
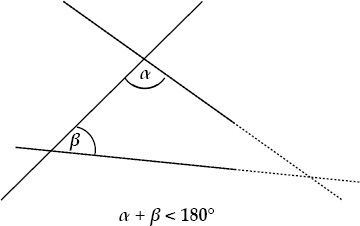
Euclid's fifth postulate states that if a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two right angles, then both straight lines, if produced indefinitely, will meet on the other side on which the sum of angles is less than two right angles.
What are the postulates of Euclidean geometry?
What are the five postulates of Euclid’s geometry?
- A straight line may be drawn from any point to another point.
- A terminated line can be produced indefinitely.
- A circle can be drawn with any centre and radius.
- All right angles are equal to each other.
What is the meaning of postulates?
Postulates are statements assumed to be true without any requirement of proof. They are built upon the knowledge that satisfies the reader (or listener) in terms of veracity.
How are postulates and Theorems similar?
Postulates and Theorems. A postulate is a statement that is assumed true without proof. A theorem is a true statement that can be proven. Listed below are six postulates and the theorems that can be proven from these postulates. Postulate 1: A line contains at least two points. Postulate 2: A plane contains at least three noncollinear points.
How many postulates are given by Euclid?
five postulatesNow let us discuss Euclid's five postulates. They are : Postulate 1 : A straight line may be drawn from any one point to any other point.
What are the 5 Euclidean postulates?
Euclid's PostulatesA straight line segment can be drawn joining any two points.Any straight line segment can be extended indefinitely in a straight line.Given any straight line segment, a circle can be drawn having the segment as radius and one endpoint as center.All right angles are congruent.More items...
How many Euclid's theorems are there?
All five axioms provided the basis for numerous provable statements, or theorems, on which Euclid built his geometry.
What are the 7 axioms of Euclid?
What were Euclidean Axioms?Things which are equal to the same thing are equal to one another.If equals are added to equals, the wholes are equal.If equals are subtracted from equals, the remainders are equal.Things which coincide with one another are equal to one another.The whole is greater than the part.More items...•
What is the other name for Euclid's 5th postulate?
Parallel postulateIn geometry, the parallel postulate, also called Euclid's fifth postulate because it is the fifth postulate in Euclid's Elements, is a distinctive axiom in Euclidean geometry.
What are the 5 theorems of geometry?
In particular, he has been credited with proving the following five theorems: (1) a circle is bisected by any diameter; (2) the base angles of an isosceles triangle are equal; (3) the opposite (“vertical”) angles formed by the intersection of two lines are equal; (4) two triangles are congruent (of equal shape and size ...
How many theorems did Euclid give in his book Elements?
Contents. Book 1 contains 5 postulates (including the infamous parallel postulate) and 5 common notions, and covers important topics of plane geometry such as the Pythagorean theorem, equality of angles and areas, parallelism, the sum of the angles in a triangle, and the construction of various geometric figures.
How many theorems are there in Euclidean geometry grade 11?
SEVEN theoremsSegment A segment is the part of the circle that is cut off by a chord. A chord divides a circle into two segments Tangent A tangent is a line that makes contact with a circle at one point on the circumference (AB is a tangent to the circle at point P). All SEVEN theorems listed in the CAPS document must be proved.
What is Euclid's 4th postulate?
All right angles are congruent or equal to one another. A right angle is an angle measuring 90 degrees. So, irrespective of the length of a right angle or its orientation all right angles are identical in form and coincide exactly when placed one on top of the other.
How many types of postulates are there?
The five postulates of Euclidean Geometry define the basic rules governing the creation and extension of geometric figures with ruler and compass.
What is the fifth proposition of Euclid?
If a straight line falling on two straight lines makes the interior angles on the same side of it taken together less than two right angles, then the two straight lines, if produced indefinitely, meet on that side on which the sum of angles is less than two right angles.
What is SAS ASA and SSS congruence postulates?
The congruency can also be tested by three postulates shown in the lesson: ASA (angle-side-angle), SAS (side-angle-side), and SSS (side-side-side). The first one claims that triangles are congruent if two angles and one side (between the angles) of one triangle are equal to two angles and one side of another triangle.
State the five postulates of Euclidean geometry?
Five common postulates of Euclidean geometry are: • You can draw a straight-line segment from any given point to others. • You can extend a straigh...
What are the uses of Euclidean geometry?
We use Euclidean geometry to design buildings, predict the location of moving objects, and survey land.
How Euclid contribute to geometry?
From a small set of axioms Euclid deduced the theorems of what is now known as Euclidean geometry. In addition, he also wrote works on conic sectio...
How does Euclid define a line?
When Euclid first formalized the geometry, he defined a general line as “breathless length” as a straight line is a line “that lies evenly with the...
Q.1. What are the seven axioms of Euclid?
Ans: The seven axioms of Euclid are given below: 1. The things that are similar to the same thing are equal to each other. 2. If equals are added t...
Q.2. What is the difference between axioms and postulates?
Ans: Axioms and postulates are essentially the same things. In math, the truths are accepted without proof. Axioms are general statements about rea...
Q.3. What are the five postulates of Euclid?
Ans: Euclid's five postulates are given below: Postulate 1: A straight line can be drawn from any point to any other point. Postulate 2: A terminat...
Q.4. What are axioms, postulates and theorems?
Ans: Axioms or postulates are the assumptions that are apparent universal truths. They are not proved. Theorems are statements that are proved usin...
Q.5. What is an axiom in math?
Ans: In math or logic, an axiom is an unprovable rule or the first principle accepted as true because it is self-evident or beneficial. "Nothing ca...
How many straight lines can be drawn parallel to a given line?
5ONE. Through any given point can be drawn exactly one straightline parallel to a given line.
Can postulate 5 live happily with the first four postulates?
Once you see that this is the geometry of great circles on spheres, you also see that postulate 5 NONE cannot live happily with the first four postulates after all. They need some minor adjustment:
How many postulates did Euclid have?
Below, you can see Euclid’s five postulates:
How many axioms does Euclid have?
The seven axioms of Euclid are given below:
What is Euclid's geometry?
The geometry of plane figures is also based upon the approach of deductive logic. That is why it is popularly called Euclid’s geometry.
Which postulate states that all right angles are similar?
Postulate 4: All the right angles are similar (equal) to one another.
What is the conclusion obtained through logical reasoning based on previously proved results and some axioms?
Theorems: The conclusion obtained through logical reasoning based on previously proved results and some axioms constitute a statement known as a theorem or a proposition.
What are the things that coincide with each other?
The things that coincide with each other are equal.
What are the subsets of a plane and a line?
Remark 2: In theoretical terms, a plane and a line consist of sets of points and lines drawn in a plane are subsets of it.
What was required of all students when studying Euclid's elements?
For centuries, when the quadrivium was included in the curriculum of all university students, knowledge of at least part of Euclid's Elements was required of all students.
How long did Euclid's axioms influence geometry?
However, Euclid's systematic development of his subject, from a small set of axioms to deep results, and the consistency of his approach throughout the Elements, encouraged its use as a textbook for about 2,000 years. The Elements still influences modern geometry books.
What is the beauty of Euclid?
The austere beauty of Euclidean geometry has been seen by many in western culture as a glimpse of an otherworldly system of perfection and certainty. Abraham Lincoln kept a copy of Euclid in his saddlebag, and studied it late at night by lamplight; he related that he said to himself, "You never can make a lawyer if you do not understand what demonstrate means; and I left my situation in Springfield, went home to my father's house, and stayed there till I could give any proposition in the six books of Euclid at sight". Edna St. Vincent Millay wrote in her sonnet " Euclid alone has looked on Beauty bare ", "O blinding hour, O holy, terrible day, When first the shaft into his vision shone Of light anatomized!". Albert Einstein recalled a copy of the Elements and a magnetic compass as two gifts that had a great influence on him as a boy, referring to the Euclid as the "holy little geometry book".
How many books are there in the Elements?
13 books. The Elements ( Ancient Greek: Στοιχεῖον Stoikheîon) is a mathematical treatise consisting of 13 books attributed to the ancient Greek mathematician Euclid in Alexandria, Ptolemaic Egypt c. 300 BC. It is a collection of definitions, postulates, propositions ( theorems and constructions ), and mathematical proofs of the propositions.
When were the elements of Euclid translated into Arabic?
The Arabs received the Elements from the Byzantines around 760; this version was translated into Arabic under Harun al Rashid c. 800. The Byzantine scholar Arethas commissioned the copying of one of the extant Greek manuscripts of Euclid in the late ninth century. Although known in Byzantium, the Elements was lost to Western Europe until about 1120, when the English monk Adelard of Bath translated it into Latin from an Arabic translation.
What was the purpose of geometry in the Victorian period?
Geometry emerged as an indispensable part of the standard education of the English gentleman in the eighteenth century; by the Victorian period it was also becoming an important part of the education of artisans, children at Board Schools, colonial subjects and, to a rather lesser degree, women. The standard textbook for this purpose was none other than Euclid's The Elements.
What is Euclid's element?
Euclid's Elements has been referred to as the most successful and influential textbook ever written. It was one of the very earliest mathematical works to be printed after the invention of the printing press and has been estimated to be second only to the Bible in the number of editions published since the first printing in 1482, the number reaching well over one thousand. For centuries, when the quadrivium was included in the curriculum of all university students, knowledge of at least part of Euclid's Elements was required of all students. Not until the 20th century, by which time its content was universally taught through other school textbooks, did it cease to be considered something all educated people had read.
