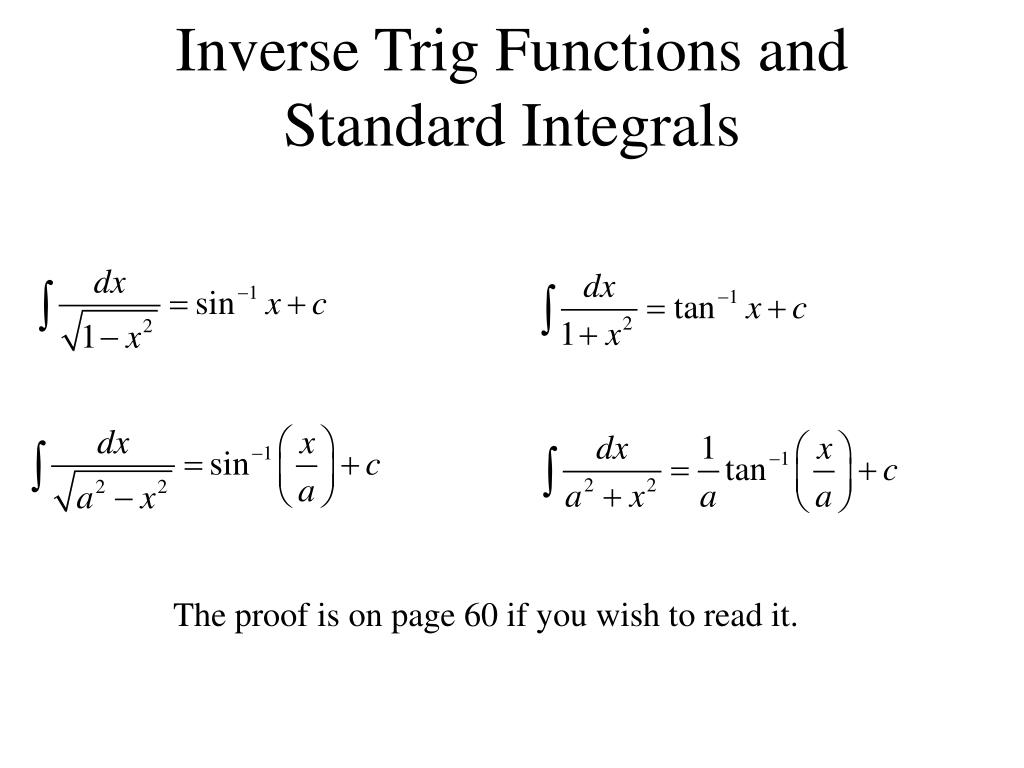
What is standard integral formula?
∫ 1 ( x 2 + a 2 ) d x = 1 a t a n − 1 ( x a ) + C. ∫ 1 x 2 – a 2 d x = l o g | x + x 2 – a 2 | + C. ∫ 1 a 2 – x 2 d x = s i n − 1 ( x a ) + C.
What are the types of integrals?
The two types of integrals are definite integral (also called Riemann integral) and indefinite integral (sometimes called an antiderivative).
What are the examples of integrals?
Examples of IntegrationExample 1: Integrate the function f(x)=2x sin(x2+1) with respect to x. Solution: ... Example 2: Find the integral ∫x2+1x2−5x+6dx ∫ x 2 + 1 x 2 − 5 x + 6 d x . ... Example 3: If ddxf(x)=4x3−3x4 d d x f ( x ) = 4 x 3 − 3 x 4 and f(2)=0 f ( 2 ) = 0 , find the function f(x) . ... Example 4: Calculate ∫ cos2 x dx.
What is the definition of definite integration?
Definition of definite integral : the difference between the values of the integral of a given function f(x) for an upper value b and a lower value a of the independent variable x.
What are the 3 types of integration?
The main types of integration are:Backward vertical integration. This involves acquiring a business operating earlier in the supply chain – e.g. a retailer buys a wholesaler, a brewer buys a hop farm.Conglomerate integration. ... Forward vertical integration. ... Horizontal integration.
What are the three integration methods?
The different methods of integration include: Integration by Substitution. Integration by Parts. Integration Using Trigonometric Identities.
What is the purpose of integrals?
Integrals are used to evaluate such quantities as area, volume, work, and, in general, any quantity that can be interpreted as the area under a curve.
What are integrals used for in real life?
In real life, integrations are used in various fields such as engineering, where engineers use integrals to find the shape of building. In Physics, used in the centre of gravity etc. In the field of graphical representation, where three-dimensional models are demonstrated. Was this answer helpful?
Why do we use integrals?
An integral is a function, of which a given function is the derivative. Integration is basically used to find the areas of the two-dimensional region and computing volumes of three-dimensional objects. Therefore, finding the integral of a function with respect to x means finding the area to the X-axis from the curve.
Why it is called definite integral?
A definite integral has a definite area to calculate. For an indefinite integral, the right and left bound for the area are not decided. – Arthur. Nov 19, 2014 at 15:27. The connection between them is given by the fundamental theorem of calculus.
What is the difference between indefinite and definite integrals?
There are no limits of integration in an indefinite integral. A definite integral represents a number when the lower and upper limits are constants. The indefinite integral represents a family of functions whose derivatives are f. The difference between any two functions in the family is a constant.
What are definite integrals in calculus?
The definite integral is defined to be exactly the limit and summation that we looked at in the last section to find the net area between a function and the x -axis. Also note that the notation for the definite integral is very similar to the notation for an indefinite integral.
How many types of integral equations are there?
There are four basic types of integral equations. There are many other integral equations, but if you are familiar with these four, you have a good overview of the classical theory. All four involve the unknown function φ(x) in an integral with a kernel K(x, y) and all have an input function f(x).
How many classifications does an integral have?
Types of Integrations There are two forms of the integrals. Indefinite Integrals: It is an integral of a function when there is no limit for integration. It contains an arbitrary constant. Definite Integrals: An integral of a function with limits of integration.
What are integrals in calculus?
In calculus, an integral is a mathematical object that can be interpreted as an area or a generalization of area. Integrals, together with derivatives, are the fundamental objects of calculus. Other words for integral include antiderivative and primitive.
What are definite integrals in calculus?
The definite integral is defined to be exactly the limit and summation that we looked at in the last section to find the net area between a function and the x -axis. Also note that the notation for the definite integral is very similar to the notation for an indefinite integral.