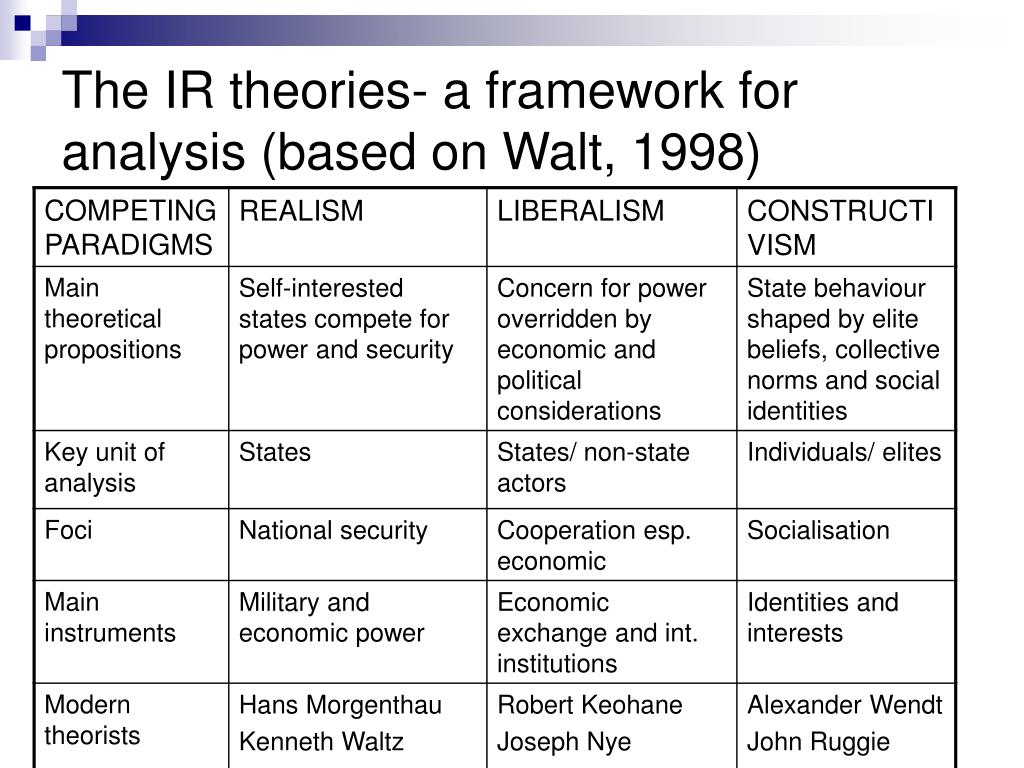
The aim of this paper is to compare learning theories in mathematics teaching, and to study their influence on mathematics learning. Numerous studies have shown that students experience mathematics anxiety, which is a feeling of tension and fear that interferes with math learning. This may be attributed to the teaching methods utilized. Therefore, teaching methods must be re-examined, taking into account the three major learning theories: behaviorism, cognitivism, and constructivism. Behaviorism is a teacher-centered instruction, while cognitivism states learning is based upon how people mentally process stimuli encountered. Mathematics teachers following a constructivist approach favor extending class time to engage in varied activities associated with the discovery and construction of knowledge. Thus, the qualitative case study method was considered more feasible and appropriate to meet the study aim. Data were collected using observation and semi-structured interviews with teachers in secondary schools in Malaysia. It was observed that positive and negative reinforcement (behaviorism), problem solving (cognitivism) and discovery learning (constructivism) were practiced by the teachers. The findings reveal that students are more successful when systematic problem solving based on Polya’s approach is incorporated into discovery learning. Consequently, there should be more emphasis on teaching methods which include less lecture, more student-directed classes and more discussion. The findings suggest that problem-solving and discovery-learning skills not only contribute to better mathematics learning but also enhance students’ creativity to cope with life challenges.
What are the four theories of mathematics education?
Theories of Mathematics Education. Four philosophies of learning are contrasted, namely 'simple' constructivism, radical constructivism, enactivism and social constructivism. Their underlying explanatory metaphors and some of their strengths and weaknesses are contrasted, as well as their implications for teaching and research.
What determines a teacher's theoretical theory of teaching and learning?
teachers' personal theories of teaching and learning develops from both internal factors (such as beliefs), external factors (such as curriculum, time), and district mandates (such as standardized testing). The intent of this study was to identify a teacher's theoretical
How do teachers define mathematics?
Cross (2009) found that teachers with more than 3 years of experience described mathematics as involving mental processes and activities. These teachers classified themselves as facilitators of instruction in the classroom and described their classes as
Do teachers’ theories of teaching and learning predict their use of math interventions?
An ordinal logistic regression statistical test was used to analyze relationships between teachers’ personal theories of teaching and learning and their use of math interventions, years of experience, gender, ethnicity, and age. A statistically significant relationship was found between teachers’ years of
Why is history important in mathematics?
What is the mathematical competence framework?
What are the different types of constructivism?
Why are examples important in math?
Who used epistemological terms?
Is the distinction between the structure and content of understanding relative?
Is math for kids complex?
See more
About this website

Teaching Mathematics with Piaget’s Theory - Semantic Scholar
Ega Gradini, S.Pd, M.Sc Teaching Mathematics... Volume IV. Nomor 1 Januari – Juni 2013| 1 Teaching Mathematics with Piaget’s Theory Ega Gradini, S.Pd, M.Sc
Zoltan Dienes’ six-stage theory of learning mathematics
Stage 1. Most people, when confronted with a situation which they are not sure how to handle, will engage in what is usually described as “trial and error” activity.
What are the three fundamental processes that contribute to the child's cognitive development?
Cognitive constructivism Piaget (1896-1980) • three fundamental processes, which contributed to the child‟s cognitive development., namely assimilation, accommodation, and equilibrium. • Assimilation involved the incorporation of new events into pre-existing cognitive structures. • Accommodation is the adjustment involved in the formation of new mental structures needed to accommodate new information. • Accommodation is the adjustment involved in the formation of new mental structures needed to accommodate new information. • When a child experienced a new event, disequilibrium set in until he was able to assimilate and accommodate the new information and thus attain equilibrium.
What is learning style?
22. Learning styles • Sensory pathways through which learners prefer to receive information. • It is a biologically and developmentally imposed set of characteristics that explain why the same lesson, readings, interactions, classroom settings and teachers affect individuals differently.
What are the two types of learning in mathematics?
Skemp (1976) defines two types of mathematical learning. Instrumental learning which involves learning processes by rote; this is usually performed by the teacher demonstrating how to solve a particular problem, followed by the students applying this knowledge to very similar problems. Relational learning however, involves understanding the concepts and the reasoning underlying the knowledge rather than just applying rules. Instrumental understanding produces procedural understanding –the ‘how’ knowledge, whereas relational understanding produces conceptual understanding – the ‘how’ and ‘why’ knowledge. He theorised that both types of learning are important as they both teach the student the rules of mathematics. For example, in calculating the area of a rectangle, students need to know that the area is length multiplied by width – this is instrumental knowledge; being able to see why this rule always works, requires relational understanding.
What is the commonality between theorists?
There is however, much commonality between theorists, for example Skemp (1964) Ausubel (1968) and Bruner (1966) believe that mathematics is hierarchal in nature; the vast majority of theorists agree that a priori learning plays an vital role in the acquisition of new mathematical learning.
Why is it important for teachers to give a brief overview of the knowledge that students will learn beforehand?
This then enables learners to link new concepts and vocabulary to existing known ideas. Existing knowledge provides a framework into which the new learning is related.
What is relational learning?
Relational learning however, involves understanding the concepts and the reasoning underlying the knowledge rather than just applying rules. Instrumental understanding produces procedural understanding –the ‘how’ knowledge, whereas relational understanding produces conceptual understanding – the ‘how’ and ‘why’ knowledge.
What are the five categories of learning?
The five categories are: verbal information, intellectual skills, cognitive strategies, motor skills, and attitudes. Gagné suggested that learning is most effective when students move from mastery of the smallest conceptual units to the most general concepts.
What is the dynamic principle of learning?
First is the Dynamic Principle – Learning is an active process that requires opportunities to be provided for students to interact.
Who advocated the use of concrete models to help learners formulate mathematical ideas?
Similarly, the use of concrete models to help learners formulate mathematical ideas is advocated by many theorists such as Bruner (1966) , Van Hieles (1958) , and Dienes (1960). Below is a brief summary of the most renowned mathematical theorist’s ideas.
What are the three theories of learning in mathematics?
This may be attributed to the teaching methods utilized. Therefore, teaching methods must be re-examined, taking into account the three major learning theories: behaviorism, cognitivism, and constructivism . Behaviorism is a teacher-centered instruction, while cognitivism states learning is based upon how people mentally process stimuli encountered. Mathematics teachers following a constructivist approach favor extending class time to engage in varied activities associated with the discovery and construction of knowledge. Thus, the qualitative case study method was considered more feasible and appropriate to meet the study aim. Data were collected using observation and semi-structured interviews with teachers in secondary schools in Malaysia. It was observed that positive and negative reinforcement (behaviorism), problem solving (cognitivism) and discovery learning (constructivism) were practiced by the teachers. The findings reveal that students are more successful when systematic problem solving based on Polya’s approach is incorporated into discovery learning. Consequently, there should be more emphasis on teaching methods which include less lecture, more student-directed classes and more discussion. The findings suggest that problem-solving and discovery-learning skills not only contribute to better mathematics learning but also enhance students’ creativity to cope with life challenges.
How did Teacher C use discovery learning?
In the observation, Teacher C used the method of discovery learning, despite sometimes using the traditional method for beginning the new lesson. In good spirits, she began to review the previous lesson and gave an introduction about the new subject before starting it. She had a lesson plan for teaching and paid attention to the students’ ideas regarding the course. She allowed the students to participate in class activities, provided opportunities for them to think about the mathematic subject, and allowed group discussions in the class. She also used the whiteboard, PowerPoint, educational CDs, and extra mathematical books in the class. While doing exercises, she paid attention to students’ ideas to clarify and to allow them to identify the problems.
Why do teachers synthesize theories?
Teachers synthesize multiple theories, some of them decades-old, in order to improve their students' learning outcomes.
What is the theory of schema?
Jean Piaget's schema theory suggests new knowledge with students' existing knowledge, the students will gain a deeper understanding of the new topic. This theory invites teachers to consider what their students already know before starting a lesson.
What is behaviorism theory?
In the classroom, behaviorism is the theory that students' learning and behavior will improve in response to positive reinforcement like rewards, praise, and bonuses. The behaviorist theory also asserts that negative reinforcement — in other words, punishment — will cause a child to stop undesired behavior. According to Skinner, these repeated reinforcement techniques can shape behavior and produce improves learning outcomes.
How many types of intelligence are there in the world of teaching?
The theory of multiple intelligence transformed the world of learning and pedagogy. Today, many teachers employ curriculums that have been developed around eight types of intelligence. Lessons are designed to include techniques that align with each individual student's learning style. 02. of 06.
What is Piaget's theory of constructivism?
Piaget's theory of constructivism, which states that individuals construct meaning through action and experience, plays a major role in schools today. A constructivist classroom is one in which students learn by doing, rather than by passively absorbing knowledge.
What is the theory of negative reinforcement?
The behaviorist theory also asserts that negative reinforcement — in other words, punishment — will cause a child to stop undesired behavior. According to Skinner, these repeated reinforcement techniques can shape behavior and produce improves learning outcomes.
What are learning theories?
Theories in education didn’t begin in earnest until the early 20th century, but curiosity about how humans learn dates back to the ancient Greek philosophers Socrates, Plato and Aristotle. They explored whether knowledge and truth could be found within oneself (rationalism) or through external observation (empiricism).
How does understanding learning theories help students?
Understanding learning theories can result in a variety of outcomes, from improving communication between students and teachers to determining what students learn.
Why are learning theories important?
Understanding how people learn is a critical step in optimizing the learning process.
How do educational theories influence learning?
Educational theories influence learning in a variety of ways. For teachers, learning theory examples can impact their approach to instruction and classroom management. Finding the right approach (even if it’s combining two or more learning theories) can make the difference between an effective and inspiring classroom experience and an ineffective one.
What is transformative learning theory?
Transformative learning theory: This theory is particularly relevant to adult learners. It posits that new information can essentially change our worldviews when our life experience and knowledge are paired with critical reflection.
What is social learning theory?
Social learning theory: This theory incorporates some of the tacit tenets of peer pressure. Specifically, students observe other students and model their own behavior accordingly. Sometimes it’s to emulate peers; other times it’s to distinguish themselves from peers. Harnessing the power of this theory involves getting students’ attention, focusing on how students can retain information, identifying when it’s appropriate to reproduce a previous behavior, and determining students’ motivation.
What was the goal of psychologists in the 19th century?
The goal was to understand objectively how people learn and then develop teaching approaches accordingly.
Why is history important in mathematics?
While evidence on the efficacy of this approach remains limited, studies to date suggest that students who confront new mathematics concepts within a meaningful historical context will develop more positive attitudes toward the subject matter. Using history in mathematics teaching is likely also to help students recognise interrelationships between the disparate concepts to which they are introduced, and thus develop a more integrated view of the field as a whole. Relevant classroom activities, based on the Singapore junior college mathematics curriculum, are outlined.
What is the mathematical competence framework?
Possessing the mathematical competence to encounter the challenges under globalisation and the ubiquity of digital technology is an important goal of education. Herein, we propose a broad conceptual framework for understanding and developing mathematical competence. The framework comprises four non-overlapping but interlinked cores: learning, knowing, applying, and viewing. Our framework encompasses ontological, epistemological, and pedagogical dimensions based on a multi-dual perspective. The proposed framework serves as a foundation for generating different learning paths to develop mathematical competence. Examples of learning paths are provided to illustrate the correlative and complementary relationships among the cores of mathematical competence
What are the different types of constructivism?
Four philosophies of learning are contrasted, namely 'simple' constructivism, radical constructivism, enactivism and social constructivism. Their underlying explanatory metaphors and some of their strengths and weaknesses are contrasted, as well as their implications for teaching and research. However, it is made clear that none of these 'implications' is incompatible with any of the learning philosophies, even if they sit more comfortably with one of them. ZDM-Classification: D20 Construction Constructivism has been a leading if not the dominant theory or philosophy of learning in the mathematics education research community ever since the heated controversy in the 1987 Montreal PME conference. What made constructivism such a hot issue was not just what it claims about learning. Rather it is the epistemological implications that follow from it. As one of the leading exponents of constructivism said "To introduce epistemological considerations into a discussion of education has always been dynamite" (Glasersfeld 1983: 41). But constructivism does not represent a single school of thought, as there are several versions and varieties, some diametrically opposed to others. What binds many of the various forms of constructivism together is the metaphor of construction from carpentry or architecture. This metaphor is about the building up of structures from pre-existing pieces, possibly specially shaped for the task. In its individualistic form the metaphor describes understanding as the building of mental structures, and the term 'restructuring', often used as a synonym for 'accommodation' or 'conceptual change' in cognitivist theory, contains this metaphor. What the metaphor need not mean in m ost versions of constructivism is that understanding is built up from received pieces of knowledge. The process is recursive (Kieren and Pirie 1991), and so the building blocks of understanding are themselves the product of previous acts of construction. Thus the distinction between the structure and content of understanding can only be relative in constructivism. Previously built structures become the content in subsequent constructions. Meanings, structures and knowledge are emergent.
Why are examples important in math?
Examples are believed to be very important in developing conceptual understanding of mathematical ideas, useful both in mathematics research and instruction (Bills & Watson in Educational Studies in Mathematics 69:77–79, 2008; Mason & Watson, 2008; Bills & Tall, 1998; Tall & Vinner, 1981). In this study, we draw on the concept of an example space (Mason & Watson, 2008) and variation theory (Runesson in Scandinavian Journal of Educational Research 50:397–410, 2006) to create a lens to study how examples are used for pedagogical purposes in undergraduate proof-based instruction. We adapted the construct of an example space and extended its application to the constructs of example neighborhood, methods of example construction, and the functions of examples. We explained how to use our new lens to analyze the collection of examples and non-examples that the students had access to. We then demonstrate our method by analyzing the collection of examples and non-examples of a mathematical group the professor of an abstract algebra class presented during lectures or assigned to students in problem sets or exams.
Who used epistemological terms?
epistemological terms by von Glasersfeld and colleagues.
Is the distinction between the structure and content of understanding relative?
Thus the distinction between the structure and content of understanding can only be relative in constructivism. Previously built structures become the content in subsequent constructions. Meanings, structures and knowledge are emergent.
Is math for kids complex?
Mathematics for children, as they ‘do’ it and build onto their own knowledge, is a complex phenomenon. As with personal knowledge of mathematics for any person, it is multifaceted, a view supported by mathematicians (e.g. Davis and Hersh, 1986) and psychologists interested in mathematics education (e.g. Vergnaud, 1983). There are many ways to observe and hence say something about children’s mathematics. One could consider mathematical thought mechanisms used by children. One could analyze specific aspects of the complexity. However, in this essay we try to observe and talk about children’s mathematical experience as a complex whole.
