
What does it mean if something is tangent to a circle?
Tangent to a circle is the line that touches the circle at only one point. There can be only one tangent at a point to circle. Point of tangency is the point at which tangent meets the circle.
How do you find tangent to a circle?
A tangent to a circle at point P with coordinates is a straight line that touches the circle at P. The tangent is perpendicular to the radius which joins the centre of the circle to the point P. As the tangent is a straight line, the equation of the tangent will be of the form y = m x + c .
What does it mean when two lines are tangent to a circle?
Tangent to a Circle TheoremA line is tangent to a circle if and only if the line is perpendicular to the radius drawn to the point of tangency. Two Tangent TheoremThe Two-Tangent Theorem states that if two tangent segments are drawn to one circle from the same external point, then they are congruent.
How do you tell if a line is tangent to a circle?
A tangent to a circle is a straight line which touches the circle at only one point. This point is called the point of tangency. The tangent to a circle is perpendicular to the radius at the point of tangency.
What does tangent mean in math?
In Geometry, the tangent is defined as a line touching circles or an ellipse at only one point. Suppose a line touches the curve at P, then the point “P” is called the point of tangency. In other words, it is defined as the line which represents the slope of a curve at that point.
What does it mean if something is tangent to something?
1 : an abrupt change of course : digression the speaker went off on a tangent. 2a : the trigonometric function that for an acute angle is the ratio between the leg opposite to the angle when it is considered part of a right triangle and the leg adjacent.
How many tangents are in a circle?
infinite tangentsAs the circle is a set of points equidistant from a fixed point, there will be infinite points that form a circle and hence, infinite tangents are possible.
How do you find the tangent?
The equation of the tangent line can be found using the formula y – y1 = m (x – x1), where m is the slope and (x1, y1) is the coordinate points of the line.
What is the Tangent of a Circle?
A tangent to a circle is a line which intersects the circle at only one point. The common point between the tangent and the circle are called the point of contact.
How many tangents can be found in a circle?
It is to be noted that there can one and only one tangent through any given point on the circle. Any other line through a point on the circle other than the tangent at that point would intersect the circle at two points. This can be easily seen from the following figure. are a few lines passing through the point , where is a point on the circle.
What is a line that touches a circle at one point called?
and are the points on the circle; is a chord of the circle. (2) Line touches the circle exactly at one point, . Such a line is called the tangent to the circle. (3) Line does not touch the circle at any point and is referred to as a non-intersecting line.
What is the distance between a circle and its center called?
The fixed point is called the center of the circle and the distance between any point on the circle and its center is called the radius .
Is a tangent a secant?
Hence, only is a tangent and are secants to the circle. Every secant has a corresponding chord to the circle. Therefore, a tangent can be considered as a special case ...
What is the tangent of a circle?
A tangent to a circle is a straight line that touches the circle at one point, called the point of tangency. At the point of tangency, the tangent of the circle is perpendicular to the radius.
How many points does a tangent connect to a circle?
A chord and a secant connect only two points on the circle. A tangent connects with only one point on a circle.
What is the second theorem of tangents?
It states that, if two tangents of the same circle are drawn from a common point outside the circle, the two tangents are congruent.
What is the square of the length of the tangent segment?
Though it may sound like the sorcery of aliens, that formula means the square of the length of the tangent segment is equal to the product of the secant length beyond the circle times the length of the whole secant.
How many circles are in a crop circle?
You can also surround your first crop circle with six circles of the same diameter as the first. This forms a crop circle nest of seven circles, with each outer circle touching exactly three other circles, and the original center circle touching exactly six circles:
What is the difference between radius and secant?
radius (the distance from the center to the circle) chord (a line segment from the circle to another point on the circle without going through the center) secant (a line passing through two points of the circle) diameter (a chord passing through the center) circumference (the distance around the circle itself.
Why do crop circles appear?
Crop circles almost always "appear" very close to roads and show some signs of tangents, which is why most researchers say they are made by human pranksters. The word "tangent" comes from a Latin term meaning "to touch," because a tangent just barely touches a circle.
How many tangents are there in a circle?
For two circles, there are generally four distinct lines that are tangent to both ( bitangent) – if the two circles are outside each other – but in degenerate cases there may be any number between zero and four bitangent lines; these are addressed below. For two of these, the external tangent lines, the circles fall on the same side of the line; for the two others, the internal tangent lines, the circles fall on opposite sides of the line. The external tangent lines intersect in the external homothetic center, whereas the internal tangent lines intersect at the internal homothetic center. Both the external and internal homothetic centers lie on the line of centers (the line connecting the centers of the two circles), closer to the center of the smaller circle: the internal center is in the segment between the two circles, while the external center is not between the points, but rather outside, on the side of the center of the smaller circle. If the two circles have equal radius, there are still four bitangents, but the external tangent lines are parallel and there is no external center in the affine plane; in the projective plane, the external homothetic center lies at the point at infinity corresponding to the slope of these lines.
How to draw tangent lines?
Another method to construct the tangent lines to a point P external to the circle using only a straightedge : 1 Draw any three different lines through the given point P that intersect the circle twice. 2 Let#N#A 1 , A 2 , B 1 , B 2 , C 1 , C 2 {displaystyle A_ {1},A_ {2},B_ {1},B_ {2},C_ {1},C_ {2}}#N#be the six intersection points, with the same letter corresponding to the same line and the index 1 corresponding to the point closer to P. 3 Let D be the point where the lines#N#A 1 B 2 {displaystyle A_ {1}B_ {2}}#N#and#N#A 2 B 1 {displaystyle A_ {2}B_ {1}}#N#intersect, 4 Similarly E for the lines#N#B 1 C 2 {displaystyle B_ {1}C_ {2}}#N#and#N#B 2 C 1 {displaystyle B_ {2}C_ {1}}#N#. 5 Draw a line through D and E. 6 This line meets the circle at two points, F and G. 7 The tangents are the lines PF and PG.
How to find angle of tangent line?
Geometrically this corresponds to computing the angle formed by the tangent lines and the line of centers, and then using that to rotate the equation for the line of centers to yield an equation for the tangent line. The angle is computed by computing the trigonometric functions of a right triangle whose vertices are the (external) homothetic center, a center of a circle, and a tangent point; the hypotenuse lies on the tangent line, the radius is opposite the angle, and the adjacent side lies on the line of centers.
How to construct bitangent lines?
The bitangent lines can be constructed either by constructing the homothetic centers, as described at that article, and then constructing the tangent lines through the homothetic center that is tangent to one circle, by one of the methods described above. The resulting line will then be tangent to the other circle as well. Alternatively, the tangent lines and tangent points can be constructed more directly, as detailed below. Note that in degenerate cases these constructions break down; to simplify exposition this is not discussed in this section, but a form of the construction can work in limit cases (e.g., two circles tangent at one point).
What is the difference between secant and tangent lines?
For comparison, secant lines intersect a circle at two points, whereas another line may not intersect a circle at all. This property of tangent lines is preserved under many geometrical transformations, such as scalings, rotation, translations, inversions, and map projections. In technical language, these transformations do not change the incidence structure of the tangent line and circle, even though the line and circle may be deformed.
How many bitangents are there in a projective plane?
If the two circles have equal radius, there are still four bitangents, but the external tangent lines are parallel and there is no external center in the affine plane; in the projective plane, the external homothetic center lies at the point at infinity corresponding to the slope of these lines.
What is a tangential polygon?
A tangential polygon is a polygon each of whose sides is tangent to a particular circle, called its incircle. Every triangle is a tangential polygon, as is every regular polygon of any number of sides; in addition, for every number of polygon sides there are an infinite number of non- congruent tangential polygons.
What is the tangent of a circle?
The tangent of a circle always forms a 90 degree, or right angle, with the radius of the circle at that point. This tangent line is a geometric concept and should not be confused with the tangent of an angle from trigonometry. In trigonometry, the tangent, abbreviated tan, is the ratio of the sides of a triangle that are opposite ...
What does tangent to the x axis mean?
What Does "tangent to the X Axis" Mean? A tangent line is a line that touches but does not cross the graph of a function at a specific point. If a graph is tangent to the x-axis, the graph touches but does not cross the x-axis at some point on the graph.
What is the first derivative of a tangent line?
The tangent line is also an important concept in calculus. The first derivative in calculus is the slope of the tangent line at a specific point. This slope represents the instantaneous rate of change in a graph at that point.
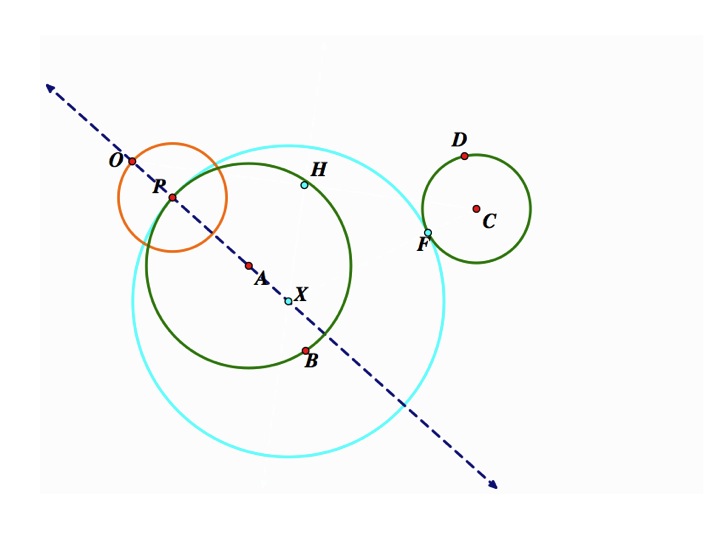
Overview
Tangent lines to one circle
A tangent line t to a circle C intersects the circle at a single point T. For comparison, secant lines intersect a circle at two points, whereas another line may not intersect a circle at all. This property of tangent lines is preserved under many geometrical transformations, such as scalings, rotation, translations, inversions, and map projections. In technical language, these transformations …
Tangent lines to two circles
For two circles, there are generally four distinct lines that are tangent to both (bitangent) – if the two circles are outside each other – but in degenerate cases there may be any number between zero and four bitangent lines; these are addressed below. For two of these, the external tangent lines, the circles fall on the same side of the line; for the two others, the internal tangent lines, the circl…
Tangent lines to three circles: Monge's theorem
For three circles denoted by C1, C2, and C3, there are three pairs of circles (C1C2, C2C3, and C1C3). Since each pair of circles has two homothetic centers, there are six homothetic centers altogether. Gaspard Monge showed in the early 19th century that these six points lie on four lines, each line having three collinear points.
Problem of Apollonius
Many special cases of Apollonius's problem involve finding a circle that is tangent to one or more lines. The simplest of these is to construct circles that are tangent to three given lines (the LLL problem). To solve this problem, the center of any such circle must lie on an angle bisector of any pair of the lines; there are two angle-bisecting lines for every intersection of two lines. The intersections of these angle bisectors give the centers of solution circles. There are four such cir…
Generalizations
The concept of a tangent line to one or more circles can be generalized in several ways. First, the conjugate relationship between tangent points and tangent lines can be generalized to pole points and polar lines, in which the pole points may be anywhere, not only on the circumference of the circle. Second, the union of two circles is a special (reducible) case of a quartic plane curve, a…
External links
• Weisstein, Eric W. "Tangent lines to one circle". MathWorld.
• Weisstein, Eric W. "Tangent lines to two circles". MathWorld.