The distributive property of multiplication for 3rd-grade math makes it easier for students to solve bigger multiplication problems they wouldn't normally be able to solve. The property states that when a number is multiplied by the sum of two numbers, the first can be distributed to both of those and multiplied by each separately, then adding the two products together.
How do you use distributive property in multiplication?
These example problems that may help you to understand the power of the Distributive Property:
- 11 × (10 + 5) = ? 11 × ( 10 + 5) = ?
- 11(10 + 5) = ? 11 ( 10 + 5) = ?
- 11(10) + 11(5) = ? 11 ( 10) + 11 ( 5) = ?
- 110 + 55 = ? 110 + 55 = ?
How do you write a distributive property?
The procedure to use the distributive property calculator is as follows:
- Enter an expression of the form a (b+c) in the input field.
- Now click the button “Submit” to get the simplified expression.
- Finally, the simplification of the given expression will be displayed in a new window.
What does distributive property mean in math?
Number properties - Definition with Examples
- Number Properties
- Commutative property : The commutative property states that the numbers on which we perform the operation can be moved or swapped from their position without making any difference to the ...
- Associative Property: The associative property gets its name from the word “Associate” and it refers to the grouping of numbers.
What is the meaning distributive property of multiplication?
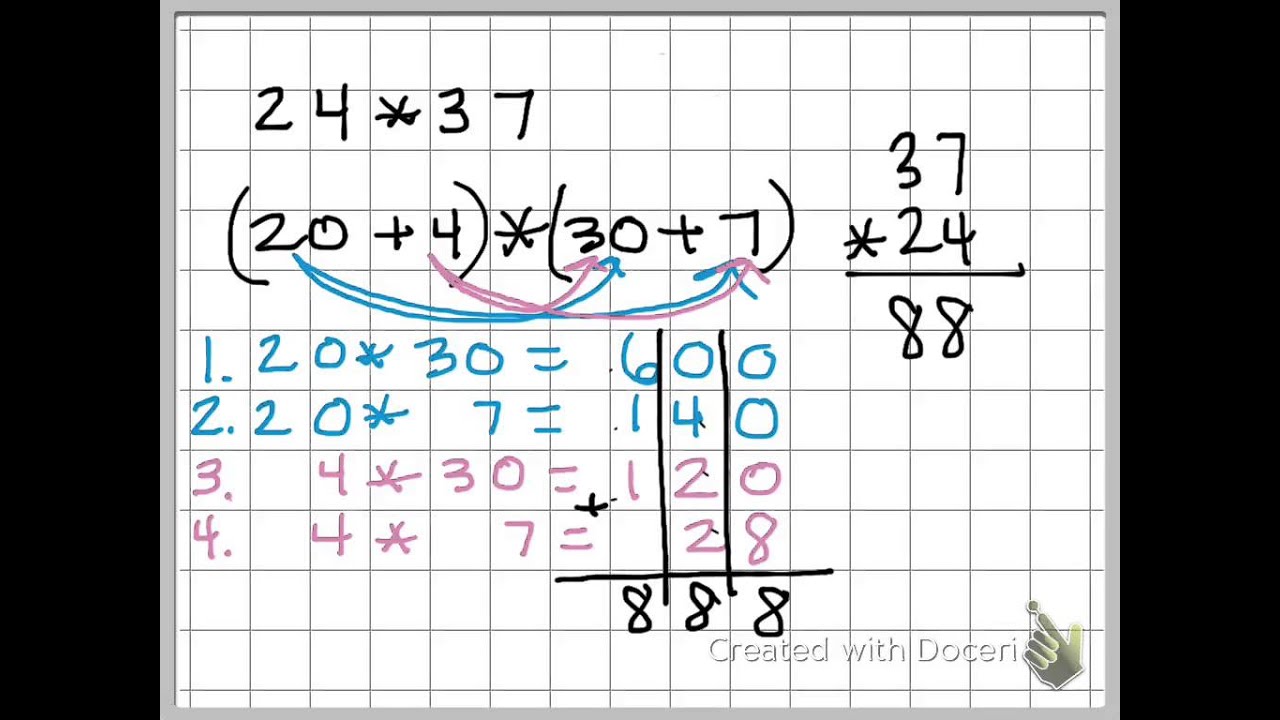
The distributive property of multiplication states that when a number is multiplied by the sum of two numbers, the first number can be distributed to both of those numbers and multiplied by each of them separately, then adding the two products together for the same result as multiplying the first number by the sum.
How do you explain distributive property to a child?
To “distribute” means to divide something or give a share or part of something. According to the distributive property, multiplying the sum of two or more addends by a number will give the same result as multiplying each addend individually by the number and then adding the products together.
What is a distributive property in multiplication?
The distributive property of multiplication states that when a number is multiplied by the sum of two numbers, the first number can be distributed to both of those numbers and multiplied by each of them separately, then adding the two products together for the same result as multiplying the first number by the sum.
What is distributive property with example?
It is used to solve expressions easily by distributing a number to the numbers given in brackets. For example, if we apply the distributive property of multiplication to solve the expression: 4(2 + 4), we would solve it in the following way: 4(2 + 4) = (4 × 2) + (4 × 4) = 8 + 16 = 24.
What is distributive property in math?
The distributive property tells us how to solve expressions in the form of a(b + c). The distributive property is sometimes called the distributive law of multiplication and division.
How do you teach the distributive property of multiplication?
Distributive property with exponentsExpand the equation.Multiply (distribute) the first numbers of each set, outer numbers of each set, inner numbers of each set, and the last numbers of each set.Combine like terms.Solve the equation and simplify, if needed.
What is distributive property look like?
Distributive Property Formally, they write this property as "a(b + c) = ab + ac". In numbers, this means, for example, that 2(3 + 4) = 2×3 + 2×4.
How do you do the distributive property step by step?
6:4411:53Algebra Basics: The Distributive Property - Math Antics - YouTubeYouTubeStart of suggested clipEnd of suggested clipFour times three x which is twelve x since 4 times 3 is 12. And 4 times the negative 5 which isMoreFour times three x which is twelve x since 4 times 3 is 12. And 4 times the negative 5 which is negative 20.. So the equivalent distributive. Form is 4x squared plus 12x minus 20..
What is a good example of distributive property?
The distributive property of multiplication over addition can be used when you multiply a number by a sum. For example, suppose you want to multiply 3 by the sum of 10 + 2. 3(10 + 2) = ? According to this property, you can add the numbers and then multiply by 3.
How to use distributive property in multiplication?
You can use the distributive property of multiplication to rewrite expression by distributing or breaking down a factor as a sum or difference of two numbers. Here, for instance, calculating 8 × 27 can made easier by breaking down 27 as 20 + 7 or 30 − 3.
What does it mean to divide something?
To “distribute” means to divide something or give a share or part of something. According to the distributive property, multiplying the sum of two or more addends by a number will give the same result as multiplying each addend individually by the number and then adding the products together.
Is division the inverse of multiplication?
Fun Facts. Even though division is the inverse of multiplication, the distributive law only holds true in case of division, when the dividend is distribu ted or broken down. For instance, using the distributive law for 132 6, 132 can be broken down as 60 60 + 12, thus making division easier. However, 132 (4 2) will give you the wrong result.
How Did I Teach the Distributive Property of Multiplication?
Normally, I use a mix of approaches to teaching math. Sometimes I use Lesson Inquiry. Sometimes I use Direct Instruction . So for this lesson, I decided on a hybrid approach. I would teach the Distributive Property of Multiplication using a hands-on, inquiry, guided questioning approach COMBINED with some direct instruction with steps.
Which Parts of the Distributive Property of Multiplication Present the Most Difficulties?
That’s an easy question to answer. ALL OF IT. Think about it. It is unlike any of the other Properties of Multiplication, so there’s no building on that. It involves notation that they are usually unfamiliar with or rarely use: mixed operations and parentheses in the same number sentence.
What is the Answer Then?
Break it down into steps. Don’t rush to get to teach the Distributive Property of Multiplication number sentence first day! Slow it down so the students understand WHY we break apart an array, then ADD the two parts back to get a final product.
Breaking Apart Arrays for the Distributive Property of Multiplication
You would think that breaking apart an array is an easy step. Not really. Arrays can be broken apart in many ways: vertically or horizontally. But is there a way to break apart an array to make the process more efficient or easier? YES!
Why Is This Important to Know?
Breaking apart an array at five means I will eventually multiply by five and almost all students can count by fives or know their five facts.
Adding the Products in the Distributive Property of Multiplication
If you were to ask students about long division and why do they bring down the next number or why do you multiply or why do you subtract, how many could explain the reason? So how do you expect third graders to explain or understand why there is an ADDITION sign in a Distributive Property of Multiplication sentence?
Day TWO, Introducing the Steps
On day two, I began by reviewing what we learned the day before. We started with a quick warmup with an anchor chart partially prepared. Using a piece of yarn, I moved the yarn around the array splitting it in different ways, until we agreed that splitting it at the five mark was the best solution.