
How to solve sum and difference identities?
sin (x – y) = (sin x)(cos y) – (cos x)(sin y) Æ Difference Identity for Sine. Sum Identity for Sine • To obtain the sum identity for sine, we replace y with –y in the difference identity for cosine equation, as follows: sin (x – (-y)) = (sin x)(cos (-y)) – (cos x)(sin (-y)) ↓ sin (x + y ) = (sin x)(cos y) + (cos x)(sin y)
What is an example of a sum formula?
Example 1: Simple Example with Defaults. Function: =SUMIF(B2:B6,100) Result: $200. Explanation: This SUMIF function, residing in C3 on the worksheet below, sums the values in cell range B2:B6 that equal 100. The default operator is equals and is not written.
What are summation formulas?
- PROBLEM 1 : Evaluate . Click HERE to see a detailed solution to problem 1.
- PROBLEM 2 : Evaluate . Click HERE to see a detailed solution to problem 2.
- PROBLEM 3 : Evaluate . ...
- PROBLEM 4 : Evaluate . ...
- PROBLEM 5 : Evaluate . ...
- PROBLEM 6 : Evaluate . ...
- PROBLEM 7 : Evaluate . ...
- PROBLEM 8 : Evaluate . ...
- PROBLEM 9 : Evaluate . ...
- PROBLEM 10 : Prove that . ...
What is the difference between formula and formulas?
Three major types are available:
- Cow milk protein-based formulas. Most infant formula is made with cow's milk that's been altered to resemble breast milk. ...
- Soy-based formulas. Soy-based formulas can be useful if you want to exclude animal proteins from your child's diet. ...
- Protein hydrolysate formulas. ...
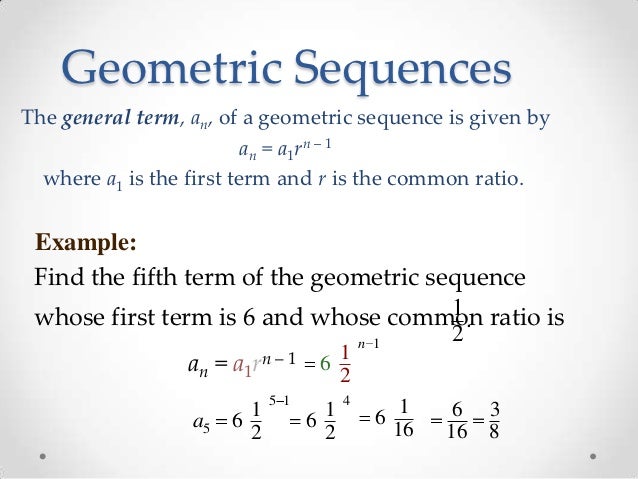
Why do we use sum and difference formulas?
We can use the sum and difference formulas to identify the sum or difference of angles when the ratio of sine, cosine, or tangent is provided for each of the individual angles. To do so, we construct what is called a reference triangle to help find each component of the sum and difference formulas.
How do you use the difference formula?
17:3621:44So the difference between 180 and 120 is 60. So the reference angle is 60. So using the 30-60-90MoreSo the difference between 180 and 120 is 60. So the reference angle is 60. So using the 30-60-90 triangle we can find out tan 60 which will help us to calculate 10 120.
What is the sum and difference formula for tangent?
0:506:36The sum and difference formula for tangent or the sum formula is tangent of u plus tangent of vMoreThe sum and difference formula for tangent or the sum formula is tangent of u plus tangent of v divided by 1 minus tangent of u times tangent of e.
What are the sum and difference formulas for cosine?
Using the Sum and Difference Formulas for CosineSum formula for cosinecos ( α + β ) = cos α cos β − sin α sin β cos ( α + β ) = cos α cos β − sin α sin βDifference formula for cosinecos ( α − β ) = cos α cos β + sin α sin β cos ( α − β ) = cos α cos β + sin α sin β
How do you do sum and difference in trigonometry?
2:5921:37So first we need to find two angles that either add or subtract to 15.. Two common angles that weMoreSo first we need to find two angles that either add or subtract to 15.. Two common angles that we know on a unit circle are 45 and 30. 45 minus 30 is 15..
What is the sum and difference pattern?
0:101:48And difference pattern. You'll notice that in the first binomial. You have the sum right here and inMoreAnd difference pattern. You'll notice that in the first binomial. You have the sum right here and in the second binomial you have a difference. So hence the name sum. And difference pattern.
What is the sum difference identity?
We can use the sum and difference formulas to identify the sum or difference of angles when the ratio of sine, cosine, or tangent is provided for each of the individual angles. To do so, we construct what is called a reference triangle to help find each component of the sum and difference formulas.
How do you evaluate the sum and difference of a function?
3:259:16Function we could say t of x to be equal to the difference of our two functions and the way weMoreFunction we could say t of x to be equal to the difference of our two functions and the way we evaluate t of x is we evaluate f of x. And then we subtract off g evaluated at x.
How do you remember the sum and difference identities?
0:214:31The way I remember is through this little chant. That I heard in high school.MoreThe way I remember is through this little chant. That I heard in high school.
What is sum difference?
SUM – The sum is the result of adding two or more numbers. DIFFERENCE – The difference of two numbers is the result of subtracting these two numbers.
What is the difference formula for cos?
Cosine-difference formula: cos ( α − β ) = cos α ⋅ cos β + sin α ⋅ sin β .
Addition and Difference Formula for Cosine Proof
Let variables u and v be any real numbers. Let A be the point (1, 0), and then use u and v to locate the points B (x 1, y 1 ), C (x 2, y 2 ), and D (x 3, y 3) on the unit circle as indicated. For convenience, we assume 0 < v < u < 2π, though the results we get are valid for all actual values of variables u and v.
Addition and Difference Formula for Sine Proof
We can prove the addition formula for sine using the cofunction identities and the subtraction formula for cosine.
Addition and Difference Formula for Tangent Proof
In proving the addition formula for the tangent, let us use the primary identity for tangent wherein tangent is equal to the sine divided by cosine.
Example 1: Using the Subtraction Formula for Cosine
Find the exact value of cos (15°) by using the factual information that 15° = 60° - 45°.
Example 3: Applying Both Sine and Cosine Sum and Difference Formulas
Find the exact value for sin (12°) cos (42°) - cos (12°) sin (42°) using the sine and cosine using the sum and difference formulas.
Example 7: Simplifying a Complex Equation to a Single Term
Use the sum and difference identities to simplify the given expression to a single term. cos (3x) cos (2y) + sin (3x) sin (2y)
Example 8: Simplifying a Given Expression to a Single Term
Use the sum and difference identities to simplify the given expression to a single term. sin (3) cos (1.2) - cos (3) sin (1.2)
What are Sum and Difference Formulas?
We have six main sum and difference formulas for the trigonometric functions including the sine function, cosine function, and tangent function. These formulas help us to evaluate the value of the trigonometric functions at angles which can be expressed as the sum or difference of special angles 0°, 30°, 45°, 60°, 90°, and 180°.
Proof of Sum and Difference Identities
As we discussed in the previous section, we have mainly six sum and difference identities of the sine function, cosine function, and tangent function. Now, we will prove them one by one. Consider a unit circle in a coordinate plane. We know that the coordinates of points on the unit circle are given by, (cosθ, sinθ).
Sum and Difference Identities Table
In the previous section, we derived the formulas of all the sum and difference identities of the trigonometric functions sine, cosine, and tangent. Now, let us summarize these formulas in the table below for a quick revision.
How to Apply Sum and Difference Formulas
Now that we have understood the sum and difference formulas of trigonometric functions, let us now use these formulas to understand their application. We can simply substitute the values of the angles into the formulas or express the given angle as a sum or difference of special angles to find their values.
Examples Using Sum and Difference Formulas
Example 1: Find the values of sin 75° and tan 75° using the sum and difference identities.
Trigonometric functions
Although trigonometry is a broad topic with loads of generalizations, we should start where all things began: in ancient Greece.
Sine addition formula, cos addition formula
The sum and difference formulas allow us to calculate the value of a trigonometric function by describing it in terms of similar functions but with different arguments. In essence, we take the angle that we got initially and decompose it into a sum or difference of two other angles.
Other sum and difference trig identities
Although the cos and sine addition formulas are the most common, the other four trigonometric functions deserve some love too. Below, you can find the sum and difference trig identities for all of them. For completeness, we mention the ones from the previous section as well.
Example: using the sum and difference identities calculator
Say that you're a teacher in between two school years and that you need to plan how to prepare the classroom for the pandemic times. In other words, you must figure out how to arrange the desks so that you keep to the new standards connected with social distancing.
/sumsquares-56e618233df78c5ba0574656.jpg)
Sum and Difference Formulas For Cosine
Sum and Difference Formulas For Sine
- Next, we will prove the sum and difference for the sine function using the cofunction identities of trigonometry. We know that sin A = cos (π/2 - A) and cos A = sin (π/2 - A). So, we have sin (α + β)= cos [π/2 - (α + β)] = cos [π/2 - α - β] = cos [(π/2 - α) - β] = cos (π/2 - α) cos β + sin (π/2 - α) sin β = sin α cos β + cos α sin β ⇒ sin (α + β) =...
Sum and Difference Formulas For Tangent
- In this section, we will prove the sum and difference identities for the tangent function. We know that tangent function can be written as the ratio of the sine and cosine, that is, tan A = sin A / cos A. So, we can write tan (α + β) as, tan (α + β)= sin (α + β) / cos (α + β) = (sin α cos β + cos α sin β) / (cos α cos β - sin α sin β) --- [Using sin (α + β) = sin α cos β + cos α sin β and cos (α + β) = cos α …