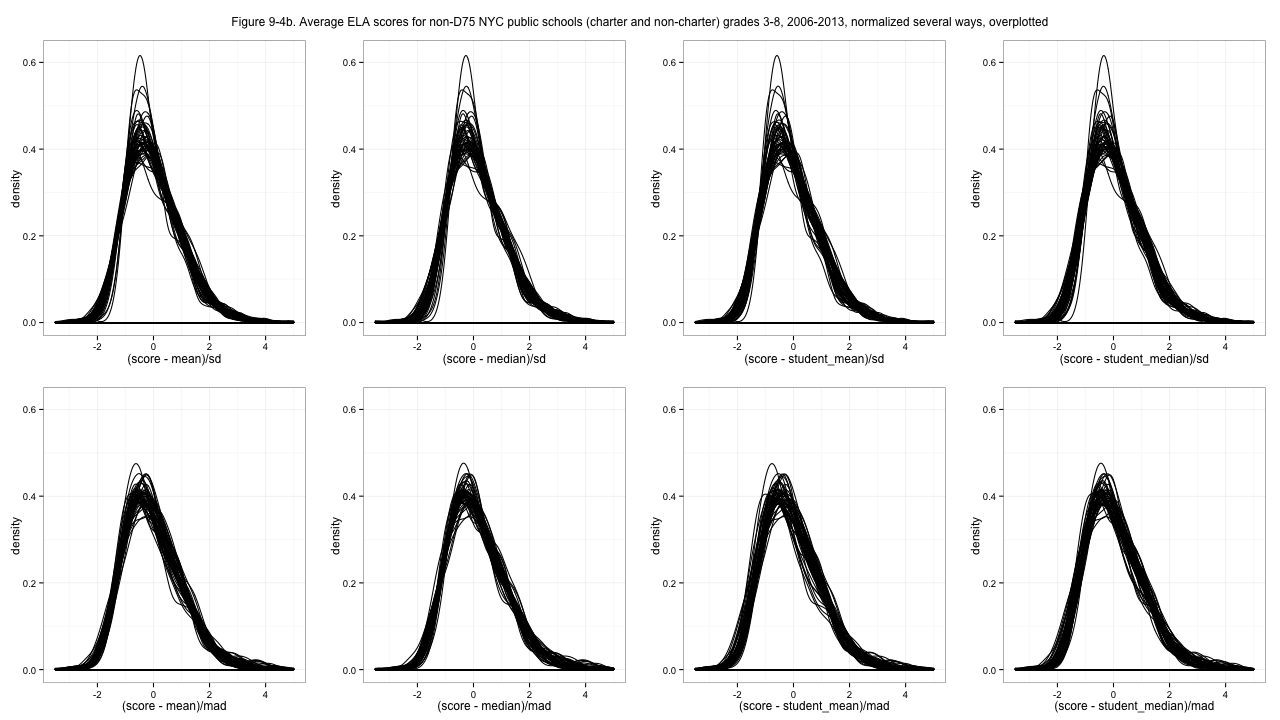
Why is standard deviation better than mean absolute deviation? The difference between the two norms is that the standard deviation is calculating the square of the difference whereas the mean absolute deviation is only looking at the absolute difference. Hence large outliers will create a higher dispersion when using the standard deviation instead of the other method.
How to calculate the mean absolute deviation?
Summing Up!
- The mean absolute deviation is a measure of dispersion or variation in a set of numerical data.
- It is the average distance between each data point of a data set and the mean.
- As MAD measures the spread of a data set, it indicates if the mean is the correct representation or misrepresentation of each data in the set.
What is the relationship between mean and standard deviation?
The standard deviation calculation is based on a couple of steps:
- Find the average closing price (mean) for the periods under consideration (the default setting is 20 periods)
- Find the deviation for each period (closing price minus average price)
- Find the square for each deviation.
- Add the squared deviations.
Is mean deviation the same as mean absolute difference?
That is to say, if X is a random variable and c is a constant:
- MD ( X + c) = MD ( X ),
- MD (− X) = MD ( X ), and
- MD ( c X) = | c | MD ( X ).
How do you interpret the mean absolute deviation?
The Mean Absolute Deviation (MAD) of a set of data is the average distance between each data value and the mean.The mean absolute deviation is the "average" of the "positive distances" of each point from the mean.The larger the MAD, the greater variability there is in the data (the data is more spread out).

Why is it better to use standard deviation?
A high standard deviation shows that the data is widely spread (less reliable) and a low standard deviation shows that the data are clustered closely around the mean (more reliable).
What is the difference between mean absolute deviation and standard deviation?
The MAD is simply the mean of these nonnegative (absolute) deviations. The standard deviation is the square root of the sum of the squares of the deviations, divided by (n-1). This measure also results in a value that in some sense represents the “typical” difference between each data point and the mean.
How do you find the mean absolute deviation from the standard deviation?
5:428:16What is Standard Deviation and Mean Absolute Deviation - YouTubeYouTubeStart of suggested clipEnd of suggested clipSo see here the number is 3.55 here the number is 6.02. So higher the number. Means the data pointsMoreSo see here the number is 3.55 here the number is 6.02. So higher the number. Means the data points are spread out more they are more widely spread out this square root is called standard deviation
What is the mean absolute deviation used for?
variationMean absolute deviation (MAD) of a data set is the average distance between each data value and the mean. Mean absolute deviation is a way to describe variation in a data set. Mean absolute deviation helps us get a sense of how "spread out" the values in a data set are.
What is the relation between mean deviation and standard deviation?
In a discrete series (when all values are not same), the relationship between mean deviation (M.D) about mean and standard deviation (S.D) is. (a) M. D=S.
How do you convert mean absolute deviation to standard deviation?
What is this? Mean Absolute Deviation = (|3-11| + |5-11| + |6-11| + |8-11| + |11-11| + |14-11| + |17-11| + |24-11|) / 8 = 5.5. And we would calculate the standard deviation as: Standard Deviation = √((3-11)2 + (5-11)2 + (6-11)2 + (8-11)2 + (11-11)2 + (14-11)2 + (17-11)2 + (24-11)2) / 8) = 6.595.
What is mean absolute deviation?
I mean, Mean Absolute Deviation is a very intuitive measurement of dispersion. It tells you exact average distant that each value deviates from their mean. Standard Deviation, on the other hand, makes the result more sensitive to outliers. Why we need this sensitivity.
Is standard deviation a measurement of dispersion?
Almost all textbooks and papers are using Standard Deviation as a measurement of dispersion. And of course, almost all the models are built based on Standard Deviation. But I don't understand how Standard Deviation has gained such popularity. I mean, Mean Absolute Deviation is a very intuitive measurement of dispersion.
Why are absolute deviations not equal to square root?
Therefore the sum of absolute deviations is not equal to the square root of the sum of squared deviations, even though the absolute function can be represented as the square function followed by a square root:
Can you use standard deviation if data is not distributed?
If your data is not normally distributed, you can still use the standard deviation, but you should be careful with the interpretation of the results. Finally you should know that both measures of dispersion are particular cases of the Minkowski distance, for p=1 and p=2.
Is standard deviation a distortion?
mathematical properties that make it more useful in statistics", it is, in fact, a distortion of the concept of variance from a mean, since it gives extra weighting to data points far from the mean.
Is standard deviation the same as Pythagorean theorem?
If you look at it closely, the formula for (population) Standard Deviation is basically the same as the Pythagorean Theorem, but with a lot more than two dimensions (and using distance from each point to the mean as the distance in each dimension).
Why is (3,1) farther from origin than (2,2)?
But that happens in the real world’s flat geometry as well – (3,1) is farther from origin as compared to (2,2), despite both being equally distant from the axes – only because (3,1) contains a bigger number. This is a direct side-effect of the Pythagoras’ principle which the Euclidean geometry follows.
Is A and B a point in n-D?
Both A and B are just sets of numbers or observations. They are not points in an n-D world. But, if we visualize each deviation as distance from the mean, and further, if, instead of visualizing all the deviations of a given set as distances in the same dimension, we picture each of them denoting the length of a unique dimension in an n-D world, so that each of A and B becomes a distinct point in an n-D world (here 3D), we at once, see that B is farther away from its mean as compared to how far A is from its mean, though MAD is same for both A and B. This is what is denoted by standard deviation.
What is the difference between standard deviation and mean?
Standard deviation is the deviation from the mean, and a standard deviation is nothing but the square root of the variance. Mean is an average of all sets of data available with an investor or company. The standard deviation used for measuring the volatility of a stock. So both Standard Deviation vs Mean plays a vital role in the field of finance. Standard deviation is easier to picture and apply. So both the tool used for strategies which can be used for application in trading and investment activity.
Why is standard deviation important?
In finance standard deviation is a statistical measurement, when its applied to the annual rate of return of an investment. It sheds the volatility of historical volatility of that investment.
What is the standard deviation used to measure the volatility of a stock?
The standard deviation used to measure the volatility of a stock, the higher the standard deviation higher the volatility of a stock. The blue-chip stock has a low standard deviation so that has low volatility. It is the simplest form the mean is an average of all data points.
Why is standard deviation used in stock trading?
Standard deviation is often used in creating for trading and investing because it helps to measure the volatility of stock prices and predict the future trend.
What is standard deviation in statistics?
Standard deviation is statistics that basically measure the distance from the mean, and calculated as the square root of variance by determination between each data point relative to the mean. Standard deviation plays a very important role in the world of finance.
What is mean in statistics?
Mean is a simple mathematical average of the set of two or more numbers. There are different types for calculation of mean, including the arithmetic mean method, which uses sums of all numbers in the series, and the geometric mean method. The simple method of mean is to make the total of all data and divide it by the number of data, then we reach to mean. Mean is nothing but the simple average of data. The mean is a statistical indicator which is used to judge the performance of a stock over a period of time through its earning over a period of time by assessing its fundamental such as P/E ratio, balance sheet, and the portfolio by estimating its average rate of return over a period of time. So both Standard Deviation vs Mean term is used in statistics for calculation purposes.
What is the simplest form of mean?
It is the simplest form the mean is an average of all data points. Standard deviation is one of the key fundamental risk measures that analytics, portfolio managers, wealth management, and financial planner used. Standard deviation is calculated based on the mean.
Why is standard deviation used?
Standard Deviation Versus Average Deviation. Standard deviation is often used to measure the volatility of returns from investment funds or strategies because it can help measure volatility. Higher volatility is generally associated with higher risk of losses, so investors want to see higher returns from funds that generate higher volatility.
What is mean absolute deviation?
The average deviation, or mean absolute deviation, is calculated similarly to standard deviation, but it uses absolute values instead of squares to circumvent the issue of negative differences between the data points and their means. To calculate the average deviation:
How to calculate standard deviation?
Standard deviation is the most common measure of variability and is frequently used to determine the volatility of markets, financial instruments, and investment returns. To calculate the standard deviation : 1 Find the mean, or average, of the data points by adding them and dividing the total by the number of data points. 2 Subtract the mean from each data point and square the difference of each result. 3 Find the mean those squared differences and then the square root of the mean.
What is mean average?
The mean average, or mean absolute deviation, is considered the closest alternative to standard deviation. It is also used to gauge volatility in markets and financial instruments, but it is used less frequently than standard deviation. Generally, according to mathematicians, when a data set is of normal distribution — that is, ...
When is standard deviation considered the most appropriate measure of variability?
Standard deviation is considered the most appropriate measure of variability when using a population sample, when the mean is the best measure of center, and when the distribution of data is normal.
What are the two ways to measure variability?
Two of the most popular ways to measure variability or volatility in a set of data are standard deviation and average deviation, also known as mean absolute deviation. Though the two measurements are similar, they are calculated differently and offer slightly different views of data.